问题1:构建一个四边形ABCD,其中AB = 4.4厘米,BC = 4厘米,CD = 6.4厘米,DA = 3.8厘米,BD = 6.6厘米?
解决方案:
Given: AB = 4.4 cm, BC = 4 cm, CD = 6.4 cm, DA = 3.8 cm and BD = 6.6 cm.
Let’s divide the quadrilateral into two triangles,
i.e., ΔABD and ΔBCD
Steps for the construction of a quadrilateral:
Step 1: Applying SSS congruency rule, Draw line BD of length 6.6 cm.
Step 2: Now, cut an arc with B as the centre and radius BC = 4 cm. Similarly by taking D as centre and radius CD = 6.4 cm.
Step 3: Join the intersection point from B and D and mark it as C.
Step 4: Now for vertex A, cut an arc by taking B as the centre and radius BA = 4.4 cm. Similarly by taking D as centre and radius DA = 3.8 cm.
Step 5: Join the intersection point from B and D and mark it as A.

问题2:构建四边形ABCD,其中AB = BC = 5.5 cm,CD = 4 cm,DA = 6.3 cm,AC = 9.4 cm测量BD?
解决方案:
Given: AB = BC = 5.5 cm, CD = 4 cm, DA = 6.3 cm, AC = 9.4 cm
We have to measure BD.
Steps for construction of the quadrilateral:
Step 1: Draw a line segment AB = 5.5 cm
Step 2: With B as centre and radius BC = 5.5 cm cut an arc. Mark the intersection point as C.
Step 3: With A as centre and radius AC = 9.4 cm cut an arc to intersect at point C.
Step 4: With C as centre and radius CD = 4 cm cut an arc. Mark the intersection point as D.
Step 5: With A as centre and radius AD = 6.3 cm cut an arc to intersect at point D.
Step 6: Now join BC, CD, and AD
Hence, the Measure of BD is 5.1 cm.

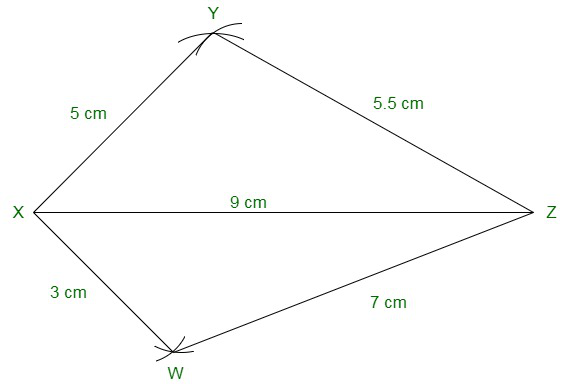
问题3:构造一个四边形XYZW,其中XY = 5厘米,YZ = 6厘米,ZW = 7厘米,WX = 3厘米,XZ = 9厘米?
解决方案:
We have, XY = 5cm, YZ = 6cm, ZW = 7cm, WX = 3cm and XZ = 9cm.
Steps to construct a quadrilateral:
Step 1: Draw a line segment XZ with length = 9 cm.
Step 2: Taking X as the centre mark an arc of radius XY = 5 cm. Taking Z as centre and radius ZY = 6 cm, repeat the procedure.
Step 3: Mark the intersection point of X and Z as Y.
Step 4: For vertex W, taking X as the centre mark an arc of radius XW = 3 cm. Taking Z as centre and radius ZW = 7 cm, repeat the procedure.
Step 5: Mark the intersection point of X and Z as W.
问题4:构造一个平行四边形PQRS,以使PQ = 5.2 cm,PR = 6.8 cm和QS = 8.2 cm?
解决方案:
We have, PQ = 5.2 cm, PR = 6.8 cm, and QS = 8.2 cm.
Steps to construct a parallelogram:
Step 1: Draw a line segment QS of length = 8.2 cm.
Step 2: Divide the line segment QS into two equal parts such that each one is = 4.1 cm. Mark the midpoint as O.
Step 3: Taking O as the centre, draw arcs of length 3.4 cm each on both the sides of the points O. Mark these points as P and R respectively
Step 4: Taking Q as a centre and radius QR = 5.2cm, draw an arc that intersects point R.
Step 5: Also, taking Q as a centre and radius QP = 5.2cm, again draw an arc that intersects point P.
Step 6: Join sides PQ, PS, QR, and RS to form a parallelogram.

问题5:构造一个菱形,菱形的边长6厘米,对角线长8厘米。测量另一个对角线?
解决方案:
In a rhombus, all the sides of a rhombus are equal and diagonals bisect each other.
We have a side 6 cm and one diagonal 8 cm.
Steps to construct a rhombus:
Step 1: Draw a line segment XZ of length 8 cm.
Step 2: Taking a radius = 6 cm, cut an arc with X as the centre. Repeat the procedure with Z as the centre and radius = 6 cm.
Step 3: Mark the intersection point of X and Z as Y.
Step 4: Taking a radius = 6 cm, cut an arc with W as the centre. Repeat the procedure with Z as the centre and radius = 6 cm.
Step 5: Mark the intersection point of X and Z as W.
Step 6: Join the line segments XY, XW, XZ, and ZY to form a rhombus.

问题6:构造一个风筝ABCD,其中AB = 4厘米,BC = 4.9厘米,AC = 7.2厘米?
解决方案:
We have, AB = 4 cm, BC = 4.9 cm, AC = 7.2 cm.
Steps to construct a kite:
Step 1: Draw a line segment AC with length = 7.2 cm.
Step 2: Taking a radius of 4 cm and cut an arc by taking A as the centre. Also, taking C as the centre, repeat the procedure with radius = 4.9 cm.
Step 3: Label the intersection point of A and C and mark it as B.
Step 4: Considering vertex D, draw an arc with A as the centre. Also, taking C as the centre, repeat the procedure with radius = 4.9 cm.
Step 5: Label the intersection point of A and C and mark it as D.

问题7:如果可能,构造四边形ABCD,假定AB = 6 cm,BC = 3.7 cm,CD = 5.7 cm,AD = 5.5 cm,BD = 6.1 cm。如果不能,请说明无法构造的原因?
解决方案:
We have, AB = 6 cm, BC = 3.7 cm, CD = 5.7 cm, AD = 5.5 cm and BD = 6.1 cm.
Steps to construct a quadrilateral:
Step 1: Draw a line segment AB with length = 6 cm.
Step 2: Taking A as the centre, draw an arc of a radius of 5.5 cm. Mark this point as D.
Step 3: Taking B as a centre, draw an arc of a radius of 6.1 cm intersecting point D.
Step 4: Also, with B as a centre, draw another arc of a radius of 3.7 cm. Mark this point as C.
Step 5: Taking D as a centre, draw an arc of a radius of 5.7 cm intersecting point C.
Step 6: Join the line segments, AD, BD, BC, and DC

问题8:如果可能,构造一个四边形ABCD,其中AB = 6 cm,BC = 7 cm,CD = 3 cm,AD = 5.5 cm,AC = 11 cm。如果不能,请说明无法构造的原因。 (不可能,因为在三角形ACD中,AD + CD
解决方案:
We have, AB = 6 cm, BC = 7 cm, CD = 3 cm, AD = 5.5 cm and AC = 11 cm.
Since, we know, In any triangle the sum of the length of its two sides must be greater than that of the third side, therefore a quadrilateral with such dimensions cannot be constructed.
Considering in triangle ACD,
AD + CD = 5.5 + 3 = 8.5 cm
Given, AC = 11 cm
Now, AD + CD < AC which is not possible.
Hence, the construction cannot be performed.