第 11 类 RD Sharma 解决方案 - 第 18 章二项式定理 - 练习 18.2 |设置 2

问题 14. 找出展开式中的中间项：

(i) (3x – x ³ /6) ⁹

解决方案：

We have,

(3x – x³/6)⁹ where, n = 9 (odd number).

So, the middle terms are ((n + 1)/2) = ((9 + 1)/2) = 10/2 = 5 and 

((n + 1)/2 + 1) = ((9 + 1)/2 + 1) = (10/2 + 1) = (5 + 1) = 6

The terms are 5^th and 6^th.

Now,

T₅ = T₄₊₁

= ⁹C₄ (3x)^9-4 (x³/6)⁴

= $\frac{9×8×7×6}{5×4×3×2×1}×27×9×\frac{x^{17}}{36×36}$

= $\frac{189x^{17}}{8}$

And, T₆ = T₅₊₁

= ⁹C₅ (3x)^9-5 (x³/6)⁵

= $-\frac{9×8×7×6}{5×4×3×2×1}×81×9×\frac{x^{19}}{216×36}$

= $-\frac{21x^{19}}{16}$

(ii) (2x ² – 1/x) ⁷

解决方案：

We have,

(2x² – 1/x)⁷ where, n = 7 (odd number).

So the middle terms are ((n + 1)/2) = ((7 + 1)/2) = 8/2 = 4 and

((n + 1)/2 + 1) = ((7 + 1)/2 + 1) = (8/2 + 1) = (4 + 1) = 5

The terms are 4^th and 5^th.

Now, 

T₄ = T₃₊₁

= ⁷C₃ (2x²)^7-3 (-1/x)³

= -\frac{7×6×5}{3×2}×16x^8×\frac{1}{x^3}

= − 560 x⁵

And, T₅ = T₄₊₁

= ⁷C₄ (2x²)^7-4 (-1/x)⁴

= $\frac{7×6×5}{3×2}×8×x^6×\frac{1}{x^4}$

= 280 x²

(iii) (3x – 2/x ² ) ¹⁵

解决方案：

We have,

(3x – 2/x²)¹⁵ where, n = 15 (odd number)

So the middle terms are ((n + 1)/2) = ((15 + 1)/2) = 16/2 = 8 and

((n + 1)/2 + 1) = ((15 + 1)/2 + 1) = (16/2 + 1) = (8 + 1) = 9

The terms are 8^th and 9^th.

Now,

T₈ = T₇₊₁

= ¹⁵C₇ (3x)^15-7 (– 2/x²)⁷

= $-\frac{15×14×13×12×11×10×9}{7×6×5×4×3×2×1}×3^8×2^7×x^{8-14}$

= $\frac{-6435×3^8×2^7}{x^{6}}$

And, T₉ = T₈₊₁

= ¹⁵C₈ (3x)^15-8 (– 2/x²)⁸

= $-\frac{15×14×13×12×11×10×9}{7×6×5×4×3×2×1}×3^7×2^8×x^{7-16}$

= $\frac{6435×3^7×2^8}{x^{9}}$

(iv) (x ⁴ – 1/x ³ ) ¹¹

解决方案：

We have, (x⁴ – 1/x³)¹¹ where, n = 11 (odd number)

So the middle terms are ((n + 1)/2) = ((11 + 1)/2) = 12/2 = 6 and

((n + 1)/2 + 1) = ((11 + 1)/2 + 1) = (12/2 + 1) = (6 + 1) = 7

The terms are 6^th and 7^th.

Now,

T₆ = T₅₊₁

= ¹¹C₅ (x⁴)^11-5 (1/x³)⁵

= $- \frac{11 \times 10 \times 9 \times 8 \times 7}{5 \times 4 \times 3 \times 2} \times \left( x \right)^{24 - 15}$

= -462 x⁹

And, T₇ = T₆₊₁

= ¹¹C₆ (x⁴)^11-6 (1/x³)⁶

= $\frac{11 \times 10 \times 9 \times 8 \times 7}{5 \times 4 \times 3 \times 2} \left( x \right)^{20 - 18}$

= 462 x²

问题 15. 找出展开式中的中间项：

(i) (x – 1/x) ¹⁰

解决方案：

We have,

(x – 1/x)¹⁰ where, n = 10 (even number)

So the middle term is (n/2 + 1) = (10/2 + 1) = (5 + 1) = 6^th term

Now,

T₆ = T₅₊₁

= ¹⁰C₅ (x)^10-5 (–1/x)⁵

= $-\frac{10×9×8×7×6}{5×4×3×2×1}$

= −252

(ii) (1 – 2x + x ² ) ⁿ

解决方案：

We have, (1 – 2x + x²)ⁿ

= (1 – x)²ⁿ

Here, n is an even number.

So the middle terms is 2n/2 + 1 = (n + 1)^th term

Now, 

T_n+1 = ²ⁿC_n (-1)ⁿ xⁿ

= $\frac{(2n)!}{(n! )^2}( - 1 )^n x^n$

(iii) (1 + 3x + 3 x ² + x ³ ) ²ⁿ

解决方案：

We have, (1 + 3x + 3 x² + x³)²ⁿ

= (1 + x )⁶ⁿ

Here, n is an even number.

So the middle terms is (6n/2 + 1) = (3n + 1)^th term 

Now, 

T_3n+1 = ⁶ⁿC_3n x³ⁿ

= $\frac{(6n)!}{(3n! )^2} x^{3n}$

(iv) (2x – x ² /4) ⁹

解决方案：

We have,

(2x – x²/4)⁹ where, n = 9 (odd number)

So the middle terms are ((n + 1)/2) = ((9 + 1)/2) = 10/2 = 5 and

((n + 1)/2 + 1) = ((9 + 1)/2 + 1) = (10/2 + 1) = (5 + 1) = 6

The terms are 5^th and 6^th.

Now,

T₅ = T₄₊₁

= ⁹C₄ (2x)^9-4 (–x²/4)⁴

= $\frac{9×8×7×6}{4×3×2×1}×\frac{2^5}{4^4}×x^{5+8}$

= $\frac{63}{4}x^{13}$

And, T₆ = T₅₊₁

= ⁹C₅ (2x)^9-5 (–x²/4)⁵

= $-\frac{9×8×7×6}{4×3×2×1}×\frac{2^4}{4^5}×x^{4+10}$

= $-\frac{63}{32}x^{14}$

(v) (x – 1/x) ²ⁿ⁺¹

解决方案：

We have,  (x – 1/x)²ⁿ⁺¹

Here, 2n + 1 is an odd number.

So the middle terms are((2n + 1 + 1)/2)^th and ((2n + 1 + 1)/2 + 1)^th terms.

The terms are (n + 1)^th and (n + 2)^th 

Now 

T_n+1 = $^{2n + 1}{}{C}_n x^{2n + 1 - n} \times \frac{( - 1 )^n}{x^n}$

=(-1)^{n  2n+1}C_n x

And T_n+2 = T_n+1+1

= $^{2n + 1}{}{C}_{n + 1} x^{2n + 1 - n - 1} \frac{( - 1 )^{n + 1}}{x^{n + 1}}$

= $(- 1)^{n + 1} \\^{2n + 1}C_{n + 1} \times \frac{1}{x}$

(六) (x/3 + 9y) ¹⁰

解决方案：

We have,

(x/3 + 9y)¹⁰ where, n = 10 (even number)

So the middle term is (n/2 + 1) = (10/2 + 1) = (5 + 1) = 6^th term.

Now,

T₆ = T₅₊₁

= ¹⁰C₅ (x/3)^10-5 (9y)⁵

= $\frac{109876}{54323^5}9^5x^5y^5$

= 61236 x⁵ y⁵ 

(vii) (3 – x ³ /6) ⁷

解决方案：

We have,

(3 – x³/6)⁷ where, n = 7 (odd number).

So the middle terms are ((n + 1)/2) = ((7 + 1)/2) = 8/2 = 4 and

((n + 1)/2 + 1) = ((7 + 1)/2 + 1) = (8/2 + 1) = (4 + 1) = 5

The terms are 4^th and 5^th.

Now,

T₄ = T₃₊₁

= ⁷C₃ (3)^7-3 (-x³/6)³

=  $-\frac{765}{321}3^4\frac{x^9}{216}$

= $-\frac{105}{8}x^9$

And, T₅ = T₄₊₁

= ⁹C₄ (3)^9-4 (-x³/6)⁴

= $\frac{765}{321}3^5\frac{x^{12}}{6^4}$

= $\frac{35}{48}x^{12}$

(viii) (2ax – b/x ² ) ¹²

解决方案：

We have,

(2ax – b/x²)¹² where, n = 12 (even number).

So the middle terms are (n/2 + 1) = (12/2 + 1) = 7^th term

Now,

T₇ = T₆₊₁

= ¹²C₆ (2ax)^12-6 (-b/x²)⁶

= ¹²C₆ (2ax)⁶ (b/x²)⁶

= ¹²C₆ (2⁶a⁶x⁶)(b⁶/x¹²)

= ¹²C₆ (2⁶a⁶b⁶/x⁶)

(ix) (p/x + x/p) ⁹

解决方案：

We have,

(p/x + x/p)⁹ where, n = 9 (odd number).

So the middle terms are ((n + 1)/2) = ((9 + 1)/2) = 10/2 = 5 and

((n + 1)/2 + 1) = ((9 + 1)/2 + 1) = (10/2 + 1) = (5 + 1) = 6

The terms are 5^th and 6^th.

Now,

T₅ = T₄₊₁

= ⁹C₄ (p/x)^9-4 (x³/p)⁴

= ⁹C₄ (p/x)⁵ (x/p)⁴

= ⁹C₄ (p/x)

And, T₆ = T₅₊₁

= ⁹C₅ (p/x)^9-5 (x/p)⁵

= ⁹C₅ (p/x)⁴(x/p)⁵

= ⁹C₅ (x/p)

(x) (x/a – a/x) ¹⁰

解决方案：

We have,

(x/a – a/x)¹⁰ where, n = 10 (even number).

So the middle terms are (n/2 + 1) = (10/2 + 1) 6^th term

Now,

T₆ = T₅₊₁

= ¹⁰C₅ (x/a)^10-5 (-a/x)⁵

= –¹⁰C₅ (x/a)⁵ (a/x)⁵

= –¹⁰C₅ 

= -252

问题 16. 在下列表达式的展开式中找出与 x 无关的项：

(i) (3/2 x ² – 1/3x) ⁹

解决方案：

We have,

(3/2 x² – 1/3x)⁹

We know, the (r+1)^th term of the expression is given by,

T_r+1 = ⁿC_r x^n-r a^r

= ⁹C_r (3/2x²)^9-r (-1/3x)^r

= $(-1)^r (^9C_r) (\frac{3^{9-2r}}{2^{9-r}}) x^{18-2r-r}$

For this term to be independent of x, we must have

=> 18 – 3r = 0

=> 3r = 18

=> r = 18/3

=> r = 6

So, the required term is 7^th term.

So, T₇ = T₆₊₁

= ⁹C₆ × (3^9-12)/(2^9-6)

= $\frac{9×8×7}{3×2}× 3^{-3} × 2^{-3}$

= 7/18

Hence, the term independent of x is 7/18.

(ii) (2x + 1/3x ² ) ⁹

解决方案：

We have,

(2x + 1/3x²)⁹

We know, the (r+1)^th term of the expression is given by,

T_r+1 = ⁿC_r x^n-r a^r

= ⁹C_r (2x)^9-r (1/3x²)^r

= $^9C_r(\frac{2^{9-r}}{3^r})x^{9-r-2r}$

For this term to be independent of x, we must have

=> 9 – 3r = 0

=> r = 3

So, the required term is 4^th term.

So, T₇ = T₆₊₁

= ⁹C₆ × (2^9-3)/(3³)

= $\frac{9×8×7}{3×2×1}×\frac{64}{27}$

= 5376/27

(iii) (2x ² – 3/x ³ ) ²⁵

解决方案：

We have,

(2x² – 3/x³)²⁵

We know, the (r+1)^th term of the expression is given by,

T_r+1 = ⁿC_r x^n-r a^r

= ²⁵C_r (2x²)^25-r (-3/x³)^r

= (-1)^{r 25}C_r × 2^25-r × 3^r x^50-2r-3r

For this term to be independent of x, we must have

=> 50 – 5r = 0

=> 5r = 50

=> r = 10

So, the required term is 11^th term.

So, T₁₁ = T₁₀₊₁

= (-1)^{10 25}C₁₀ × 2^25-10 × 3¹⁰

= ²⁵C₁₀ (2¹⁵ × 3¹⁰)

(iv) (3x – 2/x ² ) ¹⁵

解决方案：

We have,

(3x – 2/x²)¹⁵

We know, the (r+1)^th term of the expression is given by,

T_r+1 = ⁿC_r x^n-r a^r

= ¹⁵C_r (3x)^15-r (-2/x²)^r

= (-1)^{r 15}C_r × 3^15-r × 2^r x^15-r-2r

For this term to be independent of x, we must have

=> 15 – 3r = 0

=> 3r = 15

=> r = 5

So, the required term is 6^th term.

So, T₆ = T₅₊₁

= (-1)^{5 15}C5 × 3^15-5 × 25

= −3003 × 3¹⁰ × 25

(五) $(\sqrt{\frac{x}{3}} + \frac{3}{2 x^2})^{10}$

解决方案：

We have

$(\sqrt{\frac{x}{3}} + \frac{3}{2 x^2})^{10}$

We know, the (r+1)^th term of the expression is given by,

Now,  

T_r+1 = $^{10}{}{C}_r \left( \sqrt{\frac{x}{3}} \right)^{10 - r} \left( \frac{3}{2 x^2} \right)^r$

= $^{10}{}{C}_r . \frac{3^{r - \frac{10 - r}{2}}}{2^r} x^\frac{10 - r}{2} - 2r$

For this term to be independent of x, we must have

=> (10-r)/2 – 2r = 0

=> 10 – 5r = 0

=> r = 2

So, the required term is 3^th term.

So, T₃ = T₂₊₁

T₃ = $^{10}{}{C}_2 \times \frac{3^{2 - \frac{10 - 2}{2}}}{2^2}$

= $\frac{10 \times 9}{2 \times 4 \times 9}$

= 5/4

(六) $\left( x - \frac{1}{x^2} \right)^{3n}$

解决方案：

We have

$\left( x - \frac{1}{x^2} \right)^{3n}$

We know, the (r+1)^th term of the expression is given by,

Now,  

T_r+1 = $^{3n}{}{C}_r x^{3n - r} \left( \frac{- 1}{x^2} \right)^r$

= (-1)^{r 3n}C_r x^3n-r-2r a^r  

For this term to be independent of x, we must have} 

=> 3n – 3r = 0

=> r = n

So, the required term is (n + 1)^th term.

So, 

T_n+1 = (-1)^{n 3n}C_n

(七) 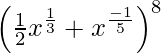$\left( \frac{1}{2} x^{\frac{1}{3}} + x^{\frac{- 1}{5}} \right)^8$

解决方案：

We have

$\left( \frac{1}{2} x^{\frac{1}{3}} + x^{\frac{- 1}{5}} \right)^8$

We know, the (r+1)^th term of the expression is given by,

Now,  

T_r+1 = $^{8}{}{C}_r \left( \frac{1}{2} x^{\frac{1}{3}} \right)^{8 - r} ( x^{\frac{- 1}{5}} )^r$

= $^{8}{}{C}_r . \frac{1}{2^{8 - r}} x^\frac{8 - r}{3} - \frac{r}{5}$

For this term to be independent of x, we must have

=> (8 – r)/3 – r/5 = 0

=> 40 – 5r – 3r = 0

=> 8r = 40

=> r = 5

So, the required term is 6^th term.

So, T₆ = T₅₊₁

T₆ = $^{8}{}{C}_5 \times \frac{1}{2^{8 - 5}}$

= $\frac{8 \times 7 \times 6}{3 \times 2 \times 8}$

= 7

(viii) (1 + x + 2x ³ ) (3/2x ² – 3/3x) ⁹

解决方案：

We have

(1 + x + 2x³) (3/2x² – 3/3x)⁹

We know, the (r+1)^th term of the expression is given by,

Now,  

T_r+1 = (1 + x + 2x³) [(3/2x²) – ⁹C₁ (3/2x²)⁸ (1/3x) + . . . – ⁹C₇ (3/2x²)² (1/3x)⁷]

For this term to be independent of r, we must have

= ⁹C₆ (3³/2³) (1/3⁶) – 2x^{3 9}C₇ (2³/3³) (1/3⁷) (1/x³)

= 7/18 – 2/27

= (189 – 36)/486

= 153/486

= 17/54

(九) $\left( \sqrt[3]{x} + \frac{1}{2 \sqrt[3]{x}} \right)^{18}$ , x > 0

解决方案：

We have

$\left( \sqrt[3]{x} + \frac{1}{2 \sqrt[3]{x}} \right)^{18}$

We know, the (r+1)^th term of the expression is given by,

Now,  

T_r+1 = $^{18}{}{C}_r ( x^{1/3} )^{18 - r} \left( \frac{1}{2 x^{1/3}} \right)^r$

= $^{18}{}{C}_r \times \frac{1}{2^r} x^\frac{18 - r}{3} - \frac{r}{3}$

For this term to be independent of r, we must have

=> (18 – r)/3 – r/3 = 0

=> 18 – 2r = 0

=> r = 9

So, the required term is 9^th term.

So, T₉ = T₈₊₁

T₉ = ¹⁸C₉ (1/2⁹)

（X） $\left( \frac{3}{2} x^2 - \frac{1}{3x} \right)^6$

解决方案：

We have

$\left( \frac{3}{2} x^2 - \frac{1}{3x} \right)^6$

Suppose the (r + 1)^th term in the given expression is independent of x.

Now,

T_r+1 = $^{6}{}{C}_r \left( \frac{3}{2} x^2 \right)^{6 - r} \left( \frac{- 1}{3x} \right)^r$

= $\left( - 1 \right)^r\\^6C_r \times \frac{3^{6 - r - r}}{2^{6 - r}} x^{12 - 2r - r}$

For this term to be independent of x, we must have

=> 12 – 3r = 0

=> r = 4

Hence, the required term is the 4^th term.

So, T₄ = T₃₊₁

= $^{6}{}{C}_4 \times \frac{3^{6 - 4 - 4}}{2^{6 - 4}}$

$= \frac{6 \times 5}{2 \times 1 \times 4 \times 9}$

= 5/12 

问题 17. 如果 (1 + x) ¹⁸的展开式中的 (2r + 4) ^th和 (r – 2) ^th项的系数相等，求 r。

解决方案：

We are given, 

(1 + x)¹⁸

We know, the coefficient of the r^th term in the expansion of (1 + x)ⁿ is ⁿC_r-1.

So, the coefficients of the (2r + 4)^th and (r – 2)^th terms in the given expansion are, 

¹⁸C_2r+4-1 and ¹⁸C_r-2-1

According to the question, we have,

=> ¹⁸C_2r+4-1 = ¹⁸C_r-2-1

=> ¹⁸C_2r+3 = ¹⁸C_r-3

We know if ⁿC_r = ⁿC_s , then r = s or r + s = n.

=> 2r + 3 = r – 3 or 2r + 3 + r – 3 = 18

=> 2r – r = –3 – 3 or 3r = 18 – 3 + 3

=> r = –6 or 3r = 18

=> r = –6 or r = 6

Ignoring r = – 6 as r cannot be negative.

Therefore, the value of r is 6.

问题 18. 如果 (1 + x) ⁴³展开式中^第( ^2r + 1) 项和第 (r + 2) 项的系数相等，求 r。

解决方案：

We are given,

(1 + x)⁴³

We know, the coefficient of the r^th term in the expansion of (1 + x)ⁿ is ⁿC_r-1.

So, the coefficients of the (2r + 1)^th and (r + 2)^th terms in the given expansion are,

⁴³C_2r+1-1 and ⁴³C_r+2-1

According to the question, we have,

=> ⁴³C_2r+1-1 = ⁴³C_r+2-1

=> ⁴³C_2r = ⁴³C_r+1

We know if ⁿC_r = ⁿC_s , then r = s or r + s = n.

=> 2r = r + 1 or 2r + r + 1 = 43

=> r = 1 or 3r = 42

=> r = 1 or r = 14

Ignoring r = 1 as it gives the same term on both the sides.

Therefore, the value of r is 14.

问题 19. 证明 (1 + x) ^{n +1}展开式中^第(r + 1) 项的系数等于展开式中第 r 项和^第(r+1) 项的系数之和(1 + x) ⁿ 。

解决方案：

We know, the coefficients of (r + 1)^th term in (1 + x)ⁿ⁺¹ is ⁿ⁺¹C_r.

So, sum of the coefficients of the r^th and (r + 1)^th terms in (1 + x)ⁿ is,

(1 + x)ⁿ = ⁿC_r-1 + ⁿC_r

As, ⁿC_r+1 + ⁿC_r = ⁿ⁺¹C_r+1

= ⁿ⁺¹C_r 

Hence proved.

问题 20. 证明 (x + 1/x) ²ⁿ展开式中与 x 无关的项是$\frac{1×3×5×7...(2n-1)}{n!}×2^n$ .

解决方案：

We have,

(x + 1/x)²ⁿ

 We know the (r + 1)^th term is given by, 

T_r+1 = ⁿC_r x^n-r a^r

= ²ⁿC_r x^2n-r (1/x)^r

= ²ⁿC_r x^2n-2r

For this term to be independent of x, we must have,

=> 2n − 2r = 0

=> 2n = 2r

=> r = n

Therefore, the required term is (n+1)^th term .

T_n+1 = ²ⁿC_n x^2n-n (1/x)ⁿ

= ²ⁿC_n

= $\frac{2n!}{n!n!}$

= $\frac{2n(2n-1)(2n-2)...5×4×3×2×1}{n!n!}$

= $\frac{(1×3×5×...(2n-1))×2^n(1×2×3×4...n)}{n!n!}$

= $\frac{(1×3×5×...(2n-1))×2^n×n!}{n!n!}$

= $\frac{1×3×5×7...(2n-1)}{n!}×2^n$

Hence proved.

问题 21. 如果展开式 (1 + x) ⁿ的^第5 项、第 6 项和^第7 项的系数在 AP 中，求ⁿ 。

解决方案：

We have, (1 + x)ⁿ

We know the coefficient of r^th term of a binomial expression is given by ⁿC_r-1.

Coefficient of 5^th term = ⁿC_5-1 = ⁿC₄

Coefficient of 6^th term = ⁿC_6-1 = ⁿC₅

Coefficient of 7^th term = ⁿC_7-1 = ⁿC₆

According to the question, we have,

=> 2 ⁿC₅ = ⁿC₄ + ⁿC₆

=> $2[\frac{n!}{(n-5)!5!}] = \frac{n!}{(n-4)!4!} + \frac{n!}{(n-6)!6!}$

=> $\frac{2}{(n-5)!5!}=\frac{1}{(n-4)!4!} + \frac{1}{(n-6)!6!}$

=> $\frac{2}{(n-5)(n-6)!×5×4!}=\frac{1}{(n-4)(n-5)(n-6)!×4!} + \frac{1}{(n-6)!×6×5×4!}$

=> $\frac{2}{(n-5)×5}=\frac{1}{(n-4)(n-5)} + \frac{1}{6×5}$

=> $\frac{2}{5}=\frac{1}{n-4} + \frac{n-5}{30}$

=> $\frac{2}{5}=\frac{30+n^2-9n+20}{30(n-4)}$

=> 60(n−4) = 150 + 5n² − 45n + 100

=> 60n − 240 = 250 + 5n² − 45n

=> 5n² − 105n + 490 = 0

=> n² − 21n + 98 = 0

=> n² − 7n − 14n + 98 = 0

=> n (n − 7) − 14 (n − 7) = 0

=> (n − 7) (n − 14) = 0

=> n = 7 or n = 14

Therefore, the value of n is 7 or 14.

问题 22. 如果展开式 (1 + x) 2n 的第 2 项、第 3 项和第 4 项的系数^在AP 中，则证明 2n ² − 9n + 7 = 0。

解决方案：

We have, (1 + x)²ⁿ

We know the coefficient of r^th term of a binomial expression is given by ⁿC_r-1.

Coefficient of 2^nd term = ²ⁿC_2-1 = ²ⁿC₁

Coefficient of 3^rd term = ²ⁿC_3-1 = ²ⁿC₂

Coefficient of 4^th term = ²ⁿC_4-1 = ²ⁿC₃

According to the question, we have,

=> 2 ²ⁿC₂ = ²ⁿC₁ + ²ⁿC₃

=> 2 = $\frac{^{2n}C_1}{^{2n}C_2}+\frac{^{2n}C_3}{^{2n}C_2}$

=> 2 = $\frac{2}{2n-2+1}+\frac{2n-3+1}{3}$

=> $\frac{2}{2n-1}+\frac{2n-2}{3}$ = 2

=> $\frac{6+4n^2-4n-2n+2}{3(2n-1)}$ = 2

=> 4n² − 6n + 8 = 12n − 6

=> 4n² − 18n + 14 = 0 

=> 2 (2n² − 9n + 7) = 0

=> 2n² − 9n + 7 = 0

Hence proved.

问题 23. 在 (1 + x) ⁿ的展开式中，连续三个项的二项式系数分别为 220、495和 792，求 n 的值。

解决方案：

We have, (1 + x)ⁿ

Let the three consecutive terms be r^th, (r+1)^th and (r+2)^th.

We know the coefficient of r^th term of a binomial expression is given by ⁿC_r-1.

Coefficient of r^th term = ⁿC_r-1 = 220

Coefficient of (r+1)^th term = ⁿC_r+1-1 = ⁿC_r = 495 

Coefficient of (r+2)^th term = ⁿC_r+2-1 = ⁿC_r+1 = 792 

Now, $\frac{^nC_{r+1}}{^nC_r}=\frac{792}{495}$

=> $\frac{n-(r+1)+1}{r+1}=\frac{792}{495}$

=> $\frac{n-r}{r+1}=\frac{8}{5}$

=> 5n − 5r = 8r + 8

=> 5n − 13r = 8  . . . . (1)

Also, $\frac{^nC_{r}}{^nC_{r-1}}=\frac{495}{220}$

=> $\frac{n-r+1}{r}=\frac{9}{4}$

=> 4n − 4r + 4 = 9r

=> 4n − 13r = −4  . . . . (2)

 Subtracting (2) from (1), we get,

=> n = 8 + 4

=> n = 12

Therefore, the value of n is 12.

问题 24. 如果展开式 (1 + x) ⁿ的第 2^项、第³项和第 4 项的系数在 AP 中，则求ⁿ的值。

解决方案：

We have, (1 + x)ⁿ

We know the coefficient of r^th term of a binomial expression is given by ⁿC_r-1.

Coefficient of 2^nd term = ⁿC_2-1 = ⁿC₁

Coefficient of 3^rd term = ⁿC_3-1 = ⁿC₂

Coefficient of 4^th term = ⁿC_4-1 = ⁿC₃

According to the question, we have,

=> 2 ⁿC₂ = ⁿC₁ + ⁿC₃

=> $2 = \frac{^{n}C_1}{^{n}C_2}+\frac{^{n}C_3}{^{n}C_2}$

=> $2 = \frac{2}{n-2+1}+\frac{n-3+1}{3}$

=> $\frac{2}{n-1}+\frac{n-2}{3} = 2$

=> $\frac{6+n^2-3n+2}{3(n-1)}$ = 2

=> n² − 3n + 8 = 6 (n − 1)

=> n² − 3n + 8 = 6n − 6

=> n² − 9n + 14 = 0

=> n² − 7n − 2n + 14 = 0

=> n (n−7) − 2 (n−7) = 0

=> (n − 2) (n − 7) = 0

=> n = 2 or n = 7

Ignoring n = 2 as it does not satisfy our condition.

Therefore, the value of n is 7.

问题 25. 如果在 (1 + x) ⁿ的展开式中，第 p 项和^第q 项的系数相等，则证明 p + q = ⁿ + 2，其中 p ≠ q。

解决方案：

We have, (1 + x)ⁿ

We know the coefficient of r^th term of a binomial expression is given by ⁿC_r-1.

Coefficient of p^th term = ⁿC_p-1

Coefficient of q^th term = ⁿC_q-1 

According to the question, we have,

=> ⁿC_p-1 = ⁿC_q-1

=> p − 1 = q − 1 or p − 1 + q − 1 = n

=> p = q or p + q − 2 = n

=> p + q − 2 = n

=> p + q = n + 2

Hence proved.

问题 26. 如果在 (1 + x) ⁿ的展开式中，三个连续项的二项式系数分别为 56、70和 56，求 n 和这些系数项的位置。

解决方案：

We have, (1 + x)ⁿ

Let the three consecutive terms be r^th, (r+1)^th and (r+2)^th.

We know the coefficient of r^th term of a binomial expression is given by ⁿC_r-1.

Coefficient of r^th term = ⁿC_r-1 = 56

Coefficient of (r+1)^th term = ⁿC_r+1-1 = ⁿC_r = 70

Coefficient of (r+2)^th term = ⁿC_r+2-1 = ⁿC_r+1 = 56

Now, $\frac{^nC_{r+1}}{^nC_r}=\frac{56}{70}$

=> $\frac{n-(r+1)+1}{r+1}=\frac{56}{70}$

=> $\frac{n-r}{r+1}=\frac{4}{5}$

=> 5n − 5r = 4r + 4

=> 5n − 9r = 4  . . . . (1)

Also, $\frac{^nC_{r}}{^nC_{r-1}}=\frac{70}{56}$

=> $\frac{n-r+1}{r}=\frac{5}{4}$

=> 4n − 4r + 4 = 5r

=> 4n − r = −4  . . . . (2)

Subtracting (2) from (1), we get,

=> n = 4 + 4 

=> n = 8

Putting n = 8 in (1), we get,

=> 5(8) − 9r = 4

=> 40 − 9r = 4

=> 9r = 36

=> r = 4

Therefore, three consecutive terms are 4^th, 5^th and 6^th terms.