问题1:将以下数字分类为有理数或无理数:
(i)2 –√5
(ii)(3 +√23)-√23
(iii)2√7/7√7
(iv)1 /√2
(v)2π
解决方案:
(i) 2 –√5
As √5 = 2.2360678… which is non-terminating and non-recurring. It is an irrational number.
When we substitute the value of √5 in equation 2 –√5, we get,
2-√5 = 2-2.2360678…
2-√5 = -0.2360678
Since the number, – 0.2360678…, is a non-terminating and non-recurring,
Therefore, 2 –√5 is an irrational number.
(ii) (3 +√23)- √23
(3 +√23) –√23 = 3+√23–√23
= 3
Since, the number 3 is rational number
Therefore, (3 +√23)- √23 is rational.
(iii) 2√7 / 7√7
2√7 / 7√7 = (2/7)× (√7/√7)
2√7 / 7√7 = (2/7)× (√7/√7)
= (2/7)×1 [As (√7/√7) = 1]
= 2/7
Since the number, 2/7 is in p/q form
Therefore, 2√7/7√7 is rational.
(iv) 1/√2
As, √2 = 1.41421… which is non-terminating and non-recurring. It is a rational number.
When we divide 1/√2 we get,
1/√2 = 1/1.41421…
=0.70710…
Since the number, 0.7071..is a non-terminating and non-recurring,
Therefore, 1/√2 is an irrational number.
(v) 2π
The value of π is 3.1415…
When we substitute the value of π in equation 2π, we get,
2π = 2 × 3.1415… = 6.2831…
Since the number, 6.2831…, is non-terminating non-recurring,
Therefore, 2π is an irrational number.
问题2:简化以下每个表达式:
(i)(3 +√3)(2 +√2)
(ii)(3 +√3)(3-√3)
(iii)(√5+√2) 2
(iv)(√5-√2)(√5+√2)
解决方案:
(i) (3+√3)(2+√2)
After opening the brackets, we get,
(3+√3)(2+√2)= (3×2)+(3×√2)+(√3×2)+(√3×√2)
(3+√3)(2+√2) = 6+3√2+2√3+√6
(ii) (3+√3)(3-√3)
After opening the brackets, we get,
(3+√3)(3-√3) = 32-(√3)2
= 9-3
(3+√3)(3-√3) = 6
(iii) (√5+√2)2
After opening the brackets, we get,
(√5+√2)2 = √52+(2×√5×√2)+ √22 [By using the formula (a + b)2 = a2 + 2ab + b2]
= 5+2×√10+2
(√5+√2)2 = 7+2√10
(iv) (√5-√2)(√5+√2)
After opening the brackets, we get,
(√5-√2)(√5+√2) = (√52-√22)
= 5-2
= 3
问题3:回想一下, π定义为圆的周长(例如c)与其直径(例如d)之比。即, π = c / d。这似乎与π不合理这一事实相矛盾。您将如何解决这一矛盾?
解决方案:
Given π = c/d = 22/7 which is equal to 3.142… which is non-terminating non-recurring decimal.
Therefore, π is irrational.
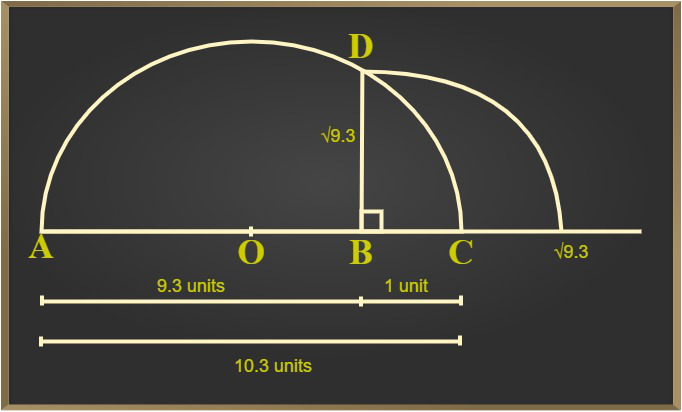
问题4:在数字行上代表(√9.3)。
解决方案:
To represent √9.3 on the number line, follow the following steps,
Step 1: Draw a 9.3 units long line segment, name the line as AB.
Step 2: Extend AB to C such that BC=1 unit.
Step 3: Now, AC = 10.3 units. Let the centre of AC be O.
Step 4: Draw a semi-circle with radius OC and centre O.
Step 5: Draw a BD perpendicular to AC at point B which is intersecting the semicircle at D.
Step 6: Join BD.
Step 7: Taking BD as radius and B as the centre point and draw an arc which touches the line segment.
The point where it intersects the line segment is at a distance of √9.3 from B as shown in the figure.
问题5:合理化以下各项的分母:
(i)1 /√7
(ii)1 /(√7-√6)
(iii)1 /(√5+√2)
(iv)1 /(√7-2)
解决方案:
(i) 1/√7
Multiply and divide 1/√7 by √7 we get,
(1×√7)/(√7×√7) = √7/7
= √7/7
(ii) 1/(√7-√6)
Multiply and divide 1/(√7-√6) by (√7+√6) we get,
[1/(√7-√6)]×(√7+√6)/(√7+√6) = (√7+√6)/(√7-√6)(√7+√6)
= (√7+√6)/√72-√62 [As, (a+b)(a-b) = a2-b2]
= (√7+√6)/(7-6)
= (√7+√6)/1
= √7+√6
(iii) 1/(√5+√2)
Multiply and divide 1/(√5+√2) by (√5-√2) we get,
[1/(√5+√2)]×(√5-√2)/(√5-√2) = (√5-√2)/(√5+√2)(√5-√2)
= (√5-√2)/(√52-√22) [As, (a+b)(a-b) = a2-b2]
= (√5-√2)/(5-2)
= (√5-√2)/3
(iv) 1/(√7-2)
Multiply and divide 1/(√7-2) by (√7+2) we get,
1/(√7-2)×(√7+2)/(√7+2) = (√7+2)/(√7-2)(√7+2)
= (√7+2)/(√72-22) [As, (a+b)(a-b) = a2-b2]
= (√7+2)/(7-4)
= (√7+2)/3