第9类RD Sharma-第22章统计数据的表格表示–练习22.1 |套装2

问题11：板球运动员在25局比赛中得分的次数如下：

26、35、94、48、82、105、53、0、39、42、71、0、64、15、34、15、34、6、71、0、64、15、34、15、34， 67、0、42、124、84、54、48、139、64、47

(i)按升序重新排列这些行程。

(ii)确定球员，是最高分。

(iii)球员没有得分多少次?

(iv)他得分了几个世纪?

(v)他得分了50次以上?

解决方案：

(i) Arranging the runs in ascending order as:

0, 0, 0, 0, 6, 15, 15, 15, 15, 26, 34, 34, 34, 34, 35, 39, 42, 42, 47, 48, 48, 53, 54, 64, 64, 64, 67, 71, 71, 82, 84, 90, 94, 124, 139.

(ii) From the above arrangement, it is observed that,

The Highest Score out of all the runs is 139.

(iii) 3 times, the player was not successful in scoring any run.

(iv) He scored 3 centuries at all.

(v) 12 times, he scored more than 50 runs.

问题12.分配的班级大小为25，第一节课的时间间隔为200-224。有七个班级间隔。

(i)写上课间隔。

(ii)写下每个间隔的等级标记。

解决方案：

Given that,

The Class size is equal to 25,

The first class interval is 200-224

There are seven class intervals in all. 

(i) These seven class interval are as follows:

200-224, 225-249, 250-274, 275-299, 300-324, 325-349, 350-374.

(ii) Class marks of the following class intervals are:

• For class interval, 200-224:

 $\begin{aligned}\frac{200+224}{2}&=\frac{424}{2}\\&=212\end{aligned}$

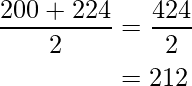
• For class interval, 225-249:

$\begin{aligned}\frac{225+249}{2}&=\frac{479}{2}\\&=237\end{aligned}$

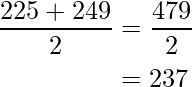
• For class interval, 250-274:

$\begin{aligned}\frac{250+274}{2}&=\frac{524}{2}\\&=287\end{aligned}$

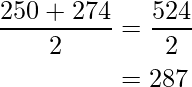
• For class interval, 300-324:

$\begin{aligned}\frac{300+324}{2}&=\frac{674}{2}\\&=312\end{aligned}$

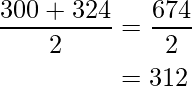
• For class interval, 325-349:

$\begin{aligned}\frac{325+349}{2}&=\frac{674}{2}\\&=337\end{aligned}$

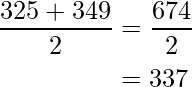
• For class interval, 350-374:

$\begin{aligned}\frac{350+374}{2}&=\frac{724}{2}\\&=362\end{aligned}$

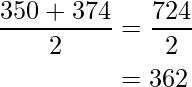
问题13：在下面的每一个中写出班级人数和班级限制：

(i)104、114、124、134、144、154和164

(ii)47、52、57、62、67、72、78、82、87、92、97、102

(iii)12.5、17.5、22.5、27.5、32.5、37.5、42.5、47.5

解决方案：

(i) 104, 114, 124, 134, 144, 154 and 164

The class size for the given data is:

114 – 104 = 10

The class limits are obtained as: 

Class mark	Lower class limit	Upper class limit	Class limit
104	104 – 10/2 = 99	104 + 10/2 = 109	99 – 109
114	114 – 10/2 = 109	114 + 10/2 = 119	109 – 119
124	119	129	119 – 129
134	129	139	129 – 139
114	139	149	139 – 149
154	149	159	149 – 159
164	159	169	159 – 169

(ii) 47, 52, 57, 62, 67, 72, 78, 82, 87, 92, 97, 102

The class size for the given data is:

52 – 47 = 5

The class limits are obtained as:  

Class mark	Lower class limit	Upper class limit	Class limit
47	47 – 5/2 = 44.5	47 + 5/2 = 49.5	44.5 + 49.5
52	49.5	54.5	49.5 + 54.5
57	54.5	59.5	54.5 – 59.5
62	59.5	64.5	59.5 – 64.5
67	64.5	69.5	64.5 – 69.5
72	69.5	74.5	69.5 – 74.5
77	74.5	79.5	74.5 – 79.5
82	79.5	84.5	79.5 – 84.5
87	84.5	89.5	84.5 – 89.5
92	89.5	94.5	89.5 – 94.5
97	94.5	99.5	94.5 – 99.5
102	99.5	104.5	99.5 – 104.5

(iii) 12.5, 17.5, 22.5, 27.5, 32.5, 37.5, 42.5, 47.5

The class size for the given data is:

17.5 – 12.5 = 5

The class limits are obtained as: 

Class mark	Lower-class limit	Upper-class limit	Class limit
12.5	12.5 – 2.5 = 10	12.5 + 2.5 = 15	10 – 15
17.5	17.5 – 2.5 = 15	17.5 + 2.5 = 20	15 – 20
22.5	22.5 – 2.5 = 20	22.5 + 2.5 = 25	20 – 25
27.5	27.5 – 2.5 = 25	27.5 + 2.5 = 30	25 – 30
32.5	32.5 – 2.5 = 30	32.5 + 2.5 = 35	30 – 35
37.5	37.5 – 2.5 = 35	37.5 + 2.5 = 40	35 – 40
42.5	42.5 – 2.5 = 40	42.5 + 2.5 = 45	40 – 45
47.5	47.5 – 2.5 = 45	47.5 + 2.5 = 50	45 – 50

问题14.以下数据提供了40个家庭中的儿童人数：

1，2，6，5，1，5，1，1，2，2，6，2，3，4，2，0，0，4，4，4，3，2，2，0，0，1，2， 2、4、3、2、1、0、5、1、2、4、3、4、1、6、2、2

用频率分布的形式表示它。

解决方案：

The frequency distribution of the following sequence of data : 

Number of children	Tally marks	Number of families
0	$\bcancel{||||}$	5
1	$\bcancel{||||}\,||$	7
2	$\bcancel{||||}\,\bcancel{||||}\,||$	12
3	$\bcancel{||||}$	5
4	$\bcancel{||||}\,|$	6
5	|||	3
6	|||	3

问题15：下面是40名IX级学生在数学上的得分：

81、55、68、79、85、43、29、68、54、73、47、35、72、64、95、44、50、77、64、35、79、52、45、54、70， 83、62、64、72、92、84、76、63、43、54、38、73、68、52、54。

解决方案：

Obtaining the frequency distribution for the marks scored by 40 students of class IX are as follows: 

Marks	Tally marks	Frequency
20 – 30	|	1
30 – 40	|||	3
40 – 50	$\bcancel{||||}$	5
50 – 60	$\bcancel{||||}\,|||$	8
60 – 70	$\bcancel{||||}\,|||$	8
70 – 80	$\bcancel{||||}\,||||$	9
80 – 90	||||	4
90 – 100	||	2
Total = 40

问题16：IX班的30名学生的身高(厘米)如下：

155、158、154、158、160、148、149、150、153、159、161、148、157、153、157、153、157、162、159、151、154、156、152、156、160， 152、147、155、163、155、157、153。

准备一个以160 – 164作为班级间隔之一的频率分配表。

解决方案：

The frequency distribution table with 160 – 164 as one of the class intervals is as follows : 

Height (in cm)	Tally marks	Frequency
145 – 149	||||	4
150 – 154	$\bcancel{||||}\,||||$	9
155 – 159	$\bcancel{||||}\,\bcancel{||||}\,||$	12
160 – 164	$\bcancel{||||}\,|$	6
Total = 30

问题17：工厂中30名工人的月工资如下：

830，835，890，810，835，836，869，845，898，890，820，860，832，833，855，845，804，808，812，840，885，835，836，878，840， 868、890、806、840、890。

解决方案：

Obtaining the frequency distribution for the monthly wages of 30 workers in a factory are as follows: 

Height (in cm)	Tally marks	Frequency
800 – 810	|||	3
810 – 820	||	2
820 – 830	|	1
830 – 840	$\bcancel{||||}\,|||$	8
840 – 850	$\bcancel{||||}$	5
850 – 860	|	1
860 – 870	|||	3
870 – 880	|	1
880 – 890	|	1
890 – 900	$\bcancel{||||}$	5
Total = 30

问题18. 11月份某个城市的每日最高气温(摄氏度)如下：

25.8，24.5，25.6，20.7，21.8，20.5，20.6，20.9，22.3，22.7，23.1，22.8，22.9，21.7，21.3，20.5，20.9，23.1，22.4，21.5，22.7，22.8，22.0，23.9，24.7， 22.8、23.8、24.6、23.9、21.1。

解决方案：

Obtaining the frequency distribution for the daily maximum temperatures (in degree Celsius) recorded in a certain city during the month of November are as follows: 

Maximum temperature (in degree Celsius)	Tally marks	Frequency
20.0 – 21.0	$\bcancel{||||}\,|$	6
21.0 – 22.0	$\bcancel{||||}$	5
22.0 – 23.0	$\bcancel{||||}\,||||$	9
23.0 – 24.0	$\bcancel{||||}$	5
24.0 – 25.0	|||	3
25.0 – 26.0	||	2
Total = 30

问题19.根据以下关于在工厂工作的28名工人的月工资(卢比)数据，使用相同的班级间隔来构建频率表，其中一个班级间隔为210 – 230(不包括230)

220、268、258、242、210、268、272、242、311、290、300、320、319、304、302、218、306、292、254、278、210、240、280、316、306， 215、256、236。

解决方案：

The following table depicts the frequency distribution on the monthly wages (in rupees) of 28 laborers working in a factory : 

Monthly wages (in rupees)	Tally marks	Frequency
210 – 230	||||	4
230 – 250	||||	4
250 – 270	$\bcancel{||||}$	5
270 – 290	|||	3
290 – 310	$\bcancel{||||}\,||$	7
310 – 330	$\bcancel{||||}$	5
Total = 28

问题20.在某些北极地区记录的每日最低摄氏温度如下：

-12.5，-10.8，-18.6，-8.4，-10.8，-4.2，-4.8，-6.7，-13.2，-11.8，-2.3，-1.2，-2.6、0、2.4、0、3.2、2.7、3.4 ，0，-2.4、0、3.2、2.7、3.4、0，-2.4，-5.8，-8.9，-14.6，-12.3，-11.5，-7.8，-2.9。

将它们表示为频率分布表，以-19.9至-15作为第一类间隔。

解决方案：

Frequency distribution with lower limit included and upper limit excluded is depicted taking the first class interval as -19.9 to  -15 :

Temperature	Tally marks	Frequency
-19.9 to -15	||	2
-15 to -10.1	$\bcancel{||||}\,||$	7
-10.1 to -5.2	$\bcancel{||||}$	5
-5.2 to -0.3	||||	4
-0.3 to -4.6	$\bcancel{||||}\,\bcancel{||||}\,\bcancel{||||}\,||$	17
Total = 35

问题21：八类的30名学生的血型记录如下：

A，B，O，O，AB，O，A，O，B，A，O，B，A，O，O，A，AB，O，A，A，A，O，O，AB，B，A， O，B，A，B，O

以频率分布表的形式表示此数据。在这些学生中找出哪个是最普通的，哪个是最稀有的血型。

解决方案：

Given that,

9 students out of the given blood groups have blood group A, 6 as B, 3 as AB and 12 as O

The frequency table representing the data along with the corresponding frequency is as follows:

Blood group	Number of students
A	9
B	6
AB	3
O	12
Total	30

Since, 12 students have their blood group as O, which is the maximum, therefore the most common blood group is O. Also, 3 students have their blood group as AB, which implies that the rarest blood group is AB.

问题22.将三枚硬币扔了30次。每次记下出现的磁头数量如下：

0、1、2、2、1、2、3、1、3、0

1，3，1，1，2，2，0，1，2，1

3，0，1，1，2，2，3，2，2，0

为上述数据准备一个频率分布表。

解决方案：

The frequency distribution table for the data of the three coins being tossed 30 times can be computed as follows:

Number of heads	Frequency
0	6
1	10
2	9
3	5
Total=30

问题23。30名儿童被问到他们在前一周看电视节目的小时数。结果发现如下：

1、6、2、3、5、12、5、8、4、8

10、3、4、12、2、8、15、1、17、6

3、2、8、5、9、6、8、7、14、2

(i)为该数据制作一个频率分布表，以班级宽度5和班级间隔之一为5 – 10。

(ii)每周有15个或更多小时的时间有多少儿童看电视。

解决方案：

(i) The following class intervals are 0 – 5, 5 – 10, 10 – 15 constructed.

The grouped frequency distribution table taking into account these class intervals is as follows:

Hours	Number of children
0 – 5	10
5 – 10	13
10 – 15	5
15 – 20	2
Total=30

(ii) The number of children who watched TV for at least 15  hours a week is 2, which implies that the number of children in the class interval 15 – 20 is equivalent to 2.