问题1:两种方式产生三角形底角时获得的外角分别为104°和136°。找到三角形的所有角度。
解决方案:
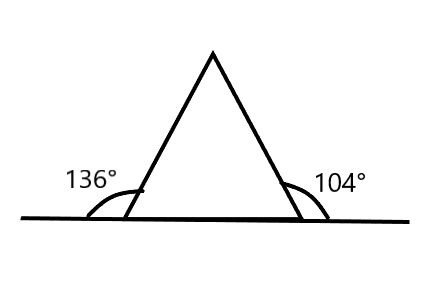
Theorems Used: The exterior angle theorem states that the measure of each exterior angle of a triangle is equal to the sum of the opposite and non-adjacent interior angles. (Exterior Angle Theorem)
∠ACD = ∠ABC + ∠BAC [Exterior Angle Theorem]
Find ∠ABC:
∠ABC + ∠ABE = 180° [Linear pair]
∠ABC + 136° = 180°
∠ABC = 44°
Find ∠ACB:
∠ACB + ∠ACD = 180° [Linear pair]
∠ACB + 1040 = 180°
∠ACB = 76°
Now,
Sum of all angles of a triangle = 180°
∠A + 44° + 76° = 180°
∠A = 180° − 44°−76°
∠ A = 60°
Angles of the triangle are ∠ A = 60°, ∠B = 44° and ∠C = 76° (ans)
问题2:在△ABC中,∠B和∠C的内部等分线在P点相遇,∠B和∠C的外部等分线在Q点相交。证明∠BPC+∠BQC= 180°。
解决方案:
In △ABC,
BP and CP are an internal bisector of ∠B and ∠C respectively
=> External ∠B = 180° – ∠B
BQ and CQ are an external bisector of ∠B and ∠C respectively.
=> External ∠C = 180° – ∠C
In triangle BPC,
∠BPC + 1/2∠B + 1/2∠C = 180°
∠BPC = 180° – (∠B + ∠C) …. (1)
In triangle BQC,
∠BQC + 1/2(180° – ∠B) + 1/2(180° – ∠C) = 180°
∠BQC + 180° – (∠B + ∠C) = 180°
∠BPC + ∠BQC = 180° [Using (1)] (Proved)
问题3:在图中,△ABC的BC,CA和AB面分别制作为D,E和F。如果∠ACD= 105°,andEAF = 45°,则求出△ABC的所有角度。
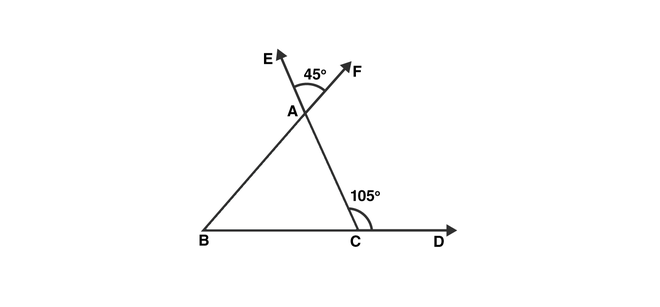
解决方案:
Theorems Used:
(i) The exterior angle theorem states that the measure of each exterior angle of a triangle is equal to the sum of the opposite and non-adjacent interior angles. (Exterior Angle Theorem)
(ii) Sum of a linear angle pair is 180°
(iii)Vertically opposite angles are equal.
∠BAC = ∠EAF = 45° [Vertically opposite angles]
∠ACD = 180° – 105° = 75° [Linear pair]
∠ABC = 105° – 45° = 60° [Exterior angle property]
问题4:在以下每个图形中计算x的值:
(一世)
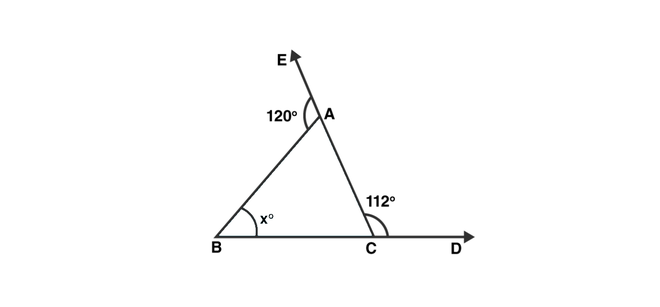
解决方案:
∠BAC = 180° – 120° = 60° [Linear pair]
∠ACB = 180° – 112° = 68° [Linear pair]
Sum of all angles of a triangle = 1800
x = 180° − ∠BAC − ∠ACB
= 180° − 60° − 68° = 52° (ans)
(ii)

解决方案:
∠ABC = 180° – 120° = 60° [Linear pair]
∠ACB = 180° – 110° = 70° [Linear pair]
Sum of all angles of a triangle = 180°
x = ∠BAC = 180° − ∠ABC − ∠ACB
= 180° – 60° – 70° = 50° (ans)
(iii)
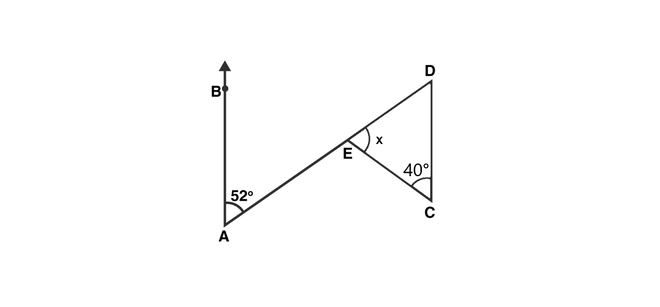
解决方案:
∠BAE = ∠EDC = 52° [Alternate angles]
Sum of all angles of a triangle = 180°
x = 180° – 40° – 52° = 180° − 92° = 88° (ans)
(iv)
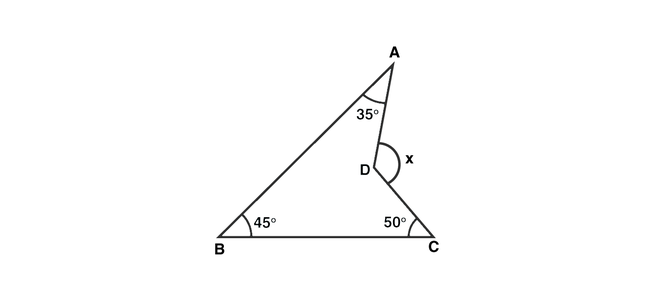
解决方案:

CD is produced to meet AB at E.
∠BEC = 180° – 45° – 50° = 85° [Sum of all angles of a triangle = 180°]
∠AEC = 180° – 85° = 95° [Linear Pair]
Now, x = 95° + 35° = 130° [Exterior angle Property]
Answer: x = 130°
问题5:在图中,AB将∠DAC以1:3的比例除,AB = DB。确定x的值。

解决方案:
Let ∠BAD = y, ∠BAC = 3y
∠BDA = ∠BAD = y (As AB = DB)
Now,
∠BAD + ∠BAC + 108° = 180° [Linear Pair]
y + 3y + 108° = 180°
4y = 72°
or y = 18°
Now, In ΔADC
∠ADC + ∠ACD = 108° [Exterior Angle Property]
x + 18° = 180°
x = 90° (ans)
问题6:ABC是一个三角形。外角B的等分线与∠C的等分线在D处相交。证明∠D=(1/2)∠A。
解决方案:
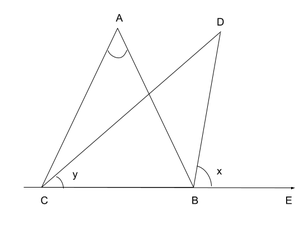
Let ∠ABE = 2x and ∠ACB = 2y
∠ABC = 180° – 2K [Linear pair]
∠A = 180° — ∠ABC — ∠ACB [Angle sum property]
= 180° -180° + 2x – 2y
= 2(x – y)
Now, ∠D = 180° – ∠DBC – ∠DCB
∠D = 180° -(x + 180° – 2x) – y
= x – y
= (1/2)∠A (Hence Proved)
问题7:在图中, AC垂直于CE,∠A:∠B:∠C= 3:2:1求∠ECD。

解决方案:
Given that ∠A:∠B:∠C = 3:2:1
Let the angles be 3x, 2x and x.
3x + 2x + x = 180° [Angle Sum property]
6x = 180°
x = 30° = ∠ACB
Therefore,
∠ECD = 180° – ∠ACB – 90° [Linear Pair]
= 180° – 30° – 90°
= 60° (ans)
问题8:在图中,AM垂直于BC,AN是∠A的等分线。如果∠B = 65°且∠C = 33°,则找到∠MAN 。

解决方案:
Let ∠BAN = ∠NAC = x [AN bisects ∠A]
Therefore, ∠ANM = x + 33° [Exterior angle property]
In △AMB,
∠BAM = 90° – 65° = 25° [Exterior angle property]
Therefore, ∠MAN = ∠BAN – ∠BAM = x – 25°
Now in △MAN,
(x – 25°) + (x + 33°) + 90° = 180° [Angle sum property]
or, 2x + 8° = 90°
or x = 41°
Therefore, ∠MAN = 41° – 25° = 16° (ans)
问题9:在△ABC中,AD将∠A和∠C>∠B一分为二。证明∠ADB>∠ADC。
解决方案:

Let us assume that ∠BAD = ∠CAD = x. [given AD bisects ∠A]
Given that,
∠C > ∠B
or, ∠C + x > ∠B + x [Adding x on both sides]
or, 180° – ∠ADC > 180° – ∠ADB [Angle sum property]
or, – ∠ADC >- ∠ADB
or, ∠ADB > ∠ADC. (proved)
问题10:在△ABC中,BD垂直于AC,CE垂直于AB。如果BD和CE在O相交,则证明∠BOC = 180°-∠A。
解决方案:
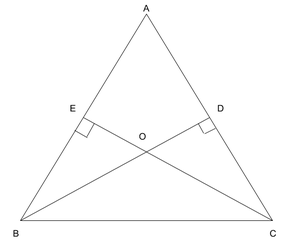
In quadrilateral AEOD,
∠A + ∠AEO + ∠EOD + ∠ADO = 360°
or, ∠A + 90° + 90° + ∠EOD = 360°
or, ∠A + ∠BOC = 360° – 90° – 90° [∠EOD = ∠BOC as they are vertically opposite angles]
or, ∠BOC = 180° – ∠A (proved)
问题11:在图中,AE将∠CAD和∠B=∠C一分为二。证明AE ||公元前。

解决方案:
Let ∠B = ∠C = x
Then,
∠CAD = ∠B + ∠C = 2x (Exterior Angle)
∠CAD/2 = x
∠EAC = ∠C [AE bisects ∠CAD and ∠C = x assumed]
These are interior angles for line AE and BC,
Therefore,
AE || BC (proved)
问题12:在图中AB || DE。查找∠ACD。

解决方案:
Since, AB || DE
Therefore,
∠ABC = ∠CDE = 40° [Alternate Angles]
∠ACB = 180° – ∠ABC – ∠BAC
= 180° – 40° – 30°
= 110°
Therefore,
∠ACD = 180° – ∠ACB [Linear Pair]
=70°
问题13.以下哪个陈述是正确的(T),哪些是错误的(F):
(i)三角形的三个角度之和为180°。
Answer: [True]
(ii)一个三角形可以有两个直角。
Answer: [False]
(iii)三角形的所有角度都可以小于60°。
Answer: [False]
(iv)三角形的所有角度都可以大于60°。
Answer: [False]
(v)三角形的所有角度都可以等于60°。
Answer: [True]
(vi)一个三角形可以有两个钝角。
Answer: [False]
(vii)一个三角形最多可以有一个钝角。
Answer: [True]
(viii)如果三角形的一个角是钝角,则它不能是直角三角形。
Answer: [True]
(lx)三角形的外角小于其内部相对角之一。
Answer: [False]
(x)三角形的外角等于两个内部对角之和。
Answer: [True]
(xi)三角形的外角大于相反的内角。
Answer: [True]
问题14:填空以使以下陈述正确
(i)三角形的角度之和是_________。
Answer: 180°
(ii)三角形的外角等于两个____________相对角。
Answer: Interior
(iii)三角形的外角总是比两个内角都大。
Answer: Greater
(iv)三角形的直角不能超过______________________。
Answer: One
(v)三角形的钝角不能超过________________________。
Answer: One