如果 tan A = 4/3，则求 cos A 的值

三角学是数学的一个重要分支，它处理直角三角形中边与角的关系。三角学在物理学中也很重要，它用于查找塔的高度、恒星之间的距离，或用于导航系统。三角学的原理是“如果两个三角形有一组相等的角，那么它们的边在相同的比率内” 。边长通常不同，但边长比相同。

三角函数

三角函数或圆函数或三角比显示角度和边的关系。这些三角比是通过取边的比来获得的。我们有六个三角比 Sin、Cos、Tan、Cosec、Sec、Cot。

• sin A = 垂直 / 斜边
• cos A = 底边 / 斜边
• tan A = 垂直 / 底
• 婴儿床 A = 底座 / 垂直
• sec A = 斜边 / 底边
• cosec A = 斜边 / 垂直

这里，A 是与垂直边相对的角度。

让我们看看直角三角形的垂直、底边和斜边是什么，

1. 垂直：角的前面是垂直的。在这种情况下，30 度前面的一侧称为垂直。
2. 底：底是包含角的边之一，斜边除外。
3. 斜边：与90°相对的一侧。这是最大的一面。

Note: Perpendicular and base changes as angle changes. During a triangle, a side is perpendicular for an angle, but an equivalent side may be a base for an additional angle, but the hypotenuse remains an equivalent because it’s a side opposite to angle 90°.

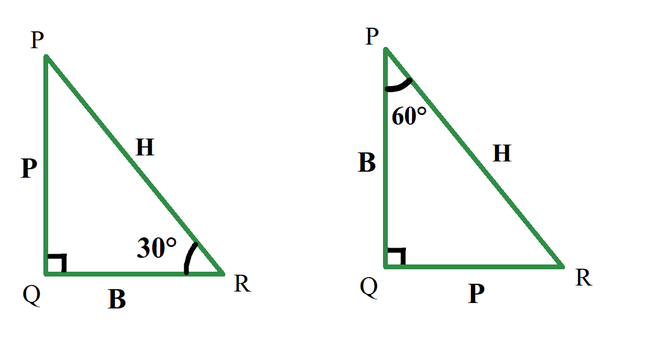
在上面的等效三角形图中，如果考虑角度 30°，则垂线是边 PQ，但如果考虑角度 60°，则垂线是边 QR。

如果 tan A = 4/3，则求 cos A 的值

解决方案：

Tan – The tan of an angle A is the ratio of lengths of perpendicular to the base.

Tan A = Perpendicular / Base

Cos – The cos of an angle A is the ratio of lengths of the base to the hypotenuse.

Cos A = Base / Hypotenuse

In a right triangle if tan A is 4/3 it looks as follows.

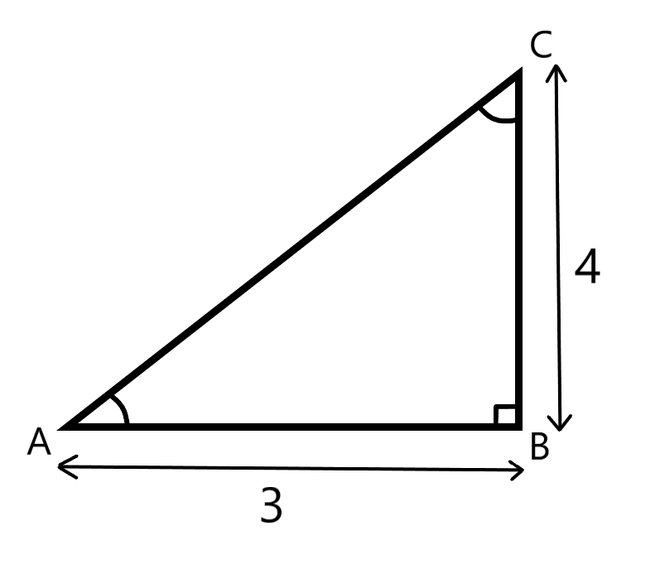
tan A = 4/3

perpendicular / base = 4/3

Calculating hypotenuse –

H² = P² + B²

H² = 4² + 3²

H² = 16 + 9

H² = 25

H =5

Cos A = Base / hypotenuse

Cos A = 3/5

示例问题

问题1：在一个直角三角形中，角A为60°，底边为3m。求斜边的长度。

解决方案：

Given:  Base = 3m

Cos 60 = 1/2

B/H = 1/2

3/H = 1/2  

H = 6  

问题2：直角三角形中，角A为30°，斜边为3m。求基地的长度。

解决方案：

Given:  Hypotenuse = 3m

Cos 30° = √3/2

B/H = √3/2  

B/3 = √3/2

B = 3√3/2

问题3：在一个直角三角形中，角A，垂线是9√3m，底边是9m，求角A。

解决方案：

Given:  perpendicular = 9√3m, Base = 9m.

Tan A = 9√3 / 9

Tan A = √3

Tan (60°) = √3

Angle A =  60°