📌  相关文章
📜  给定 sin A = 12/37，求 cos A 和 tan A

📅  最后修改于: 2022-05-13 01:54:15.663000             🧑  作者: Mango

给定 sin A = 12/37，求 cos A 和 tan A

三角学是直角三角形的角和边之间的关系。在直角三角形中，有3个角，其中一个角是直角（90°），另外两个角是锐角，有3条边。与直角相对的一侧称为斜边。根据它们之间的角度，这些边之间有 6 个比率，它们被称为三角比。

6个三角比是：

  • 正弦 (sin)
  • 余弦 (cos)
  • 切线（棕褐色）
  • 割线 (cosec)
  • 正割（秒）
  • 余切 (cot)

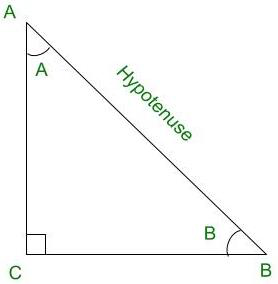
直角三角形 ACB

正弦（sin）：

角的正弦由与角和斜边相反的边的长度之比定义。对于上述三角形，sin A = BC/AB

余弦（cos）：

角的余弦由与角和斜边相邻的边的长度之比定义。对于上述三角形，cos A = AC/AB

切线（tan）：

角的正切定义为与角相对的边与与角相邻的边的长度之比。对于上述三角形，tan A = BC/AC

余割（cosec）：

角的余割由斜边的长度与角对边的比值定义。对于上述三角形， cosec A = AB/BC

割线（秒）：

角的割线定义为斜边的长度与与角相邻的边和边的比值 对于上述三角形，sec A = AB/AC

余切（cot）：

角的余切定义为与角相邻的边与对角的边的长度之比。对于上述三角形，cot A = AC/BC

给定 sin A = 12/37，求 cos A 和 tan A。

解决方案：

类似问题

问题 1：如果 sinA = 3/5，求 cosA 和 tanA

解决方案：

问题 2：如果 Sin A = 3/4，计算 cos A 和 tan A。

解决方案：

问题 3：如果 cosA = 12/13，求 sinA 和 tanA。

解决方案：