第 11 类 RD Sharma 解决方案 - 第 30 章衍生品 - 练习 30.4 |设置 3
问题 21. 将 (2x 2 – 3) sin x 与 x 微分。
解决方案:
We have,
=> y = (2x2 – 3) sin x
On differentiating both sides, we get,
![]()
On using product rule we get,
= ![]()
= ![]()
= ![]()
问题 22. 区分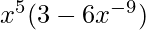 关于 x。
关于 x。
解决方案:
We have,
=> y = ![]()
On differentiating both sides, we get,
![]()
On using product rule we get,
= ![]()
= ![]()
= ![]()
= ![]()
问题 23. 区分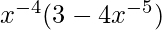 关于 x。
关于 x。
解决方案:
We have,
=> y = ![]()
On differentiating both sides, we get,
![]()
On using product rule we get,
= ![]()
= ![]()
= ![]()
= ![]()
= ![]()
= ![]()
问题 24. 区分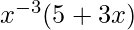 关于 x。
关于 x。
解决方案:
We have,
=> y = ![]()
On differentiating both sides, we get,
![]()
On using product rule we get,
= ![]()
= ![]()
= ![]()
= ![]()
= ![]()
问题 25. 区分![由 QuickLaTeX.com 渲染 \frac{ax+b}{cx+d}]() 关于 x。
关于 x。
解决方案:
We have,
=> y =
On differentiating both sides, we get,
![]()
On using product rule we get,
= ![]()
= ![]()
= ![]()
= ![]()
= ![]()
= ![]()
= ![]()
问题 26. 将 (ax + b) n (cx + d) m与 x 微分。
解决方案:
We have,
=> y = (ax + b)n (cx + d)m
On differentiating both sides, we get,
![]()
On using product rule we get,
= ![]()
= ![]()
= ![]()
= ![]()
问题 27. 以两种方式进行微分,使用乘积规则,否则使用函数(1 + 2 tan x) (5 + 4 cos x)。验证答案是否相同。
解决方案:
We have,
=> y = (1 + 2 tan x) (5 + 4 cos x)
On using product rule we get,
![Rendered by QuickLaTeX.com \frac{d}{dx}[(1 + 2 tan x) (5 + 4 cos x)]=(5 + 4 cos x)\frac{d}{dx}(1+2tanx)+(1+2tanx)\frac{d}{dx}(5+4cosx)](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_11_RD_Sharma_Solutions_%E2%80%93_Chapter_30_Derivatives_%E2%80%93_Exercise_30.4_%7C_Set_3_43.jpg)
= ![]()
= 10 sec2 x + 8 cos x sec2 x − 4 sin x − 8 sin x tan x
= ![]()
= ![]()
= ![]()
= ![]()
= 10 sec2 x + 8 cos x − 4 sin x
By using an alternate method, we have,
![]()
= ![]()
= ![]()
On using chain rule, we get,
= 0 − 4 sin x + 10 sec2 x + 8 cos x
= 10 sec2 x + 8 cos x − 4 sin x
Hence proved.
问题 28. 通过乘积规则和其他方法区分以下每个功能,并验证两种方法的答案是否相同。
(一) (3x 2 + 2) 2
解决方案:
We have,
=> y = (3x2 + 2)2
On using product rule we get,
![]()
= ![]()
= 12x (3x2 + 2)
= 36 x3 + 24x
By using an alternate method, we have,
![]()
On using chain rule, we get,
= 36 x3 + 0 + 24 x
= 36 x3 + 24x
Hence proved.
(ii) (x + 2)(x + 3)
解决方案:
We have,
=> y = (x + 2)(x + 3)
On using product rule we get,
![]()
= (x+3)(1)+(x+2)(1)
= x + 3 + x + 2
= 2x + 5
By using an alternate method, we have,
![]()
On using chain rule, we get,
= 2x + 5
Hence proved.
(iii) (3 sec x − 4 cosec x) (−2 sin x + 5 cos x)
解决方案:
We have,
=> y = (3 sec x − 4 cosec x) (−2 sin x + 5 cos x)
On using product rule we get,
![Rendered by QuickLaTeX.com \frac{d}{dx}[(3secx−4cosecx)(−2sinx+5cosx)]=(−2sinx+5cosx)\frac{d}{dx}(3secx−4cosecx)+(3secx−4cosecx)\frac{d}{dx}(−2sinx+5cosx)](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_11_RD_Sharma_Solutions_%E2%80%93_Chapter_30_Derivatives_%E2%80%93_Exercise_30.4_%7C_Set_3_57.jpg)
= (−2 sin x + 5 cos x) (3 sec x tan x + 4 cot x cosec x)+ (3 sec x − 4 cosec x) (−2 cos x − 5 sin x)
= −6 sin x sec x tan x − 8 sin x cot x cosec x + 15 cos x sec x tan x + 20 cos x cot x cosec x − 6 sec x cos x − 15 sec x sin x + 8 cosec x cos x + 20 cosec x sin x
= −6 tan2 x − 8 cot x + 15 tan x + 20 cot2 x − 6 − 15 tan x + 8 cot x + 20
= − 6 − 6 tan2 x + 20 cot2 x + 20
= −6 (1 + tan2 x) + 20 (cot2 x + 1)
= −6 sec2 x + 20 cosec2 x
By using an alternate method, we have,
![Rendered by QuickLaTeX.com \frac{d}{dx}[(3secx−4cosecx)(−2sinx+5cosx)]=\frac{d}{dx}(−6secxsinx+15secxcosx+6cosecxsinx-20cosecxcosx)](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_11_RD_Sharma_Solutions_%E2%80%93_Chapter_30_Derivatives_%E2%80%93_Exercise_30.4_%7C_Set_3_58.jpg)
= ![]()
= ![]()
On using chain rule, we get,
= −6 sec2 x − (−20 cosec2 x)
= −6 sec2 x + 20 cosec2 x
Hence proved.