如果 sin a = 3/5，求 cos a 和 tan a

三角学是一门数学学科，研究直角三角形的边长和角之间的关系。三角函数，也称为测角函数、角函数或圆函数，是建立角度与直角三角形的两条边之比之间关系的函数。六个主要的三角函数是正弦、余弦、正切、余切、正割或余割。

Angles defined by the ratios of trigonometric functions are known as trigonometry angles. Trigonometric angles represent trigonometric functions. The value of the angle can be anywhere between 0-360°.

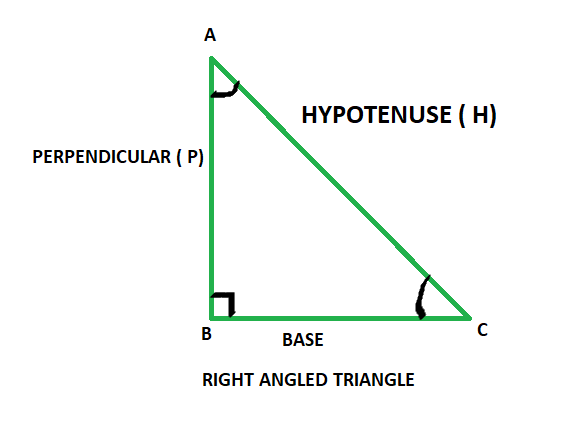
如上图中的直角三角形所示：

• 斜边：与直角相对的边是斜边，它是直角三角形中最长的边，与90°角相对。
• 底：角 C 所在的一侧称为底。
• 垂直：考虑角度 C 的对边。

三角函数

三角函数有 6 个基本的三角函数，它们是正弦、余弦、正切、余割、正割和余切。现在让我们看看三角函数。六个三角函数如下，

• 正弦：它被定义为垂直和斜边的比率，它表示为 sin θ
• 余弦：定义为底边与斜边的比值，表示为 cos θ
• 正切：它被定义为一个角度的正弦和余弦之比。因此，切线的定义是垂直与底的比值，并表示为 tan θ
• cosecant：它是 sin θ 的倒数，表示为 cosec θ。
• 割线：它是 cos θ 的倒数，表示为 sec θ。
• cotangent：它是 tan θ 的倒数，表示为 cot θ。

根据上图，三角比是

Sin θ = Perpendicular / Hypotenuse = AB/AC

Cosine θ = Base / Hypotenuse = BC / AC

Tangent θ = Perpendicular / Base = AB / BC

Cosecant θ = Hypotenuse / Perpendicular = AC/AB

Secant θ = Hypotenuse / Base = AC/BC

Cotangent θ = Base / Perpendicular = BC/AB

互惠身份

Sin θ = 1/ Cosec θ                    OR        Cosec θ = 1/ Sin θ

Cos θ = 1/ Sec θ                       OR        Sec θ = 1 / Cos θ

Cot θ = 1 / Tan θ                     OR         Tan θ = 1 / Cot θ

Cot θ = Cos θ / Sin θ               OR         Tan θ = Sin θ / Cos θ

Tan θ.Cot θ = 1

三角比值

	0°	30°	45°	60°	90°
Sin θ	0	1/2	1/√2	√3/2	1
Cos θ	1	√3/2	1/√2	1/2	0
Tan θ	0	1/√3	1	√3	Not Defined
Sec θ	Not Defined	2	√2	2/√3	1
Cosec  θ	1	2/√3	√2	2	Not Defined
Cot θ	Not Defined	√3	1	1/√3	0

补角和补角的三角恒等式

• 互补角：和等于90°的一对角
• 补角：和等于 180° 的一对角

互补角的恒等式是

sin (90° – θ) = cos θ

cos (90° – θ) = sin θ

tan (90° – θ) = cot θ

cot (90° – θ) = tan θ

sec (90° – θ) = cosec θ

cosec (90° – θ) = sec θ

补角的恒等式

sin (180° – θ) = sin θ

cos (180° – θ) = – cos θ

tan (180° – θ) = – tan θ

cot  (180° – θ) = – cot θ

sec (180° – θ) = – sec θ

cosec (180° – θ) = – cosec θ

三角学象限

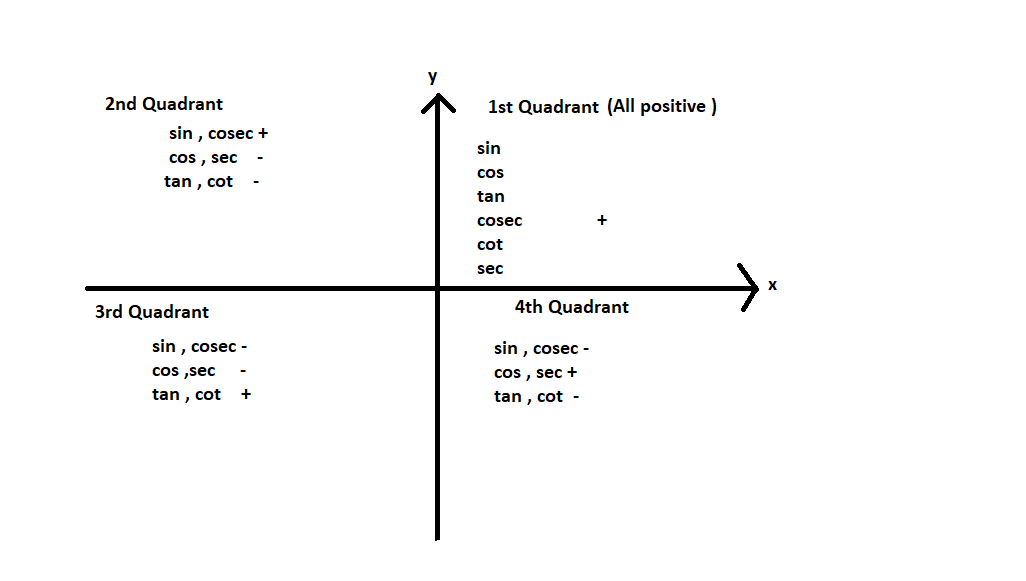
象限

如果 sin a = 3/5 求 cos a 和 tan a

解决方案：

In right angled triangle 

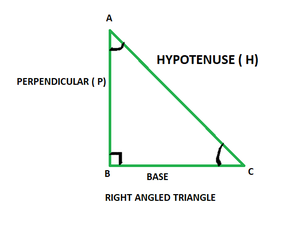
We have sin a = 3/5

therefore Sin θ = Perpendicular / Hypotenuse = AB/AC

therefore AB = 3 

                 AC =  5

therefore as per the Pythagoras theorem 

we have AC² = BC² + AB²

                    5²   = BC² + 3²

               25  = BC² + 9

             25-9 = BC²

                 16 = BC²

                 BC = 4

So now we have AB = 3

                          AC = 5

                          BC = 4

Now to find cos a and tan a 

Cosine θ = Base / Hypotenuse = BC / AC

           so cos a = 4/5

and Tangent θ = Perpendicular / Base = AB / BC

          so tan a = 3/4

类似问题

问题 1：证明三角方程：cos theta sec theta/cot theta = tan theta？

解决方案：

Here we have cos theta sec theta / cot theta = tan theta

Therefore { cos θ sec θ }/ cot θ = tan θ

By taking L.H.S

cos θ sec θ / cot θ

we can write cos θ sec θ as 1  

       = (cos θ sec θ)/cot θ

       = 1/cot θ                                   { Cos θ = 1/ Sec θ   therefore Cos θ Sec θ  = 1}

       = tan θ                                            { Tan θ = 1 / Cot θ }

Therefore LHS = RHS

{cos θ sec θ}/ cot θ = tan θ

Hence Proved

问题 2：如果 tan a = 4/5 找到 cos a 和 sin a？

解决方案：

In right angled triangle

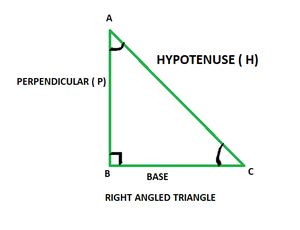
We have tan a = 3/4

Therefore Tangent θ = Perpendicular / Base = AB / BC

                AB = 3

                BC = 4

Therefore as per the Pythagoras theorem

We have     AC² = BC² + AB²

                   AC² = 4² + 3²

                  AC²  = 16+ 9

                  AC² = 25

                   AC = 5                                    

So now we have AB = 3

                           AC = 5

                           BC = 4

Now to find cos a and sin a

Cosine θ = Base / Hypotenuse = BC / AC

 so cos a = 4/5

and Sin θ = Perpendicular / Hypotenuse = AB/AC

   so sin a = 3/5