第 12 类 RD Sharma 解 - 第 19 章不定积分 - 练习 19.3 |设置 2

问题 11. 整合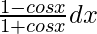$∫\frac{1-cosx}{1+cosx}dx$

解决方案：

Let I =$∫\frac{1-cosx}{1+cosx}dx$

On simplifying the above equation, we get

I = $∫\frac{2 sin^2(\frac{x}{2})}{2 cos^2(\frac{x}{2})} dx$

= $∫\frac{sin^2 (\frac{x}{2})}{cos^2 (\frac{x}{2})} dx$

= ∫ tan² x/2 dx

= $∫(sec^2\frac{x}{2} - 1) dx -(1)$

On integrating the equation(1), we get

= $\frac{(tan \frac{x}{2})}{(\frac{1}{2})} - x +c$

Hence, I = 2 tan x/2 – x + c 

问题 12. 整合$∫ \frac{1}{1-sin\frac{x}{2}} dx$

解决方案：

 Let I = $∫ \frac{1}{1-sin\frac{x}{2}} dx$

Now multiply with the conjugate,

= $∫ \frac{1}{(1-sin\frac{x}{2})} × \frac{(1 + sin \frac{x}{2})}{(1+ sin \frac{x}{2})} dx\\ = ∫\frac{(1+ sin\frac{x}{2})}{(1- sin^2 \frac{x}{2})} dx\\ = ∫\frac{(1+ sin\frac{x}{2})}{(cos^2 \frac{x}{2})} dx\\ = ∫\frac{1}{(cos^2\frac{x}{2})} dx + ∫\frac{(sin \frac{x}{2})}{(cos^2\frac{x}{2})} dx\\= ∫ sec^2 \frac{x}{2} dx + ∫ \frac{sec \frac{x}{2} tan \frac{x}{2}} dx$

On integrating the equation, we get

= $\frac{(tan \frac{x}{2})}{(\frac{1}{2})} + \frac{(sec \frac{x}{2})}{(\frac{1}{2})} + c$

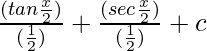
= 2 tan x/2 + 2 sec x/2 +c

Hence, I = 2 (tan x/2 + sec x/2) + c

问题 13. 整合$∫ \frac{1}{(1 + cos 3x)} dx$

解决方案：

Let I = $∫ \frac{1}{(1 + cos 3x)} dx$

Now multiply with the conjugate,

= ∫ 1/(1 + cos 3x) × (1 – cos 3x)/(1 – cos 3x) dx

= ∫ (1 – cos 3x)/ (1 – cos² 3x) dx

= ∫ (1 – cos 3x)/ (sin² 3x) dx

= ∫ (1/ sin² 3x) – (cos 3x/ sin² 3x) dx

= ∫ (cosec² 3x – cosec3x cot3x) dx                   -(1)

On integrating the equation(1), we get

= – cot 3x/3 + cosec 3x/3 + c

= (-1/3) × (cos 3x/ sin 3x) + (1/3) × (1/sin 3x) + c

= (1 – cos 3x) / 3 sin 3x + c

Therefore, I = (1 – cos 3x) / 3 sin 3x + c

问题 14. 积分 ∫(e ^x + 1) ² e ^x dx

解决方案：

Let I = ∫ (e^x + 1)² e^x dx                   -(1)

(e^x + 1) = t                   -(2)

On differentiating the above equation, we get

e^x dx = dt                   -(3)

Now, put the eq(2) and (3) in eq(1)

= ∫ (t²) dt                   -(4)

On integrating the equation(4), we get

= (t³ /3) + c

Therefore, I = (e^x + 1)³ /3 + c

问题 15. 积分 ∫ (e ^x + (1 + e ^x )) ² dx

解决方案：

Let I = ∫ (e^x + (1/e^x))² dx

= ∫ (e^2x + (1/e^2x) + 2)dx                   -(1)

On integrating the equation(1), we get

= (e^2x/2) – (1/2 e^-2x) + 2x + c

Therefore, I = (e^2x/2) – (1/2 e^-2x) + 2x + c

问题 16. 整合$∫ \frac{(1 + cos 4x)}{(cot x - tan x)} dx$

解决方案：

Let I = $∫ \frac{(1 + cos 4x)}{(cot x - tan x)} dx$

= $∫\frac{(2 cos^2 2x)}{(\frac{cos x}{sin x}) - (\frac{sin x}{cos x})} dx \\ = ∫ \frac{(2 cos^2 2x)}{\frac{cos^2x - sin^2x}{cosx sinx}} dx \\ = ∫ \frac{(2 cos^2 2x. cosx.sinx)}{(cos^2x - sin^2x)} dx$

On simplifying the above equation,

= ∫ cos²2x. (sin2x / cos2x) dx

= ∫ cos 2x. sin2x dx

= 1/2∫ sin (2x + 2x) + sin (2x – 2x) dx

= 1/2∫(sin 4x + sin 0) dx

= 1/2∫(sin 4x + 0) dx

= 1/2 ∫sin 4x dx                   -(1)

On integrating the equation(1), we get

 = (-1/2) ((cos 4x)/4) + c

Therefore, I = (-1/8) (cos 4x) + c

问题 17. 整合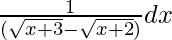$∫ \frac{1} {(\sqrt{x +3} - \sqrt{x + 2})} dx$

解决方案：

Let I = $∫ \frac{1} {(\sqrt{x +3} - \sqrt{x + 2})} dx$

Now multiply with the conjugate,

= $∫ \frac{1}{(\sqrt{x +3} - \sqrt{x + 2})} × \frac{(\sqrt{x +3} + \sqrt{x + 2})}{(\sqrt{x +3} + \sqrt{x + 2})} dx\\ = ∫\frac{(\sqrt{x +3} + \sqrt{x + 2})}{(x+3-x-2)} dx$

= ∫(x +3)^1/2 + (x + 2)^1/2 dx                   -(1)

On integrating the equation(1), we get

= $\frac{(x+3)^{\frac{3}{2}}}{(\frac{3}{2})} + \frac{(x+2)^{\frac{3}{2}}}{(\frac{3}{2})} + c$

= (2/3)(x + 3)^3/2 +(2/3) (x + 2)^3/2 + c

Hence, I = (2/3){(x + 3)^3/2 + (x + 2)^3/2} +c

问题 18. 积分 ∫ tan ² (2x – 3) dx

解决方案：

Let I = ∫ tan²(2x – 3) dx

= ∫ sec² (2x – 3) – 1 dx                   -(1)

Now put,  2x – 3 = t                   -(2)

2dx = dt                   -(3)

Put eq(3) and (2) in eq(1)

= 1/2∫sec² t dt – ∫1dx                   -(4)

On integrating the equation(4), we get

= 1/2 tan t – x + c

= 1/2 tan(2x – 3) – x + c

Therefore, I = 1/2 tan(2x – 3) – x + c

问题 19. 整合$∫ \frac{1}{(cos^2x(1+tanx)^2} dx$

解决方案：

Let I = $∫ \frac{1}{(cos^2x(1+tanx)^2} dx$

$= ∫ \frac{1}{cos^2x(1-\frac{sinx}{cosx}^2)} dx\\ = ∫ \frac{1}{(cosx - sinx)^2} dx\\ = ∫ \frac{1}{(1-sin2x)} dx\\ = ∫ \frac{1}{1+cos(\frac{π}{2} + 2x)} dx\\ = ∫ \frac{1}{2cos^2(\frac{π}{4} + x)} dx\\ = \frac{1}{2} ∫ sec^2(\frac{π}{4} + x) dx$

On integrating the equation, we get

= 1/2 tan(π/4 + x) + c

Therefore, I = 1/2 tan(π/4 + x) + c