第 12 类 RD Sharma 解 - 第 19 章不定积分 - 练习 19.8 |设置 2

问题 26. 计算 ∫ 2cosx – 3sinx/ 6cosx + 4sinx dx

解决方案：

Let us assume I = ∫ 2cosx – 3sinx/ 6cosx + 4sinx dx

= ∫ 2cosx – 3sinx/ 2(3cosx + 2sinx) dx

= ∫ 2cosx – 3sinx/ 2(3cosx + 2sinx) dx ………..(i)      

Let 3cosx + 2sinx = t

d(3cosx + 2sinx) = dt

(-3sinx + 2cosx) dx = dt

(2cosx – 3sinx) dx = dt

Put all these values in equation(i), we get

= 1/2 ∫ dt/t

Integrate the above equation then, we get

= 1/2 log|t| + c

= 1/2 log|3cosx + 2sinx| + c

Hence, I = 1/2 log|3cosx + 2sinx| + c

问题 27. 计算 ∫ cos2x + x + 1/ (x ² + sin2x + 2x) dx

解决方案：

Let us assume I = ∫ cos2x + x + 1/ (x² + sin2x + 2x) dx                               (i)

Let x² + sin2x + 2x = t

d(x² + sin2x + 2x) = dt

(2x + 2cos2x + 2) dx = dt

2(x + cos2x + 1) dx = dt

(x + cos2x + 1) dx = dt/2

Put all these values in equation(i), we get

= 1/2 ∫ dt/t

Integrate the above equation then, we get

= 1/2 log|t| + c

= 1/2 log|x² + sin2x + 2x| + c

Hence, I = 1/2 log|x² + sin2x + 2x| + c

问题 28. 计算 ∫ 1/ cos(x + a) cos(x + b) dx

解决方案：

Let us assume I = ∫ 1/ cos(x + a) cos(x + b) dx

On multiplying and dividing the above equation by sin[(x + b) – (x + a)], we get

= $∫ \frac{1}{cos(x+a) cos(x+b)} × \frac{sin[(x +b)-(x+a)]}{ sin[(x +b)-(x+a)]} dx$

= $∫ \frac{1}{ sin[(x +b)-(x+a)]} × \frac{sin[(x +b)-(x+a)]}{ cos(x+a) cos(x+b)} dx$

= $∫ \frac{1}{sin(b-a)} × \frac{sin[(x +b)cos(x+a)-sin(x+a)cos(x+b)]}{ cos(x+a) cos(x+b)} dx$

= $\frac{1}{sin(b-a)} ∫ \frac{[sin(x +b)cos(x+a)]}{cos(x+a) cos(x+b)} dx- ∫ \frac{[sin(x+a)cos(x+b)]}{ cos(x+a) cos(x+b)} dx$

 = 1/ sin(b – a) ∫ tan(x + b) dx – ∫ tan(x + a) dx

Integrate the above equation then, we get

= 1/ sin(b – a) [log(sec(x + b)) – log(sec(x + a))] + c

Hence, I = 1/ sin(b – a) [log(sec(x + b)/sec(x + a))] + c

问题 29. 计算 ∫ -sinx + 2cosx/(2sinx + cosx) dx

解决方案：

Let us assume I = ∫ -sinx + 2cosx/(2sinx + cosx) dx ………..(i) 

Let 2sinx + cosx = t 

d(2sinx + cosx) = dt

(2cosx – sinx) dx = dt

(-sinx + 2cosx) dx = dt

Put all these values in equation(i), we get

= ∫ dt/t

Integrate the above equation then, we get

= log|t| + c

= log|2sinx + cosx| + c

Hence, I = log|2sinx + cosx| + c

问题 30. 计算 ∫ cos4x – cos2x/ (sin4x – sin2x) dx

解决方案：

Let us assume I = ∫ cos4x – cos2x/ (sin4x – sin2x) dx

= – ∫ 2sin3x sinx / 2cos3x sinx dx     

= – ∫ sin3x / cos3x dx ………..(i) 

 Let cos3x = t

d(cos3x) = dt

-3sin3x dx = dt

– sin3x dx = dt/3

Put all these values in equation(i), we get

= 1/3 ∫ dt/t

Integrate the above equation then, we get

= 1/3 log|t| + c

= 1/3 log|cos3x| + c

Hence, I = 1/3 log|cos3x| + c

问题 31. 计算 ∫ secx/ log(secx + tanx) dx

解决方案：

Let us assume I = ∫ secx/ log(secx + tanx) dx  ………..(i) 

Let log(secx + tanx) = t                 

d(log(secx + tanx) = dt

secx dx = dt  

Put all these values in equation(i), we get

= ∫ dt/t

Integrate the above equation then, we get

= log |t| + c

= log |log(secx + tanx)| + c

Hence I = log |log(secx + tanx)| + c

问题 32. 计算 ∫ cosecx/log|tanx/2| dx

解决方案：

Let us assume I = ∫ cosecx/ log|tanx/2| dx    ………..(i) 

Let log|tanx/2| = t           

d(log|tanx/2|) = dt

cosec x dx = dt                              

Put all these values in equation(i), we get

= ∫ dt/t

Integrate the above equation then, we get

= log |t| + c

= log |log tanx/2| + c

Hence, I = log |log tanx/2| + c

问题 33. 计算 ∫ 1/ xlogxlog(logx) dx

解决方案：

Let us assume I = ∫ 1/ xlogxlog(logx) dx   ………..(i) 

Put log(logx) = t     

d(log(logx)) = dt

1/ xlogx dx = dt

Put all these values in equation(i), we get

= ∫ dt/t

Integrate the above equation then, we get

= log |t| + c

= log |log(logx)| + c

Hence, I = log |log(logx)| + c 

问题 34. 计算 ∫ cosec ² x/ 1 + cot x dx

解决方案：

Let us assume I = ∫ cosec² x/ 1+cot x dx   ………..(i) 

Put 1 + cotx = t          then,

d(1 + cotx) = dt

– cosec² x dx = dt

Put all these values in equation(i), we get

= – ∫ dt/t

Integrate the above equation then, we get

= – log |t| + c

= – log |1 + cotx| + c

Hence, I = – log |1 + cotx| + c

问题 35. 计算 ∫ 10x ⁹ + 10 ^x log _e 10/ (10 ^x + x ¹⁰ ) dx

解决方案：

Let us assume I= ∫ 10x⁹ + 10^x log_e 10/ (10^x + x¹⁰)dx  ………..(i) 

Put 10^x + x¹⁰ = t             

d(10^x + x¹⁰) = dt

(10^x log_e 10 + 10x⁹) dx = dt 

Put all these values in equation(i), we get

= ∫ dt/t

Integrate the above equation then, we get

= log |t| + c

= log |10^x + x¹⁰| + c

Hence, I = log |10^x + x¹⁰| + c

问题 36. 计算 ∫ 1 – sin2x/ x + cos ² x dx

解决方案：

Let us assume I = ∫ 1 – sin2x/ x + cos²x dx  ………..(i) 

Put x + cos²x = t      

d(x + cos²x) = dt

(1 – 2sinxcosx) dx = dt

(1 – sin2x) dx = dt

Integrate the above equation then, we get

= ∫ dt/t

Integrate the above equ then, we get

= log |t| + c

= log |x + cos²x| + c

Hence, I = log |x + cos²x| + c

问题 37. 计算 ∫ 1 + tanx/ x + logsecx dx

解决方案：

Let us assume I = ∫ 1 + tanx/ x + logsecx dx  ………..(i) 

Put x + logsecx = t            

d(x+logsecx) = dt

(1 + tanx) dx = dt

Put all these values in equation(i), we get

= ∫ dt/t

Integrate the above equation then, we get

= log |t| + c

= log |x + log secx| + c

Hence, I = log |x + log secx| + c

问题 38. 计算 ∫ sin2x/ a ² + b ² sin ² x dx

解决方案：

Let us assume I = ∫ sin2x/ a² + b²sin²x dx  ………..(i) 

Put a² + b²sin²x = t      

d(a² + b²sin²x) = dt

b²(2sinxcosx) dx = dt

sin2x dx = dt/b²

Put all these values in equation(i), we get

= 1/b² ∫ dt/t

Integrate the above equation then, we get

= 1/b² log |t| + c

= 1/b² log |a² + b²sin²x| + c

Hence, I = 1/b² log |a² + b²sin²x| + c

问题 39. 计算 ∫ x + 1/ x(x + logx) dx

解决方案：

Let us assume I = ∫ x + 1/ x(x + logx) dx   ………..(i) 

Put x + logx = t           

d(x + logx) = dt

(1 + 1/x) dx = dt

(x +1)/ x dx = dt

Put all these values in equation(i), we get

= ∫ dt/t

Integrate the above equation then, we get

= log |t| + c

= log |x + logx| + c

Hence, I = log |x + logx| + c

问题 40. 评估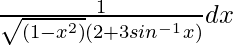$∫ \frac{1}{ \sqrt{(1 - x^2)} (2 + 3sin^{-1}x)} dx$

解决方案：

Let us assume I = $∫ \frac{1}{ \sqrt{(1 - x^2)} (2 + 3sin^{-1}x)} dx$    ………..(i) 

Put 2 + 3sin^-1x = t           

d(2 + 3sin^-1x) = dt

(3 × 1/ √(1 – x²)) dx = dt

(1/ √(1 – x²)) dx = dt/3

Put all these values in equation(i), we get

= 1/3 ∫ dt/t

Integrate the above equation then, we get

= 1/3 log |t| + c

= 1/3 log |2 + 3sin^-1x| + c

Hence, I = 1/3 log |2 + 3sin^-1x| + c

问题 41. 计算 ∫ sec ² x/ tanx + 2 dx

解决方案：

Let us assume I = ∫ sec²x/ tanx + 2 dx ………..(i) 

Put tanx + 2 = t          

d(tanx + 2) = dt

(sec²x) dx = dt

Put all these values in equation(i), we get

= ∫ dt/t

Integrate the above equation then, we get

= log |t| + c

= log |tanx + 2| + c

Hence, I = log |tanx + 2| + c

问题 42. 计算 ∫ 2cos2x + sec ² x/ sin2x + tanx – 5 dx

解决方案：

Let us assume I = ∫ 2cos2x + sec²x/ sin2x + tanx – 5 dx   ………..(i) 

Put sin2x + tanx – 5 = t         

d(sin2x + tanx – 5) = dt

(2cos2x + sec²x) dx = dt

Put all these values in equation(i), we get

= ∫ dt/t

Integrate the above equation then, we get

= log |t| + c

= log |sin2x + tanx – 5| + c

Hence, I = log |sin2x + tanx – 5| + c

问题 43. 计算 ∫ sin2x/ sin5xsin3x dx

解决方案：

Let us assume I = ∫ sin2x/ sin5xsin3x dx

= ∫ sin(5x – 3x)/ sin5xsin3x dx

= ∫ (sin5x cos3x – cos5x sin3x)/ sin5xsin3x dx        [Using formula: sin(a-b) = sina cosb – cosa sinb]

= ∫ (sin5x cos3x)/ sin5xsin3x dx – ∫ (cos5x sin3x)/ sin5xsin3x dx

= ∫ cos3x/sin3x dx – ∫ cos5x/sin5x dx

= ∫ cot3x dx – ∫ cot5x dx 

Integrate the above equation then, we get

= 1/3 log|sin3x| – 1/5 log|sin5x| + c  

Hence, I = 1/3 log|sin3x| – 1/5 log|sin5x| + c

问题 44. 计算 ∫ 1 + cotx/ x + logsinx dx

解决方案：

Let us assume I = ∫ 1 + cotx/ x + logsinx dx    ………..(i) 

Put x + logsinx = t          

d(x + logsinx) = dt

(1 + cotx) dx = dt                    

Put all these values in equation(i), we get

= ∫ dt/t

Integrate the above equation then, we get

= log |t| + c

= log |x + log sinx| + c

Hence, I = log |x + log sinx| + c

问题 45. 计算 ∫ 1/ √x (√x + 1) dx

解决方案：

Let us assume I = ∫ 1/ √x (√x + 1) dx    ………..(i) 

Put √x + 1 = t            

 d(√x + 1) = dt

(1/2√x) dx = dt

(1/√x) dx = 2dt                        

Put all these values in equation(i), we get

= 2∫ dt/t

Integrate the above equation then, we get

= 2 log |t| + c

= 2 log |√x + 1| + c

Hence, I = 2 log |√x + 1| + c

问题 46. 评估 ∫ tan2x tan3x tan 5x dx

解决方案：

Let us assume I = ∫ tan2x tan3x tan 5x dx   ………..(i) 

Now,

tan(5x) = tan(2x + 3x)

tan(5x) = tan2x + tan3x/ (1 – tan2x tan3x)      [By using formula: tan(a + b) = tan a + tan b/ (1- tana tanb)]

tan(5x)(1 – tan2x tan3x) = tan2x + tan3x

(tan5x-tan2x tan3x tan5x) = tan2x + tan3x

tan2x tan3x tan5x = tan5x – tan2x – tan3x      ………..(ii) 

Using equation (i) and equation (ii), we get

= ∫ tan5x – tan2x – tan3x dx

Integrate the above equation then, we get

= 1/5 log|sec5x| – 1/2log|sec2x| -1/3log|sec3x| + c

Hence, I = 1/5 log|sec5x| – 1/2log|sec2x| – 1/3log|sec3x| + c

问题 47. 计算 ∫ {1 + tanx tan(x + θ)} dx

解决方案：

Let us assume I = ∫ {1 + tanx tan(x + θ)}  dx    ………..(i) 

As we know that,

tan(a – b) = tan a – tan b/ (1+ tana tanb)

tan(x + θ – x) = tan (x + θ) – tan x/ (1+ tan(x + θ) tanx)

tan θ = tan (x + θ) – tan x/ (1+ tan(x + θ) tanx)

(1+ tan(x + θ) tanx) = tan (x + θ) – tan x/tan θ    ………..(ii) 

By using equation (i) and (ii), we get

= ∫ tan (x + θ) – tan x/ tan θ dx

= 1/tan θ ∫ tan (x + θ) – tan x dx

Integrate the above equation then, we get

= 1/tan θ [-log|cos(x + θ)| + log |cosx|] + c

= 1/tan θ [log |cosx| – log|cos(x + θ)|] + c

Hence, I = 1/tan θ [log {cosx/ cos(x + θ)}] + c

问题 48. 计算 ∫ sin2x/ sin(x – π/6)sin(x + π/6) dx

解决方案：

Let us assume I = ∫ sin2x/ sin(x – π/6)sin(x + π/6) dx    ………..(i) 

= ∫ sin2x/ sin²x – sin²π/6 dx

= ∫ sin2x/ sin²x – 1/4 dx

Put sin²x – 1/4 = t    

d(sin²x – 1/4) = dt

(2sinx cosx) dx = dt

(sin2x) dx = dt                        

Put all these values in equation(i), we get

= ∫ dt/t

Integrate the above equation then, we get

= log |t| + c

= log |√x + 1| + c

Hence, I = log |sin²x – 1/4| + c

问题 49. 计算 ∫ e ^x-1 + x ^e-1 / e ^x + x ^e dx

解决方案：

Let us assume I = ∫ e^x-1 + x^e-1/ e^x + x^e dx    ………..(i) 

= 1/e ∫ e^x + ex^e-1/ e^x + x^e dx

= 1/e ∫ e^x + ex^e-1/ e^x + x^e dx

Put e^x + x^e= t      

d(e^x + x^e) = dt

(e^x + ex^e-1) dx = dt

(e^x + ex^e-1) dx = dt                        

Put all these values in equation(i), we get

= 1/e ∫ dt/t

Integrate the above equation then, we get

= 1/e log |t| + c

= 1/e log |e^x + x^e| + c

Hence, I = 1/e log |e^x + x^e| + c

问题 50. 计算 ∫ 1/sinx cos ² x dx

解决方案：

Let us assume I = ∫ 1/sinx cos²x dx

= ∫sin²x + cos²x/sinx cos²x dx

= ∫sin²x/sinx cos²x + cos²x/sinx cos²x dx

= ∫sinx/ cos²x + cosecx dx

= ∫secx tanx dx +∫ cosecx dx

Integrate the above equation then, we get

= sec x + log|tanx/2| + c

Hence, I = sec x + log|tanx/2| + c

问题 51. 计算 ∫ 1/cos3x – cosx dx

解决方案：

Let us assume I = ∫ 1/cos3x – cosx dx

∫ 1/cos3x – cosx dx = ∫ sin²x + cos²x / 4cos³x – 4cosx dx

= ∫ sin²x + cos²x / 4cos(cos²x – 1) dx

= -1/4 ∫ sin²x/ sin²xcosx dx + ∫ cos²x / sin²xcosx dx

= -1/4 ∫ secx + cosecx cotx dx

Integrate the above equation then, we get

= -1/4 [log|secx + tanx| – cosecx] + c

Hence, I = 1/4 [cosecx + log|secx + tanx|] + c