解决线性不等式单词问题

我们精通多变量方程。线性方程表示一维中的点、二维中的线和三维世界中的平面。线性不等式的解表示笛卡尔平面的一个区域。我们必须知道如何将现实生活中的问题转化为线性不等式。

线性不等式

在正式定义线性不等式之前，让我们通过现实生活中的情况来了解它们，并首先观察为什么它们的需求会出现。假设阿尔伯特去书展给自己买了一些小说。他总共有200卢比。书展有一项特殊的销售政策，任何书籍的价格为 70 卢比。现在他知道他可能无法将全部金额花在书籍上。假设 x 是他买的书的数量。这种情况可以用以下等式在数学上表示，

70x < 200

由于他不能把所有的钱都花在书本上，而且他花的钱总是少于 200 卢比。目前的情况只能用上面给出的公式来表示。现在让我们用正式的描述来研究线性不等式，

Two real numbers or two algebraic expressions which are related by symbols such as ‘>’, ‘<‘, ‘≥’ and’≤’ form the inequalities. Linear inequalities are formed by linear equations which are connected with these symbols. These inequalities can be further classified into two parts: 

1. Strict Inequalities: The inequalities with the symbols such as ‘>’ or ‘<‘.
2. Slack Inequalities: The inequalities with the symbols such as ‘≥’ or ‘≤’.

求解线性不等式的规则：

在解决线性不等式时，我们应该牢记某些规则。

1. 不等式两边可以加上或减去相等的数，而不会影响其符号。
2. 不等式的两边都可以除以或乘以任何正数，但当它们乘以或除以负数时，线性不等式的符号相反。

现在通过对线性不等式的简要介绍，让我们看看关于这个概念的一些文字问题。

示例问题

问题1：考虑开头给出的问题。艾伯特去书展给自己买了一些小说。他总共有200卢比。书展有一项特殊的销售政策，任何书籍的价格为 70 卢比。现在他知道他可能无法将全部金额花在书籍上。假设 x 是他买的书的数量。用数学和图形方式表示这种情况。

解决方案：

We know that Albert cannot buy books for all the money he has. So, let’s say the number of books he buys is “x”. Then, 

70x < 200

⇒ x < $\frac{20}{7}$

To plot the graph of this inequality, put x = 0. 

0 < $\frac{20}{7}$ Thus, x = 0 satisfies the inequality. So, the graph for the following inequality will look like, 

问题2：考虑足球俱乐部皇家马德里队的前锋在最近3场比赛中的表现。罗纳尔多和本泽马在最近三场比赛中总共打进了不到9球。众所周知，C罗比本泽马多进了三个球。罗纳尔多可能打进多少球？

解决方案：

Let’s say the number of goals scored by Benzema and Ronaldo are y and x respectively. 

x = y + 3 …..(1)

x + y < 9  …..(2) 

Substituting the value of x from equation (1) in equation (2). 

y + 3 + y < 9 

⇒2y  < 6 

⇒y < 3

Possible values of y: 0,1,2 

Possible values of x: 3,4,5

问题 3：一间教室至少可以放 9 张桌子，面积为 1 平方米。我们知道教室的周长是 12m。找出教室的长度和宽度的界限。

解决方案：

It can fit 9 tables, that means the area of the classroom is atleast 9m². Let’s say the length of the classroom is x and breadth is y meters. 

2(x + y) = 12 {Perimeter of the classroom}

⇒ x + y = 6 

Area of the rectangle is given by, 

xy > 9 

⇒x(6 – x) > 9 

⇒6x – x² > 9 

⇒ 0 > x² – 6x + 9 

⇒ 0 > (x – 3)²

⇒ 0 > x – 3 

⇒ x < 3 

Thus, length of the classroom must be less than 3 m. 

So, then the breadth of the classroom will be greater than 3 m. 

问题 4：为下列情况制定线性不等式并绘制其图形。

假设 Aman 和 Akhil 去了一家文具店。 Aman 买了 3 个笔记本，Akhil 买了 4 本书。假设每个笔记本的成本是“x”，每本书是“y”。总支出不到500卢比。

解决方案：

Cost of each notebook was “x” and for each book, it was “y”. Then the inequality can be described as,

3x + 4y < 500

Putting (x,y) → (0,0) 

3(0) + 4(0) < 500

Origin satisfies the inequality. Thus, the graph of its solutions will look like, x.

问题 5：为下列情况制定线性不等式并绘制其图形。

一家音乐商店以成本价的五倍出售其吉他。如果店主的利润超过 3000 卢比，请找出店主的最低成本价。

解决方案：

Let’s say the selling price of the guitar is y, and the cost price is x.

y – x > 3000 ….(1)

It is also given that, 

y = 5x ….(2) 

Substituting the value of y from equation (2) to equation (1). 

5x – x > 3000 

⇒ 4x > 3000 

⇒  x > $\frac{3000}{4}$

⇒ x> 750 

Thus, the cost price must be greater than Rs 750. 

问题6：长方形的长是宽的4倍。矩形的周长小于 20。为给定的情况在两个变量中建立一个线性不等式，绘制它的图形并计算长度和宽度的界限。

解决方案：

Let’s say the length is “x” and breadth is “y”. 

Perimeter = 2(x + y) < 20 ….(1)

⇒ x + y < 10 

Given : x = 4y 

Substituting the value of x in equation (1). 

x + y < 10

⇒ 5y < 10 

⇒ y < 2

So, x < 8 and y < 2. 

问题 7：Rahul 和 Rinkesh 在同一支球队踢球。上一场比赛，拉胡尔的进球数比林克什多2个，但加起来还不到8球。求解线性不等式并将其绘制在图表上。

解决方案：

The equations obtained from the given information in the question,

Suppose Rahul scored x Number of goals and Rinkesh scored y number of goals,

Equations obtained will be, x = y+2 ⇢ (1)

x+y< 8 ⇢ (2)

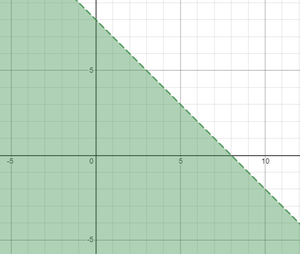
Solving both the equations,

y+2 + y < 8

2y < 6

y< 3

Putting this value in equation (2),

x< 5

问题 8：一个班有 100 名学生，女生比男生多，可以推断出女生有多少吗？

解决方案：

Let’s suppose that B is denoted for boys and G is denoted for girls.

Now, since Girls present in the class are more than boys, it can be written in equation form as,

G > B 

The total number of students present in class is 100 (given),

It can be written as, G+ B= 100

B = 100- G

Substitute G> B in the equation formed,

G> 100 – G

2G > 100

G> 50

Hence, it is fixed that the number of girls has to be more than 50 in class, it can be 60, 65, etc. Basically any number greater than 50 and less than 100.

问题 9：在上一个问题中，女孩的数量是否有可能正好是 50 或正好是 100？如果不是，那为什么？

回答：

No, It is not possible for the Number of girls to be exactly 50 since while solving, it was obtained that, G> 50 

In any case if G= 50 is a possibility, from equation G+ B= 100, B = 50 will be obtained.

This simply means that the number of boys is equal to the number of girls which contradicts to what is given in the question.

No, it is not possible for G to be exactly 100 as well, as this proves that there are 0 boys in the class.

问题 10：求解线性不等式并绘制相同的图形，

7x+ 8y < 30

x= y/2

解决方案：

The linear inequality is given as, 7x+ 8y< 30 

At x= 0, y= 30/8= 3.75

At y= 0, x= 30/7= 4.28

These values are the intercepts.

The graph for the above shall look like, 

Putting x= y/2, that is, y= 2x in the linear inequality,

7x + 16x < 30

x = 1.304

y = 2.609