三角形的面积–坐标几何| 10级数学

坐标几何定义为使用平面上任意尺寸的坐标点对几何进行的研究。使用坐标几何，可以找到两点之间的距离，以比例划分线，找到线的中点，计算笛卡尔平面中三角形的面积，等等。

根据给定的参数，有多种方法可以找到三角形的面积，例如三角形的底边和高度，顶点的坐标，边的长度等。以下是找到三角形面积的3种方法。

方法1：使用三角形的底和高

当给出三角形的底数和高度时，我们将使用此方法，该方法是所有方法中最简单的。对于给定的三角形，如果三角形的高度为“ h ”且三角形的底数为“ b ”，则三角形的面积为：

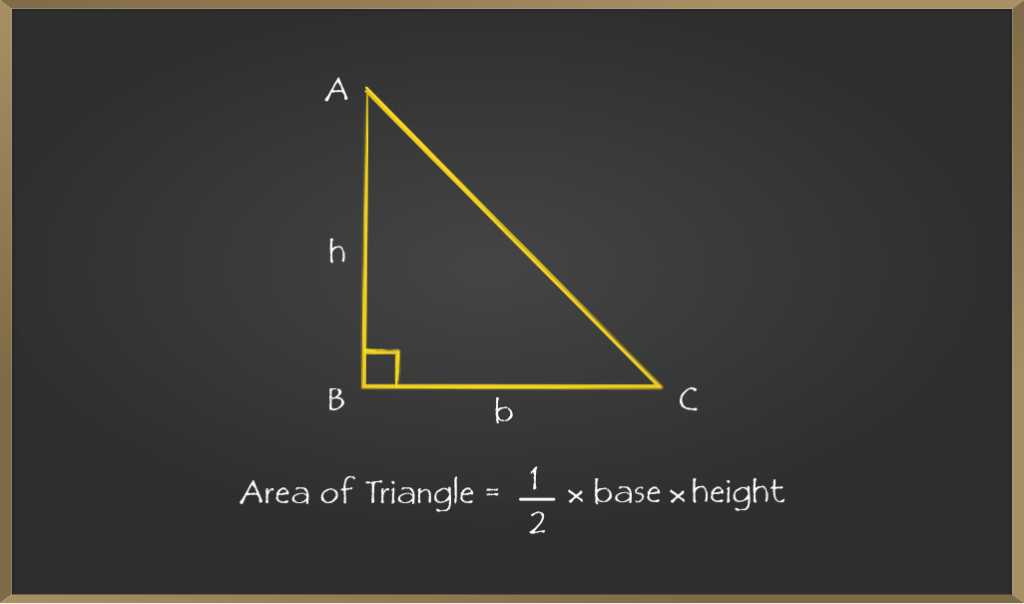
公式的推导

步骤1：考虑直角三角形ABC。

步骤2：现在从点A画一条水平线，从点C画一条垂直线。让点D成为两条线相交的点。

第3步：图形将看起来像一个矩形，即，如果我们添加2个相似的三角形，则会生成一个矩形。

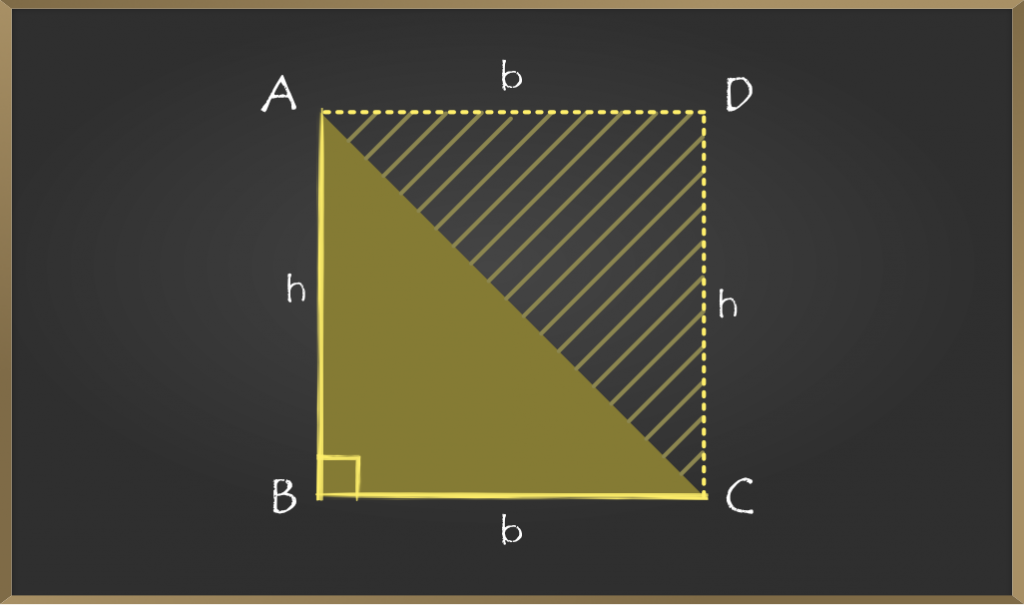
步骤4：由于我们需要三角形ABC的面积，因此我们可以将其写为(矩形ABCD / 2的面积)。

步骤5：继续执行第4步：

=> Area of ABC = (area of rectangle ABCD / 2)

=> Area of ABC = (b × h) / 2

Hence, proved that area of the triangle is (1 / 2) × b × h

公式中的样本问题

示例1：找到高度和底边分别为6厘米和5厘米的三角形的面积?

解决方案：在问题中，明确提到高度和底数是：

Given, h = 6 and b = 5

Area of triangle is given as =  (1 / 2) × b × h

=> (1 / 2) × 6 × 5

=> 3 × 5 = 15

Hence, the area of the given triangle is 15 cm²

示例2：找到面积为12 cm ²且底边为6 cm的三角形的高度?

解决方案：

Given, area = 12 and b = 6

Area of triangle is = (1 / 2) × b × h

=> 12 = (1 / 2) × 6 × h

=> h = 12 / 3 = 4

Hence, the height of the given triangle is 4 cm.

方法2：使用苍鹭公式

如果未给出三角形的底边和高度，则如果给出三角形的边，则可以使用Heron公式。

如果a，b，c是三角形的边，则三角形的面积为：

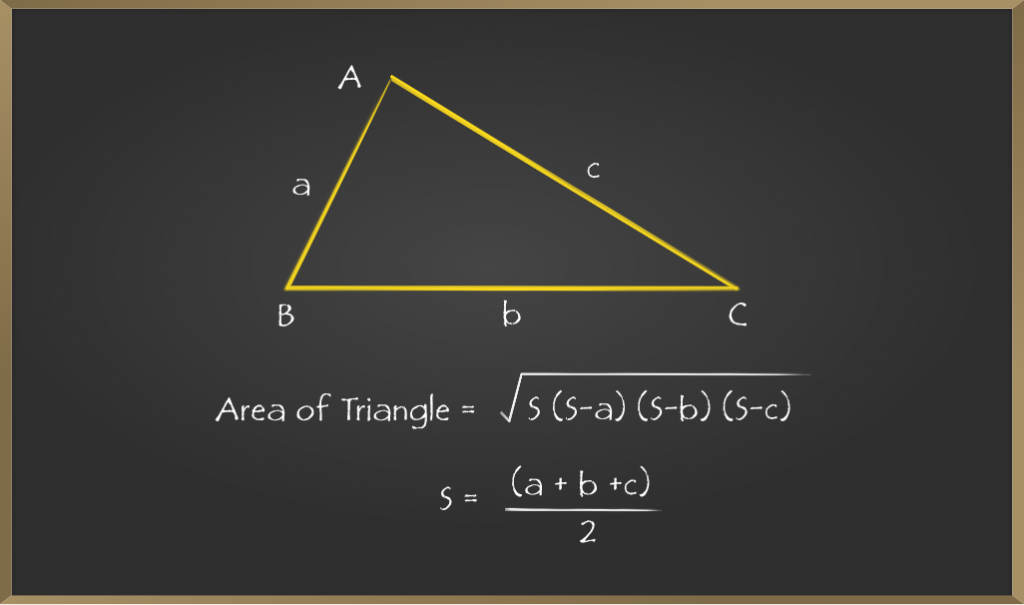
公式的推导

步骤1：众所周知，半周长s =(a + b + c)/ 2

=> 2s = a + b + c

=> 2s – 2a = a + b + c – 2a         [subtracting both sides 2a]

=> 2(s – a) = b + c – a        —————1

Similarly,

=> 2(s – b) = c + a – b       —————2

=> 2(s – c) = a + b – c        —————3

We will be using these relations later in the derivation.

第2步：现在，让我们看一下侧面a，b，c的斜角三角形

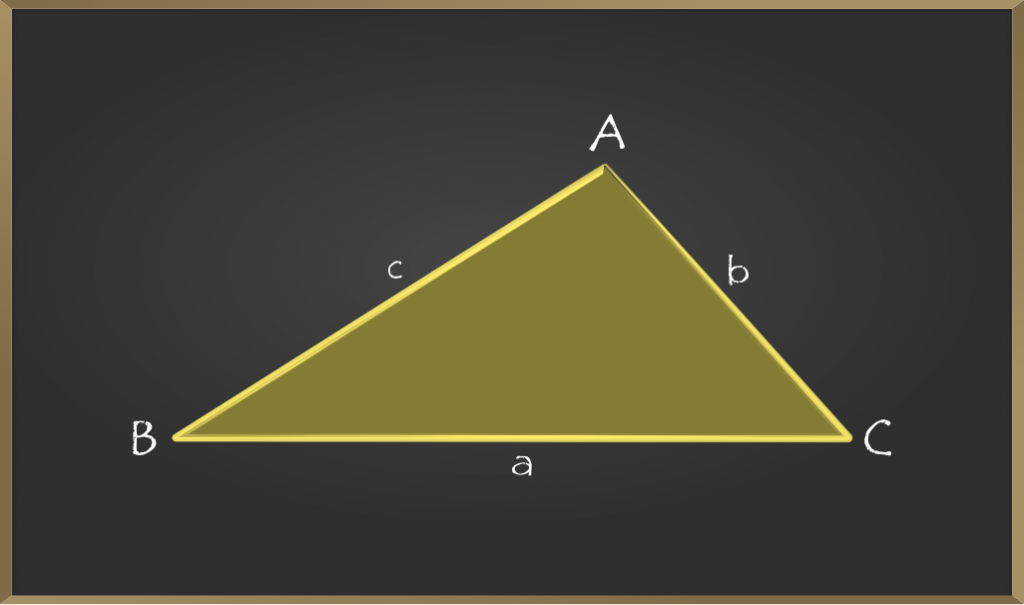
步骤3：由于我们没有三角形的高度或高度，因此从BC的A垂直于D绘制一个垂直线，其底数为’a’。

步骤4：现在，如果我们清楚地观察到，形成了两个三角形，分别为ΔABD和 ∆ ADC 。如果BD的长度是d，则DC的长度将是– d。

步骤5：现在，在毕达哥拉斯定理的三角形ABD中

=> h² = c² – d²      ——————–4

Similarly in ∆ADC

=> h² = b² – (a – d)²

From equation 4 substitute value of h²

=> c² – d² = b² – (a – d)²

=> c² – d² = b² – (a² + d² – 2ad)

After simplification of the equation we will get,

d = (c² + a² + b²) / 2a

Now substitute above value in the equation 4

=> h² = c² – [(c² + a² + b²) / 2a]²

=> h² = (c – {(c² + a² + b²) / 2a})(c + {(c² + a² + b²) / 2a})        because [a² – b² = (a + b)(a – b)]

=> h² = (1 / 4a²)[ b² – (a – c)²][(a + c)² – b²]

=> h² = (1 / 4a²)[(b – a + c)(b + a – c)(a + c – b)(a + b + c)]      because [a² – b² = (a + b)(a – b)]

From equation 1, 2 and 3 substitutes into above equation

=> h² = (1 / 4a²) [2(s – a) × 2(s – b) × 2(s – c) × 2s]

=> h² = (4 / a²) [s(s – a)(s – b)(s – c)]

=> h = (2 / a) √[s(s – a)(s – b)(s – c)]              ————–5

步骤6：从方法1中我们知道是否给定了三角形的底边和高度，那么三角形的面积为(底边×高度)/ 2。现在用此公式替换高度

=> area of ABC = (1 / 2) × a × (2 / a) √[s(s – a)(s – b)(s – c)] 

After simplification

=> area of ABC = √[s(s – a)(s – b)(s – c)] 

因此，证明了三角形面积的苍鹭公式。

苍鹭公式的样本问题

示例1：如果三角形的边分别为3 cm，4 cm和5 cm，则找到三角形的面积。

解决方案：

Let a = 3, b = 4, and  c = 5 

First, we have to find semi perimeter 

=> s = (a + b + c) / 2

=> s = (3 + 4 + 5) / 2 

=> s = 12 / 2 = 6

As we know heron’s formula is √[s(s – a)(s – b)(s – c)], so substituting values in it

=> √[s(s – a)(s – b)(s – c)]

=> √[6(6 – 3)(6 – 4)(6 – 5)]

=> √[6 × 3 × 2 × 1]

=> √36 = 6

The area of the triangle is 6 cm²

示例2：使用苍鹭公式推导公式，以求出边为a的等边三角形的面积

解决方案：

To find semi perimeter

=> s = (a + b + c) / 2

=> s = (a + a + a ) / 2

=> s = 3a / 2

Now using heron’s formula

=> √[s(s – a)(s – b)(s – c)]

=> √[(3a / 2)((3a / 2) – a)((3a / 2) – a)((3a / 2) – a)]

=> √[(3a / 2)(a / 2)(a / 2)(a / 2)]

=> √(3a⁴ / 16)

=> √3(a²) / 4 

Hence area of a equilateral triangle is  √3(a²) / 4

方法3：使用顶点的坐标

在前面的方法中，我们看到了不同的条件，在方法3中，如果给出了三角形的坐标，则将看到如何找到三角形的面积。

如果三角形的坐标为(x1，y1)，(x2，y2)和(x3，y3)，则三角形的面积为

公式的推导

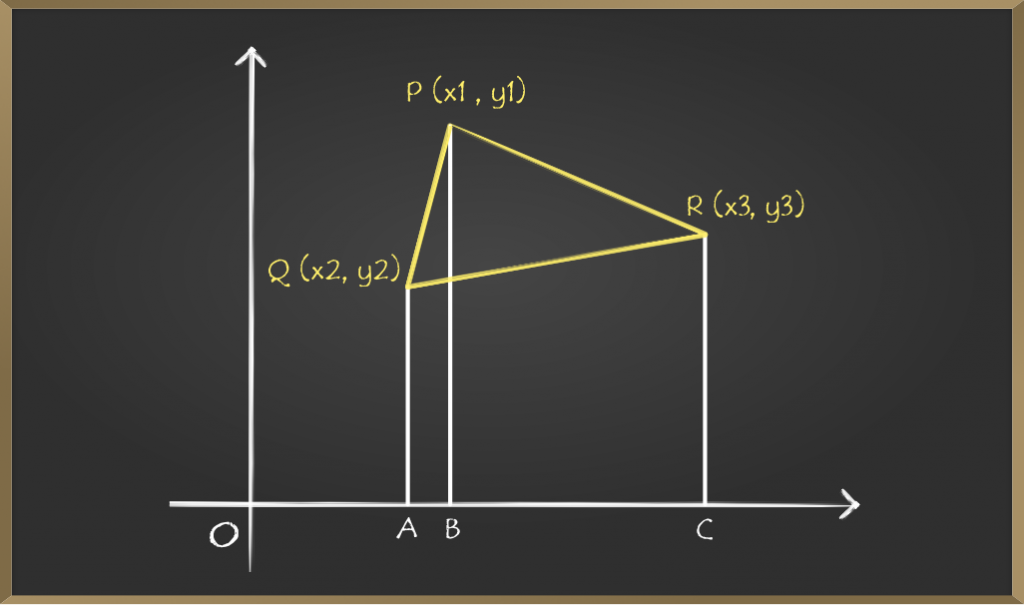
步骤1：分别在A，B和C处绘制从坐标P，Q和R到X轴的垂直线。

步骤2：现在，如果我们仔细看一下图，会在坐标平面中形成三个不同的梯形，例如PQAB，PBCR和QACR。

步骤3：因此， ΔQPR的面积计算为

Area of ∆PQR = [Area of trapezium PQAB + Area of trapezium PBCR] – [Area of trapezium QACR]      —-(1)

步骤4：现在计算所有3个梯形的面积。

Since Area of a trapezium = (1 / 2) (sum of the parallel sides) × (distance between sides)

梯形PQAB的发现区域

=> Area of trapezium PQAB = (1 / 2)(QA + PB) × AB

=> QA = y2

=> PB = y1

=> AB = OB – OA = x1 – x2

=> Area of trapezium PQAB = (1 / 2)(y1 + y2)(x1 – x2 ) —-(2)

梯形PBCR的发现区域

=>Area of trapezium PBCR =(1 / 2) (PB + CR) × BC

=>PB = y1

=>CR = y3

=>BC = OC – OB = x3 – x1

=>Area of trapezium PBCR =(1 / 2) (y1 + y3 )(x3 – x1) —-(3)

梯形QACR的发现区域

=>Area of trapezium QACR = (1 / 2) (QA + CR) × AC

=>QA = y2

=>CR = y3

=>AC = OC – OA = x3 – x2

=>Area of trapezium QACR =(1 / 2)(y2 + y3 ) (x3 – x2 )—-(4)

步骤5：将(2)，(3)和(4)替换为(1)，

=> Area of ∆PQR = (1 / 2)[(y1 + y2)(x1 – x2 ) + (y1 + y3 )(x3 – x1) – (y2 + y3 ) (x3 – x2 )]

=> Area of ∆PQR = (1 / 2) |[x1 (y2 – y3 ) + x2 (y3 – y1 ) + x3(y1 – y2)]|

因此，这是在给出坐标的情况下找到三角形面积的公式。

Note: Observe that there is a mod, which indicates that, if we got a negative value we should only consider the numerical value as the area can’t be negative.

公式中的样本问题

示例1：顶点为A(1，2)，B(4，2)和C(3，5)的∆ABC面积是多少?

解决方案：

首先，让我们画一个图以更好地理解。

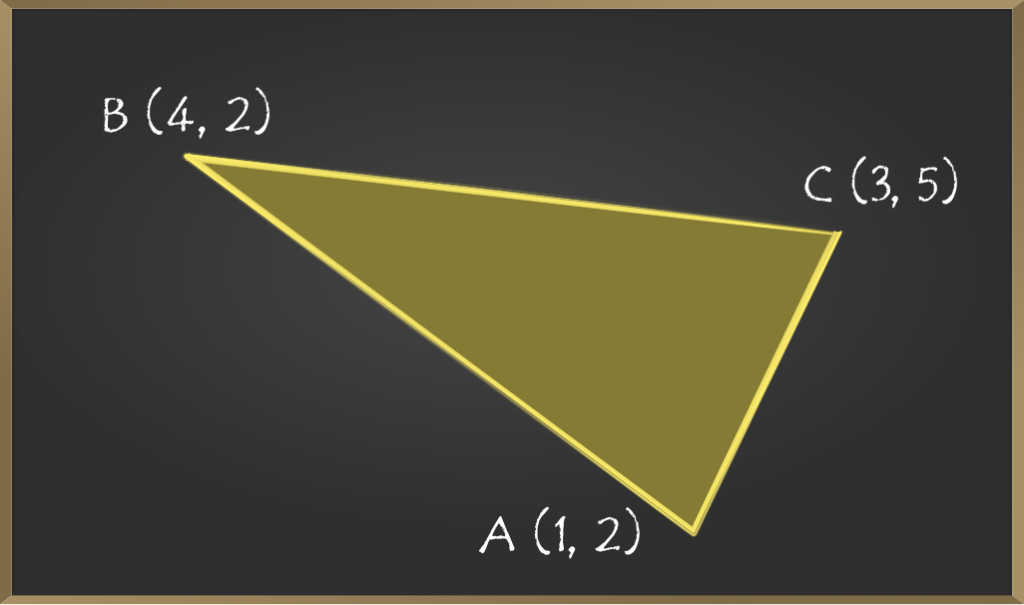
现在将给定坐标与(x1，y1)，(x2，y2)和(x3，y3)进行比较。

Let, (x1, y1) = (1, 2)

=> (x2, y2) = (4, 2)

=> (x3, y3) = (3, 5)

现在我们必须替换(1/2)[x1(y2-y3)+ x2(y3-y1)+ x3(y1-y2)]中的值

=> (1 / 2) [1 (2 – 5 ) + 4 (5 – 2 ) + 3(2 – 2)]

=> (1 / 2) [(- 3) + 12 + 0]

=> (1 / 2) [9] = 4.5

Hence the area of the triangle is 4.5 sq units

示例2：三角形的面积为坐标(x1、1)，(2、3)和(4、5)的1的x1的值是多少?

解决方案：

首先，让我们画一个图以更好地理解。

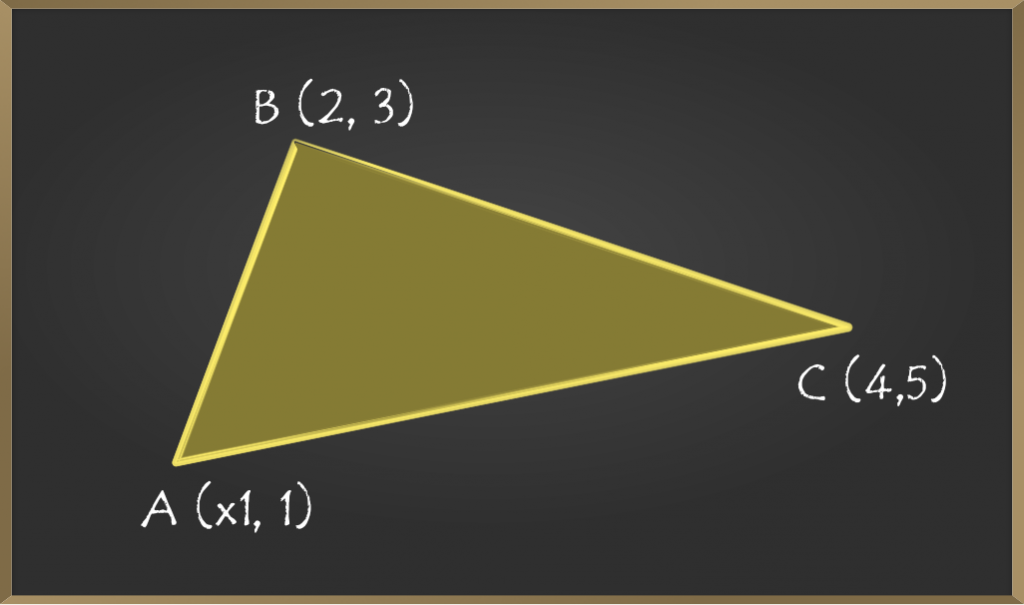
在这个问题中，我们必须找到值“ x1”，它是点A的X坐标。

假定三角形的面积为1。

现在将给定坐标与(x1，y1)，(x2，y2)和(x3，y3)进行比较。

Let, (x1, y1) = (x1, 1)

=> (x2, y2) = (2, 3)

=> (x3, y3) = (4, 5)

现在我们必须替换(1/2)[x1(y2-y3)+ x2(y3-y1)+ x3(y1-y2)]中的值

=> (1 / 2) [x1(3 – 5 ) + 2(5 – 1 ) + 4(1 – 3)] = 1

=> (1 / 2) [x1(- 2) + 8 + -8] = 1

=> -x1 = 1

=> x1 = ±1 square units.

Hence, the value of x1 can be both -1 and 1