评估以下各项(14-19)
问题14。 
解决方案:
Given:![]() -(1)
-(1)
Putting the values of sin 30° = 1/2, tan 30° = 1/√3, tan 60° = √3, sin 90° = cos 0° = 1 in eq(1)
= 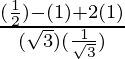
= ![]()
= 3/2
问题15。 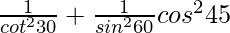
解决方案:
Given: ![]() -(1)
-(1)
= tan230° + cosec260° − cos245°
Putting the values of cosec60° = 2/√3, cos45° = 1/√2, tan30° = 1/√3 in eq(1)
= ![]()
= 4/3 + 4/3 – 1/2
= (4 + 4)/3 – 1/2
= 8/3 – 1/2
= 13/6
问题16. 4(正弦4 30°+余弦2 60°)– 3(余弦2 45°–正弦2 90°)–正弦2 60°
解决方案:
Given: 4(sin430° + cos260°) – 3(cos245° – sin290°) – sin260° -(1)
Putting the values of cos45° = 1/√2, sin30° = cos30° = 1/2, sin60° = √3/2, sin90° = 1 in eq(1)
= 4((1/2)4 + (1/2)2) – 3((1/√2)2 – (1)2) – (√3/2)2
= 4((1/16) + (1/4)) – 3((1/2) – 1) – (3/4)
= 4(5/16) – 3(-1/2) – 3/4
= 5/4 + 3/2 – 3/4
= (5 + 6 – 3)/4
= 8/4
= 2
问题17。 
解决方案:
Given: ![]()
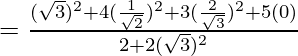
![]()
= 3 + 4(1/2) + 3(4/3)
= 3 + 2 + 4
= 9
问题18:评估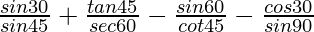
解决方案:
Given: ![]()
= 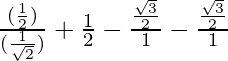
= ![]()
= ![]()
问题19。 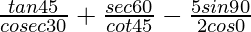
解决方案:
Given: ![]()
As we know that
cosecθ = 1/sinθ, cotθ = 1/tanθ
Now,
= tan45°sin30° + sec60°tan45° – ![]()
= 1 – 1/2 + 2 – 5/2
= (1 – 5)/2 + 2
= -2 + 2
= 0
在以下每个中找到X的值
问题20. 2sin3x =√3
解决方案:
Given,
2sin3x = √3
sin3x = √3/2
As we know that,
sin60° = √3/2
So, sin3x = sin60°
Now,
3x = 60°
x = 60°/3
x = 20°
问题21. 2sin x / 2
解决方案:
Given
2sinx/2 = 1 or sin x/2 = 1/2
We know sin30° = 1/2
So,
sinx/2 = sin30°
x/2 = 30°
x = 60°
问题22.√3sinx= cosx
解决方案:
Given,
√3sinx = cosx or sinx/cosx = 1/√3
tan x = 1/√3
We know tan30° = 1/√3
So,
tan x = tan30°
x = 30°
问题23. tan x = sin45°cos45°+ sin30°
解决方案:
Given: tan x = sin45°cos45° + sin30°
Putting the values of sin 45° = cos 45° = 1/√2, sin 30° = 1/2
tan x = ((1/√2 × 1/√2) + 1/2)
tan x = (1/√2)2 + 1/2
tan x = 1/2 + 1/2
tan x = 1
tan x = tan45°
So, x = 45°
问题24.√3tan2x = cos60°+ sin45°cos45°
解决方案:
Putting the values of sin 45° = cos 45° = 1/√2, cos 60° = 1/2 in the given equation
√3tan 2x = 1/2 + 1/√2 × 1/√2
√3tan 2x = 1/2 + (1/√2)2
√3tan 2x = 1/2 + 1/2
tan 2x = 1/√3
tan 2x = tan30°
So, x = 15°
问题25. cos 2x = cos60°cos30°+ sin60°sin30°
解决方案:
Given: cos 2x = cos60°cos30° + sin60°sin30°
Putting the values of sin 30° = cos 60° = 1/2, cos 30° = sin 60° = √3/2
cos 2x = 1/2 × √3/2 + √3/2 × 1/2
cos 2x = √3/4 + √3/4
cos 2x = √3/2
cos 2x = cos30°
So, x = 15°
问题26.如果θ= 30°,请验证:
(i)tan2θ= 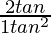
解决方案:
Putting the value of given θ in the above equation
We get
![]()
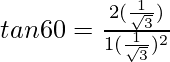
√3 = ![]()
√3 = ![]()
√3 = √3
Hence Proved
(ii)tan2θ= 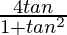
解决方案:
Putting the value of given θ in the above equation
We get
![]()
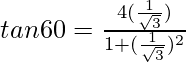
√3 = ![]()
√3 = ![]()
√3 = √3
Hence Proved
(iii)cos2θ= 
解决方案:
Putting the value of given θ in the above equation
We get
![]()
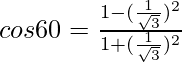
1/2=(3-1)(3+1)
1/2 = 2/4 or 1/2
Hence Proved
(ⅳ)cos3θ= 4cos 3θ – 3cosθ
解决方案:
Putting the value of given θ in the above equation
We get
cos3(30°) = 4cos3 30° − 3cos 30°
cos 90° = 4cos330° − 3cos 30°
![]()
0 = 4(3√3/8) – 4(3√3/8)
0 = 3(√3/2) – 3(√3/2)
0 = 0
Hence Proved