问题27.如果A = B = 60°,请确认
(i)cos(AB)= cos A cos B + sin A sin B
解决方案:
We know sin 60° = √3/2, sin 30° = 1/2
Putting the values of A and B in the equation below
cos(60° − 60°) = cos60°cos60° + sin60°sin60°
cos(0) = cos260° + sin260°
1 = (1/2)2 + (√3/2)2
1 = 1/4 + 3/4
1 = 4/4 = 1
Hence Proved
(ii)sin(AB)= sin A cos B − cos A sin B
解决方案:
Putting the values of A and B in the equation below
sin(60° − 60°) = sin60°cos60° − cos60°sin60°
sin(0°) = (1/2)(√3/2) – (√3/2)(1/2)
0 = √3/4 – √3/4
0 = 0
Hence Proved
(iii)tan(A-B)= 
解决方案:
Putting the values of A and B in the equation below
tan(60° − 60°) = ![]()
tan0 =![]()
0 = 0
Hence Proved
问题28.如果A = 30°且B = 60°,请验证
(i)sin(A + B)= sin A cos B cos A sin B
解决方案:
We know sin 60° = cos 30° = √3/2, sin 30° = cos 60° = 1/2
Putting the values of A and B in the following equations
sin(30° + 60°) = sin 30° cos 60° cos 30° sin 60°
sin 90° = (1/2)(1/2) + (√3/2)(√3/2)
1 = 1/4 + 3/4
1 = 1
Hence Proved
(ii)cos(A + B)= cos A cos B − sin A sin B
解决方案:
Putting the values of A and B in the following equations
cos(30° + 60°) = cos 30° cos 60° – sin 30° sin 60°
cos 90° = (√3/2)(1/2) – (1/2)(√3/2)
0 = √3/4 – √3/4
0 = 0
Hence Proved
问题29.如果sin(A + B)= 1且cos(A-B)= 1,则0°
解决方案:
Given sin(A + B) = 1, cos(A − B) = 1, 0° < A + B ≤ 90° and A ≥ B,
We know that, sin90° = 1 and cos0° = 1
sin(A + B) = 1 and sin90∘= 1
We can say that
(A + B) = 90° -(1)
cos(A − B) = 1 and cos0° = 1
(A − B) = 0°
A = B -(2)
Substituting eq(2) in (1)
A + A = 90°
2A = 90°
A = 45°
A = B = 45°
问题30.如果tan(A + B)=√3且tan(A-B)= 1 /√3,则0° B,找到A和B。
解决方案:
Given tan(A + B) = √3
tan(A − B) = 1/√3
0∘< A + B ≤ 90∘and A > B,
We know that, tan30° = 1/√3 and tan60° = √3
Now,
tan(A + B) = √3 and tan60 = √3
(A + B) = 60° -(1)
Again,
tan(A − B) = 1/√3 and tan30° = 1/√3
(A − B) = 30° -(2)
Solving eq(1) and (2),
(A + B) = 60°
(A − B) = 30°
Solving the above two equations we get
2A = 90°
A = 90°/2 = 45°
问题31.如果sin(A – B)= 1/2且cos(A + B)= 1/2,则0°
解决方案:
Given, sin(A − B) = 1/2
cos(A + B) = 1/2
0° < A + B ≤ 90° and A < B,
We know that, sin30° = 1/2and cos60° = 1/2
Since sin(A − B) = 1/2 and sin30° = 1/2
So (A − B) = 30° -(1)
Again
cos(A + B) = 1/2 and cos60° = 1/2
So (A + B) = 60° -(2)
Solving eq(1) and (2) we get
2A = 90°
A = 90°/2 = 45°
From (2)
(A + B) = 60°
Also, A = 45°
B = 60° − 45°
B = 15°
Therefore, A = 45°, B = 15°
问题32.在与B成直角的∆ABC中,∠A=∠C。查找的值
(i)罪A cos C +罪A A sin C
解决方案:
Given: In ∆ABC right-angled at B, ∠A = ∠C
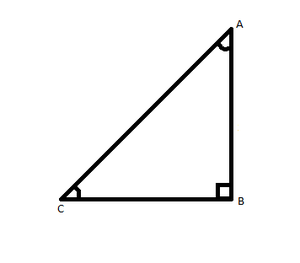
We know in a triangle ∠A + ∠B +∠C = 180°
2∠A = 180° – 90° = 45° [∠B = 90°]
Now,
We have sin A cos C + cos A sin C
Putting the values of each angle
sin 45° cos 45° + cos 45° sin 45°
= (1/√2)(1/√2) + (1/√2)(1/√2)
= 1/2 + 1/2
= 1
(ii)罪A罪B + cos A cos B
解决方案:
We have sin A sin B + cos A cos B
Putting the values of each angle
sin 45° sin 90° + cos 45° cos 90°
= (1/√2)(1) + (1/√2)(0)
= (1/√2) + 0
= 1/√2
问题33.如果sin(A + 2B)=√3/ 2且cos(A + 4B)= 0,并且A> B,则求出锐角A和B。
解决方案:
Given, sin(A + 2B) = √3/2, cos(A + 4B) = 0, A > B,
We know that, sin60° = √3/2 and cos90° = 0
Since sin(A + 2B) = √3/2 and sin60° = √3/2
So (A + 2B) = 60° -(1)
Again cos(A + 4B) = 0 and cos90° = 0
So (A + 4B) = 90° -(2)
From (1) from (2) we get,
2B = 30°
B = 30°/2 = 15°
From (2)
(A + 4B) = 90°
Also, B = 15°
A = 90° − 60°
A = 30°
Therefore, A = 30°, B = 15°
问题34.在ΔPQR中,在Q处成直角,PQ = 3 cm,PR = 6 cm。确定∠P和∠R。
解决方案:
Given In ΔPQR, right-angled at Q, PQ = 3 cm and PR = 6 cm.
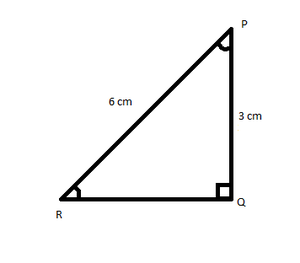
We know cos P = Base/Hypotenuse =3/6
cos 60° = 1/2
So ∠P = 60°
In ΔPQR
∠P + ∠Q + ∠R = 180°
∠R = 180° – 90° – 60°
∠R = 30°
Therefore, ∠P = 60°, ∠R = 30°
问题35.如果sin(A-B)= sin A cos B-cos A sin B和cos(A-B)= cos A cos B + sin A sin B,则求出sin15°和cos15°的值。
解决方案:
To find sin15°:
We have sin15° = sin(45° – 30°)
A = 45°and B = 30°
Now putting the values of A and B in the following equation
sin(A − B) = sin A cos B − cos A sin B
sin(45° − 30°) = sin 45° cos 30° − cos 45° sin 30°
sin15° = (1/√2)(√3/2) – (1/√2)(1/2))
sin15° = (√3 – 1)/2√2
To find cos15°:
cos15° = cos(45° – 30°)
A = 45° and B = 30°
Again putting the values of A and B in the following equation
cos(A − B) = cos A cos B + sin A sin B
cos(45° − 30°) = cos 45° cos 30° + sin 45° sin 30°
sin15° = (1/√2)(√3/2) + (1/√2)(1/2))
sin15° = (√3 + 1)/2√2
问题36.如果∠B= 60°且AB = 15单位,则在右△ABC中,与C成直角。找到剩余的角度和边。
解决方案:
Given In a right △ABC right-angled at C, ∠B = 60° ∠C = 90°
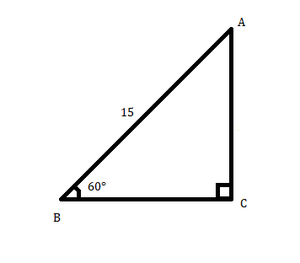
We know in a triangle ∠A + ∠B + ∠C = 180°
∠A = 180° – 150° = 30°
sin 30° = BC/AB = BC/15
BC/15 = 1/2
BC = 7.5 units
cos 30° = AC/AB = AC/15
AC/15 = √3/2
AC = 15√3/2 units
问题37.如果ΔABC是直角三角形,使得∠C= 90°,∠A= 45°,并且BC = 7个单位。查找∠B,AB和AC。
解决方案:
Given: In ΔABC ∠C = 90°, ∠A = 45°, and BC = 7 units
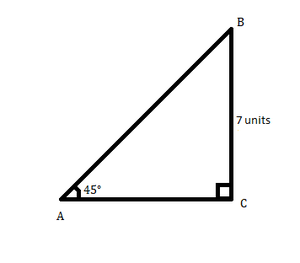
We know in a triangle ∠A + ∠B + ∠C = 180°
∠B = 180° – 135° = 45°
cos B = BC/AB = 7/AB
cos 45° = 7/AB
7/AB = 1/√2
AB = 7/√2 units
tan A = BC/AC
tan 45° = 7/AC
7/AC = 1
AC = 7 units
问题38.在矩形ABCD中,AB = 20 cm ,, BAC = 60°,计算边BC和对角线AC,BD。
解决方案:
Given: rectangle ABCD, AB = 20 cm, ∠BAC = 60°
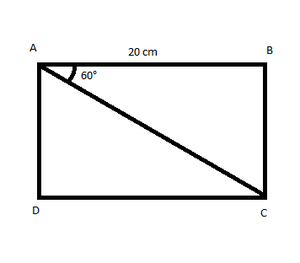
tan 60° = BC/AC
√3 = BC/20
BC = 20√3 cm
cos 60° = AB/AC
1/2 = 20/AC
AC = 40 cm
In rectangle both diagonals are equal
问题39.如果A和B是锐角,使得tan A = 1/2,tan B = 1/3,并且tan(A + B)= 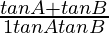 找到(A + B)。
找到(A + B)。
解决方案:
Given, A and B are acute angles such that tanA = 1/2 and tanB = 1/3
tan(A + B) = 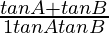
tan(A + B) = 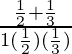
tan(A + B) = 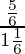
tan(A + B) = 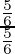
tan(A + B) = 1
We know, tan45° = 1
Therefore, (A + B) = 45°
问题40.证明(√3+1)(3 – cot 30°)= tan 3 60°– 2sin 60°
解决方案:
Taking R.H.S
tan3 60° – 2sin 60°
(√3) – 2(√3/2) = 3√3 – √3 = 2√3
Now taking L.H.S
(√3 + 1)(3 – cot 30°)
= (√3 + 1)(3 – √3)
= (√3 + 1)√3(√3 – 1)
= 2√3
L.H.S = R.H.S
Hence Proved
Given sin(A + B) = 1, cos(A − B) = 1, 0° < A + B ≤ 90° and A ≥ B,
We know that, sin90° = 1 and cos0° = 1
sin(A + B) = 1 and sin90∘= 1
We can say that
(A + B) = 90° -(1)
cos(A − B) = 1 and cos0° = 1
(A − B) = 0°
A = B -(2)
Substituting eq(2) in (1)
A + A = 90°
2A = 90°
A = 45°
A = B = 45°
Given tan(A + B) = √3
tan(A − B) = 1/√3
0∘< A + B ≤ 90∘and A > B,
We know that, tan30° = 1/√3 and tan60° = √3
Now,
tan(A + B) = √3 and tan60 = √3
(A + B) = 60° -(1)
Again,
tan(A − B) = 1/√3 and tan30° = 1/√3
(A − B) = 30° -(2)
Solving eq(1) and (2),
(A + B) = 60°
(A − B) = 30°
Solving the above two equations we get
2A = 90°
A = 90°/2 = 45°
解决方案:
Given, sin(A − B) = 1/2
cos(A + B) = 1/2
0° < A + B ≤ 90° and A < B,
We know that, sin30° = 1/2and cos60° = 1/2
Since sin(A − B) = 1/2 and sin30° = 1/2
So (A − B) = 30° -(1)
Again
cos(A + B) = 1/2 and cos60° = 1/2
So (A + B) = 60° -(2)
Solving eq(1) and (2) we get
2A = 90°
A = 90°/2 = 45°
From (2)
(A + B) = 60°
Also, A = 45°
B = 60° − 45°
B = 15°
Therefore, A = 45°, B = 15°
问题32.在与B成直角的∆ABC中,∠A=∠C。查找的值
(i)罪A cos C +罪A A sin C
解决方案:
Given: In ∆ABC right-angled at B, ∠A = ∠C

We know in a triangle ∠A + ∠B +∠C = 180°
2∠A = 180° – 90° = 45° [∠B = 90°]
Now,
We have sin A cos C + cos A sin C
Putting the values of each angle
sin 45° cos 45° + cos 45° sin 45°
= (1/√2)(1/√2) + (1/√2)(1/√2)
= 1/2 + 1/2
= 1
(ii)罪A罪B + cos A cos B
解决方案:
We have sin A sin B + cos A cos B
Putting the values of each angle
sin 45° sin 90° + cos 45° cos 90°
= (1/√2)(1) + (1/√2)(0)
= (1/√2) + 0
= 1/√2
问题33.如果sin(A + 2B)=√3/ 2且cos(A + 4B)= 0,并且A> B,则求出锐角A和B。
解决方案:
Given, sin(A + 2B) = √3/2, cos(A + 4B) = 0, A > B,
We know that, sin60° = √3/2 and cos90° = 0
Since sin(A + 2B) = √3/2 and sin60° = √3/2
So (A + 2B) = 60° -(1)
Again cos(A + 4B) = 0 and cos90° = 0
So (A + 4B) = 90° -(2)
From (1) from (2) we get,
2B = 30°
B = 30°/2 = 15°
From (2)
(A + 4B) = 90°
Also, B = 15°
A = 90° − 60°
A = 30°
Therefore, A = 30°, B = 15°
问题34.在ΔPQR中,在Q处成直角,PQ = 3 cm,PR = 6 cm。确定∠P和∠R。
解决方案:
Given In ΔPQR, right-angled at Q, PQ = 3 cm and PR = 6 cm.

We know cos P = Base/Hypotenuse =3/6
cos 60° = 1/2
So ∠P = 60°
In ΔPQR
∠P + ∠Q + ∠R = 180°
∠R = 180° – 90° – 60°
∠R = 30°
Therefore, ∠P = 60°, ∠R = 30°
问题35.如果sin(A-B)= sin A cos B-cos A sin B和cos(A-B)= cos A cos B + sin A sin B,则求出sin15°和cos15°的值。
解决方案:
To find sin15°:
We have sin15° = sin(45° – 30°)
A = 45°and B = 30°
Now putting the values of A and B in the following equation
sin(A − B) = sin A cos B − cos A sin B
sin(45° − 30°) = sin 45° cos 30° − cos 45° sin 30°
sin15° = (1/√2)(√3/2) – (1/√2)(1/2))
sin15° = (√3 – 1)/2√2
To find cos15°:
cos15° = cos(45° – 30°)
A = 45° and B = 30°
Again putting the values of A and B in the following equation
cos(A − B) = cos A cos B + sin A sin B
cos(45° − 30°) = cos 45° cos 30° + sin 45° sin 30°
sin15° = (1/√2)(√3/2) + (1/√2)(1/2))
sin15° = (√3 + 1)/2√2
问题36.如果∠B= 60°且AB = 15单位,则在右△ABC中,与C成直角。找到剩余的角度和边。
解决方案:
Given In a right △ABC right-angled at C, ∠B = 60° ∠C = 90°

We know in a triangle ∠A + ∠B + ∠C = 180°
∠A = 180° – 150° = 30°
sin 30° = BC/AB = BC/15
BC/15 = 1/2
BC = 7.5 units
cos 30° = AC/AB = AC/15
AC/15 = √3/2
AC = 15√3/2 units
问题37.如果ΔABC是直角三角形,使得∠C= 90°,∠A= 45°,并且BC = 7个单位。查找∠B,AB和AC。
解决方案:
Given: In ΔABC ∠C = 90°, ∠A = 45°, and BC = 7 units

We know in a triangle ∠A + ∠B + ∠C = 180°
∠B = 180° – 135° = 45°
cos B = BC/AB = 7/AB
cos 45° = 7/AB
7/AB = 1/√2
AB = 7/√2 units
tan A = BC/AC
tan 45° = 7/AC
7/AC = 1
AC = 7 units
问题38.在矩形ABCD中,AB = 20 cm ,, BAC = 60°,计算边BC和对角线AC,BD。
解决方案:
Given: rectangle ABCD, AB = 20 cm, ∠BAC = 60°

tan 60° = BC/AC
√3 = BC/20
BC = 20√3 cm
cos 60° = AB/AC
1/2 = 20/AC
AC = 40 cm
In rectangle both diagonals are equal
问题39.如果A和B是锐角,使得tan A = 1/2,tan B = 1/3,并且tan(A + B)= 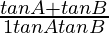 找到(A + B)。
找到(A + B)。
解决方案:
Given, A and B are acute angles such that tanA = 1/2 and tanB = 1/3
tan(A + B) = ![]()
tan(A + B) = 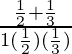
tan(A + B) = ![]()
tan(A + B) = ![]()
tan(A + B) = 1
We know, tan45° = 1
Therefore, (A + B) = 45°
问题40.证明(√3+1)(3 – cot 30°)= tan 3 60°– 2sin 60°
解决方案:
Taking R.H.S
tan3 60° – 2sin 60°
(√3) – 2(√3/2) = 3√3 – √3 = 2√3
Now taking L.H.S
(√3 + 1)(3 – cot 30°)
= (√3 + 1)(3 – √3)
= (√3 + 1)√3(√3 – 1)
= 2√3
L.H.S = R.H.S
Hence Proved