三角函数的极限| 11年级数学

我们知道有六个三角函数。考虑到函数的域和范围，可以根据函数的连续性来计算每个函数趋于某一点的极限。我们可以在每个函数的图中观察到，每个函数在同一点的限制是不同的。

正弦函数

函数f(x)= sin(x)是在其整个域上的连续函数，其域由所有实数组成。如下图所示，此函数的范围是[-1，1]：

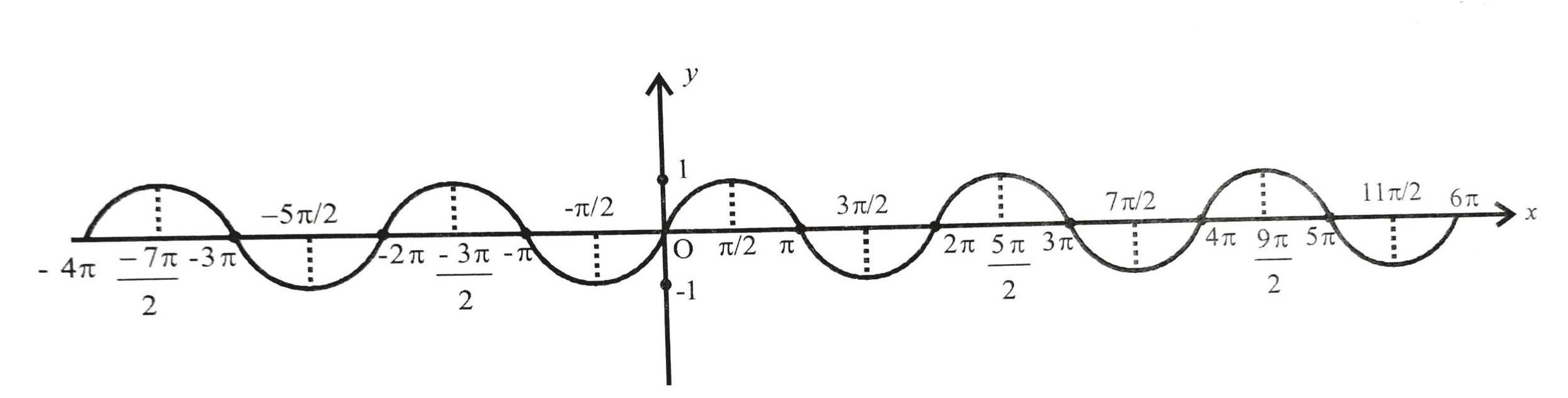
因此，如果正弦函数的极限是在任何给定的实数下计算的，则它总是被定义并且位于[-1，1]之间。

f(x) = sin(x)

lim_x→a f(x) = lim_x→a sin(x)

                 = sin(a), where a is a real number

例1：求出lim _{x→π/ 2} sin(x)

解决方案：

As we know sin(x) is defined at π/2

Therefore,   

lim_x→π/2 sin(x) = sin(π/2) = 1                                        

示例2：找到lim _x→πsin (x)

解决方案：

As we know sin(x) is defined at π

Therefore,   

lim_x→π sin(x) = sin(π) = 0                                             
 

余弦函数

函数f(x)= cos(x)是在其整个域上的连续函数，其域由所有实数组成。

如下图所示，此函数的范围是[-1，1]：

因此，如果余弦函数的极限是在任何给定的实数下计算的，则它总是被定义并且位于[-1，1]之间。

f(x) = cos(x)

lim_x→a f(x) = lim_x→a cos(x)

                   = cos(a), where a is a real number

例1：求出lim _{x→π/ 2} cos(x)

解决方案：

As we know cos(x) is continuous and defined at π/2

Therefore,   

lim_x→π/2 cos(x) = cos(π/2) = 0                                  

示例2：找到lim _x→π cos(x)

解决方案：

As we know cos(x) is continuous and defined at π

Therefore,  

lim_x→π cos(x) = cos(π) = -1 

切线函数

函数f(x)= tan(x)在所有实数上定义，除了cos(x)等于0的值，即所有整数n的值π/ 2 +πn。因此，它的域是除π/ 2 +πn，n€Z之外的所有实数。

该函数的范围是(-∞，+ c)。

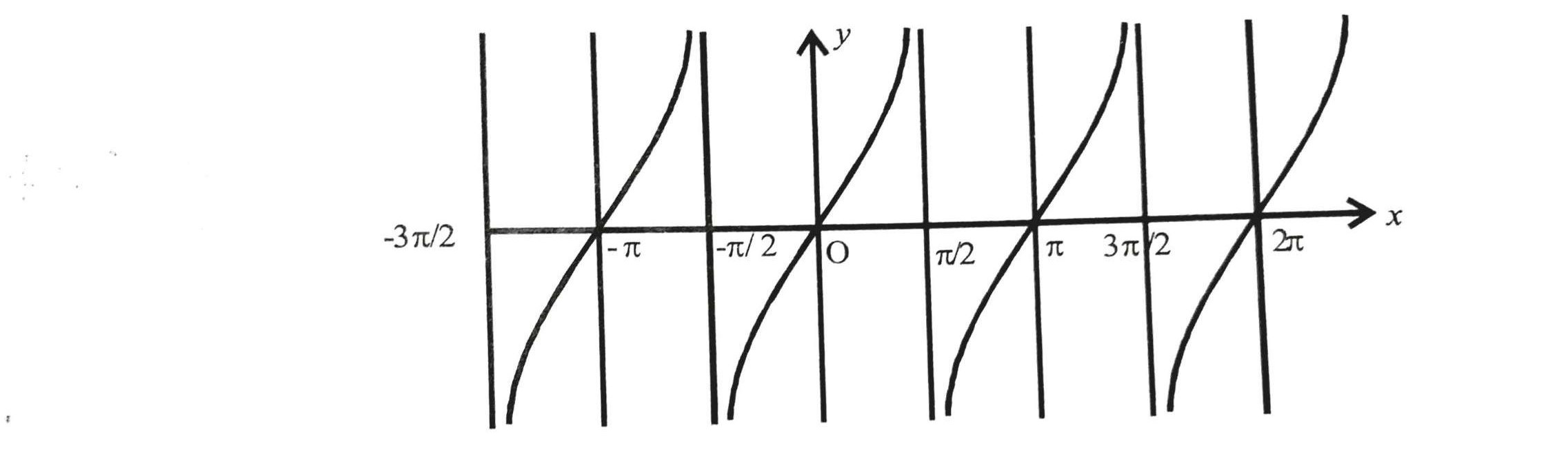
因此，如果切线函数的极限是在其范围内计算的，则它总是被定义并且位于(-∞，+∞)之间。

f(x) = tan(x)

lim_x→a f(x) = lim_x→a  tan(x)

                 = tan(a), where a belongs to real no. except π/2 + πn, n € Z

例1：求出lim _{x→π/ 2} tan(x)

解决方案：

As we can see in graph tan(x) is tending to +∞ as x→-π/2 and to -∞ as x→+π/2

So, the right hand and left-hand limits of this function at π/2 are not same

Therefore,

lim_x→π/2 tan(x) = Not defined

示例2：找到lim _x→ πtan(x)

解决方案：

As we know tan(x) is continuous and defined at π

Therefore,  

lim_x→π tan(x) = tan(π) = 0   

Cosec函数

函数f(x)= cosec(x)是在所有实数上定义的，但sin(x)等于0的值即所有整数n的值πn除外。因此，它的域是除πn，n€Z之外的所有实数。

此函数的范围是(-∞，-1] U [1，+∞)。

因此，如果余弦函数的极限是在其范围内计算的，则它总是被定义并且位于其范围之间。

f(x) = cosec(x)

lim_x→a f(x) = lim_x→a cosec(x)

                  = cosec(a), where a belongs to real no. except for πn , n € Z

示例1：找到lim _{x→ π} cosec(x)

解决方案：

As we know sin(x) is 0 as x→π           

So, 1/sin(x) i.e. cosec(x) at π cannot be defined

Therefore,

lim_x→π cosec(x) = Not defined

示例2：找到lim _{x→ π / 2} cosec(x)

解决方案：

As we know tan(x) is continuous and defined at π/2

Therefore,   

lim_x→π/2 cosec(x) = cosec(π/2) = 1  

正割函数

函数f(x)= sec(x)在所有实数上定义，除了cos(x)等于0的值，即所有整数n的值π/ 2 +πn。因此，它的域是除π/ 2 +πn，n€Z之外的所有实数。

该函数的范围是(-∞，-1] U [1，+∞)

因此，如果秒函数的极限是在其域中计算的，则它总是被定义并且位于其范围之间。

f(x) = sec(x)

lim_x→a f(x) = lim_x→a sec(x)

                  = sec(a), where a belongs to real no. except for π/2 + πn, n € Z

示例1：找到lim _{x→ π / 2} sec(x)

解决方案：

As we know cos(x) is 0 as x→π/2

So, 1/cos(x) i.e. sec(x) at π/2 cannot be defined

Therefore,

lim_x→π/2 sec(x) = Not defined

示例2：找到lim _{x→ πsec} (x)

解决方案：

As we know tan(x) is continuous and defined at π

Therefore,

lim_x→π cosec(x) = sec(π) = -1  

婴儿床函数

函数f(x)= cot(x)是在所有实数上定义的，但tan(x)等于0的值(即，所有整数n的值πn)除外。因此，它的域是除πn，n€Z之外的所有实数。

该函数的范围是(-∞，+∞)

因此，如果cot函数的极限是在其范围内计算的，则它总是被定义并且位于其范围之间。

f(x) = cot(x)

lim_x→a f(x) = lim_x→a cot(x)

                 = cot(a), where a belongs to real no. except for πn, n € Z

示例1：找到lim _x→ πcot(x)

解决方案：

As we know tan(x) is 0   as x→π

So, 1/tan(x) i.e. cot(x) at π cannot be defined

Therefore,

limx→π cot(x) = Not defined

示例2：找到lim _{x→π/ 2} cot(x)

解决方案：

As we know cot(x) is continuous and defined at π/2

Therefore,   

lim_x→π/2 cot(x) = cot(π/2) = 0  

夹心定理(挤压定理)

该定理用于计算那些不能轻易计算极限的函数的极限(例如x = 0时的sin x / x)。

函数g(x)被压缩或夹在两个函数h(x)和g(x)之间，使得f (x)≤g(x)≤h(x ) 。

在这种情况下的图形如下所示：

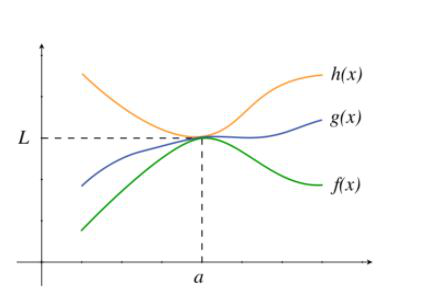
我们可以说h(x)是g(x)的上限，f(x)是在点a处的下限，如上图所示：

if,

lim_x→a h(x) = L and lim_x→a f(x) = L,

where a is the point at which limit is being calculated and L is the value of the limit

Then,

lim_x→a g(x) = L

范例1：假设：g(x)= x2sin(1 / x)，求出：lim _x→0 g(x)

解决方案：

We know,

-1≤ sin(1/x) ≤ 1 under its domain       

Multiplying with x2,

-x2 ≤ x2sin(1/x) ≤ x2

Then let f(x) = -x2 and h(x) = x2

f(x) ≤ g(x) ≤ h(x)  

Using sandwich theorem,

As lim_x→a h(x) = lim_x→a f(x) = L

Therefore,

lim_x→a g(x) = L

lim_x→0 f(x) = lim_x→0 -x2 = 0 and  

lim_x→0 h(x) = lim_x→0 x2 = 0

Therefore, 

lim_x→0 g(x) = 0

例2：给定：g(x)= cos(x)/ x，求出：lim _x→∞g (x)

解决方案：

We know,

-1≤ cos(x)≤ 1 under its domain    

Dividing by x,

-1/x ≤ cos(x)/x ≤ 1/x

Then let f(x) = -1/x and h(x) = 1/x          

f(x) ≤ g(x) ≤ h(x)  

               

Using sandwich theorem,

As lim_x→a h(x) = lim_x→a f(x) = L

Therefore, 

lim_x→a g(x) = L

lim_x→∞ f(x) = lim_x→∞ -1/x = 0 and  

lim_x→∞ h(x) = lim_x→∞ 1/x = 0

Therefore, 

lim_x→∞ g(x) = 0