第11类RD Sharma解决方案–第23章直线-练习23.18

问题1：找出通过原点并与直线√3x+ y = 11形成45°角的直线的方程。

解决方案：

As line 1 passes through origin, there won’t be any intercept, and it will be in the form of y = mx (m as slope)

For line 2: √3x+y = 11, slope is M = -√3

Here, it is given that these two lines make an angle of 45° between them. Hence,

As we know that, two lines having slopes as m and M will have angle θ between them as follows:

$\mathbf{tan θ = |\frac{M-m}{1+mM}|}$

Here, we have θ = 45°, m=m and M = -√3

tan 45° = $|\frac{-\sqrt{3}-m}{1+m(-\sqrt{3})}|$

1 = $|\frac{-\sqrt{3}-m}{1-\sqrt{3}m}|$

We will have two cases,

1 = $\frac{-\sqrt{3}-m}{1-\sqrt{3}m}$ and 1 = $-\frac{-\sqrt{3}-m}{1-\sqrt{3}m}$

1-√3m = -√3-m and 1-√3m = √3+m

√3m-m = 1+√3 and √3m+m = 1-√3

m = $\frac{1+\sqrt{3}}{\sqrt{3}-1}$ and m = $\frac{1-\sqrt{3}}{\sqrt{3}+1}$

By rationalizing, we get

m = $\frac{(1+\sqrt{3})^2}{3-1}$ and m = $-\frac{(1-\sqrt{3})^2}{3-1}$

m = $\frac{1+3+2\sqrt{3}}{2}$ and m = $-\frac{1+3-2\sqrt{3}}{2}$

m = 2+√3 and m = -(2-√3)

m = √3+2 and m = √3-2

Hence, the equation of line will be,

y = (√3+2)x and y = (√3-2)x

问题2：找到通过原点并与直线x + y +√3(yx)= a呈75°角倾斜的直线的方程。

解决方案：

As line 1 passes through origin, there won’t be any intercept and it will be in the form of y = mx (m as slope)

For line 2: x+y+√3(y-x)=a, 

x+y+√3y-√3x=a

x(1-√3)+y(1+√3)=a

slope is M = $\frac{\sqrt{3}-1}{\sqrt{3}+1}$

After rationalizing, we get

M = $\frac{(\sqrt{3}-1)^2}{3-1} = \frac{(3+1-2\sqrt{3})^2}{2}$ = 2-√3

Here, it is given that these two lines make an angle of 75° between them. Hence,

As we know that, two lines having slopes as m and M will have angle θ between them as follows:

$\mathbf{tan θ = |\frac{M-m}{1+mM}|}$

Here, we have θ = 75°, m=m and M = 2-√3

tan 75° = $|\frac{(2-√3)-m}{1+m(2-√3)}|$

tan (45°+30°)= $|\frac{(2-√3)-m}{1+m(2-√3)}|$

Using the trigonometric identity, 

$tan (a+b) = \frac{tan \hspace{0.1cm}a + tan\hspace{0.1cm} b}{1-(tan\hspace{0.1cm} a) (tan\hspace{0.1cm} b)}$

$\frac{tan 45\degree + tan 30\degree}{1-(tan 45\degree) (tan 30\degree)} = |\frac{(2-\sqrt{3})-m}{1+m(2-\sqrt{3})}|$

$\frac{1 + \frac{1}{\sqrt{3}}}{1-(1) (\frac{1}{\sqrt{3}})} = |\frac{(2-√3)-m}{1+m(2-√3)}|\\ \frac{\frac{√3+1}{\sqrt{3}}}{\frac{√3-1}{\sqrt{3}}} = |\frac{(2-√3)-m}{1+m(2-√3)}|\\ \frac{√3+1}{√3-1} = |\frac{(2-√3)-m}{1+m(2-√3)}|\\ \frac{(√3+1)^2}{3-1} = |\frac{(2-√3)-m}{1+m(2-√3)}|\\ \frac{(3+1+2√3)^2}{2} = |\frac{(2-√3)-m}{1+m(2-√3)}|\\ 2+√3 = |\frac{(2-√3)-m}{1+m(2-√3)}|$

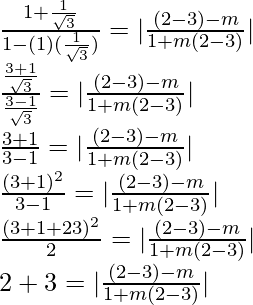
We will have two cases,

2+√3 = $\frac{(2-\sqrt{3})-m}{1+m(2-\sqrt{3})}$ and 2+√3 = $-\frac{(2-\sqrt{3})-m}{1+m(2-\sqrt{3})}$

(2+√3)(1+m(2-√3)) = 2-√3-m and (2+√3)(1+m(2-√3)) = -(2-√3-m)

(2+√3+m(2²-(√3)²) = 2-√3-m and (2+√3+m(2²-(√3)²) = √3-2+m

2+√3+m(4-3) = 2-√3-m and 2+√3+m(4-3) = √3-2+m

2+√3+m+m = 2-√3 and 2+√3+m-m = √3-2

2m = -2-√3+2-√3 and 2+√3 = √3-2

2m = -2√3 and m is not defined

m = -√3 and m is not defined

Hence, the equation of line will be,

y = -√3x and x = 0

问题3：找到通过(2，-1)并与6x + 5y-8 = 0线成45°角的直线的方程。

解决方案：

As line 1 passes through (2,-1), then it will be in the form of 

y-(-1) = m(x-2)  (m as slope)

y+1 = m(x-2)

For line 2: 6x+5y-8=0

5y = -6x + 8

$y = \frac{-6}{5}x + \frac{8}{5}$

slope is M = $\frac{-6}{5}$

Here, it is given that these two lines make an angle of 45° between them. Hence,

As we know that, two lines having slopes as m and M will have angle θ between them as follows:

$\mathbf{tan θ = |\frac{M-m}{1+mM}|}$

Here, we have θ = 45°, m=m and M = $\frac{-6}{5}$

tan 45° = $|\frac{\frac{-6}{5}-m}{1+m(\frac{-6}{5})}|$

$1 = |\frac{\frac{-6-5m}{5}}{1-m(\frac{6}{5})}|\\ 1 = |\frac{\frac{-6-5m}{5}}{\frac{5-6m}{5}}|\\ 1 = |\frac{-6-5m}{5-6m}|$

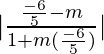
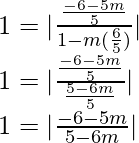
We will have two cases,

1 = $\frac{-6-5m}{5-6m}$ and 1 = $-\frac{-6-5m}{5-6m}$

5-6m = -6-5m and 5-6m = -(-6-5m)

6m-5m = 5+6 and 5-6m = 5m+6

m = 11 and 5m+6m = 5-6

m = 11 and m = $\frac{-1}{11}$

Hence, the equation of line will be,

y+1 = 11(x-2) and y+1 = $\frac{-1}{11}(x-2)$

11x-y-23=0 and 11y+x+9 = 0

问题4：找到通过点(h，k)并与直线y = mx + c^{成角度tan -1 m的直线的方程。}

解决方案：

As line 1 passes through (h,k), then it will be in the form of

y-(k) = M(x-h)  (M as slope)

For line 2: y=mx+c (m as slope)

Here, it is given that these two lines make an angle of tan^-1 m between them. Hence,

As we know that, two lines having slopes as m and M will have angle θ between them as follows:

$\mathbf{tan θ = |\frac{M-m}{1+mM}|}$

Here, we have θ = tan^-1 m,

tan (tan^-1 m) = $|\frac{M-m}{1+mM}|$

$m = |\frac{M-m}{1+mM}|$

We will have two cases,

m = $\frac{M-m}{1+mM}$ and m = $-\frac{M-m}{1+mM}$

m(1+mM) = M-m and m(1+mM) = -(M-m)

m+m²M = M-m and m+m²M = m-M

2m = M-m²M and m²M = -M

M = $\frac{2m}{1-m^2}$ and M = 0

Hence, the equation of line will be,

y-(k) = 0(x-h) and y-k = $\frac{2m}{1-m^2}(x-h)$

y-k = 0 and (y-k)(1-m²) = (2m)(x-h)

问题5：找到通过点(2,3)并与线3x + y-5 = 0成45°倾斜的直线的方程。

解决方案：

As line 1 passes through (2,3), then it will be in the form of

y-3 = M(x-2)  (M as slope)

For line 2: 3x+y-5=0

y = -3x+5

slope m = -3

Here, it is given that these two lines make an angle of 45° between them. Hence,

As we know that, two lines having slopes as m and M will have angle θ between them as follows:

$\mathbf{tan θ = |\frac{M-m}{1+mM}|}$

Here, we have θ = 45°,

tan 45° = $|\frac{M-(-3)}{1+(-3)M}|$

$1 = |\frac{M+3}{1-3M}|$

We will have two cases,

$1 = \frac{M+3}{1-3M}$ and $1 = -\frac{M+3}{1-3M}$

1-3M = M+3 and 1-3M = -(M+3)

M+3M = 1-3 and 1-3M = -M-3

4M = -2 and 3M-M = 1+3

M = $\frac{-2}{4}$ and 2M = 4

M = $\frac{-1}{2}$ and M = 2

Hence, the equation of line will be,

$y-3 = \frac{-1}{2}(x-2)$ and y-3 = 2(x-2)

2y-6 = -(x-2) and y-3 = 2x-4

x+2y-8=0 and 2x-y-1=0

问题6：在等腰直角三角形的边找到方程，该方程的斜边为3x + 4y = 4，相反的顶点为点(2,2)。

解决方案：

As △ABC is an isosceles right angled triangle at B.

∠A = ∠C = 45°

We can say that, AB and BC makes 45° with AC.

Let the slope of AB as m₁ and BC as m₂.

AB: (y-2) = m₁(x-2)

BC: (y-2) = m₂(x-2)

Slope of AC : 3x+4y=4

$y = \frac{-3}{4}x + 1$

Slope of AC is $\frac{-3}{4}.$

Here, it is given that these two lines make an angle of 45° between them. Hence,

As we know that, two lines having slopes as m and M will have angle θ between them as follows:

$\mathbf{tan θ = |\frac{M-m}{1+mM}|}$

Here, we have θ = 45°, and M = $\frac{-3}{4}$

tan 45° = $|\frac{\frac{-3}{4}-m}{1+m(\frac{-3}{4})}|$

$1 = |\frac{\frac{-3-4m}{4}}{1-m(\frac{3}{4})}|\\ 1 = |\frac{\frac{-3-4m}{4}}{\frac{4-3m}{4}}|\\ 1 = |\frac{-3-4m}{4-3m}|$

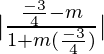
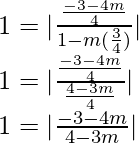
We will have two cases,

1 = $\frac{-3-4m}{4-3m}$ and 1 = $-\frac{-3-4m}{4-3m}$

4-3 = -3-4 and 4-3 = -(-3-4)

4m-3 = -3-4 and 4-3 = 3+4

m = -7 and 4+3 = 4-3

m = -7 and m = $\frac{1}{7}$

Hence, the equation of lines will be,

AB: (y-2) = m₁(x-2)

$y-2 = \frac{1}{7}(x-2)$

7y-x-12=0

BC: (y-2) = m₂(x-2)

y-2 = -7(x-2)

7x+y-16=0

问题7：等边三角形的一侧的方程为xy = 0，一个顶点为(2 +√3,5)。证明第二边是y +(2-√3)x = 6并找到第三边的方程。

解决方案：

As equation of one side of equilateral triangle is x-y=0 and one vertex is (2+√3,5),

As, the point (2+√3,5) does not satisfy x-y=0. Then this is the vertex opposite of the line x-y=0

In equilateral triangle,

∠A = ∠B = ∠C = 60°

We can say that, AC and BC makes 60° with AB.

Let the slope of AC as m₁ and BC as m₂.

AB: (y-5) = m₁(x-(2+√3))

BC: (y-5) = m₂(x-(2+√3))

Slope of AC : x-y=0

y = x

Slope of AC is 1.

Here, it is given that these two lines make an angle of 60° between them. Hence,

As we know that, two lines having slopes as m and M will have angle θ between them as follows:

$\mathbf{tan θ = |\frac{M-m}{1+mM}|}$

Here, we have θ = 60°, m = m₁ and m₂ and M = 1

tan 60° = $|\frac{1-m}{1+m(1)}|$

√3= $|\frac{1-m}{1+m}|$

We will have two cases,

√3 = $\frac{1-m}{1+m}$ and √3 = $-\frac{1-m}{1+m}$

m = $\frac{1-\sqrt{3}}{1+\sqrt{3}}$ and √3(1+m) = -(1-m)

m = $\frac{1-\sqrt{3}}{1+\sqrt{3}}$ and √3+√3m = m-1

m = $\frac{1-\sqrt{3}}{1+\sqrt{3}}$ and √3m-m = -1-√3

m = $\frac{1-\sqrt{3}}{1+\sqrt{3}}$ and m = $\frac{1+\sqrt{3}}{1-\sqrt{3}}$

After rationalizing, we get

m = $\frac{(1-\sqrt{3})^2}{1-3}$ and m = $\frac{(1+\sqrt{3})^2}{1-3}$

m = $\frac{1+3-2\sqrt{3}}{-2}$ and m = $\frac{1+3+2\sqrt{3}}{-2}$

m = -(2-√3) and m = -(2+√3)

Hence, the equation of lines will be,

AB: (y-5) = m₁(x-(2+√3))

y-5 = -(2-√3)(x-(2+√3))

y-5 = -(2-√3)x+ (2²-(√3)²)

(2-√3)x+y-6 = 0

BC: (y-5) = m₂(x-(2+√3))

(y-5) = -(2+√3)(x-(2+√3))

y-5 = -(2+√3)x+(2+√3)^2

(2+√3)x+y-5 = 4+3+4√3

(2+√3)x+y = 12+4√3

问题8：求出两条直线的等式，它们通过(1,2)形成一个正方形的两个边，其中4x + 7y = 12是一个对角线。

解决方案：

Let the point opposite of diagonal 4x+7y=12 be C(1,2)

Here, △BCD form an isosceles right angled triangle at C.

∠B = ∠D = 45°

We can say that, CD and BC makes 45° with BD.

Let the slope of CD as m₁ and BC as m₂.

CD: (y-2) = m₁(x-1)

BC: (y-2) = m₂(x-1)

Slope of BD : 4x+7y=12

$y = \frac{-4}{7}x + 3$

Slope of BD is $\frac{-4}{7}.$

Here, it is given that these two lines make an angle of 45° between them. Hence,

As we know that, two lines having slopes as m and M will have angle θ between them as follows:

$\mathbf{tan θ = |\frac{M-m}{1+mM}|}$

Here, we have θ = 45° and M = $\frac{-4}{7}$

tan 45° = $|\frac{\frac{-4}{7}-m}{1+m(\frac{-4}{7})}|$

$1 = |\frac{\frac{-4-7m}{7}}{1-m(\frac{4}{7})}|\\ 1 = |\frac{\frac{-4-7m}{7}}{\frac{7-4m}{7}}|\\ 1 = |\frac{-4-7m}{7-4m}|$

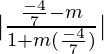
We will have two cases,

1 = $\frac{-4-7m}{7-4m}$ and 1 = $-\frac{-4-7m}{7-4m}$

7-4m = -4-7m and 7-4m = -(-4-7m)

7m-4m = -4-7 and 7-4m = 4+7m

3m = -11 and 7m+4m = 7-4

m = $\frac{-11}{3}$ and 11m = 3

m = $\frac{-11}{3}$ and m = $\frac{3}{11}$

Hence, the equation of lines will be,

CD: (y-2) = m₁(x-1)

$y-2 = \frac{-11}{3}(x-1)$

3y-6 = -11x+11

11x+3y-17=0

BC: (y-2) = m₂(x-1)

$y-2 = \frac{3}{11}(x-1)$

11y-22 = 3x-3

3x-11y+19=0

问题9：找到两条直线，通过(1,2)，与x + y = 0线成60°角，得出方程。还要找到由三条线形成的三角形的面积。

解决方案：

As equation of one side of equilateral triangle is x+y=0 and one vertex is (1,2),

As, the point (1,2) does not satisfy x+y=0. Then this is the vertex opposite of the line x+y=0

Hence, the lines make an equilateral triangle,

∠A = ∠B = ∠C = 60°

We can say that, AC and BC makes 60° with AB.

Let the slope of AC as m₁ and BC as m₂.

AB: (y-2) = m₁(x-1)

BC: (y-2) = m₂(x-1)

Slope of AC : x+y=0

y = -x

Slope of AC is -1.

Here, it is given that these two lines make an angle of 60° between them. Hence,

As we know that, two lines having slopes as m and M will have angle θ between them as follows:

$\mathbf{tan θ = |\frac{M-m}{1+mM}|}$

Here, we have θ = 60° and M = -1

tan 60° = $|\frac{-1-m}{1+m(-1)}|$

√3= $|\frac{-1-m}{1-m}|$

We will have two cases,

√3 = $\frac{-1-m}{1-m}$ and √3 = $-\frac{-1-m}{1-m}$

√3(1-m) = -1-m and √3(1-m) = 1+m

√3-√3m = -1-m and √3-√3m = m+1

√3m-m = √3+1 and +√3m+m = √3-1

m = $\frac{\sqrt{3}+1}{\sqrt{3}-1}$ and m = $\frac{\sqrt{3}-1}{\sqrt{3}+1}$

After rationalizing, we get

m = $\frac{(\sqrt{3}+1)^2}{3-1}$ and m = $\frac{(\sqrt{3}-1)^2}{3-1}$

m = $\frac{1+3+2\sqrt{3}}{2}$ and m = $\frac{1+3-2\sqrt{3}}{2}$

m = 2+√3 and m = 2-√3

Hence, the equation of lines will be,

AB: (y-2) = (2+√3)(x-1)

y-2=(2+√3)x-2-√3

(2+√3)x-y-√3=0 ……………….(i)

BC: (y-2) = (2-√3)(x-1)

y-2=(2-√3)x-2+√3

(2-√3)x-y+√3=0 ……………….(ii)

Using (i) and x+y=0, we get

$A = (\frac{-1-\sqrt{3}}{2},\frac{1+\sqrt{3}}{2})$

C = (1,2)

Using distance formula, AC

$AC = \sqrt{(1-\frac{-1-\sqrt{3}}{2})^2+(2-\frac{1+\sqrt{3}}{2})^2}\\ AC = \sqrt{(\frac{2+1+\sqrt{3}}{2})^2+(\frac{4-1-\sqrt{3}}{2})^2}\\ AC = \sqrt{(\frac{3+\sqrt{3}}{2})^2+(\frac{3-\sqrt{3}}{2})^2}\\ AC = \sqrt{\frac{9+3+6\sqrt{3}+9+3-6\sqrt{3}}{4}}\\ AC = \sqrt{\frac{24}{4}}\\ AC = \sqrt{6}$

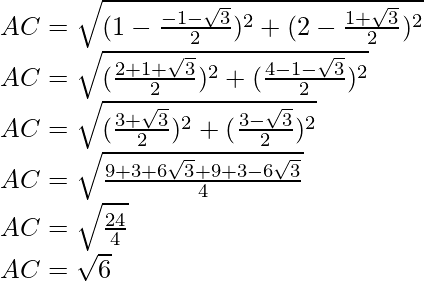
Area of equilateral triangle ABC,

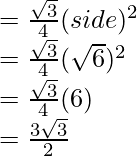$= \frac{\sqrt{3}}{4}(side)^2\\ = \frac{\sqrt{3}}{4}(\sqrt{6})^2\\ = \frac{\sqrt{3}}{4}(6)\\ = \frac{3\sqrt{3}}{2}$ sq. unit

问题10：等腰三角形的两侧由等式7x-y + 3 = 0和x + y-3 = 0给出，并且其第三侧通过点(1，-10)。确定第三边的方程式。

解决方案：

Let the equation of the line AB and AC 7x-y+3=0 and x+y-3=0 respectively.

∠B = ∠C

Slope of line AB: 7x-y+3=0

y = 7x+3

Slope m₁ = 7

Slope of line AC: x+y-3=0

y = -x+3

Slope m₂ = -1

Let the slope of line BC be m, which passes through the point (1,-10)

y-(-10) = m(x-1)

y+10 = m(x-1)

The angle between the lines AB and BC is equal to the angle between the lines AC and BC, say θ

As we know that, two lines having slopes as m and M will have angle θ between them as follows:

$\mathbf{tan θ = |\frac{M-m}{1+mM}|}$

By taking positive sign

tan θ = $\frac{m-7}{1+7m} = \frac{m-(-1)}{1+m(-1)}$

tan θ = $\frac{m-7}{1+7m} = \frac{m+1}{1-m}$

Solving it, we get

m = -3 or $\frac{1}{3}$

By taking negative sign

tan θ = $\frac{m-7}{1+7m} = -\frac{m-(-1)}{1+m(-1)}$

tan θ = $\frac{m-7}{1+7m} = -\frac{m+1}{1-m}$

Solving it, we get

m² = -1 (which is not possible)

Line BC: when m = -3

y+10 = -3(x-1)

y+10 = -3x+3

3x+y-13=0

Line BC: when m = $\frac{1}{3}$

$y+10 = \frac{1}{3}(x-1)$

3y+30 = x-1

x-3y-31=0

问题11：证明点(3，-5)位于平行线2x + 3y-7 = 0和2x + 3y + 12 = 0之间，并通过(3，-5)截去上述线来找到线的方程呈45°角。

解决方案：

Let the line 1: 2x+3y-7=0

Line 2: 2x+3y+12=0

As the slope of line 1 and line 2 is same, these lines are parallel.

Slope of lines M = $\frac{-2}{3}$

To check whether (3,-5) lies between these lines

Taking x=3 and y=-5

(2x+3y-7)(2x+3y+12)<0 (If its true then the point lies between these lines)

(2(3)+3(-5)-7)(2(3)+3(-5)+12)

(6-15-7)(6-15+12)

(-16)(3) <0, which is a negative value.

Hence, the point (3,-5) lies between the lines.

Let the slope of the line be m, which is passing through the point (3,-5)

y-(-5) = m(x-3)

y+5 = m(x-3)

As it is given that the line is cutting above lines at an angle of 45°.

As we know that, two lines having slopes as m and M will have angle θ between them as follows:

$\mathbf{tan θ = |\frac{M-m}{1+mM}|}$

Taking θ = 45° and M = $\frac{-2}{3}$

By taking positive sign

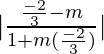
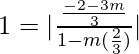
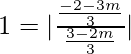
tan 45° = $|\frac{\frac{-2}{3}-m}{1+m(\frac{-2}{3})}|$

$1 = |\frac{\frac{-2-3m}{3}}{1-m(\frac{2}{3})}|$

$1 = |\frac{\frac{-2-3m}{3}}{\frac{3-2m}{3}}|$

$1 = |\frac{-2-3m}{3-2m}|$

We will have two cases,

1 = $\frac{-2-3m}{3-2m}$ and 1 = $-\frac{-2-3m}{3-2m}$

3-2m = -2-3m and 3-2m = -(-2-3m)

3m-2m = -2-3 and 3-2m = 2+3m

m = -5 and 3m+2m = 3-2

m = -5 and m = $\frac{1}{5}$

Hence, the equation of line will be,

when m = -5

y+5 = (-5)(x-3)

y+5 = -5x+15

5x+y-10=0

when m = $\frac{1}{5}$

y+5 = $\frac{1}{5}$(x-3)

5y+25 = x-3

x-5y-28=0

问题12：等边三角形的底边的等式为x + y = 2，其顶点为(2，-1)。求出边的长度和等式。

解决方案：

∠A = ∠B = ∠C = 60°

We can say that, AC and AB makes 60° with BC.

Let the slope of AC as m₁ and AB as m₂ which passes through the point (2,-1).

AB: (y-(-1)) = m₁(x-2)

y+1 = m₁(x-2)

AC: (y-(-1)) = m₂(x-2)

y+1 = m₂(x-2)

Slope of BC : x+y=2

y = -x+2

Slope of BC is -1.

Here, it is given that these two lines make an angle of 60° between them. Hence,

As we know that, two lines having slopes as m and M will have angle θ between them as follows:

$\mathbf{tan θ = |\frac{M-m}{1+mM}|}$

Here, we have θ = 60°, m = m₁ and m₂ and M = -1

tan 60° = $|\frac{-1-m}{1+m(-1)}|$

√3= $|\frac{-1-m}{1-m}|$

We will have two cases,

√3 = $\frac{-1-m}{1-m}$ and √3 = $-\frac{-1-m}{1-m}$

√3(1-m) = -1-m and √3(1-m) = 1+m

√3-√3m = -1-m and √3-√3m = m+1

√3m-m = √3+1 and +√3m+m = √3-1

m = $\frac{\sqrt{3}+1}{\sqrt{3}-1}$ and m = $\frac{\sqrt{3}-1}{\sqrt{3}+1}$

After rationalizing, we get

m = $\frac{(\sqrt{3}+1)^2}{3-1}$ and m = $\frac{(\sqrt{3}-1)^2}{3-1}$

m = $\frac{1+3+2\sqrt{3}}{2}$ and m = $\frac{1+3-2\sqrt{3}}{2}$

m = 2+√3 and m = 2-√3

Hence, the equation of lines will be,

AB: y+1 = m₁(x-2)

y+1 = (2+√3)(x-2)

y+1 = (2+√3)x-4-2√3

(2+√3)x-y-5-2√3=0 ……………….(i)

AC: y+1 = m₂(x-2)

y+1 = (2-√3)(x-2)

y+1 = (2-√3)x-4+2√3

(2-√3)x-y-5+2√3=0 ……………….(ii)

Using (ii) and x+y=2, we get

$C = (\frac{15+\sqrt{3}}{6},-\frac{3+\sqrt{3}}{6})$

A = (2,-1)

Using distance formula, AC

$AC = \sqrt{(2-\frac{15+\sqrt{3}}{6})^2+(-1-(-\frac{3+\sqrt{3}}{6}))^2}\\ AC = \sqrt{(\frac{12-15-\sqrt{3}}{6})^2+(\frac{-6+3+\sqrt{3}}{6})^2}\\ AC = \sqrt{(\frac{-3-\sqrt{3}}{6})^2+(\frac{-3+\sqrt{3}}{6})^2}\\ AC = \sqrt{\frac{9+3+6\sqrt{3}+9+3-6\sqrt{3}}{36}}\\ AC = \sqrt{\frac{24}{36}}\\ AC = \sqrt{\frac{2}{3}}$

AC = AB = BC = $\sqrt{\frac{2}{3}}$

问题13：如果一个正方形的两个相对顶点分别是(1,2)和(5,8)，则求出其另外两个顶点的坐标和其边的方程。

解决方案：

Let’s consider a square ABCD.

According to the property of square, the diagonal bisects the angle.

Hence, ∠AOB = ∠AOD = 45°

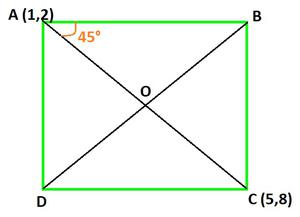
Slope of the diagonal AC:

$\frac{8-2}{5-1} = \frac{6}{4} = \frac{3}{2}$

Let the slope of the line AB and AD be m1 and m2, which passes through the point (1,2)

AB: y-2 = m₁(x-1)

AD: y-2 = m₂(x-1)

Here, it is given that these two lines make an angle of 45° with AC. Hence,

As we know that, two lines having slopes as m and M will have angle θ between them as follows:

$\mathbf{tan θ = |\frac{M-m}{1+mM}|}$

Here, we have θ = 45°, m = m₁ and m₂ and M = $\frac{3}{2}$

tan 45° = $|\frac{\frac{3}{2}-m}{1+m(\frac{3}{2})}|$

$1 = |\frac{\frac{3-2m}{2}}{\frac{2+3m}{2}}|$

$1= |\frac{3-2m}{2+3m}|$

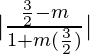
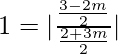
We will have two cases,

1 = $\frac{3-2m}{2+3m}$ and 1 = $-\frac{3-2m}{2+3m}$

2+3m = 3-2m and 2+3m = -(3-2m)

3m+2m = 3-2 and 2+3m = 2m-3

5m = 1 and 3m-2m = -3-2

m = $\frac{1}{5}$ and m = -5

m = $\frac{1}{5}$ or -5

Hence, the equation of lines will be,

AB: y-2 = m₁(x-1)

$y-2 = \frac{1}{5}(x-1)$

5y-10 = x-1

x-5y+9=0 ……………….(i)

AD: y-2 = m₂(x-1)

y-2 = -5(x-1)

y-2 = -5x+5

5x+y-7=0 ……………….(ii)

As BC is parallel to AD, So 

The equation BC will be 5x+y+λ=0 and as BC passes through C(5,8), we get

5(5)+(8)+λ=0

33+λ=0

λ = -33

Hence, Equation of BC is 5x+y-33=0 ……………….(iii)

Now as CD is parallel to AB, So

The equation CD will be x-5y+λ=0 and as CD passes through C(5,8), we get

5-5(8)+λ=0

-35+λ=0

λ = 35

Hence, Equation of CD is x-5y+35=0 ………………………(iv)

Solving (i) and (iii), we get

B(6,3)

Solving (ii) and (iv), we get

D(0,7)

Hence, the equation of lines are

AB = x-5y+9=0

BC = 5x+y-33=0

CD = x-5y+35=0

AD = 5x+y-7=0

And the vertices of square are

A(1,2), B(6,3), C(5,8) and D(6,3)