微积分的连续性和不连续性– CBSE 12级

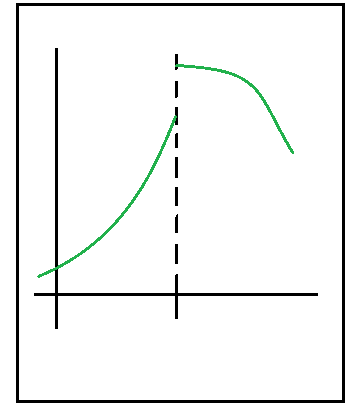
如果一个人可以在不举笔的情况下就可以在图形上绘制曲线，那么该函数就是连续的。当且仅当满足以下三个条件时，函数才能在x = a处连续。

1. The function is defined at x = a; that is, f(a) equals a real number
2. The limit of the function as x approaches a exists
3. The limit of the function as x approaches a is equal to the function value at x = a

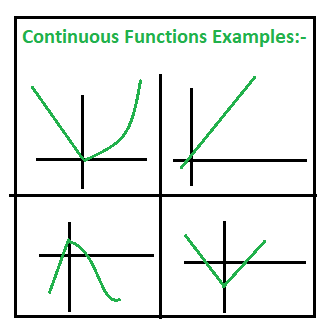
函数f(x)被说成是在开区间(A，B)如果连续在给定的任何点间隔的函数是连续的。在闭区间[a，b]的情况下，该函数被称为连续函数：

• f(x) is be continuous in the open interval (a, b)
• lim_x⇢a f(x) = f(a)
• lim_x⇢b f(x) = f(b)

示例1：证明函数f(x)= 5x – 3在x = 0处是连续的。

Solution:

Given, f(x) = 5x – 3

At x = 0 , f(0) = (5 × 0) – 3 = -3 
lim_x⇢0 f(x) = lim_x⇢0 (5x – 3) = (5 × 0) – 3 = -3 
lim_x⇢0 f(x) = f(0) 

Therefore, f(x) is continuous at x = 0.   

示例2：检查函数f(x)= | x – 5 |的连续性。

Solution:

Given function, f(x) = |x – 5| 

Domain of f(x) is real and infinite for all real x 
Here , f(x) = |x – 5| is a modulus function 
As , every modulus function is continuous 
Therefore , f(x) is continuous in its domain R.   

示例3：函数f(x)= x – sinx + 5在x =π处是连续的吗?

Solution:

Given function is f(x) = x – sinx + 5 

L.H.L = lim_x⇢π^– (x – sinx + 5) = lim_x⇢π^– [(π – h) – sin(π – h) + 5] = π + 5 

R.H.L = lim_x⇢π⁺ (x – sinx + 5) = lim_x⇢π⁺ [(π + h) – sin(π + h) + 5] = π + 5 

And, f(π) = π – sinπ + 5 = π  + 5 

Since , L.H.L = R.H.L = f(π) 
Therefore , f(x) is continuous at x = π 

示例4：检查函数f(x)= 2x – 1在x = 3的连续性。

Solution:

Given f(x) = 2x – 1 

At x = 3, f(x) = (2 × 3) – 1 = 5 
lim_x⇢3 f(x) = lim_x⇢3 f(x) = (2×3) – 1 = 5 
lim_x⇢3 f(x) = f(3) 
Therefore, f(x) is continuous at x = 3

例5：检查函数是否连续?

Solution:

For x > 0, y = x and x < 0, y = -x
 

So, We Know it is continuous for x > 0 and x < 0. To check if it is continuous at x = 0 , check the limit:
 

lim_x⇢0^–  |x| = lim_x⇢0^– (-x) = 0
 

lim_x⇢0⁺  |x| = lim_x⇢0⁺ (x) = 0
 

So, lim_x⇢0 |x| = 0 , which is equal to the value of the function at 0. Therefore, It is continuous everywhere. 

间断性

如果函数在a点不连续，则函数在点x = a处不连续。在以下任何情况下，函数“ f”在x = a处都是不连续的：

1. f(a) is not defined
2. lim_x⇢a⁺ f(x) and lim_x⇢a^–  f(x) exists, but are not equal.
3. lim_x⇢a⁺ f(x) and lim_x⇢a^– f(x) exists and are equal but not equal to f(a).

间断的类型

三种不连续的基本类型

1. 可移动(点)间断
2. 无限间断
3. 跳跃间断

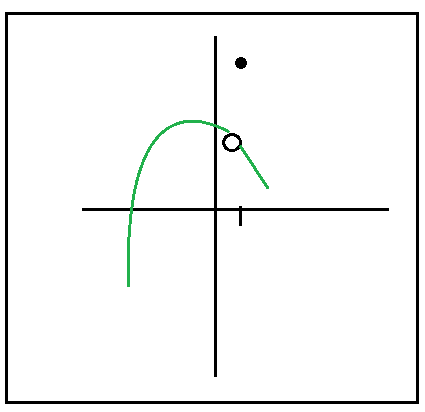
可移动(点)不连续性：该图形在单个x值处有一个孔。想象您正在走在路上，有人拆了一个人孔盖。这是不连续的类别，其中函数在x = a处具有明确定义的两侧极限，但未定义f(a)或f(a)不等于其极限。

• _limx⇢af (x)≠f(a)
• f(a)= _limx⇢af (x)

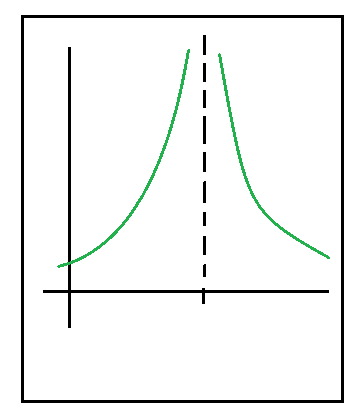
无限间断：该函数趋向于正或负无穷大。想象一下，一条道路越来越靠近一条河，而另一边没有桥。该函数在x = a处发散，从而使其具有不连续的性质。也就是说，没有定义f(a)。由于x = a处的函数值趋于无穷大或不接近特定的有限值，因此也未定义x→a的函数极限。

跳转不连续性：图形从一个地方跳到另一个地方。想象一个超级英雄去散步，他走到了尽头，因为他能飞到另一条路。在这种类型的不连续性中，存在在x = a处函数的右手极限和左手极限；但是两者并不相等。

• _{^LIMx⇢a+ F(X)≠LIMx⇢a ^–} F(x)的