9类RD Sharma解决方案–第四章代数身份-练习4.3 |套装2

问题11。如果3x-2y = 11且xy = 12，则找到27x ³ -8y ^3的值

解决方案：

Given, 3x-2y=11 and xy=12

we know that (a-b)³ = a³-b³-3ab(a-b)

(3x-2y)³ = 11³

27x³-8y³-3(12)(11)=1331

27x³-8y³=1331+2376

27x³-8y³=3707

Hence, the value of 27x³-8y³ = 3707

问题12.如果(x ⁴ + 1 / x ⁴ )= 119，则找到(x ³ -1 / x ³ )的值

解决方案：

Given, (x⁴+1/x⁴) =119 —— 1

we know that (x+y)² = x²+y²+2xy

substitute the given value in eq-1

(x²+1/x²)² = x⁴+1/x⁴+2(x²)(1/x²)

= x⁴+1/x⁴+2

= 119+2

= 121

(x²+1/x²)² = ±11

Now, find (x-1/x)

we know that (x-y)²=x²+y²-2xy

(x-1/x)² = x²+1/x²-2*x*1/x

= x²+1/x² -2

= 11 -2

= 9

(x-1/x) = ±3

we need to find x³-1/x³

we know that , a³-b³=(a-b)(a²+b²-ab)

x³-(1/x)³=(x-1/x)(x²+(1/x)²-x*1/x)

Here, x²+1/x² = 11 and x-1/x=3

x³-1/x³ = 3(11+1)

= 3(12)

= 36

Hence, the value of x³-1/x³=36

问题13：评估以下各项：

(a)(103) ³

(b)(98) ³

(c)(9.9) ³

(d)(10.4) ³

(e)(598) ³

(女)(99) ³

解决方案：

Given:

(a) (103)³

we know that (a+b)³ = a³+b³+3ab(a+b)

(103)³ can be written as (100+3)³

Here, a=100 and b=3

(103)³ = (100+3)³

=100³+3³+3(100)(3)(100+3)

=1000000+27+900(103)

=1092727

The value of (103)³=1092727

(b) (98)³

we know that (a-b)³ = a³-b³-3ab(a-b)

(98)³ = (100-2)³

= 100³-2³-3(100)(2)(100-2)

= 1000000-8-600(98)

= 941192

The value of (98)³ = 941192

(c) (9.9)³

we know that (a-b)³ = a³-b³-3ab(a-b)

(10-0.1)³ = (10)³-(0.1)³-3(10)(0.1)(10-0.1)

= 1000 – 0.001-3(9.9)

= 970.299

The value of (9.9)³=970.299

(d) (10.4)³

we know that (a+b)³= a³+b³+3ab(a+b)

(10+0.4)³=(10)³+(0.4)³+3(10)(0.4)(10+0.4)

= 1000+0.064+12(10.4)

= 1124.864

The value of (10.4)³=1124.864

(e) (598)³

we know that (a-b)³ = a³-b³-3ab(a-b)

(600-2)³ = (600)³-2³-3(600)(2)(600-2)

= 216000000 – 8 -(3600*598)

= 216000000 -8 – 2152800

= 213847192

The value of (598)³ = 213847192

(f) (99)³

we know that (a-b)³ = a³-b³-3ab(a-b)

(100-1)³ = (100)³ -1³ -3(100)(1)(100-1)

= 1000000 – 1 -300*99

= 1000000 – 1 -29700

= 970299

The value of (99)³ = 970299

问题14：评估以下各项

(a)111 ³ – 89 ³

(b)46 ³ +34 ³

(c)104 ³ +96 ³

(d)93 ³ – 107 ³

解决方案：

Given:

(a) 111³ – 89³

The above equation can be written as (100+11)³ – (100-11)³

we know that , (a+b)³ -(a-b)³= 2[b³+3a²b]

Here, a=100 b=11

(100+11)³ – (100-11)³ = 2[11³+3(100)²(11)]

= 2[1331 + 330000]

= 2[331331]

= 662662

The value of 111³-89³ = 662662

(b) 46³ + 34³

The above equation can be written as (40+6)³ – (40-6)³

we know that , (a+b)³ +(a-b)³= 2[a³+3ab²]

Here, a = 40 and b=6

(40+6)³ – (40-6)³ = 2[(40)³+3(6)²(40)]

= 2[64000+3*36*40]

=2[68320]

= 136640

The value of 46³ +34³=136640

(c) 104³ +96³

The above equation can be written as (100+4)³ + (100-4)³

we know that, (a+b)³ +(a-b)³= 2[a³+3ab²]

here, a = 100 , b= 4

(100+4)³ +(100-4)³ = 2[(100)³ +3(100)(4)²]

= 2[1000000 + 300*16]

= 2[1004800]

= 2009600

The value of 104³ + 96³ = 2009600

(d) 93³ – 107³

The above equation can be written as (100-7)³ – (100+7)³

we know that, (a-b)³ -(a+b)³= -2[b³+3a²b]

here, a = 100 , b= 7

(100-7)³ – (100+7)³ = -2[7³+3*(100)²*7]

= -2[210343]

= -420686

The value of 93³ – 107³ = -420686

问题15.如果x + 1 / x = 3，则计算x ² + 1 / x ² ，x ³ + 1 / x ³ ，x ⁴ + 1 / x ⁴

解决方案：

Given, x+1/x=3

we know that (x+y)²= x²+y²+2xy

(x+1/x)²= x²+(1/x)²+2x(1/x)

(3)² = x²+(1/x)² +2

x²+1/x² =7

squaring on both the sid

(x²+1/x²)² = 49

x⁴+1/x⁴+2(x²)(1/x²) = 49

x⁴ +1/x⁴ = 49-2

x⁴+1/x⁴ = 47

again cubing on both the sides ,

(x+1/x)³ = x³+1/x³+3*x*1/x(x+1/x)

3³ = x³+1/x³+3(3)

x³+1/x³=27-9

x³+1/x³ = 18

The value x²+1/x² =7, x³+1/x³ = 18, x⁴+1/x⁴ = 47

问题16.如果x ⁴ + 1 / x ⁴ = 194，计算x ² + 1 / x ² ，x ³ + 1 / x ³ ，x + 1 / x

解决方案：

Given,

x⁴+1/x⁴=194 —– 1

add and substract (2*x²*1/x²) on the left side in above given equation

x⁴+1/x⁴ +2*x²*1/x² -2*x*1/x² = 194

x⁴+1/x⁴+2*x²*1/x² -2 =194

(x²)²+(1/x²)²+ 2*x²*1/x² = 196

(x²+1/x²)² = 196

(x²+1/x²) = 14 ——— 2

add and subtract (2*x*1/x) on the left side in above given equation

x²+1/x²+2*x*1/x-2*x*1/x =14

(x+1/x)² = 14 +2

(x+1/x) = 4 ———— 3

Now cubing eq-3 on both sides.

(x+1/x)³ = 4³

x³+1/x³+3*x*1/x(x+1/x) = 64

x³+1/x³ +3*4 = 64

x³+1/x³ = 64 -12

= 52

Hence, the values of (x²+1/x²) = 14, (x³+1/x³) = 52, (x+1/x) = 4

问题17。如果，则^{找到27x 3} + 8y ^3的值

(a)3x + 2y = 14且xy = 8

(b)3x + 2y = 20且xy = 14/9

解决方案：

(a) Given, 3x+2y = 14 and xy = 8

cubing on both the sides

(3x+2y)³ = 14³

we know that, (a+b)³=a³+b³+3ab(a+b)

27x³+8y³+3(3x)(2y)(3x+2y) = 2744

27x³+8y³+18xy(3x+2y) = 2744

27x³+8y³+18*8*14 = 2744

27x³+8y³ = 2744 – 2016

27x³ +8y³ = 728

Hence, the value of 27x³ +8y³ = 728

(b) Given, 3x+2y = 20 and xy=14/9

cubing on both the sides

we know that, (a+b)³=a³+b³+3ab(a+b)

27x³+8y³+3(3x)(2y)(3x+2y) = 8000

27x³+8y³+18xy(3x+2y) = 8000

27x³+8y³+18*14/9*20 = 8000

27x³+8y³ = 8000 – 560

= 7440

Hence, the value of 27x³+8y³ = 7440

问题18.如果4x-5z = 16且xz = 12，则^{找到64x 3} -125z ^3的值

解决方案：

Given, 64x³ – 125z³

Here, 4x -5z = 16 and xz = 12

cubing (4x-5z)³ = 16³

we know that (a-b)³ =a³-b³-3ab(a-b)

(4x -5z)³ = (4x)³-(5z)³-3(4x)(5z)(4x-5z)

(16)³ = 64x³ -125z³-60(4x-5z)

4096 = 64x³-125z³-60(16)

64x³-125z³ = 4096 + 960

= 5056

Hence, the value of 64x³ – 125z³ = 5056

问题19.如果x-1 / x = 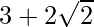$3+2\sqrt2$ ，找到x ³ -1 / x ^3的值

解决方案：

Given, x-1/x =$3+2\sqrt{2}$

cubing both the sides,

we know that , (a-b)³ = a³-b³-3ab(a-b)

(x-1/x)³ = x³ -1/x³ -3*x*1/x(x-1/x)

(3+2\sqrt{2})^3 = x³-1/x³ -3(3$3+2\sqrt{2}$)

$3^3+(2\sqrt2)^3+3*3*(2\sqrt{2})(3+2\sqrt{2}) = x^3-1/x^3-3(3-2\sqrt{2})$

$3^3+(2\sqrt2)^3+3*3*(2\sqrt{2})(3+2\sqrt{2})+3(3-2\sqrt{2}) = x^3-1/x^3$

x³-1/x³ = 108+$76\sqrt{2}$

Hence, the value of x³-1/x³ = 108+$76\sqrt{2}$