第 10 类 RD Sharma 解决方案 - 第 11 章构造 - 练习 11.1

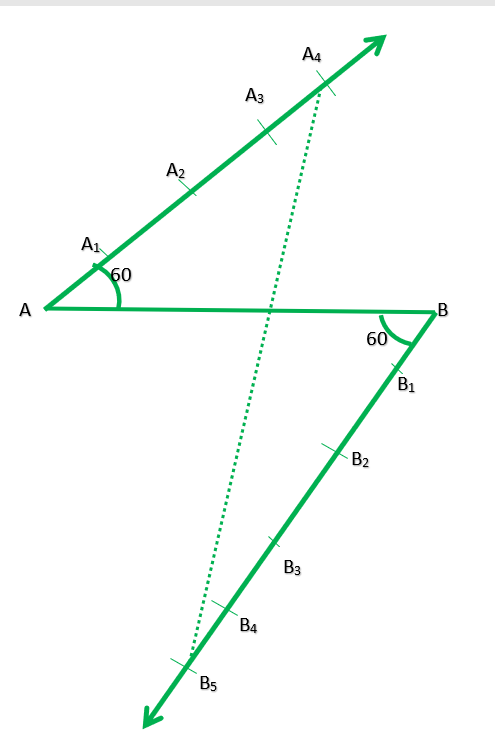
问题 1. 确定一个点，该点在内部以 2 : 3 的比例划分长度为 12 厘米的线段。另外，证明你的结构是合理的。

解决方案：

Steps of construction:

Step 1. Draw a line segment AB =12cm.

Step 2. Through the A and B draw two parallel line on each sides of AB.

Step 3. Cut 2 equal parts on AX and 3 equal parts on BY such that AX₁=X₁X₂  and BX₁=Y₁Y₂=Y₂Y₃

Step 4. Join X2Y3 which intersects AB at P $\frac{AP}{PB}=\frac{2}{3}$

Justification: 

In ∆AX₂P and ∆BY₃P, we have

∠APX₂=∠BPY₃          {Because they are vertically opposite angle}

∠X₂AP=∠Y₃BP

∆AX₂P and ∆BY₃P {Because AA similarity}

Therefore, $\frac{AP}{BP}=\frac{AX_2}{BY_3}=\frac{2}{3}$ {Because of C.P.CT}

问题 2. 以 4 : 3 的比例在内部划分一条长度为 9 厘米的线段。另外，给出构造的理由。

解决方案：

Steps of construction:

Step 1. Draw a line segment AB = 9 cm.

Step 2. Through the points, A and B, draw two parallel lines AX and BY on the opposite side of AB

Step 3. Cut 4 equal parts on AX and 3 equal parts on BY such AX₁=X₁X₂=X₂X₃=X₃X₄ and BY₁=Y₁Y₂=Y₂Y₃

Step 4. Join x₄y₃ which intersects AB at P.

Therefore, $\frac{AP}{PB}=\frac{4}{3}$

Justification:

In ∆PX₄ and ∆BPY₃, we have

∠APX₄=∠BPY₃         {Because they are vertically opposite angles}

∠PAX₄=∠PBY₃       {Because they are alternate interior angle}

 ∆APX₄     ∆BPY₃   {Because AA similarity}

Therefore, $\frac{PA}{PB}=\frac{AX_4}{BY_3}=\frac{4}{3}$ {Because of C.P.C.T}

问题 3. 将长度为 14 厘米的线段在内部以 2 : 5 的比例分割。另外，证明你的结构是合理的。

解决方案：

Steps of construction:

Step 1. Draw a line segment AB = 14 cm.

Step 2. Draw a ray AX making an acute angle with AB.

Step 3. From B, draw another ray BY parallel to AX.

Step 4. From AX, cut off 2 equal parts and from B, cut off 5 equal parts.

Step 5. Join 2 and 5 which intersects AB at P.

P is the required point which divides AB in the ratio of 2 : 5 internally.

Justification:

In ∆PX₂ and ∆BPY₅, we have

∠APX₂=∠BPY₅         (vertically opposite angles)

∠PAX₂=∠PBY₅        (Because they are alternate interior angle)

∆APX₂     ∆BPY₅     (Because AA similarity)

Therefore,

$\frac{PA}{PB}=\frac{AX_2}{BY_5}=\frac{2}{5}$

(Because of C.P.C.T)

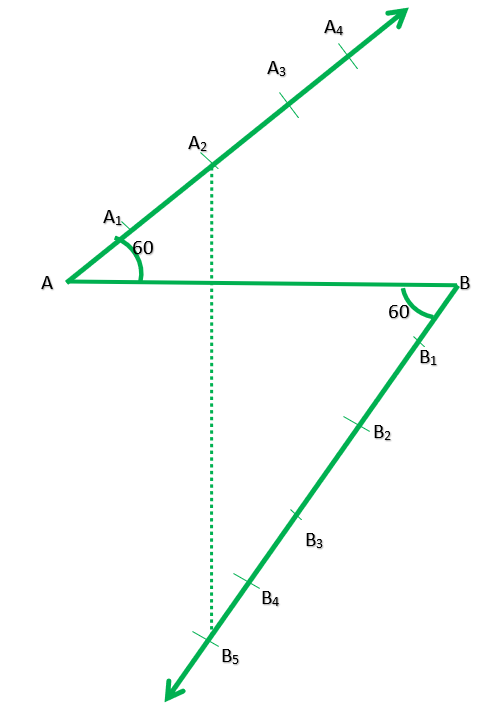
问题 4. 画一条长度为 8 厘米的线段，并在内部按 4 : 5 的比例进行分割。

解决方案：

Steps of construction:

Step 1. Draw a line of 8cm.

Step 2. Through the points, A and B, draw two parallel lines AX and BY on the opposite side of AB

Step 3. Cut 4 equal parts AX and 3 equal parts on BY.

Step 4. Join x₄y₅ which intersects AB at P.

Justification:

In ∆PX₄ and ∆BPY₅, we have

∠APX₄=∠BPY₅         (vertically opposite angles)

∠PAX₄=∠PBY₅       (alternate interior angle)

∆APX₄     ∆BPY₅   (AA similarity)

Therefore,

$\frac{PA}{PB}=\frac{AX_4}{BY_5}=\frac{4}{5}$

(Because of C.P.C.T)