11类RD Sharma解决方案-第33章概率–练习33.4 |套装1

问题1(a)。如果A和B是与随机实验相关的互斥事件，使得P(A)= 0.4和P(B)= 0.5，则找出：

(i)P(AUB)

(ii) $P(\bar{A}∩ \bar{B})$

(iii) $P(\bar{A} ∩ B)$

(iv) $P(A ∩ \bar{B})$

解决方案：

A & B are mutually exclusive events,                

Hence, P(A n B) = 0

(i) P(A U B) = P(A) + P(B) – P(A n B)          -(A & B are mutually exclusive events hence P(A n B) = 0)

= 0.4 + 0.5 – 0

= 0.9

(ii)$P(\bar{A} ∩ \bar{B}) = 1 - P(A U B)$

= 1-0.9

= 0.1

(iii) $P(\bar{A} ∩ B)$ = P(B) – P(A ∩ B)

= 0.5 – 0

= 0.5

(iv)$P(A ∩ \bar{B})$ = P(A) – P(A ∩ B)

= 0.4 – 0

= 0.4

问题1(b)。 A和B是两个事件，使得P(A)= 0.54，P(B)= 0.69和P(A∩B)= 0.35，然后找到：

(i)P(AUB)

(ii) $P(\bar{A} ∩ \bar{B})$

(iii) $P(A ∩ \bar{B})$

(iv) $P(B ∩ \bar{A})$

解决方案：

(i) P(A U B) = P(A) + P(B) – P(A ∩ B)

P(A U B) = 0.54 + 0.69 – 0.35

P(A U B) = 0.88

(ii) $P(\bar{A} ∩ \bar{B})$= 1 – P(A U B)

=  1-0.88

= 0.12

(iii) $P(A ∩ \bar{B})$ = P(A) – P(A ∩ B)

 = 0.54-0.35

= 0.19

(iv) $P(B ∩ \bar{A})$= P(B)-P(A ∩ B)

= 0.69-0.35

= 0.34

问题1(c)。填写下表中的空白                         

	P(A)	P(B)	P(A ∩ B)	P(A U B)
i	1/3	1/5	1/15	—
ii	0.35	—	0.25	0.6
iii	0.5	0.35	—	0.7

解决方案：

(i) P(A) = 1/3

P(B) = 1/5

P(A U B) = 1/15

P(A U B) = P(A) + P(B) – P(A ∩ B)

= 7/15

(ii) P(A) = 0.35

P(A ∩ B) = 0.25

P(A U B) = 0.6

P(A U B) = P(A) + P(B) – P(A ∩ B)

0.60 = 0.35 + P(B) – 0.25

0.60 – 0.35 + 0.25 = P(B) 

P(B) = 0.50

= 0.50

(iii) P(A) = 0.5

P(B) = 0.35

P(A U B) = 0.7

P(A ∩ B) = P(A) + P(B) – P(A U B)

= 0.5 + 0.35 – 0.7

= 0.15

问题2. A和B是与随机实验相关的两个事件，即P(A)= 0.3，P(B)= 0.4，P(AUB)= 0.5。找出P(A∩B)?

解决方案：

P(A) = 0.3

P(B) = 0.4

P(A U B) = 0.5

P(A ∩ B) = P(A) + P(B) -P(A U B)

= 0.3+0.4 – 0.5

= 0.2

问题3. A和B是与随机实验相关的两个事件，即P(A)= 0.5，P(B)= 0.3，P(A n B)= 0.2。查找P(AUB)?

解决方案：

P(A) = 0.5

P(B) = 0.3

P(A U B) = 0.2

P(A U B) = P(A) + P(B) – P(A ∩ B)

= 0.5 + 0.3 – 0.2

= 0.6

问题4：A和B是与随机实验相关的两个事件，因此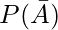， $P(\bar{A})$ = 0.5，P(AUB)= 0.8，P(A n B)= 0.3。找到P(B)?

解决方案：

P(A) = 1 – $P(\bar{A})$

= 1 – 0.5

= 0.5

By applying the addition theorem of probability-

P(A U B) = P(A) + P(B) – P(A ∩ B)

0.8 = 0.5 +P(B) – 0.3

0.8 = P(B) + 0.2

P(B) = 0.8 – 0.2

= 0.6

问题5. A和B是两个互斥的事件，因此P(A)= 1/2和P(B)= 1/3。找到P(A或B)?

解决方案：

 A and B are two mutually exclusive events 

Therefore, P(A ∩ B) = 0

P(A U B) = P(A) + P(B)

= 1/2 + 1/3

= 5/6

问题6.有三个事件A，B和C，其中一个必须且仅会发生。对A的赔率是8比3，对B的赔率是5比2，对C的赔率是多少?

解决方案：

$P(\bar{A})$ / P(A) = 8/3

1- P(A)/P(A) = 8/3          -($P(\bar{A})$ = 1 – P(A))

P(A) = 1 + 8/3 = 3/11         -(1)

Similarly, P(B) = 2/7          -(2)

A, B & C are mutually exclusive events 

P(A U B U C) = P(S)

= P(A) + P(B) + P(C) = 1

= 3/11 + 2/7 + P(C) = 1

= 43/77 + P(C) = 1

= 1  – 43/77 = P(C)

= P(C) = 34/77 

= $P(\bar{C})$ = 1 – P(C)

= 43/77

Odds against C is =

$P(\bar{C})$ : P(C) = 43/77  : 34/77

= 43 : 34

问题7.必须发生两个事件之一。考虑到一个人的机会是其他人的三分之二，是否找到其他人胜算的机会?

解决方案：

Let chance in favor of other be p,

So, therefore

p + 2/3p = 1

(5/3) * p = 1

p = (3/5)

Odds in favor of other will be:

= (2/5)/(3/5) = (3/2)

= 3 : 2

问题8.一张纸牌是从52张纸牌中随机抽取的。找到成为黑桃或国王的可能性了吗?

解决方案：

A card is drawn from well shuffled pack of 52 cards,

Hence, S(sample space) = ⁵²C₁

= 52          -(1)(ⁿ C _r    = n! / (n – r)! 

                         n – number of items 

                         r – how many times an item is taken)

Let A = event of choosing a card of spade

A = ¹³C₁          -(a set of spade have 13 cards)

= 13          -(2)

Let B = event of choosing a  king 

B = ⁴C₁          -(there are 4 kings in pack of 52 cards)

= 4           -(3)

We can also have an event where the king drawn is of spade,

Hence, P(A n B) = 1          -(4)

P(A U B) = P(A) + P(B) – P(A ∩ B)

= 13/52 + 4/52 – 1/52          -(From 1, 2, 3, 4)

= 18/52

= 4/13

问题9.在一次掷出两个骰子的情况下，找到既不是加倍也不是9的概率吗?

解决方案：

Two dices are thrown hence the sample space will be –

S = 6 ² 

= 36          -(1)

Let A be the event of choosing doublet – 

A = {(1,1), (2,2), (3,3), (4,4), (5,5), (6,6)}

A = 6          -(2)

Let B be the event of choosing sum equal to 9

B = {(3,6), (4,5), (5,4), (6,3)}

B = 4          -(3)

Here event A and B can’t occur together i.e. sum is 9 and dice are doublet

Hence, P(A ∩ B) = 0          -(4)

Now,

P (A U B) = P(A) + P(B) – P(A ∩ B)          -(From 1, 2, 3, 4)

= 6/36 + 4/36

= 10/36

= 5/18

Now, the probability that neither double nor the total is 9, 

= 1 – P (A U B)

= 1 – 5/18

= 13/18

问题10.从500中随机选择一个自然数。一个数字被5或3整除的概率是多少?

解决方案：

Since a number is chosen from the first 500 natural numbers, 

Hence, n(S) = 500          -(1)

Let A be event of choosing a number divisible by 3,

A = {3,6,9 ……………….., 498}

n(A) = 166           (t _n  = a + (n-1) *d   

                              a = 3, d = 3, t _n = 498

                              498 = 3 + (n -1)3

                             n = 166)

P(A) = 166/500          -(2)

Let B be the event of choosing a number divisible by 5,

B = {5,10,15,20. . . . . .495,500}

n(B) = 100                  

P(B) = 100/500          -(3)

We can also have a event where the number is divisible by 5 and 3

P(A n B) = {15,30,45. . . . 495}

n(A n B) = 33

P(A n B) = 33/500          -(4)

Now,

P(A U B) = P(A) + P(B) – P(A ∩ B)

= 166/500 + 100/500 – 33/500          -(From 1, 2, 3, 4)

= 233/500

问题11：一次掷两次骰子。这个数字之一出现3的概率是多少?

解决方案：

Two dice are thrown, Hence the sample space will be

n(S) = 36          -(1)

Let A be the event of getting 3 at first throw –

n(A) = 6

P(A) = 6/36

= 1/6          -(2) ((3,1), (3,2). . . (3,6))

Let B be the event of getting 3 at second throw-

n(B) = 6

P(B) = 6/36

= 1/6         -(3)

Also, P(A ∩ B) = 1/36          -(4)(A ∩ B = (3,3))

P(A U B) = P(A) + P(B) – P(A ∩ B)

=1/6 + 1/6 – 1/36          -(From 2, 3, 4)

= 11/36

问题12.从52张纸牌中抽取一张纸牌。发现它是黑桃还是国王?

解决方案：

There are 52 cards, Hence the sample space will be-

n(S) = 52          -(1)

Let A be the event of getting a Spade card

n(A) = 13        -(There are 13 spade cards in pack of 52 cards)

P(A) = 13/52

=1/4          -(2)

Let B be the event of getting a King

n(B) = 4          -(a pack of cards has 4 kings)

P(B) = 4/52      

=1/13          -(3)

Also,

P(A ∩ B) = 1/52          -(4)(A ∩ B = A king of spade)

P(A U B) = P(A) + P(B) – P(A ∩ B)

= 1/4 + 1/13 – 1/52         -(From 2, 3, 4)

= 4/13

问题13.学生通过英语和印地语的概率为0.5，而未通过英语和印地语的概率为0.1。如果通过英语的概率为0.75，那么通过印地语考试的概率是多少?

解决方案：

Let E be the event of passing in the English exam –

P(E)  = 0.75          -(1)

Let H be the event of passing in the Hindi exam –

P(H) = ?

The probability that a student will pass in English and Hindi is 0.5,

Hence, P(E n H) = 0.5          – (2)

The probability that will pass in neither is 0.1,

Hence, 

$P(\bar{E} ∩ \bar{H})$ = 0.1

1 – P(E U H) = $P( \bar{E} ∩ \bar{H} )$

P(E U H) = 1 – 0.1

= 0.9          -(3)

Now,

P(E U H) = P(E) + P(H) – P(E ∩ H)

0.9 = 0.75 + P(H) – 0.5          -(From 1, 2, 3)

P(H) = 0.65
   

问题14.从1到100中选择一个数字。找到该数字可被4或6整除的概率吗?

解决方案：

One number is chosen from 1 to 100,

Hence, the sample space is – n(S) – 100

Let A be the event of choosing a number divisible by 6;

n(A) = {6, 12, 18, 24. . . . 96}

= 25          -(use the T _n  term formula)

P(A) = 25/100

= 1/4         -(1)

Let B be the event of choosing a number divisible by 4;

n(B) = {4, 8, 12, 20. . . . 100}

= 25          -(use the T n term formula)

P(B) = 25/100

= 1/4          -(2)

Also, (A ∩ B) = {12,24,36. . . 96}

n(A ∩ B) = 8

P(A ∩ B) = 8/100          -(3)

P(A U B) = P(A) + P(B) – P(A∩ B)

= 1/4  + 1/4 – 8/100          -(From 1, 2, 3)

= 33/100