类12 NCERT解决方案-数学第I部分-第6章导数的应用-第6章的其他练习|套装1

问题1.使用微分，找到以下各项的近似值：

(i)(17/81) ^1/4

(ii)33 ^-1/5

解决方案：

(i) (17/81)^1/4 

Let y = x^1/4, x = 16/81 and △x = 1/81

△y = (x + △x)^1/4 – x^1/4

= (17/81)^1/4 – (16/81)^1/4

= (17/81)^1/4 – (2/3)

So, 

(17/81)^1/4 = (2/3) + △y 

Here, dy is approximately equal to △y 

dy = (dy/dx)△x

= $\frac{1}{4x^{\frac{3}{4}}}(△x)$

= $\frac{1}{4(\frac{16}{81})^{\frac{3}{4}}}(\frac{1}{81})$

= 27/32 × 1/81

= 1/96

= 0.010

Hence, the approximate value of (17/81)^1/4 = 2/3 + 0.010 = 0.677

(ii) 33^-1/5

Let y = x^-1/5, x = 32 and △x = 1

△y = (x + △x)^-1/5 – x^-1/5

= (33)^-1/5 – (32)^-1/5

= (33)^-1/5 – 1/2

So, 

(33)^-1/5 = 1/2 + △y 

Here, dy is approximately equal to △y 

dy = (dy/dx)△x

= $\frac{-1}{5x^{\frac{6}{5}}}(△x)$

= $\frac{-1}{5(32)^{\frac{6}{5}}}(1)$

= -1/320

= -0.003

Hence, the approximate value of (33)^-1/5 = 1/2 – 0.003 = 0.497

问题2。证明f(x)= log x / x给出的函数在x = e处具有最大值。

解决方案：

The given function is f(x) = log x/x

$f'(x)=\frac{x(\frac{1}{x})-\log x}{x^2}=\frac{1-\log x}{x^2}$

Now, f'(x) = 0

1 – log x = 0

log x = 1

log x = log e

x = e

Now, $f''(x)=\frac{x^2(-\frac{1}{x})-(1-\log x)(2x)}{x^4}$

 $=\frac{-x-2x(1-\log x}{x^3}$

 $=\frac{-3+3\log x}{x^3}$

$f''(e)=\frac{-3+2\log e}{e^3}=\frac{-3+2}{e^3}=\frac{-1}{e^3}<0$

Therefore, by second derivatives test, f is the maximum at x = e.

问题3：等腰三角形的等边三角形的底边b固定，以每秒3 cm的速度减小。当两个相等边等于底边时，面积减小的速度有多快?

解决方案：

Given an isosceles triangle with fixed base b.

Let the other two sides be of length x.

Now its given that,

dx/dy = -3cm/s

Now semi-perimeter(s) = x + x + b/2

s = x + b/2

Area [by heron’s formula] = $\sqrt{s(s-x)(s-x)(s-b)}$

$A=(s-x).\sqrt{s(s-b)}$

$A=\frac{b}{2}\sqrt{(x+\frac{b}{2})(x-\frac{b}{2})}$

$A=\frac{b}{2}\sqrt{x^2}\frac{-b^2}{2}$

$A=\frac{b}{4}\sqrt{4x^2-b^2}$

To find: dA/dt = ?

DA/dt = ?

$\frac{dA}{dt}=\frac{b}{4}.\frac{1}{2\sqrt{4x^2-b^2}}.8x.\frac{dx}{dt}$

$\frac{dA}{dt}=\frac{b}{4}.\frac{1}{2b\sqrt{3}}.8b.(-3)$

dA/dt = -√3b cm²/s

Hence, the area is decreasing at the rate = √3b cm²/s

问题4：找到通过点(1、2)的曲线x ² = 4y的法线方程。

解决方案：

Given area: x² = 4y

On Differentiating both sides with respect to y,

2x(dx/dy) = 4

dx/dy = 2/x

Slope = -1/m = -2/x

By point slope form equation of normal will be,

y – 2 = -1(x – 1)

x + y = 3 is the required equation of normal.

问题5。证明曲线x的任意点θ处的法线x =acosθ+aθsinθ，y =asinθ–aθcosθ与原点的距离是恒定的。

解决方案：

Given curve,

x = acosθ + aθsin θ

y = asinθ – aθcos θ

Now -dx/dy = slope of normal =$\frac{\frac{-dx}{dθ}}{\frac{dy}{dθ}}$           -(1)

$\frac{dx}{dθ}$ = -asinθ + asinθ + aθcosθ

$\frac{dx}{dθ}$ = aθcosθ          -(2)

 $\frac{dx}{dθ}$ = acosθ + aθsinθ – acosθ

 $\frac{dx}{dθ}$ = aθsinθ                -(3)

$\frac{-dx}{dy}=\frac{-aθ\cos θ}{aθ\sin θ}$     -(From 1, 2 & 3)

-dx/dy = -cotθ

Now using point slope from, equation of normal will be, 

$y-a\sin θ+aθ\cos θ=\frac{-\cos θ}{\sin θ}(x-a\cos θ-aθ\sinθ)$

ysinθ – asin^-2θ + aθcosθsinθ = -xcosθ + acos²θ + aθsinθcosθ

ysinθ + ysinθ − a = 0

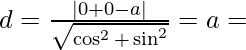$d=\frac{|0+0-a|}{\sqrt{\cos^2θ+\sin^2θ}}=a=$ constant.

Hence proved

问题6.求出以下函数f给出的区间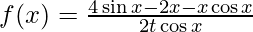： $f(x)=\frac{4\sin x-2x-x\cos x}{2t\cos x}$是

(i)增加(ii)减少

解决方案：

$f(x)=\frac{4\sin x-2x-x\cos x}{2+\cos x}$

$f'(x)=\frac{(2+\cos x)(4\cos x-2+x\sin x-\cos x)+(4\sin x-2x-x-\cos x)(\sin x)}{(2+\cos x)^2}$

$f'(x)=\frac{4\cos x-cos^2x}{(2+\cos x)^2}$

(i) For f(x) tp be increasing f'(x) ≥ 0

$\frac{4\cos x-cos^2x}{(2+\cos x)^2}>0$

 $\cos x(4-\cos x)>0$

Now, 4 – cos x > 0           -(because 4 – cos x ≥ 3)

So, cos x > 0

Hence, f(x) is increasing for 0 < x < x/2 and 3π/2 < x < 2π

(ii) For f(x)to be decreasing,

f'(x) < 0

$\frac{4\cos x-\cos^2x}{(\cos x+2)^2}<0$

cosx(4 − cosx) < 0

cosx < 0

Hence, f(x) is decreasing for π/2 < x < 3π/2

问题7.找到由f(x)给出的函数f的时间间隔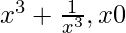$x^3+\frac{1}{x^3},x≠0$是

(i)增加(ii)减少

解决方案：

f(x) = $x^3+\frac{1}{x^3},x≠ 0$

f'(x) $=3x^2-\frac{1}{3x^4}$

(i) For f(x) to be increasing,

f'(x) > 0

$3x^2-\frac{1}{3x^4}>0$

$\frac{9x^6-1}{3x^4}>0$

9x⁶ > 1       

$x>(\frac{1}{9})^{\frac{1}{6}}$or $x∈((\frac{1}{9}^{\frac{1}{6}}),∞)$

(ii) For f(x) to be decreasing,

f'(x) < 0 $3x^2-\frac{1}{3x^4}<0$

9x⁶ < 1               

$x<(\frac{1}{9})^{\frac{1}{6}}$or $x∈(∞,(\frac{1}{9}^{\frac{1}{6}}))$

问题8.找出椭圆上刻有等腰三角形的最大面积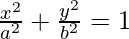$\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$其顶点位于主轴的一端。

解决方案：

Given ellipse: $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$

Its major axis is the x-axis

Using parametric form of ellipse, x = acosθ, y = bsinθ,

If coordinates of A are (acosθ, bsinθ)

Then B’S coordinates will be (acosθ, -bsin θ).

Now, OC = a, OD = acos θ, so CD = a(1 + cos θ)

AB = |AD| + |BD| = 2b sin θ

Area of △ABC = 1/2.AB.CD

= 1/2.2bsin θ.a(1 + cos θ)

 △(θ) = ab.sinθ.(1 + sin θ)

For maxima/minima, put △'(θ) = 0

△'(θ) = ab[cosθ[1 + cosθ] + sinθ[-sinθ]]

△'(θ) = ab[2cos²θ + cosθ – 1] = 0

2cos²θ + cosθ – 1 = 0

2cos²θ + 2cosθ – cosθ – 1 = 0

2cosθ(cosθ + 1) – 1(cosθ + 1) = 0

(2cosθ – 1).(cosθ + 1) = 0

cosθ = 1/2 or cosθ = -1

If cosθ = -1, then sinθ = 0 & △(θ) = 0

But if cosθ = 1/2, sinθ = √3/2 & △(θ) = ab.$\frac{\sqrt{3}}{2}(1+\frac{1}{2})$

$△(θ)_{max}=\frac{2\sqrt{3}ab}{4}$

问题9.建造一个具有矩形底部和矩形侧面，顶部开口的水箱，其深度为2m，容积为8m3。如果建造水箱，底座的成本为每平方米70卢比，侧面的成本为每平方米45卢比。最便宜的战车价格是多少?

解决方案：

Given:

Depth of tank = 2m

Volume = 8m³

Let the length be equal to x & width be to y

The base area will be equal to x.y.

Area of sides will be equal to; 2x, 2y, 2x, 2y

Now, volume = x.y.2 = 2xy = 8m³

so, xy = 4m²          -(1)

y = 4/x

Total cost = 70.base + 45.(sides)

c = 70xy + 45(2x + 2y + 2x + 2y)

c = 70.4 + 45.4(x + y)          -(xy = 4)

c(x) = 180 – \$\frac{180.4}{x^2}=0$

$1-\frac{4}{x^2}=0$

x² = 4                        

x = ±2, x = 2,           (Rejecting -ve value)

y = 4/x = 4/2 = 2

Now cost c(x) = 280 + 180(x + 4/x)

c = 280 + 180(2 + 2)

c = 1000 rupees

问题10.圆和正方形的周长之和为k，其中k为常数。证明当正方形的边是圆的半径的两倍时，它们的面积之和最小。

解决方案：

Let the sides of square be x & radius of circle be r.

Perimeter of square = 4x

Circumference of circle = 2πr

Now given that, 4x + 2πr = k          -(1)

x = $\frac{k-2πr}{4}$

Area of square = x²

Area of circle = πr²

Sum of areas = x² + πr²

$s(r)=(\frac{k-2πr}{4})^2+πr^2$

Put s'(r) = 0

$s(r)=2(\frac{k-2πr}{4})(\frac{-π}{2})+2πr=0$          -(From eq(1))

$πr=\frac{π}{2}\frac{k-2πr}{4}$

8πr = kπ – 2π²r

8r = k – 2πr

8r = (4x + 2πr) – 2πr           -(k = 4x + 2πr)

8r = 4x

x = 2r

Hence, proved that the sides of the square is double the radius of the circle.

问题11：窗户是矩形的，上面是半圆形的开口。窗户的总周长为10 m。找到窗户的尺寸，以允许整个开口进入最大的光线。

解决方案：

Let the length of the rectangle = x

the breadth of the rectangle = y

and the radius of the semicircle = x/2

So given that total perimeter of the window = 10m

P = πx/2 + x + 2y = 10

x(1 + π/2) + 2y = 10

2y = 10 – x(1 + π/2)

y = 5 – x(1/2 – π/4)          -(1)

Now, the area of the window

$A=\frac{πx^2}{2}+xy$          -(2)

From eq(1) put the value of y in eq(2), we get

$A=x.[5 - x(\frac{1}{2} - \frac{π}{4})]+\frac{πx^2}{2}$

= 5x – x²(1/2 + π/4) + πx²/8

On differentiating we get

A’ = 5 – 2x(1/2 + π/4) + 2xπ/8

= 5 – x(1 + π/2) + xπ/4

Put A’ = 0

 5 – x(1 + π/2) + xπ/4 = 0

-x(1 + π/2) + xπ/4 = -5  

x(-1 – π/2 + π/4) = -5

x(-1 – π/2 + π/4) = -5

x(1 + π/4) = 5

x = 5/ (1 + π/4) 

x = 20/ π + 4

Hence, the length of the rectangle = 20/ π + 4

Now put the value of x in eq(1)

y = 5 – (20/ π + 4)(1/2 – π/4)      

y = 10/π + 4

Hence, breadth of the rectangle = 10/π + 4

and the radius of the semicircle = x/2 = $\frac{\frac{20}{π + 4}}{2}$ = 10/π + 4

第6章衍生物的应用–第6章的其他练习|套装2