类12 NCERT解决方案-数学第I部分–第6章导数的应用-练习6.3 |套装2

第6章衍生物的应用-练习6.3 |套装1

问题14：在指定点找到给定曲线的切线和法线的方程式：

(i)在(0，5)处y = x ⁴ – 6x ³ + 13x ² – 10x + 5

(ii)在(1，3)处^{y = x 4} – 6x ³ + 13x ^{2 – 10x + 5}

(iii)在(1，1)处y = x ³

(iv)y = x ² at(0，0)

(v)x = cos t，y =在t =π/ 4时的sin t

解决方案：

(i) Given curve

y = x⁴ – 6x³ + 13x² – 10x + 5

Given point, (0, 5)

dy/dx = 4x³ – 18x² + 26x – 10

dy/dx = 4(0)³ – 18(0)² + 26(0) – 10

dy/dx = -10,

-dx/dy = 1/10 

Now, with the help of points slope form 

y – y₁ = m(x – x₁)

y – 5 = -10(x – 0)

y + 10x = 5 is the required equation of the tangent 

For equation of normal,

y – y1 = $\frac{-dx}{dy}(x-x_1)$

y – 5 = $\frac{1}{10}(x-0)$

10y – x – 50 is the equation of normal.

(ii) Given curve: y = x⁴ – 6x³ + 13x² – 10x + 5

Given point is (1, 3)

dy/dx = 4x³ – 18x² + 26x – 10 

dy/dx = 4(1)³ – 18(1)² + 26(1) – 10

dy/dx = 4 – 18 + 26 – 10 = 2

dy/dx = 2       

-dx/dy = -1/2  

Using point slope form, equation of tangent is 

y – y₁ = dy/dx(x – x1)

y – 3 = 2(x – 1)

y – 2x = 1 is the equation of tangent.

Using point slope form, equation of normal is

y –  y₁ = -dx/dy(x – 1)

y – 3 = -1/2(x – 1)

2y – 6 = -x + 1

2y + x = 7 is the equation of normal.

(iii) Given curve : y = x³

Given point is (1, 1)

dy/dx = 3x²

dy/dx = 3(1)² = 3

dy/dx = 3 & -dx/dy = -1/3

Using point slope form, equation of tangent is y – y₁ = dy/dx(x – x₁)

y – y₁ = dy/dx(x – x₁)

y – 1 = 3(x – 1)

y – 3x + 2 = 0 is the equation of tangent

Using point slope form, equation of normal is 

y – y1 = $\frac{-dx}{dy}(x-x_1)$

y – 1 = $\frac{-1}{3}(x-1)$

3y – 3 = -x + 1

3y + x = 4 is the equation of normal.

(iv) Given curve: y = x²

Given point (0, 0)

dy/dx = 2x

dy/dx = 0

dy/dx = 0 & -dx/dy = not defined is 

y – y₁ = dy/dx(x – x₁)

y – 0 = 0(x – 0)

y = 0 is the equation of tangent.

Using point slope form, equation of normal is 

y – y₁ = $\frac{-dx}{dy}(x-x_1)$           -(1)

-dx\dy is undefined, so we can write eq(1) as 

$\frac{-dy}{dx}(y-y_1)=x-x_1$

Now putting dy/dx = 0 we get

0(y – 0) = x-0

x = 0 is the equation of normal.

(v) Equation of curve: x = cos t and y = sin t

Point t = π/4

$\frac{dy}{dx}=\frac{\frac{dy}{dt}}{\frac{dx}{dt}}$    -(1)

$\frac{dx}{dt}=\frac{d(\cos t)}{dt}=-\sin t$

$\frac{dy}{dt}=\frac{d(\sin t)}{dt}=\cos t$

On putting these values in eq(1), we get

$\frac{dy}{dx}=\frac{\cos t}{-\sin t}=-\cot t$

$\frac{dy}{dx}=-\cot \frac{π}{4}=-1$

dy/dx = -1 & -dx/dy = 1  

Now for t = π/4,

y₁ = sin t = sin(π/4) = 1/√2

x₁ = cos t = cos(π/4) = 1/√2

The point is (1/√2, 1/√2) 

y – y₁ = $\frac{dy}{dx}(x-x_1)$

y – (1/√2) = -1(x – 1/√2)

y – 1/√2 = -x + 1/√2

x + y = √2 is the equation of normal is 

$y-y_1=\frac{-dx}{dy}(x-x_1)$

y – 1/√2 = 1(x – 1/√2)

x = y is the equation of normal.

问题15.求曲线y = x ² – 2x + 7的切线的方程为

(i)平行于第2x – y + 9 = 0行

(ii)垂直于线5y – 15x = 13

解决方案：

Given curve: y = x² – 2x + 7

On differentiating w.r.t. x, we get

dy/dx = 2x – 2         -(1)

(i) Tangent is parallel to 2x – y + 9 = 0 that means,

Slope of tangent = slope of 2x – y + 9 = 0

y = 2x + 9

Slope = 2            -(Comparing with y = mx + e)

dy/dx = slope = 2

2x – 2 = 2

x₁ = 2

Corresponding to x₁ = 2,

y₁ = x₁² – 2x₁ + 7 

y₁ = (2)² – 2(2) + 7

y₁ = 7

The point of contact is (2, 7).

Using point slope form, equation of tangent is 

y – y₁ = $\frac{dy}{dx}(x-x_1)$

y – 7 = 2(x – 2)

y – 2x = 3 is the equation of tangent. 

(ii) Tangent is perpendicular to the line 5y – 15x = 13

That means (slope of tangent) x (slope of line) = -1

For, slope of line 5y – 15x = 13

5y = 15x + 13

y = 3x + 13/15

Slope = 3

(Slope of tangent) x 3 = -1

Slope of tangent =-1/3 

Now, y = x² – 2x + 7

dy/dx = 2x – 2

On comparing dy/dx with slope, we get

2x – 2 = -1/3

6x – 6 = -1

6x = 5

x₁ = 5/6

For x₁ = 5/6,          

y₁ = x₁² – 2x₁ + 7 

y₁ = (5/6)₁² – 2(5/6)₁ + 7 

y₁ = 217/36 

Now using point slope form, equation of tangent is 

y – y₁ = m(x – x₁)

$y-\frac{217}{36}=\frac{-1}{3}(x-\frac{5}{6})$

$\frac{36y-217}{36}=-1\frac{(6x-5)}{6.3}$

36y – 217 = -12x + 10

36y + 12x = 227 is the required equation of tangent.

问题16：证明在x = 2和x = -2的点处，曲线y的切线y = 7x ^{3 + 11是平行的。}

解决方案：

Given curve: y = 7x³ + 11

On differentiating w.r.t. x, we get

dy/dx = 21x²

dy/dx = 21(2)² = 84

The slopes at x – 2 & -2 are the same,

Hence the tangent will be parallel to each other.

问题17.在曲线y = x ³上找到切线的斜率等于该点的y坐标的点。

解决方案：

Given curve: y = x³

On differentiating w.r.t. x, we get

dy/dx = 3x²          -(1)

Now let us assume that the point is (x₁, y₁)

dy/dx = 3x₁² 

Also, slope of tangent at (x1, y1) is equal to y₁.

So, 3x₁² = y₁         -(2)

Also, (x1, y1) lies on y = x³ x3,so

y₁ = x₁³           -(3)

From eq(2) & (3)

3x₁² = x₁³  

3x₁² = x₁³ = 0

x₁²(3 – x₁) = 0 

For x₁ = 0, y₁ = x₁³ = (0)3 = 0

One such point is (0, 0)

For x₁ = 3, y_1. = (3)3 = 27

Second point is (3, 27)

问题18.对于曲线y = 4x ³ – 2x ⁵ ，找到切线穿过原点的所有点。

解决方案：

Given curve: y = 4x³ – 3x⁵

Clearly at x = 0, y = 0, i.e the curve passes through origin. 

Now the tangent also passes through origin. 

Equation of a line passing through origin is y = mx.

Now tangent is touching the curve, so 

y = mx will satisfy in curve.

mx = 4x³ – 2x⁵         -(1)

Now dy/dx = 12x² – 10x⁴

Also m is the slope of tangent, so

m = 12x² – 10x⁴          -(2)

From eq(1) & (2),

(12x² – 10x⁴)x = 4x³ – 3x⁵

x³(12 – 10x²) = x³(4 – 2x²)          -(3)

For the first point, x = 0

For x₁ = 0, y₁ = 4x₁³ – 2x₁⁵ = 0 

So, (0, 0) is one such point

Now for other roots of 3

12 – 10x² = 4 – 2x²

8 = 8x²

x² = 1

x = ±1

For x₂ = 1, y₂ = 4x₂³ – 2x₂⁵ = 4(1)³ – 2(1)⁵ = 2 

For x₃ = -1,  y₃ = 4x₃³ – 2x₃⁵ = 4(-1)³ – 2(-1)⁵ = -2   

The other points are(1, 2) & (-1, -2)

问题19.在曲线x ² + y ² – 2x – 3 = 0上找到切线平行于x轴的点。

解决方案：

Given curve: x² + y² – 2x – 3 = 0

On differentiating w.r.t. x, we get

3x + 2y(dy/dx) – 2 – 0 = 0

$x+y\frac{dy}{dx}=1 \frac{dy}{dx}=\frac{1-x}{y}$

Given that the tangent are parallel to x-axis,

So, dy/dx = slope = 0

1 – x/y = 0

For x₁ = 1,

x₁² + y₁² – 2x₁ – 3 = 0      

(1)² + y₁² – 2(1) – 3 = 0      

 y₁² = 4     

y₁² = ≠2

The points are (1, 2) & (1, -2)

问题20：找到曲线ay ² = x ^{3的点(am 2} ，am ³ )的法线方程。

解决方案：

Given curve: ay² = x³

On differentiating w.r.t. x, we get

2ay.dy/dx = 3x²

$\frac{dy}{dx}=\frac{3x^2}{2ay}$ & $\frac{-dx}{dy}=\frac{-2ay}{3x^2}$

$\frac{-dx}{dy}=\frac{-2a.am^3}{3.(am^2)^2}=\frac{-2}{3m}$

So, by point slope form, equation of normal is,

$y-y_1=\frac{-dx}{dy}(x-x_1)$ 

$y-am^3=\frac{-2}{3m}(x-am^2)$

3my – 3am⁴ = -2x + 2am²

Hence, 3my + 2x = 2am² + 3am⁴ is the required equation of normal.

问题21.找到与直线x + 14y + 4 = 0平行的曲线y = x ^{3 + 2x + 6的法线方程。}

解决方案：

Given curve: y = x³ + 2x + 6

On differentiating w.r.t. x, we get

$\frac{dy}{dx}=3x^2+2$

$\frac{-dx}{dy}=\frac{-1}{3x^2+2}$       -(Slope of normal)

Now, the normal are parallel to x + 14y + 4 = 0

$\frac{-1}{3x^2+2}=\frac{-1}{14}$

13 = 3x² + 2            

3x² = 12 ⇒  x² = 4

x = ±2

x₁ = 2 & x₂ = -2

For x₁ = 2; y₁ = $x_1^3+2x_1+6$ = (2)³ + 2(2) + 6 = 18

For x₂ = -2; y₂ = $x_2^2+2x_2+6$ = (-2)³ + 2(-2) + 6 = -6

Normal through (2,18) is 

y – 18 = $\frac{-1}{14}(x-2)$

14y – 252 = -x + 2

14y + x = 254 is one such equation.

Normal through (-2, -6) is 

y + 6 = $\frac{-1}{14}(x+2)$

14y + 84 = -x – 2

14y + x + 86 = 0 is the other equation of normal.

问题22查找切线和法线的方程来抛物线Y ² = 4AX在点(在^图2，2原子)。

解决方案：

Given parabola: y² = 4ax

On differentiating w.r.t. x, we get

$2y.\frac{dy}{dx}=4a$

$\frac{dy}{dx}=\frac{2a}{y} ;\frac{-dx}{dy}=\frac{-y}{2a}$

$\frac{dy}{dx}=\frac{2a}{2at}=\frac{1}{t}$

Now, by point slope form, equation of tangent is,

y – y₁ = $\frac{dy}{dx}(x-x_1)$

$y-2at=\frac{1}{t}(x-at^2)$

ty = x + at² is the equation of tangent to the parabola y² = 4ax at (at², 2at)

Now $\frac{-dx}{dy}=\frac{-2at}{2a}=-t$

Now, by point slope form, equation of normal is,

y – y₁ = $\frac{-dx}{dy}(x-x_1)$

y – 2at = -t(x – at²)

y + xt = 2at + at³ is the equation of normal to the parabola y² = 4ax at (at², 2at)

^{问题23.证明如果8k 2} = 1，曲线x = y ²和xy = k切成直角。

解决方案：

Given curves: x = y² & xy = k

Two curves intersect at right angles f the tangents through their point 

intersection is perpendicular to each other.

Now if tangents are perpendicular their product of their slopes will be equal to -1.

Curve 1: x = y²

1 = 2y.dy/dx

dy/dx = 1/2 y = m₁          -(1)

Curve 2: xy = k

y = k/x

$\frac{-k}{x^2}=m_2$

Let’s find their point of intersection 

x = y² & xy = k

k/y = y² 

y = k^1/3

x = y²      

x = k^2/3

The point is (k^2/3, k^1/3)

m₁ = 1/2k^1/3

For curves to be intersecting each other at right angles,

m₁m₂ = -1

$\frac{1}{2k^\frac{1}{3}}.\frac{-1}{k^\frac{1}{3}}=1$

$2k^\frac{2}{3}=1$

8k² = 1  

Hence proved        

问题24.找到双曲线的切线和法线的方程$\frac{x^2}{a^2}-\frac{y^2}{b^2}=1$在点(x _o ，y _o )。

解决方案：

Given curve: $\frac{x^2}{a^2}-\frac{y^2}{b^2}=1$

On differentiating both sides with respect to x,

$\frac{2x}{a^2}-\frac{2y}{b^2}.\frac{dy}{dx}=0$ 

$\frac{dy}{dx}=\frac{xb^2}{ya^2}\&\frac{-dx}{dy}=\frac{-ya^2}{xb^2}$

Now, $\frac{dy}{dx}=\frac{x_ob^2}{y_0a^2}=m_T$

Equation of tangent by point slope form is,

$y-y_1=m_T(x-x_1)$

$y-y_0=\frac{x_0b^2}{y_0a^2}(x-x_0)$

$\frac{yy_0-y_0^2}{b^2}=\frac{xx_0-x_0^2}{a^2}$

$\frac{yy_0}{b^2}-\frac{xx_0}{a^2}=\frac{y_0^2}{a^2}=-1$      -((x₀,y₀) lie on $\frac{x^2}{a^2}-\frac{b^2}{b^2}=1$)

$\frac{xx_0}{a^2}-\frac{yy_0}{b^2}=1$ is the equation of tangent.

Now, $\frac{-dx}{dy}=\frac{-y_0a^2}{x_0b^2}=m_N$

Equation of normal by point slope form is,

$y-y_1=m_N(x-x_1)$

$y-y_0=\frac{-y_0a^2}{x_0b^2}(x-x_0)$

x₀b²y – x₀b²y₀ = -y₀a²x + y₀a²x₀

x₀b²y + y₀a²x = x₀y₀(a² + b²) is the equation of normal

问题25.求曲线y =的切线的方程。 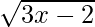$\sqrt{3x-2}$与4x – 2y + 5 = 0线平行。

解决方案：

Given curve: $y=\sqrt{3x-2}$

On differentiating w.r.t. x, we get

$\frac{dy}{dx}=\frac{1}{2\sqrt{3x-2}}.3$

Now, it is given that the tangent is parallel to the line 4x – 2y + 5 = 0, 

so their slopes must be equal.

Slope of 2y = 4x + 5 is 2. 

So,

$\frac{dy}{dx}=\frac{3}{2\sqrt{3x-2}}=2$

$\frac{3}{4}=\sqrt{3x-2}$

9/16 = 3x – 2

x₁ = 41/48

Now, $y_1=\sqrt{3x_1-2}$

$y_1=\sqrt{3.\frac{41}{48}-2}=\frac{3}{4}$

The point is (41/48, 3/4)

Now by point slope form, the equation of tangent will be 

y – y₁ = m(x – x₁)

$y-\frac{3}{4}=2(x-\frac{41}{48})$

$y-\frac{3}{4}=2x-\frac{41}{24}$

24y – 48x + 23 = 0 is the required equation of tangent.

问题26.曲线y的法线y = 2x ² + 3 sin x在x = 0处的斜率是

(A)3(B)1/3(C)-3(D)-1/3

解决方案：

Given curve: y = 2x² + 3 sin x

On differentiating w.r.t. x, we get

dy/dx = 4x + 3cos x

$\frac{-dx}{dy}=\frac{-1}{4x+3\cos x}$               -(Slope of normal)

$\frac{-dx}{dy}=\frac{-1}{4(0)+3\cos 0}=\frac{-1}{3}$

Hence, the correct option is D.

问题27.线y = x +1与曲线y ² = 4x在该点的切线

(A)(1，2)(B)(2，1)(C)(1，-2)(D)(-1，2)

解决方案：

Given curve: y² = 4x

On differentiating w.r.t. x, we get

$2y\frac{dy}{dx}=4$       

$\frac{dy}{dx}=\frac{2}{y}$

y = x + 1 is tangent, slope is 1, so, 2/y = 2

y₁ = 2,

y₁² = 4x₁ 

x₁ = $\frac{2^2}{4}$ 

x₁ = 1

So, (1, 2) is the point.

Hence, the correct option is A.