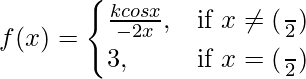第 12 类 RD Sharma 解决方案 - 第 9 章连续性 - 练习 9.1 |设置 2

问题 16. 讨论函数的连续性

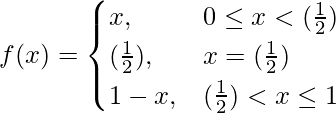$f(x)=\begin{cases}x,&0\leq x<(\frac{1}{2}) \\(\frac{1}{2}),&x=(\frac{1}{2}) \\1-x,&(\frac{1}{2})<x\leq1\end{cases}$在点 x = 1/2。

解决方案：

Given that, 

$f(x)=\begin{cases}x,&0\leq x<(\frac{1}{2}) \\(\frac{1}{2}),&x=(\frac{1}{2}) \\1-x,&(\frac{1}{2})<x\leq1\end{cases}$

So, here we check the continuity of the given f(x) at x = 1/2,

Let us consider LHL,

$\lim_{x\to{\frac{1}{2}}^-}f(x) =\lim_{h\to0}f(\frac{1}{2}-h)$

$=\lim_{h\to0}\frac{1}{2}-h=\frac{1}{2}$

Now, let us consider RHL,

$\lim_{x\to{\frac{1}{2}}^+}f(x) =\lim_{h\to0}f(\frac{1}{2}+h)$

$=\lim_{h\to0}1-(\frac{1}{2}+h)=\frac{1}{2}$

f(1/2) = 1/2

Thus, LHL= RHL = f(1/2) = 1/2

Hence, the f(x) is continuous at x = 1/2. 

问题 17. 讨论的连续性$f(x)=\begin{cases}2x-1,& \text{if }x<0 \\2x+1,& \text{if }x\geq0\end{cases}$在点 x = 0。

解决方案：

Given that, 

$f(x)=\begin{cases}2x-1,& \text{if }x<0 \\2x+1,& \text{if }x\geq0\end{cases}$

So, here we check the continuity of the given f(x) at x = 10,

Let us consider LHL,

$\lim_{x\to0^-}f(x) =\lim_{h\to0}f(0-h)$

$=\lim_{h\to0}2(-h)-1=-1$

Now, let us consider RHL,

$\lim_{x\to0^+}f(x) =\lim_{h\to0}f(0+h)$

$=\lim_{h\to0}2h+1=1$

Thus, LHL ≠ RHL

Hence, the f(x) is discontinuous at x = 0. 

问题 18.函数的 k 值是多少$f(x)=\begin{cases}\frac{x^2-1}{x-1},& x\neq1 \\k,& x=1\end{cases}$在 x = 1 处连续？

解决方案：

Given that, 

$f(x)=\begin{cases}\frac{x^2-1}{x-1},& x\neq1 \\k,& x=1\end{cases}$

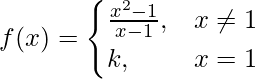
Also, f(x) is continuous at x = 1

So, 

LHL = RHL = f(1)        ……(i)

Let us consider LHL,

$\lim_{x\to1^-}f(x) =\lim_{h\to0}f(1-h)$

$=\lim_{h\to0}\frac{(1-h)^2-1}{(1-h)-1}$

$=\lim_{h\to0}\frac{h^2-2h}{-h}=2$

f(1) = k

From eq(i), we get

LHL = F(1)

Therefore, k = 2

问题 19. 确定常数 k 的值，使得函数

$f(x)=\begin{cases}\frac{x^2-3x+2}{x-1},& \text{if }x\neq1 \\k,& \text{if }x=1\end{cases}$在 x = 1 处连续。

解决方案：

Given that, 

$f(x)=\begin{cases}\frac{x^2-3x+2}{x-1},& \text{if }x\neq1 \\k,& \text{if }x=1\end{cases}$

Also, f(x) is continuous at x = 1

So, LHL = RHL = f(1)        …..(i)

Let us consider LHL,

$\lim_{x\to1^-}f(x) =\lim_{h\to0}f(1-h)$

$=\lim_{h\to0}\frac{(1-h)^2-3(1-h)+2}{(1-h)-1}$

$=\lim_{h\to0}\frac{h^2+h}{-h}$

$=\lim_{h\to0}-h-1=-1$

f(1) = k

From eq(i), we get

LHL = F(1)

Therefore, k = -1

问题 20.函数的 k 值是多少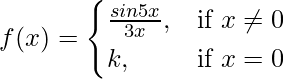$f(x)=\begin{cases}\frac{sin5x}{3x},& \text{if }x\neq0 \\k,& \text{if }x=0\end{cases}$在 x = 0 处连续？

解决方案：

Given that, 

$f(x)=\begin{cases}\frac{sin5x}{3x},& \text{if }x\neq0 \\k,& \text{if }x=0\end{cases}$

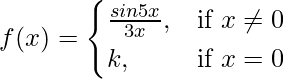
Also, f(x) is continuous at x = 0

So, LHL = RHL = f(0)        …..(i)

Let us consider LHL,

$\lim_{x\to0^-}f(x) =\lim_{h\to0}f(0-h)$ 

$=\lim_{h\to0}\frac{sin5(-h)}{3(-h)}$

$=\lim_{h\to0}\frac{-sin5h}{-3h}$

$=\lim_{h\to0}\frac{sin5h}{3h}\frac{5h}{3h}=\frac{5}{3}$

f(0) = k

Thus, from eq(i), we get

k = 5/3

Therefore, k = 5/3

问题 21. 确定常数 k 的值，使得函数

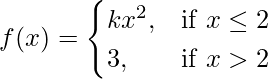$f(x)=\begin{cases}kx^2,& \text{if }x\leq2 \\3,& \text{if }x>2\end{cases}$在 x = 2 处连续。

解决方案：

Given that, 

$f(x)=\begin{cases}kx^2,& \text{if }x\leq2 \\3,& \text{if }x>2\end{cases}$

Also, f(x) is continuous at x = 2

Then, f(2) = k(2)² = 4k

$\lim_{x\to2^-}f(x)=\lim_{x\to2^+}f(x)=f(2)$

⇒ $\lim_{x\to2^-}(kx^2)=\lim_{x\to2^+}(3)=4k$

⇒ k × 2² = 3 = 4k

⇒ 4k = 3 = 4k

⇒ 4k = 3

⇒ k = 3/4

Hence, the value of k is 3/4

问题 22. 确定常数 k 的值，使得函数

$f(x)=\begin{cases}\frac{sin2x}{5x},& \text{if }x\neq0 \\k,& \text{if }x=0\end{cases}$在 x = 0 处是连续的。

解决方案：

Given that, 

$f(x)=\begin{cases}\frac{sin2x}{5x},& \text{if }x\neq0 \\k,& \text{if }x=0\end{cases}$

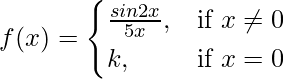
Also, f(x) is continuous at x = 0

So, LHL = RHL = f(0)        ….(i)

Let us consider LHL,

$\lim_{x\to0^-}f(x) =\lim_{h\to0}f(0-h)$

$=\lim_{h\to0}\frac{sin2(-h)}{5(-h)}$

$=\lim_{h\to0}\frac{-sin2h}{-5h}$

$=\lim_{h\to0}\frac{sin2h}{5h}×\frac{2h}{5h}=2/5$

f(0) = k

From eq(i), we get

k = 2/5

问题 23. 求 a 的值，使得函数$f(x)=\begin{cases}ax+5,& \text{if }x\leq2 \\x-1,& \text{if }x>2\end{cases}$在 x = 2 处是连续的。

解决方案：

Given that, 

$f(x)=\begin{cases}ax+5,& \text{if }x\leq2 \\x-1,& \text{if }x>2\end{cases}$

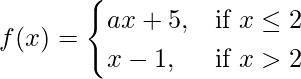
Also, f(x) is continuous at x = 2

So, LHL = RHL = f(2)        …….(i)

Let us consider LHL,

$\lim_{x\to2^-}f(x) =\lim_{h\to0}f(2-h)$

$=\lim_{h\to0}a(2-h)+5$

= 2a + 5

Now, let us consider RHL,

$\lim_{x\to2^+}f(x) =\lim_{h\to0}f(2+h)$

$=\lim_{h\to0}2+h-1=1$

From eq(i), we get

2a + 5 = 1 

⇒ a = -2

问题 24. 证明函数

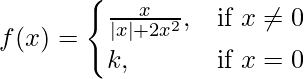$f(x)=\begin{cases}\frac{x}{|x|+2x^2},& \text{if }x\neq0 \\k,& \text{if }x=0\end{cases}$在 x = 0 处保持不连续，无论 k 的选择如何。

解决方案：

Given that, 

$f(x)=\begin{cases}\frac{x}{|x|+2x^2},& \text{if }x\neq0 \\k,& \text{if }x=0\end{cases}$

We have, at x = 0

Let us consider LHL,

$\lim_{x\to0^-}f(x) =\lim_{h\to0}f(0-h)$

$=\lim_{h\to0}\frac{-h}{|-h|+2(-h)^2}$

$=\lim_{h\to0}\frac{-h}{h+2h^2}$

$=\lim_{h\to0}\frac{-1}{1+2h}=-1$

f(0) = k

Now, let us consider RHL,

$\lim_{x\to0^+}f(x) =\lim_{h\to0}f(0+h)$

$=\lim_{h\to0}\frac{h}{|h|+2h^2}$

$=\lim_{h\to0}\frac{1}{1+2h}=1$

Since, LHL ≠ RHL, 

Therefore, f(x) will remain discontinuous at x = 0, regardless the value of k.

问题 25. 如果 f(x) 在 x = π/2 处连续，则求 k 的值，其中

$f(x)=\begin{cases}\frac{kcosx}{π-2x},& \text{if }x\neq(\frac{π}{2}) \\3,& \text{if }x=(\frac{π}{2})\end{cases}$

解决方案：

Given that, 

$f(x)=\begin{cases}\frac{kcosx}{π-2x},& \text{if }x\neq(\frac{π}{2}) \\3,& \text{if }x=(\frac{π}{2})\end{cases}$

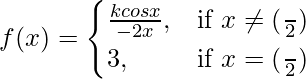
Also, f(x) is continuous at x = π/2

LHL = RHL

⇒ $\lim_{x\to{\frac{π}{2}}^-}f(x)=\lim_{x\to{\frac{π}{2}}^+}f(x)=\lim_{h\to{\frac{π}{2}}}f(x)=f(\frac{π}{2})$

⇒ $\lim_{x\to{\frac{π}{2}}^-}\frac{kcosx}{π-2x}=3$

⇒ $k\lim_{x\to\frac{π}{2}}\frac{sin(\frac{π}{2}-x)}{2(\frac{π}{2}-x)}=3$

⇒ $\frac{k}{2}\lim_{x\to\frac{π}{2}}\frac{sin(\frac{π}{2}-x)}{(\frac{π}{2}-x)}=3$

⇒ k/2 = 3

⇒ k = 6

问题 26. 确定函数对应的 a、b、c 的函数

$f(x)=\begin{cases}\frac{sin(a+1)x+sinx}{x},& \text{if }x<0 \\c,& \text{for }x=0\\\frac{\sqrt{x+bx^2}-√x}{bx^{3/2}},&\text{for }x>0\end{cases}$

在 x = 0 处是连续的。

解决方案：

Given that, 

$f(x)=\begin{cases}\frac{sin(a+1)x+sinx}{x},& \text{if }x<0 \\c,& \text{for }x=0\\\frac{\sqrt{x+bx^2}-√x}{bx^{3/2}},&\text{for }x>0\end{cases}$

Also, f(x) is continuous at x = 0

So, LHL = RHL = f(0)        …..(i)

f(0) = 0

Let us consider LHL,

$\lim_{x\to0^-}f(x) =\lim_{h\to0}f(0-h)$

= $\lim_{h\to0}\frac{sin(a+1)(-h)+sin(-h)}{-h}$

= $\lim_{h\to0}\frac{-sin(ah+h)-sinh}{-h}$      

= $\lim_{h\to0}\frac{sin(a+1)h}{h}+\lim_{h\to0}\frac{sinh}{h}$

= a + 1 + 1 = a + 2

Now, let us consider RHL,

$\lim_{x\to0^+}f(x) =\lim_{h\to0}f(0+h)$

= $\lim_{h\to0}\frac{\sqrt{h+bh^2}-√h}{bh^{3/2}}$

= $\lim_{h\to0}\frac{\sqrt{h+bh^2}-√h}{bh^{3/2}}\frac{\sqrt{h+bh^2}+√h}{\sqrt{h+bh^2}+√h}$

= $\lim_{h\to0}\frac{h+bh^2-h}{bh^{3/2}(\sqrt{h+bh^2}+√h)}$

= $\lim_{h\to0}\frac{bh^2}{bh^2(\sqrt{1+bh}+1)}=1/2$

From eq(i), we get

a + 2 = 1/2 ⇒ a = -3/2

c = 1/2 and b ∈ R -{0}

Hence, a = -3/2, b ∈ R -{0}, c =1/2

问题 27. 如果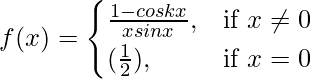$f(x)=\begin{cases}\frac{1-coskx}{xsinx},& \text{if }x\neq0 \\(\frac{1}{2}),& \text{if }x=0\end{cases}$在 x = 0 处连续，求 k。

解决方案：

Given that, 

$f(x)=\begin{cases}\frac{1-coskx}{xsinx},& \text{if }x\neq0 \\(\frac{1}{2}),& \text{if }x=0\end{cases}$

Also, f(x) is continuous at x = 0

So, LHL = RHL = f(0)       …….(i)

f(0) = 1/2

Let us consider LHL,

$\lim_{x\to0^-}f(x) =\lim_{h\to0}f(0-h)$

$=\lim_{h\to0}\frac{1-cosk(-h)}{-hsin(-h)}$

$=\lim_{h\to0}\frac{1-coskh}{hsinh}$

$=\lim_{h\to0}\frac{2sin^2(\frac{kh}{2})}{h.2sin(\frac{h}{2}).cos(\frac{h}{2})}$

$=\lim_{h\to0}(\frac{sin(\frac{kh}{2})}{\frac{kh}{2}})^2\frac{\frac{k^2h^2}{4}}{\frac{sin(\frac{h}{2})}{\frac{h}{2}}(\frac{h}{2})}.(\frac{1}{h})$

$=\lim_{h\to0}(\frac{sin(\frac{kh}{2})}{\frac{kh}{2}})^2\frac{\frac{k^2}{4}}{\frac{sin(\frac{h}{2})}{\frac{h}{2}}(\frac{1}{2})}$

= k²/2

Using eq(i) we get,

k²/2 = 1/2 ⇒ k = ±1

问题 28. 如果$f(x)=\begin{cases}\frac{x-4}{|x-4|},& \text{if }x<4 \\a+b,& \text{if }x=4\\\frac{x-4}{|x-4|}+b& \text{if }x>4\end{cases}$在 x = 4 处连续，求 a，b。

解决方案：

Given that, 

$f(x)=\begin{cases}\frac{x-4}{|x-4|},& \text{if }x<4 \\a+b,& \text{if }x=4\\\frac{x-4}{|x-4|}+b& \text{if }x>4\end{cases}$

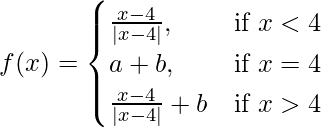
Also, f(x) is continuous at x = 4

So, LHL = RHL = f(4)        ……(i)

f(4) = a + b     …..(ii)

Let us consider LHL,

$\lim_{x\to4^-}f(x) =\lim_{h\to0}f(4-h)$

$=\lim_{h\to0}\frac{(4-h)-4}{|(4-h)-4|}+a$

$=\lim_{h\to0}\frac{-h}{h}+a$

= a – 1        ……(iii)

Now, let us consider RHL,

$\lim_{x\to4^+}f(x) =\lim_{h\to0}f(4+h)$

$=\lim_{h\to0}\frac{(4+h)-4}{|(4+h)-4|}+b$

$=\lim_{h\to0}\frac{h}{h}+b$

= b + 1           ……(iv)

From eq(i), we get

a – 1 = b + 1 ⇒ a – b = 2         …..(v)

From eq(ii) and eq(iii), we get

a + b = a – 1 ⇒ a – b = -1

From eq(ii) and (iv), we get

a + b = b + 1 ⇒ a = 1

Thus, a = 1 and b = -1

问题 29.函数的 k 值是多少

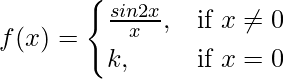$f(x)=\begin{cases}\frac{sin2x}{x},& \text{if }x\neq0 \\k,& \text{if }x=0\end{cases}$在 x = 0 处连续？

解决方案：

Given that, 

$f(x)=\begin{cases}\frac{sin2x}{x},& \text{if }x\neq0 \\k,& \text{if }x=0\end{cases}$

Also, f(x) is continuous at x = 0

So, LHL = RHL = f(0)        …..(i)

f(0) = k

Let us consider LHL,

$\lim_{x\to0^-}f(x) =\lim_{h\to0}f(0-h)$

$=\lim_{h\to0}\frac{sin2(0-h)}{-h}$

$=\lim_{h\to0}\frac{-sin2h}{-h}=2$

Using eq(i), we get 

k = 2

问题 30. 让 f(x) = 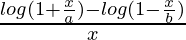$\frac{log(1+\frac{x}{a})-log(1-\frac{x}{b})}{x}$ , x ≠ 0。求 f 在 x = 0 处的值，使 f 在 x = 0 处变得连续。

解决方案：

Given that,

f(x) = $\frac{log(1+\frac{x}{a})-log(1-\frac{x}{b})}{x}$

Also, f(x) is continuous at x = 0

So, LHL=RHL=f(0)        ….(i)

Let us consider LHL,

$\lim_{x\to0^-}f(x) =\lim_{h\to0}f(0-h)$

$=\lim_{h\to0}\frac{log(1-\frac{h}{a})-log(1+\frac{h}{b})}{-h}$

$=\lim_{h\to0}\frac{log(1+(\frac{-h}{a}))}{(\frac{-h}{a})a}+\frac{log(1+\frac{h}{b})}{h}$

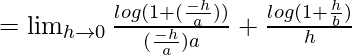
= 1/a + 1/b = (a + b)/ab

From eq(i), we get

f(0) = (a + b)/ab