第 11 课 RD Sharma 解决方案 - 第 28 章 3D 坐标几何简介 - 练习 28.3

问题 1. 三角形的顶点是 A(5, 4, 6), B(1, -1, 3) 和 C(4, 3, 2)。角 A 的内平分线在 D 处与 BC 相交。求 D 的坐标和长度 AD。

解决方案：

We know that the angle bisector divides opposite side in ratio of other two sides 

⇒D divides BC in ratio of AB:AC

A(5, 4, 6), B(1, -1, 3) and C(4, 3, 2)

$AB=\sqrt{16+25+9}=\sqrt{50}=5\sqrt{2}\\ AC=\sqrt{1+1+16}=\sqrt{18}=3\sqrt{2}$

AB:AC=5:3=m:n

$D(x,y)=(\frac{mx_2+mx_1}{m+n},\frac{my_2+my_1}{m+n},\frac{mz_2+zn_1}{m+n})$

Substitute values for m:n=5:3

(x₁,y₁,z₁)=(1,-1,3)

(x₂,y₂,z₂)=(4,3,2)

$D=(\frac{22}{8},\frac{3}{2},\frac{19}{8})$

问题 2. z 坐标为 8 的点 C 位于连接点 A(2, -3, 4) 和 B(8, 0, 10) 的线段上。找到它的坐标。

解决方案：

Z-coordinate 8

A(2, -3, 4) and B(8, 0, 10)

DR’s of AB=(6,3,6)

DR’s of BC=(x-8,y-0,8-10)

Given A,B,C lie on same line 

So values of DR’s should be proportional

$\frac{x-8}{6}=\frac{y}{3}=\frac{8-10}{6}$  

So x=6, y=-1

point is (6,-1,8)

问题 3. 证明三个点 A(2, 3, 4), B(-1, 2, -3) 和 C(-4, 1, -10) 共线，求 C 除以 AB 的比值。

解决方案：

If points are collinear then all points lie on same line

and DR’s should be proportional 

A(2, 3, 4), B(-1, 2, -3) and C(-4, 1, -10)

DR’s of AB=(3,1,7)

DR’s of BC=(3,1,7)

So A,B,C are collinear 

Length of AC$=\sqrt{36+4+196}=\sqrt{236}$

Length of AB$=\sqrt{9+1+49}=\sqrt{59}$

Ratio is AC:AB=2:1

So C divides AB in ratio 2:1 externally

问题 4. 求连接 (2, 4, 5) 和 (3, 5, 4) 的线除以 yz 平面的比率。

解决方案：

yz plane means x=0

Given (2,4,5) and (3,5,4)

Assume ratio to be m:n

Lets equate x term

$0=\frac{3m+2n}{m+n}$

3m=-2n

m:n=-2:3

Which means yz plane divides the line in 2:3 ratio externally.

问题 5. 求连接点 (2, -1, 3) 和 (-1, 2, 1) 的线段除以平面 x+ y + z = 5 的比率。

解决方案：

(2, -1, 3) and (-1, 2, 1)

x+y+z=5

Assume plane divides line in ratio λ:1

so point P which is dividing line in λ:1 ratio is

$P=(\frac{-λ+2}{λ+1},\frac{2λ-1}{λ+1},\frac{λ+3}{λ+1})$

P lies on plane  x+y+z=5

-λ+2+2λ-1+λ+3=5λ5

3λ=-1⇒λ=-1:3

问题 6. 如果点 A(3, 2, -4), B(9, 8, -10) 和 C(5, 4, -6) 共线，求 C 除以 AB 的比率。

解决方案：

A(3, 2, -4), B(9, 8, -10) and C(5, 4, -6)

$AC=\sqrt{4+4+4}=2\sqrt{3}\\ AB=\sqrt{36+36+36}=6\sqrt{3}\\ BC=\sqrt{16+16+16}=4\sqrt{3}$

AC:BC=1:2

问题 7. 三角形 ABC 边的中点由 (-2, 3, 5)、(4, -1, 7) 和 (6, 5, 3) 给出。求 A、B 和 C 的坐标。

解决方案：

Given midpoints (-2, 3, 5), (4, -1, 7) and (6, 5, 3)

Assume D is midpoint of AB, E is midpoint  of BC

F is midpoint of CA

A(x₁,y₁,z₁) B(x₂,y₂,z₂) C(x₃,y₃,z₃)

From midpoint formula, we get following equations 

x₁+x₂=-4;   x₂+x₃=8;   x₃+x₁=12

y₁+y₂=6;    y₂+y₃=-2;  y₃+y₁=10

z₁+z₂=10;  z₂+z₃=14;  z₃+z₁=6

Solving above set of equations we get

A=(0,9,1)

B=(-4,-3,9)

C=(12,1,5)

问题 8. A(1, 2, 3), B(0, 4, 1), C(-1, -1, -3) 是三角形 ABC 的顶点。求角 ∠BAC 的平分线与 BC 相交的点。

解决方案：

A(1, 2, 3), B(0, 4, 1), C(-1, -1, -3)

Angle bisector at A divides  BC in ratio of AB:AC

$AB=\sqrt{1+4+4}=3 AC=\sqrt{4+9+36}=7$

Assume D divides  BC

so $D=(\frac{-3}{10},\frac{25}{10},\frac{-2}{10})$

问题 9. 求球体 x2+y2 +z2 = 504 与连接点 (12, -4, 8) 和 (27, -9, 18) 的直线的比值。

解决方案：

(12, -4, 8) and (27, -9, 18)

Assume point P is dividing line λ:1 ratio, we get

$P=(\frac{27λ+12}{λ+1},\frac{-9λ-4}{λ+1},\frac{8λ+8}{λ+1})$

P lies on sphere, so substitute in sphere equation

x²+y²+z²=504

9(λ+4)²+(9λ+4)²+4(9λ+4)²=504(λ+1)²

729λ²+81λ²+324λ²+648λ+72λ+288λ+144+16+64=504λ²+1008λ+504

(1134-504)λ²+(1008-1008)λ+224-504=0

630λ2=280

λ2=4/9

λ=2:3

问题 10. 证明平面 ax + by + cz + d = 0 以比率划分连接点 (x ₁ ,y ₁ ,z ₁ ) 和 (x ₂ ,y ₂ ,z ₂ ) 的线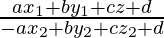$\frac{ax_1+by_1+cz+d}{-ax_2+by_2+cz_2+d}$

解决方案：

Assume ratio is λ:1

Plane is ax+by+cz+d=0

points (x₁,y₁,z₁) and  (x₂,y₂,z₂)

Assume point of intersection of line and plane is D

$D=(\frac{λx_2+x_1}{λ+1},\frac{λy_2+y_1}{λ+1},\frac{λz_2+z_1}{λ+1})$

As D lies on plane, substitute D in plane equation, we get

λ(ax₂+by₂+cz₂+d)+ax₁+by₁+cz₁+d=0

 ⇒$λ=-\frac{ax_1+by_1+cz_1+d}{ax_2+by_2+cz_2+d}$

问题 11. 求三角形的质心，其边为 (1, 2, -3)、(3, 0, 1) 和 (-1, 1, -4) 的中点。

解决方案：

(1, 2, -3), (3, 0, 1) and (-1, 1, -4)

Centroid of triangle is given by

$(\frac{x_1+x_2+x_3}{3},\frac{y_1+y_2+y_3}{3},\frac{z_1+z_2+z_3}{3})$

We know that 

x₁+x₂=2

x₂+x₃=6

x₁+x₃=-2

Adding all gives ⇒2(x₁+x₂+x₃)=6

so x₁+x₂+x₃=3

Similarly, y₁+y₂+y₃=3,  z₁+z₂+z₃=-6

centroid =(1,1,-2)

问题 12. 三角形 ABC 的质心在点 (1, 1, 1)。如果A和B的坐标分别是(3, -5, 7)和(-1, 7, -6)，求C点的坐标。

解决方案：

Given centroid (1,1,1)

A(3,-5,7) and B(-1,7,-6)

Equating terms, we get

$1=\frac{3-1+x_3}{3}\\ 1=\frac{-5+7+y_3}{3}\\ 1=\frac{7-6+z_3}{3}$

(x₃,y₃,z₃)=(1,1,2)

问题 13. 求三等分连接点 P(4, 2, -6) 和 Q(10, -16, 6) 的线段的点的坐标。

解决方案：

Trisection points are those which divide line in ratio 1:2 or 2:1

P(4, 2, -6) and Q(10, -16, 6)

Consider 1:2 case, we get

$(\frac{10+8}{3},\frac{-16+4}{3},\frac{6-12}{3})=(6,-4,-2)$

consider 2:! case, we get

$(\frac{20+4}{3},\frac{-32+2}{3},\frac{12-6}{3})=(8,-10,2)$

(6,-4,-2) and (8,-10,2) are trisection  points

问题 14. 使用截面公式，证明点 A(2, -3, 4), B(-1, 2, 1) 和 C(0, 1/3, 2) 共线。

解决方案：

A(2, -3, 4), B(-1, 2, 1) and C(0, 1/3, 2)

DR’s of BC are $(-1,\frac{5}{3},-1)$

DR’s of AC are$(2,\frac{-10}{3},2)$

Its clear that all DR’s are proportional

问题 15. 假设 P(3, 2, -4), Q(5, 4, -6) 和 R(9, 8, -10) 是共线的。求 Q 除以 PR 的比率。

解决方案：

 P(3, 2, -4), Q(5, 4, -6) and R(9, 8, -10)

$PQ=\sqrt{4+4+4}=2\sqrt{3}\\ QR=\sqrt{16+16+16}=4\sqrt{3}$

PQ:QR=1:2

问题 16. 求连接点 (4, 8, 10) 和 (6, 10, -8) 的线段除以 yz 平面的比率。

解决方案：

(4, 8, 10) and (6, 10, -8) is divided by the yz-plane

Equation of yz-plane is x=0

Assume ratio is m:n

Equating x-term, we get

$0=\frac{6m+4n}{m+n}$ 

m:n=-2:3

So, XY plane divides the line segment in ratio 2:3 externally.