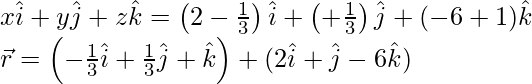问题11.找到线的方向余弦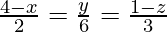 。另外,将其简化为矢量形式
。另外,将其简化为矢量形式
解决方案:
Given:
![]()
![]()
x = -2λ + 4, y = 6λ, z = -3λ + 1
So,
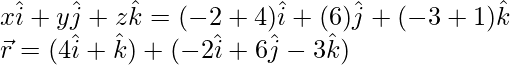
Direction ratios of the line are = -2, 6, -3
Direction cosines of the lines are,
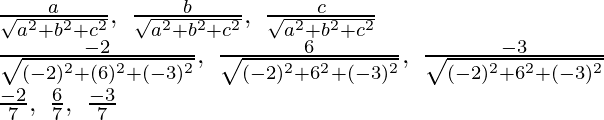
问题12.线的笛卡尔方程为x = ay + b,z = cy + d。找到其方向比率,并将其简化为矢量形式。
解决方案:
x = ay + b
z = cy + d
![]()
So, DR’s of line are (a, 1, c)
From above equation, we can write
x = aλ + b
y = λ
z = cλ + d
So vector equation of line is
![]()
问题13.找到通过具有位置矢量的点的直线的矢量方程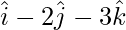 并平行于将点与位置向量连接在一起的线
并平行于将点与位置向量连接在一起的线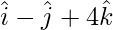 和
和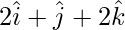 。同样,找到该方程式的笛卡尔等效项。
。同样,找到该方程式的笛卡尔等效项。
解决方案:
We know that, equation of a line passing through![]() and parallel to vector
and parallel to vector![]() is
is
![]() ……. (i)
……. (i)
Here,
![]()
and, \vec{b} = line joining![]() and
and![]()
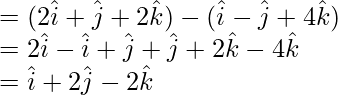
Equation of the line is
![]()
For Cartesian form of equation put![]()
![]()
Equating coefficients of![]()
x = 1 + λ, y = -2 + 2λ, z = -3 – 2λ
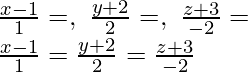
问题14.找到线上的点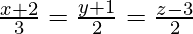 与点P(1、3、3)的距离为5个单位。
与点P(1、3、3)的距离为5个单位。
解决方案:
Given, line is![]()
General points Q on line is (3λ – 2, 2λ -1), 2λ + 3)
Distance of points P from Q =![]()
PQ =![]()
(5)2 = (3λ -3)2 + (2λ – 4) + (2λ)2
25 = 9λ2 + 9 – 18λ + 4λ2 + 16 – 16λ + 4λ2
17λ2 – 34λ = 0
17λ (λ – 2) = 0
λ = 0 or 2
So, points on the line are (3(0) – 2, 2(0) – 1, 2(0) + 3)
(3(2) – 2, 2(2) – 1, 2(2) + 3)
= (-2, -1, 3), (4, 3, 7)
问题15:证明点的位置向量是 和
和 是共线的。
是共线的。
解决方案:
Let the given points are A,B.C with position vectors![]() respectively.
respectively.
![]()
We know that, equation of a line passing through![]() and
and![]() are
are
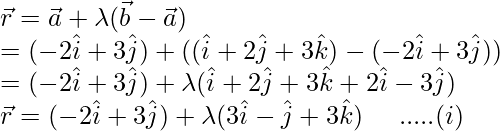
If A, B, C are collinear then![]() must satisfy equation (i)
must satisfy equation (i)
![]()
Equation the coefficients of![]()
-2 + 3 = 7 , λ = 3
3 – λ = 0 , λ = 3
3λ = -1 , λ =![]()
Since, value of λ are not equal, so,
Given points are collinear.
问题16.找到通过点(1、2、3)并与该线平行的线的笛卡尔方程和矢量方程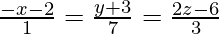
解决方案:
We know that, equation of a line passing through a point (x1, y1, z1) and having direction ratios proportional to a, b, c is
![]()
Here,
(x1, y1, z1) = (1, 2, 3) and
Given line![]()
![]()
Its parallel to the required line, so
a = μ , b = 7μ, c =![]() μ
μ
So, equation of required line using equation (i) is,
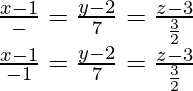
Multiplying the denominators by 2
![]()
x = -2λ + 1, y = 14λ + 2, z = 3λ + 3
So, vector form of the equation of required line,
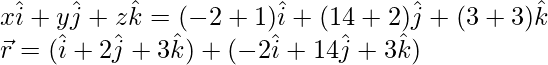
问题17.直线的笛卡尔方程为3x + 1 = 6y – 2 = 1 – z。找到它穿过的固定点,其方向比率以及其矢量方程。
解决方案:
Given equation of line is,
3x + 1 = 6y -2 = 1 – z
Dividing all by 6
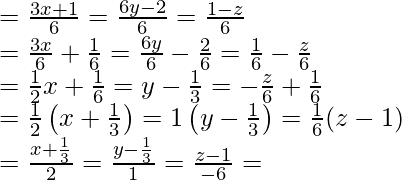
Comparing it with equation of line equation of line passing through (x,1 y1, z1) and the direction ratios a, b, c,
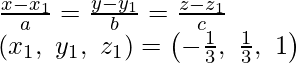
a = 2, b = 1, -6
So, direction ratios of the line are -2, 1, -6
From equation (i)
![]()
So, vector equation of the given line is,