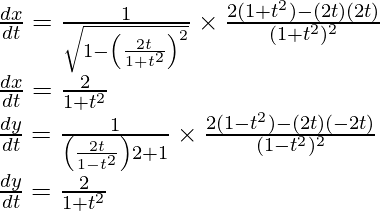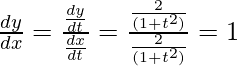第 12 类 RD Sharma 解决方案 - 第 11 章微分 - 练习 11.7 |设置 3
问题 21. 如果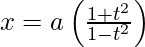 和
和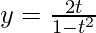 , 找
, 找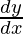
解决方案:
Here,
![]()
Differentiate it with respect to t using chain rule,
![Rendered by QuickLaTeX.com \frac{dx}{dt}=a\left[\frac{(1+t^2)\frac{d}{dt}(1+t^2)-(1+t^2)\frac{d}{dt}(1-t^2)}{(1-t^2)^2}\right]\\ =a\left[\frac{(1-t^2)(2t)-(1+t^2)(-2t)}{(1-t^2)^2}\right]\\ =a\left[\frac{2t-2t^2+2t+2t^3}{(1-t^2)^2}\right]\\ \frac{dy}{dt}=\frac{4at}{(1-t^2)^2}\ \ \ \ \ ......(1)](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_11_Differentiation_%E2%80%93_Exercise_11.7_%7C_Set_3_4.jpg)
And,
![]()
Differentiate it with respect to t using quotient rule,
![Rendered by QuickLaTeX.com \frac{dy}{dt}=a\left[\frac{(1-t^2)\frac{d}{dt}(t)-(t)\frac{d}{dt}(1-t^2)}{(1-t^2)^2}\right]\\ =a\left[\frac{(1-t^2)(1)-(t)(-2t)}{(1-t^2)^2}\right]\\ =a\left[\frac{1-t^2+2t}{(1-t^2)^2}\right]\\ \frac{dy}{dt}=\frac{2(1+t^2)}{(1-t^2)}\ \ \ \ \ ......(2)](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_11_Differentiation_%E2%80%93_Exercise_11.7_%7C_Set_3_6.jpg)
问题 22. 查找 , 如果 y = 12(1 – cos t), x = 10(t – sin t),
, 如果 y = 12(1 – cos t), x = 10(t – sin t), 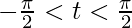
解决方案:
It is given that,
y = 12(1 – cos t),
x = 10(t – sin t)
Therefore,
![Rendered by QuickLaTeX.com \frac{dx}{dt}=\frac{d}{dt}[10(t-sin\ t)]\\ =10.\frac{d}{dt}(t-sin\ t)\\ =10(1- cos\ t)](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_11_Differentiation_%E2%80%93_Exercise_11.7_%7C_Set_3_9.jpg)
![Rendered by QuickLaTeX.com \frac{dy}{dt}=\frac{d}{dt}[12(t-cos\ t)]\\ =12.\frac{d}{dt}(1-cos\ t)\\ =12(0- (-sin\ t)\\ =12sin\ t](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_11_Differentiation_%E2%80%93_Exercise_11.7_%7C_Set_3_10.jpg)
Therefore,
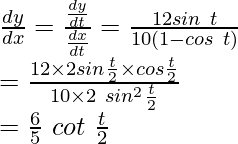
问题 23. 如果 x = a( θ – sin θ ) 且 y = a(1 – cos θ),求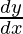 ,在 θ =
,在 θ = 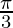
解决方案:
Here,
x = a(θ – sin θ)
and
y = a(1 – cos θ)
Then,
![Rendered by QuickLaTeX.com \frac{dx}{dθ}=\frac{d}{dθ}[a(θ-sin\ θ]\\ =a(1-cos\ θ)](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_11_Differentiation_%E2%80%93_Exercise_11.7_%7C_Set_3_14.jpg)
![Rendered by QuickLaTeX.com \frac{dy}{dθ}=\frac{d}{dθ}[a(1+cos\ θ]\\ =a(-sin\ θ)](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_11_Differentiation_%E2%80%93_Exercise_11.7_%7C_Set_3_15.jpg)
Therefore,
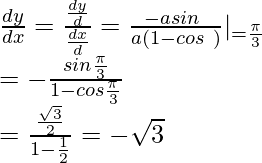
问题 24. 如果 x = a sin 2t (1 + cos 2t) 并且 y = b cos 2t (1 – cos 2t),证明在 t = 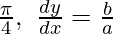
解决方案:
Consider the given functions,
x = a sin 2t (1 + cos 2t)
and
y = b cos 2t (1 – cos 2t)
Write again the functions,
x = a sin 2t + ![]() sin 4t
sin 4t
Differentiate the above function with respect to t,
![]()
y = b cos 2t (1 – cos 2t)
y = b cos 2t – b cos2 2t
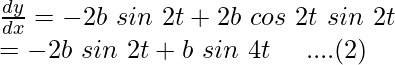
From equation (1) and (2)
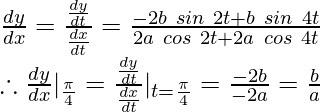
问题 25. 如果 x = cos t (3 – 2cos 2 t) 和 y = sin t (3 – 2 sin 2 t),求 在 t =
在 t = 
解决方案:
Here, the given function:
x = cos t (3 – 2cos2t)
x = cos t – 2cos3t
![]()
y = sin t (3 – 2 sin2t)
y = 3cos t – 2sin3t
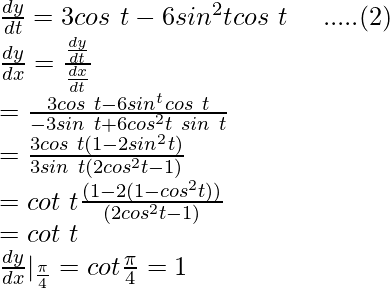
问题 26. 如果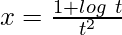 ,
, 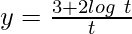 找
找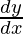
解决方案:
Here,
![]()
and
![]()
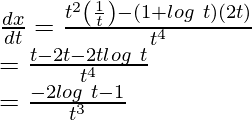
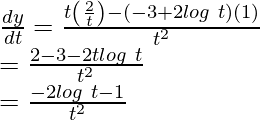
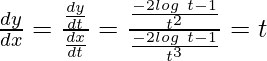
问题 27. 如果 x = 3sin t – sin3t, y = 3cos t – cos3t,求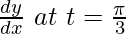
解决方案:
x = 3sin t – sin3t
and,
y = 3cos t – cos3t
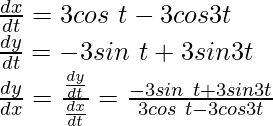
When, ![]()
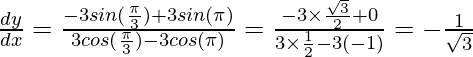
问题 28. 如果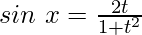 ,
,  找
找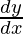
解决方案:
![]()
and,
![]()
![]()
and
![]()