10类NCERT解决方案–第3章两个变量的线性方程对–练习3.5

问题1.以下哪对线性方程组具有唯一解，无解或无穷多个解。如果有独特的解决方案，请使用交叉乘法方法找到它。

(i)x – 3y – 3 = 0，3x – 9y – 2 = 0

解决方案：

Here, 

a₁ = 1, b₁ = -3, c₁ = -3

a₂ = 3, b₂ = -9, c₂ = -2

So,

$\frac{a_1}{a_2} = \frac{1}{3}$

$\frac{b_1}{b_2} = \frac{-3}{-9} = \frac{1}{3}$

$\frac{c_1}{c_2} = \frac{-3}{-2} = \frac{3}{2}$

As, $\frac{a_1}{a_2} = \frac{b_1}{b_2} ≠ \frac{c_1}{c_2}$

Hence, the given pairs of equations have no solution.

(ii)2x + y = 5，3x + 2y = 8

解决方案：

Rearranging equations, we get

2x + y -5 = 0

3x + 2y -8 = 0

Here,

a₁ = 2, b₁ = 1, c₁ = -5

a₂ = 3, b₂ = 2, c₂ = -8

So,

$\frac{a_1}{a_2} = \frac{2}{3}$

$\frac{b_1}{b_2} = \frac{1}{2}$

As, $\frac{a_1}{a_2} ≠ \frac{b_1}{b_2}$

Hence, the given pairs of equations have unique solution.

For cross multiplication,

$\mathbf{\frac{x}{b_1c_2-b_2c_1} = \frac{y}{c_1a_2-c_2a_1} = \frac{1}{a_1b_2-a_2b_1}}$

$\frac{x}{(1)(-8)-(2)(-5)} = \frac{y}{(-5)(3)-(-8)(2)} = \frac{1}{(2)(2)-(3)(1)}$

$\frac{x}{(-8)+10} = \frac{y}{(-15)+16} = \frac{1}{4-3}$

$\frac{x}{2}$ = y = 1

Hence, 

$\frac{x}{2}$ = 1

x = 2

and, y = 1

Hence, the required solution is x = 2 and y = 1.

(iii)3x – 5y = 20，6x – 10y = 40

解决方案：

Rearranging equations, we get

3x – 5y – 20 = 0

6x – 10y – 40 = 0

Here,

a₁ = 3, b1 = -5, c1 = -20

a₂ = 6, b₂ = -10, c₂ = -40

So,

$\frac{a_1}{a_2} = \frac{3}{6} = \frac{1}{2}$

$\frac{b_1}{b_2} = \frac{-5}{-10} = \frac{1}{2}$

$\frac{c_1}{c_2} = \frac{-20}{-40} = \frac{1}{2}$

As,

$\frac{a_1}{a_2} = \frac{b_1}{b_2} = \frac{c_1}{c_2}$

Hence, the given pairs of equations have infinitely many solutions.

(iv)x – 3y – 7 = 0，3x – 3y – 15 = 0

解决方案：

Here,

a₁ = 1, b1 = -3, c1 = -7

a₂ = 3, b₂ = -3, c₂ = -15

So,

$\frac{a_1}{a_2} = \frac{1}{3}$

$\frac{b_1}{b_2} = \frac{-3}{-3} = 1$

As,

$\frac{a_1}{a_2} ≠ \frac{b_1}{b_2}$

Hence, the given pairs of equations have unique solution.

For cross multiplication,

$\mathbf{\frac{x}{b_1c_2-b_2c_1} = \frac{y}{c_1a_2-c_2a_1} = \frac{1}{a_1b_2-a_2b_1}}$

$\frac{x}{(-3)(-15)-(-3)(-7)} = \frac{y}{(-7)(3)-(-15)(1)} = \frac{1}{(1)(-3)-(3)(-3)}$

$\frac{x}{45-21} = \frac{y}{(-21)+15} = \frac{1}{-3+9}$

$\frac{x}{24} = \frac{y}{-6} = \frac{1}{6}$

Hence,

x = $\frac{24}{6}$

x = 4

and, y = $\frac{-6}{6}$

y = -1

Hence, the required solution is x = 4 and y = -1.

问题2。(i)对于下面的线性方程组，对于a和b的哪个值具有无限数量的解?

2x + 3y = 7

(a – b)x +(a + b)y = 3a + b – 2

解决方案：

Here,

a₁ = 2, b1 = 3, c1 = -7

a₂ = a-b, b₂ = a+b, c₂ = -(3a+b-2)

For having infinite number of solutions, it has to satisfy below conditions:

$\frac{a_1}{a_2} = \frac{b_1}{b_2} = \frac{c_1}{c_2}$

$\frac{2}{a-b} = \frac{3}{a+b} = \frac{7}{(3a+b-2)}$

Now, on comparing

$\frac{2}{a-b} = \frac{3}{a+b}$

2(a+b) = 3(a-b)

2a+2b = 3a – 3b

a – 5b = 0 …………………(1)

And, now on comparing

$\frac{3}{a+b} = \frac{7}{(3a+b-2)}$

3(3a+b-2) = 7(a+b)

9a+3b-6 = 7a+7b

2a-4b-6=0 

Reducing form, we get

a-2b-3=0 …………………(2)

Now, new values for

a₁ = 1, b₁ = -5, c₁ = 0

a₂ = 1, b₂ = -2, c₂ = -3

Solving Eq(1) and Eq(2), by cross multiplication,

$\mathbf{\frac{a}{b_1c_2-b_2c_1} = \frac{b}{c_1a_2-c_2a_1} = \frac{1}{a_1b_2-a_2b_1}}$

$\frac{a}{(-5)(-3)-(-2)(0)} = \frac{b}{(0)(1)-(-3)(1)} = \frac{1}{(1)(-2)-(1)(-5)}$

$\frac{a}{15-0} = \frac{b}{0+3} = \frac{1}{-2+5}$

$\frac{a}{15} = \frac{b}{3} = \frac{1}{3}$

Hence,

a = $\frac{15}{3}$

a = 5

and, b = $\frac{3}{3}$

b = 1

Hence, For values a = 5 and b = 1 pair of linear equations have an infinite number of solutions

(ii)以下对线性方程式对k的哪个值无解?

3x + y = 1

(2k – 1)x +(k – 1)y = 2k + 1

解决方案：

Here,

a₁ = 3, b₁ = 1, c₁ = -1

a₂ = (2k-1), b₂ = k-1, c₂ = -(2k+1)

For having no solution, it has to satisfy below conditions:

$\frac{a_1}{a_2} = \frac{b_1}{b_2} ≠ \frac{c_1}{c_2}$

$\frac{3}{2k-1} = \frac{1}{k-1} ≠ \frac{1}{2k+1}$

Now, on comparing

$\frac{3}{2k-1} = \frac{1}{k-1}$

3(k-1) = 2k-1

3k-3 = 2k-1

k = 2

And, now on comparing

$\frac{1}{k-1} ≠ \frac{1}{2k+1}$

2k+1 ≠ k-1

k ≠ -2

Hence, for k = 2 and k ≠ -2 the pair of linear equations have no solution.

问题3.用替换和交叉乘法解决以下线性方程对：

8x + 5y = 9

3x + 2y = 4

解决方案：

8x + 5y – 9 = 0  …………………(1)

3x + 2y – 4 = 0  …………………(2)

Substitution Method

From Eq(2), we get

x = 4-2y/3

Now, substituting it in Eq(1), we get

8(4-2y/3) + 5y – 9 = 0

32-16y/3 + 5y – 9 = 0

32 – 16y + 15y – 27 = 0

y = 5

Now, substituting y = 5 in Eq(2), we get

3x + 2(5) – 4 = 0

3x = -6

x = -2

Cross Multiplication Method

Here,

a₁ = 8, b₁ = 5, c₁ = -9

a₂ = 3, b₂ = 2, c₂ = -4

So,

$\frac{a_1}{a_2} = \frac{8}{3}$

$\frac{b_1}{b_2} = \frac{5}{2}$

As,

$\frac{a_1}{a_2} ≠ \frac{b_1}{b_2}$

Hence, the given pairs of equations have unique solution.

For cross multiplication,

$\mathbf{\frac{x}{b_1c_2-b_2c_1} = \frac{y}{c_1a_2-c_2a_1} = \frac{1}{a_1b_2-a_2b_1}}$

$\frac{x}{(5)(-4)-(2)(-9)} = \frac{y}{(-9)(3)-(-4)(8)} = \frac{1}{(8)(2)-(3)(5)}$

$\frac{x}{(-20)+18} = \frac{y}{(-27)+32} = \frac{1}{16-15}$

$\frac{x}{-2} = \frac{y}{5}$ = 1

Hence,

$\frac{x}{-2}$ = 1

x = -2

and, $\frac{y}{-5}$ = 1

y = 5

Hence, the required solution is x = -2 and y = 5.

问题4.在以下问题中形成一对线性方程组，并通过任何代数方法找到它们的解(如果存在)：

(i)每月宿舍费用的一部分是固定的，其余部分取决于人们在烂摊子里吃饭的天数。当学生A吃饭20天时，她必须支付₹1000的旅费，而学生B却要吃饭26天，则要支付₹1180的旅费。查找每天的固定费用和食物费用。

解决方案：

Let’s take,

Fixed charge = x

Charge of food per day = y

According to the given question,

x + 20y = 1000 ……………….. (1)

x + 26y = 1180 ………………..(2)

Subtracting Eq(1) from Eq(2) we get

6y = 180

y = 30

Now, substituting y = 30 in Eq(2), we get

x + 20(30) = 1000

x = 1000 – 600

x= 400.

Hence, fixed charges is ₹ 400 and charge per day is ₹ 30.

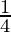(ii)一小部分变成$\frac{1}{3}$当从分子中减去1时，它变成$\frac{1}{4}$分母加上8时。找到分数。

解决方案：

Let the fraction be $\frac{x}{y}$.

So, as per the question given,

$\frac{x-1}{y} = \frac{1}{3}$

3x – y = 3  …………………(1)

$\frac{x}{y+8} = \frac{1}{4}$

4x –y =8  ………………..(2)

Subtracting Eq(1) from Eq(2) , we get

x = 5

Now, substituting x = 5 in Eq(2), we get

4(5)– y = 8

y = 12

Hence, the fraction is $\mathbf{\frac{5}{12}}$.

(iii)Yash在测试中获得40分，每个正确答案获得3分，而每个错误答案则失去1分。如果每个正确答案被授予4分，而每个错误答案被扣除2分，那么Yash将获得50分。测试中有多少个问题?

解决方案：

Let’s take 

Number of right answers = x 

Number of wrong answers = y

According to the given question;

3x−y=40 ……….……..(1)

4x−2y=50

2x−y=25 ……………….(2)

Subtracting Eq(2) from Eq(1), we get

x = 15

Now, substituting x = 15 in Eq(2), we get

2(15) – y = 25

y = 30-25

y = 5

Hence, number of right answers = 15 and number of wrong answers = 5

Hence, total number of questions = 20

(iv)地点A和地点B在高速公路上相距100公里。一辆车同时从A出发，另一辆车从B出发。如果汽车以相同的方向以不同的速度行驶，它们将在5个小时内相遇。如果他们彼此相遇，他们会在1小时内见面。两辆车的速度是多少?

解决方案：

Let’s take

Speed of car from point A = x km/he 

Speed of car from point B = y km/h

When car travels in the same direction,

5x – 5y = 100

x – y = 20 ………………(1)

When car travels in the opposite direction,

x + y = 100  ………………..(2)

Subtracting Eq(1) from Eq(2), we get

2y = 80

y = 40

Now, substituting y = 40 in Eq(1), we get

x – 40 = 20

x = 60

Hence, the speed of car from point A = 60 km/h

Speed of car from point B = 40 km/h.

(v)如果矩形的长度减少5个单位，而宽度增加3个单位，则矩形的面积减少9个平方单位。如果将长度增加3个单位，将宽度增加2个单位，则面积将增加67平方单位。找到矩形的尺寸。

解决方案：

Let’s take

Length of rectangle = x unit

Breadth of the rectangle = y unit

Area of rectangle will be = xy sq. units

According to the given conditions,

(x – 5) (y + 3) = xy -9

3x – 5y – 6 = 0 ……………………(1)

(x + 3) (y + 2) = xy + 67

2x + 3y – 61 = 0 ……………………..(2)

Using cross multiplication method, we get,

$\frac{x}{(-5)(-61)-(3)(-6)} = \frac{y}{(-6)(2)-(-61)(3)} = \frac{1}{(3)(3)-(2)(-5)}$

$\frac{x}{305 +18} = \frac{y}{-12+183} = \frac{1}{9+10}$

$\frac{x}{323} = \frac{y}{171} = \frac{1}{19}$

Hence, 

$\frac{x}{323} = \frac{1}{19}$

x = 17

and, $\frac{y}{171} = \frac{1}{19}$

y = 9

Hence, the required solution is x = 17 and y = 9.

Length of rectangle = 17 units

Breadth of the rectangle = 9 units