第 10 类 RD Sharma 解决方案 - 第 14 章坐标几何 - 练习 14.3 |设置 2

问题 21. 求位于连接 A(-3, 10) 和 B(6, -8) 的线段上的点 P(-1, y) 与其相除的比率。另外，求 y 的值。

解决方案：

Assume P divide A(-3, 10) and B(6, -8) in the ratio of k : 1

Given: coordinates of P as (-1, y)

After applying the section formula for x – coordinate,

We will get

$-1 = \frac{6k - 3}{ k + 1}\\ -(k + 1) = 6k - 3\\ 7k = 2\\ k = \frac{2}{7}$

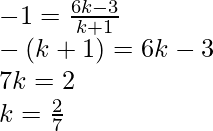
Therefore, 

AB is divided by point P in the ratio of 2 : 7

By applying the value of k, to find the y-coordinate 

We will get

$y = \frac{-8k + 10}{k + 1}\\ y = \left(\frac{-8\times\frac{2}{7} + 10)}{ \frac{2}{7} + 1}\right)$

y = (-16 + 70)/(2 + 7) = 54/9

y = 6 

Hence, 

The y-coordinate of P is 6.

问题 22. 求点 A 的坐标，其中 AB 是圆心为 (2, -3) 且 B 为 (1, 4) 的圆的直径。

解决方案：

Assume the coordinates of point A be (x, y)

Given: AB is the diameter, 

So the center in the mid-point of the diameter

Thus,

(2, -3) = (x + 1/ 2, y + 4/2)

2 = x + 1/2 and -3 = y + 4/2 

4 = x + 1 and -6 = y + 4

x = 3 and y = -10

Hence, the coordinates of A are (3, -10)

问题 23. 如果点 (-2, 1)、(1, 0)、(x, 3) 和 (1, y) 形成平行四边形，求 x 和 y 的值。

解决方案：

Consider A(-2, 1), B(1, 0), C(x , 3) and D(1, y) are the given points of the parallelogram.

As we know that the diagonals of a parallelogram bisect each other.

Thus, 

Coordinates of mid-point of AC = Coordinates of mid-point of BD

((x – 2)/2, (3 – 1)/2) = (1+1)/2, (y + 0)/2 

((x – 2)/2, 1) = (1, y/2) 

(x – 2)/2 = 1

x – 2 = 2

x = 4

and y/2 = 1

y = 2

Hence, the value of x is 4 and the value of y is 2.

问题 24. 点 A(2, 0)、B(9, 1)、C(11, 6) 和 D(4, 4) 是四边形 ABCD 的顶点。判断 ABCD 是否为菱形。

解决方案：

Given: A(2, 0), B(9, 1), C(11, 6) and D(4, 4).

Mid-point of AC coordinates are $(11+\frac{2}{ 2},\ 6+\frac{0}{ 2}) = (\frac{13}{2},\ 3)$

Mid-point of BD coordinates are $(9+\frac{4}{2},\ 1+\frac{4}{2}) = (\frac{13}{2},\ \frac{5}{2})$

Here, 

Coordinates of the mid-point of AC ≠ Coordinates of mid-point of BD, 

ABCD is not a parallelogram.

Hence, 

ABCD cannot be a rhombus too.

问题 25. 点 (-4,6) 分割连接点 A(-6,10) 和 B(3,-8) 的线段的比例是多少？

解决方案：

Assume the line segment AB is divided by point (-4, 6) in the ratio of k : 1.

After applying the Section Formula, 

We will get

$(-4,\ 6)=\left(\frac{3k-6}{k+1},\ \frac{-8k+10}{k+1}\right)\\ -4=\frac{3k-6}{k+1}$

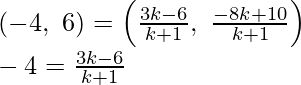
-4k -4 = 3k – 6

7k = 2

k : 1 = 2 : 7

We can also check for the y-coordinate also.

Hence, 

The ratio in which the line segment AB is divided by point (-4,6) is 2 : 7.

问题 26. 求 y 轴分割连接点 (5, -6) 和 (-1, -4) 的线段的比率。另外，求分割点的坐标。

解决方案：

Assume P(5, -6) and Q(-1, -4) be the given points.

Consider the line segment PQ is divided by y-axis in the ratio k : 1.

After applying the Section Formula for the x-coordinate (as it’s zero) 

We will get,

$\frac{-k+5}{k+1}=0$

-k + 5 = 0

k = 5

Therefore, 

The ratio in which the y-axis divides the given 2 points is 5 : 1

Now further, for finding the coordinates of the point of division

On putting k = 5, we will get

$\left(\frac{-5+5}{5+1},\ \frac{-4\times5-6}{5+1}\right)=\left(0,\ \frac{-13}{3}\right)$

Therefore, 

The coordinates of the point of division are (0, -13/3) 

问题 27. 证明 A(-3, 2), B(-5, 5), C(2, -3) 和 D(4, 4) 是菱形的顶点。

解决方案：

Given: A(-3, 2), B(-5, 5), C(2, -3) and D(4, 4)

Further,

Mid-point of AC coordinates are $\left(-3+\frac{2}{ 2},\ 2\ -\ \frac{3}{ 2}\right) = \left(\frac{-1}{2},\ \frac{-1}{2}\right)$

And,

Mid-point of BD coordinates are $\left(-5+\frac{4}{ 2},\ -5+ \frac{4}{ 2}\right) = \left(\frac{-1}{2},\ \frac{-1}{2}\right)$

Therefore, 

The mid-point for both the diagonals are the same. 

Thus, 

ABCD is a parallelogram.

Now, 

For the sides

$AB=\sqrt{(-5+3)^2+(-5-2)^2}\\ AB=\sqrt{4+49}\\ AB=\sqrt{53}\\ BC=\sqrt{(-5-2)^2+(-5+3)^2}\\ BC=\sqrt{49+4}\\ BC=\sqrt{53}$

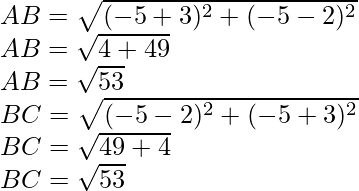
AB = BC

We can see that ABCD is a parallelogram with adjacent sides equal.

Therefore, 

ABCD is a rhombus.

问题 28. 求顶点在 A(0, -1)、B(2, 1) 和 C(0, 3) 的 ΔABC 的中值长度。

解决方案：

Assume AD, BE and CF be the medians of ΔABC

Now,

Coordinates of D are $\left(2+\frac{0}{ 2},\ 1+\frac{3}{2}\right)$ = (1, 2)

Coordinates of E are $\left(\frac{0}{2},\ 3-\frac{1}{ 2}\right)$ = (0, 1)

Coordinates of F are $\left(2+\frac{0}{2},\ 1-\frac{1}{2}\right)$ = (1, 0)

Further,

The length of the medians

Length of the median AD = $\sqrt{(1-0)^2+(2+1)^2}$ = √10 units

Length of the median BE = $\sqrt{(2-0)^2+(1-1)^2}$ = 2 units

Length of the median CF = $\sqrt{(1-0)^2+(0-3)^2}$ = = √10 units

问题 29. 求顶点在 A(5, 1)、B(1, 5) 和 C(-3, -1) 的 ΔABC 的中位数的长度。

解决方案：

Given: Vertices of ΔABC as A(5, 1), B(1, 5) and C(-3, -1).

Consider AD, BE and CF be the medians

Coordinates of D are $\left(1-\frac{3}{ 2},\ 5-\frac{1}{2}\right)$ = (-1, 2)

Coordinates of E are $\left(5-\frac{3}{ 2},\ 1-\frac{1}{2}\right)$ = (1, 0)

Coordinates of F are $\left(5+\frac{1}{ 2},\ 1+\frac{5}{2}\right)$ = (3, 3)

Further, 

The length of the medians

Length of the median AD = $\sqrt{(5+1)^2+(1-2)^2}$ = √37 units

Length of the median BE = $\sqrt{(1-1)^2+(5-0)^2}$ = 5 units

Length of the median CF = $\sqrt{(3+3)^2+(3+1)^2}$ = √52 units

问题 30. 求将连接点 (-4, 0) 和 (0, 6) 的线段分成四等份的点的坐标。

解决方案：

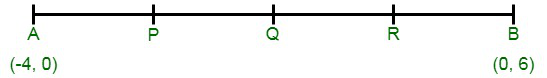
Consider A(-4, 0) and B(0, 6) as they are the given points

And, 

Assume P, Q and R be the points which divide AB is four equal points, as shown in the fig.

Thus, 

As we know that AP : PB = 1 : 3

By applying the Section Formula the coordinates of P are

$\left(\frac{1\times0+3(-4)}{1+3},\ \frac{1\times6+3\times0}{1+3}\right)=\left(-3,\ \frac{3}{2}\right)$

And,

We can see that Q is the mid-point of AB

Thus, the coordinates of Q are

$\left(\frac{-4+0}{2},\ \frac{0+6}{2}\right)=(-2, 3)$

Finally, 

The ratio of AR : BR is 3 : 1

Then, after applying the Section Formula the coordinates of R are

$\left(\frac{3\times0+1\times(-4)}{3+1},\ \frac{3\times6+1\times0}{3+1}\right)=\left(-1,\ \frac{9}{2}\right)$

问题 31. 证明连接点 (5, 7) 和 (3, 9) 的线段的中点也是连接点 (8, 6) 和 (0, 10) 的线段的中点）。

解决方案：

Assume M be the mid-point of AB. Coordinates of the mid-point of this line segment joining two points A (5, 7) and B (3, 9).

$=\left(\frac{x_1+x_2}{2},\ \frac{y_1+y_2}{2}\right)\\ =\left(\frac{5+3}{2},\ \frac{7+9}{2}\right)\\ =\left(\frac{8}{2},\ \frac{16}{2}\right)\\ =(4,\ 8)$

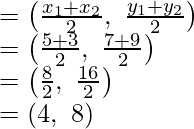
Now coordinates of the mid-point of the line segment joining the points (8, 6) and (0, 10) are;

$=\left(\frac{8+0}{2},\ \frac{6+10}{2}\right)\\ =\left(\frac{8}{2},\ \frac{16}{2}\right)\\ =(4,\ 8)$

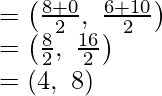
Thus, this is the same as the first case.

问题 32. 求点 (1, 2) 到连接点 (6, 8) 和 (2, 4) 的线段中点的距离。

解决方案：

Assume M be the mid-point of the line segment joining the points (6, 8) and (2, 4)

Now 

Coordinates of M will be

$=\left(\frac{x_1+x_2}{2},\ \frac{y_1+y_2}{2}\right)\\ =\left(\frac{6+3}{2},\ \frac{8+4}{2}\right)\\ =\left(\frac{8}{2},\ \frac{12}{2}\right)\\ =(4,\ 6)$

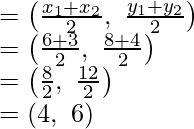
Now, 

Distance between the points (4, 6) and (1, 2)

$=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\\ =\sqrt{(1-4)^2+(2-6)^2}\\ =\sqrt{(-3)^2+(-4)^2}\\ =\sqrt{9+16}\\ =\sqrt{25}$ = 5 units

问题 33. 如果 A 和 B 分别是 (1, 4) 和 (5, 2)，求 P 的坐标时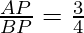$\frac{AP}{BP}=\frac{3}{4}$

解决方案：

Here, Point P divides the line segment joining the points (1, 4) and (5, 2) in the ratio of AP : PB = 3 : 4

Coordinates of P will be

$=\left(\frac{mx_1+nx_2}{m+n},\ \frac{my_1+ny_2}{m+n}\right)\\ =\left(\frac{3\times5+4\times1}{3+4},\ \frac{3\times2+4\times4}{3+4}\right)\\ =\left(\frac{15+4}{7},\ \frac{6+16}{7}\right)\\ =\left(\frac{19}{7},\ \frac{22}{7}\right)$

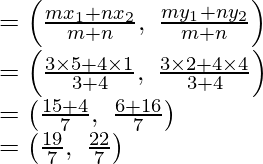
问题 34. 证明点 A (1, 0)、B (5, 3)、C (2, 7)和 D (-2, 4) 是平行四边形的顶点。

解决方案：

If ABCD is a parallelogram, 

Then its diagonal AC and BD will bisect each other at O

Consider O is the mid-point of AC, 

Then coordinates of O will be;

$=\left(\frac{x_1+x_2}{2},\ \frac{y_1+y_2}{2}\right)\\ =\left(\frac{1+2}{2},\ \frac{0+7}{2}\right)\\ =\left(\frac{3}{2},\ \frac{7}{2}\right)$

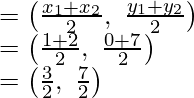
And assume O is the mid-point of BD,

Then coordinates of O will be;

$=\left(\frac{5+(-2)}{2},\ \frac{3+4}{2}\right)\\ =\left(\frac{5-2}{2},\ \frac{3+4}{2}\right)\\ =\left(\frac{3}{2},\ \frac{7}{2}\right)$

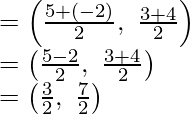
We see that coordinates of the mid-points of AC and BD are same

Therefore, AC and BD  bisect each other at O

Now, length of AC 

$=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\\ =\sqrt{(2-1)^2+(7-0)^2}\\ =\sqrt{1^2+7^2}\\ =\sqrt{1+49}\\ =\sqrt{50}$

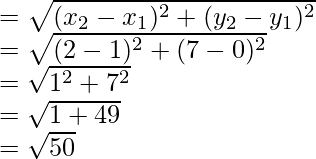
and length of BD = 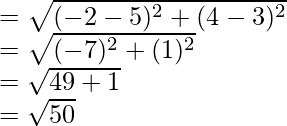$=\sqrt{(-2-5)^2+(4-3)^2}\\ =\sqrt{(-7)^2+(1)^2}\\ =\sqrt{49+1}\\ =\sqrt{50}$

We can see that AC = BD

Therefore, ABCD is a rectangle.

问题 35. 确定点 P (m, 6) 与 A (-4, 3) 和 B (2, 8) 的连接的比值。另外，求 m 的值。

解决方案：

Assume the ratio be r : s in which P (m, 6) divides the line segment joining the points A (-4, 3) and B (2, 8)

Therefore,

$m=\frac{rx_2+sx_1}{r+s}$

and $6=\frac{ry_2+sy_1}{r+s}\\ =\frac{r\times8+s\times3}{r+s}\\ =\frac{8r+3s}{r+s}=6$

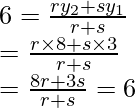
⇒ 8r + 3s = 6r + 6s

⇒ 8r – 6r = 6s – 3s

⇒ 2r = 3s

$⇒ \frac{r}{s}=\frac{3}{2}$

Therefore,

Ratio is 3 : 2

Now, 

$m=\frac{3\times2+2\times(-4)}{3+2}\\ =\frac{6-8}{5}\\ =\frac{-2}{5}$

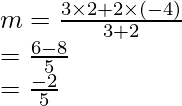
Hence, m = -2/5 

问题 36. 确定点 (-6, a) 与 A (-3, -1) 和 B (-8, 9) 的连接的比值。另外，求 a 的值。

解决方案：

Assume the point P (-6, a) divides the join of A (-3, -1) and B (-8, 9) in the ratio m : n

Therefore,

$-6=\frac{mx_2+nx_1}{m+n}\\ =\frac{m(-8)+n(-3)}{m+n}$

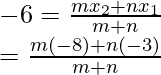
-6 = (-8m -3n)/(m + n)

-6m – 6n = -8m – 3n

8m – 6m = 6n – 3n

2m = 3n

m/n = 3/2

Therefore,

Ratio = 3 : 2

and

$a=\frac{my_2+ny_1}{m+n}=\frac{3\times9+2\times(-1)}{3+2}\\ =\frac{27-2}{5}\\ =\frac{25}{5}\\ =5$

问题 37. ABCD 是由点 A (-1, -1)、B (-1, 4)、C (5, 4)和 D (5, -1) 连接而成的矩形。 P、Q、R和 S 分别是边 AB、BC、CD和 DA的中点。四边形 PQRS 是正方形吗？一个矩形？还是菱形？证明你的答案。

解决方案：

ABCD is a rectangle whose vertices are A (-1,-1), B (-1,4), C (5, 4) and D (5, -1). 

P, Q, R, and S are the mid-points of the sides AB, BC, CD and DA respectively 

And are joined PR and QS are also joined.

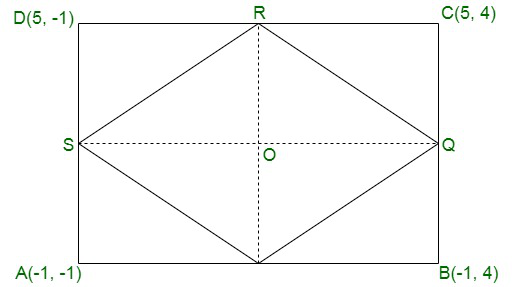
Now coordinates of P will be

$=\left(\frac{x_1+x_2}{2},\ \frac{y_1+y_2}{2}\right)\\ =\left(\frac{-1-1}{2},\ \frac{-1+4}{2}\right)\\ =\left(\frac{-2}{2},\ \frac{3}{2}\right)\\ =(-1,\ \frac{3}{2})$

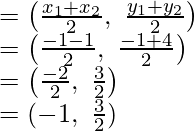
Similarly, the coordinates of Q, will be:

$=\left(\frac{x_1+x_2}{2},\ \frac{y_1+y_2}{2}\right)\\ =\left(\frac{-1+5}{2},\ \frac{4+4}{2}\right)\\ =\left(\frac{4}{2},\ \frac{8}{2}\right)\\ =(2,\ 4)$

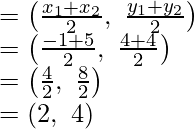
Coordinates of R will be:

$=\left(\frac{x_1+x_2}{2},\ \frac{y_1+y_2}{2}\right)\\ =\left(\frac{5+5}{2},\ \frac{4-1}{2}\right)\\ =\left(\frac{10}{2},\ \frac{3}{2}\right)\\ =(5,\ \frac{3}{2})$

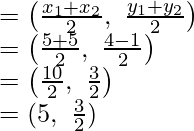
Coordinates of S will be:

$=\left(\frac{x_1+x_2}{2},\ \frac{y_1+y_2}{2}\right)\\ =\left(\frac{5-1}{2},\ \frac{-1-1}{2}\right)\\ =\left(\frac{4}{2},\ \frac{-2}{2}\right)\\ =(2,\ -1)$

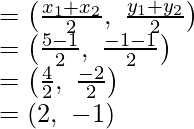
Coordinates of P (-1, 3/2), Q (2, 4), R(5, 3/2) and S (2, -1)

$PQ=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\\ =\sqrt{(2+1)^2+(4-\frac{3}{2})^2}\\ =\sqrt{(3)^2+(\frac{5}{3})^2}\\ =\sqrt{9+\frac{25}{4}}\\ =\sqrt{\frac{36+25}{4}}\\ =\sqrt{\frac{61}{4}}\\ =\frac{\sqrt{61}}{2}$

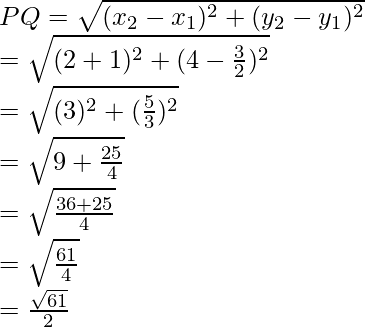
Now, assume the diagonals PQ and QS intersect each other at O

Assume O is the mid-point of PR,

Then coordinates of O will be

$\left(\frac{-1+5}{2},\ \left(\frac{3}{2}+\frac{3}{2}\right)\frac{1}{2}\right)\\ =\left(\frac{4}{2},\ \frac{3}{2}\right)\\ =\left(2,\ \frac{3}{2}\right)$

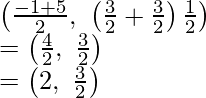
Similarly, of O is the mid-point of QS, then the coordinates of O will be

$\left(\frac{2+2}{2},\ \frac{4+(-1)}{2}\right)\\ =\left(\frac{4}{2},\ \frac{3}{2}\right)\\ =\left(2,\ \frac{3}{2}\right)$

Now, we see that the coordinates of O in both case is same and adjacent sides are also equal

Then it may be a square or a rhombus

Now length of PR = 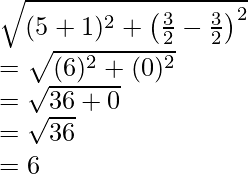$\sqrt{(5+1)^2+\left(\frac{3}{2}-\frac{3}{2}\right)^2}\\ =\sqrt{(6)^2+(0)^2}\\ =\sqrt{36+0}\\ =\sqrt{36}\\ =6$

And length of OS

$\sqrt{(2-2)^2+(-1-4)^2}\\ =\sqrt{(0)^2+(-5)^2}\\ =\sqrt{0+25}\\ =\sqrt{25}\\ =5$

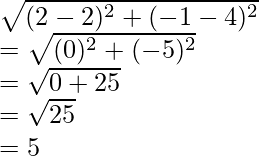
Because diagonal are not equal

Hence, PQRS is a rhombus.

问题 38. 点 P、Q、R和 S 将连接点 A (1, 2) 和 B (6, 7) 的线段分成 5 个相等的部分。求点 P、Q和 R的坐标。

解决方案：

Points P, Q, R and S divides AB in 5 equal parts and assume coordinates of P, Q, R and S are,

(x₁, y₁), (x₂, y₂), (x₃, y₃), (x₄, y₄) 

$x=\frac{m_1x_2+m_2x_1}{m_1+m_2}$

⇒ P divides AB in ratio 1 : 4

Therefore,

$x_1=\frac{1\times6+4\times1}{1+4}\\ =\frac{6+4}{5}\\ =\frac{10}{5}\\ =2\\ y_1=\frac{1\times7+4\times2}{1+4}\\ =\frac{7+8}{5}\\ =\frac{15}{5}\\ =3$

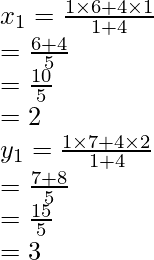
Hence, Coordinates of P are (2, 3)

⇒ Q divides AB in the ratio 2 : 3

Therefore,

$x_2=\frac{2\times6+3\times1}{2+3}\\ =\frac{12+3}{5}\\ =\frac{15}{5}\\ =3\\ y_2=\frac{2\times7+3\times2}{2+3}\\ =\frac{14+6}{5}\\ =\frac{20}{5}\\ =4$

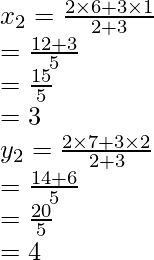
Hence, Coordinates of 3, 4

⇒ R divides AB in ration 3 : 2

Therefore,

$x_3=\frac{3\times6+2\times1}{3+2}\\ =\frac{18+2}{5}\\ =\frac{20}{5}\\ =4\\ y_2=\frac{3\times7+2\times2}{3+2}\\ =\frac{21+4}{5}\\ =\frac{25}{5}\\ =5$

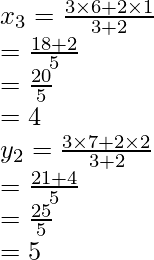
Hence, Coordinates of R are (4, 5).

问题 39. 如果 A 和 B 是两个坐标分别为(-2, -2) 和 (2, -4) 的点，求P 的坐标使得 AP = 3/7 AB

解决方案：

AP = 3/7 AB

7AP = 3AB

7AP = 3(AP + BP)

⇒ 7AP = 3AP + 3BP

⇒ 7AP – 3AP = 3BP

⇒ 4 AP = 3 BP

⇒ $\frac{AP}{BP}=\frac{3}{4}$

Therefore, 

AP : BP = 3 : 4

Because P divides AB in the ratio of 3 : 4 whose end points are A(-2, -2) and B(2, -4)

Therefore, Coordinates of P will be

$x=\frac{mx_2+nx_1}{m+n}\\ y=\frac{my_2+ny_1}{m+n}\\ x=\frac{3(2)+4(-2)}{3+4}\\ =\frac{6-8}{7}\\ =\frac{-2}{7}$

$\\ y=\frac{3(-4)+4(-2)}{3+4}\\ =\frac{-12-8}{7}\\ =\frac{-20}{7}$

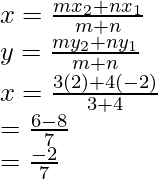
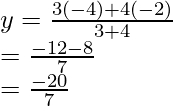
Therefore,

Coordinates of P will be $\left(\frac{-2}{7},\ \frac{-20}{7}\right)$

问题 40. 求将连接 A (-2, 2) 和 B (2, 8) 的线段分成四个相等部分的点的坐标。

解决方案：

Assume P, Q and R divides the line segment AB in four equal parts

Co-ordinates of A are (-2, 2) and of B are (2, 8)

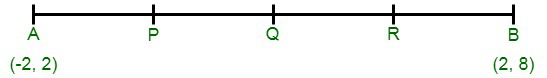
It can be seen that Q divides AB in two equal parts while P bisects AQ and R, bisect QB.

Now,

Coordinates of Q will be :

$\frac{x_1+x_2}{2},\ \frac{y_1+y_2}{2}\\ =\left(\frac{-2+2}{2},\ \frac{2+8}{2}\right)\\ =\left(\frac{0}{2},\ \frac{10}{2}\right)\\ =(0, 5)$

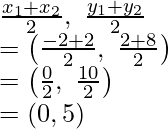
Similarly, coordinates of P will be:

$\frac{x_1+x_2}{2},\ \frac{y_1+y_2}{2}\\ =\left(\frac{-2+0}{2},\ \frac{2+5}{2}\right)\\ =\left(\frac{-2}{2},\ \frac{7}{2}\right)\\ =(-1, \frac{7}{2})$

Coordinates of R will be:

$\frac{x_1+x_2}{2},\ \frac{y_1+y_2}{2}\\ =\left(\frac{0+2}{2},\ \frac{5+8}{2}\right)\\ =\left(\frac{2}{2},\ \frac{13}{2}\right)\\ =(1, \frac{13}{2})$

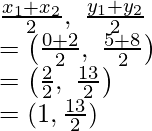
Hence, Coordinates of P are(1, 7/2) 

Coordinates of Q are (0, 5)

Coordinates of R are (1, 13/2)