第 10 类 RD Sharma 解决方案 - 第 14 章坐标几何 - 练习 14.5 |设置 3

问题 23. 如果 1/a + 1/b = 1，证明点 (a, 0)、(0, b) 和 (1, 1) 共线。

解决方案：

Let us assume that the points are A (a, 0), B (0, b) and C (1, 1) form a triangle ABC

Area of ∆ABC = 1/2[x₁(y₂ – y₃) + (y₃ – y₁)x₂ + x₃(y₁ – y₂)]

= 1/2[a(b – 1) + 0(1 – 0) + 1(0 – b)]

= 1/2[ab – a + 0 + 1(-b)]

= 1/2[ab – a – b]

If the points are collinear, then area of ∆ABC = 0

⇒1/2(ab – a – b) = 0

⇒ab – a – b = 0

⇒ ab = a + b

Now on dividing by ab, we get

$\frac{ab}{ab}=\frac{a}{ab}+\frac{b}{ab}$

⇒$1=\frac{1}{b}+\frac{1}{a}⇒\frac{1}{a}+\frac{1}{b}=1$

问题 24. 点 A 将 P (-5, 1) 和 Q (3, 5) 的连接除以 k : 1 的比率。 求 ΔABC 的面积为 (1, 5) 的 k 的两个值) 和 C (7, -2) 等于 2 个单位。

解决方案：

Let us assume that the coordinates of A be (x, y) which divides the join of P (-5, 1) and Q (3, 5) in the ratio. 

So, the coordinates of A will be $(\frac{mx_2+nx_1}{m+n},\frac{my_2+ny_1}{m+n})$ 

Or $(\frac{k*3+1*(-5)}{k+1},\frac{k(5)+1(1)}{k+1})$ or $(\frac{3k-5}{k+1},\frac{5k+1}{k+1})$

It is given that area of ∆ABC is 2 units and vertices are B(1, 5), C(7, -2) and A are the vertices 

So, Area of ∆ABC = 1/2[x₁(y₂ – y₃) + (y₃ – y₁)x₂ + x₃(y₁ – y₂)]

⇒$2=\frac{1}{2}[\frac{3k-5}{k+1}(5+2)+1(-2-\frac{5k+1}{k+1}+7(\frac{5k+1}{k+1}-5)]$

⇒$2=\frac{1}{2}[\frac{3k-5}{k+1}*7-2-\frac{5k+1}{k+1}+7(\frac{5k+1}{(k+1)-35})]\\ ⇒4=[\frac{21k-35}{k+1}-\frac{5k+1}{k+1}+\frac{35k+7}{k+1}-37]\\ ⇒4=[\frac{21k-35-5k+1+35k+7-37k-37}{k+1}]\\ ⇒|\frac{14k-66}{k+1}|=±4$

(i) (14k – 66)(k + 1) = 4 ⇒ 14k – 66 = 4k + 4

⇒ 14k – 4k = 66 + 4

⇒10k = 70

⇒ k = 70/10 = 7

(ii) (14k – 66)(k + 1) = -4 ⇒ 14 – 66 = -4k – 4

⇒ 14k + 4k = -4 + 66 ⇒ 18k = 62 

⇒ k = 62/18 = 31/9  

Hence, the value of k is 7, 31/9 

问题 25。三角形的面积是 5。它的两个顶点是 (2, 1) 和 (3, -2)。第三个顶点位于 y = x + 3 上。找到第三个顶点。

解决方案：

Let us considered ABC is a triangle whose vertices are (2, 1), (3, -2) and (x, y)

Also the area of triangle ABC is 5

So, 

Area of ∆ABC = 1/2[x₁(y₂ – y₃) + (y₃ – y₁)x₂ + x₃(y₁ – y₂)]

5 = 1/2[x(1 + 2) + 2(-2 – y) + 3(y – 1)]

10 = [3x-4-2y+3y-3] 

10 = 3x + y – 7

3x + y = 10 + 7

3x + y = 17

But it is given that the point (x, y) lies on y = x + 3

So, 3x + x + 3 = 17

⇒ 4x = 17 – 3

4x = 14

⇒ x = 7/2

and y = x + 3 = 7/2 +3 = 13/2

Hence, the third vertex is (7/2, 13/2)

问题 26. 四个点 A (6, 3), B (-3, 5), C (4, -2) 和 D (x, 3x) 以这样的方式给出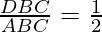$\frac{△DBC}{△ABC}=\frac{1}{2}$ , 找到 x?

解决方案：

Let us assume that ABCD is a quadrilateral whose vertices are A (6, 3), B (-3, 5), C (4, -2) and D (x, 3x)

Now, AC and BD are joined

Area of triangle = 1/2[x₁(y₂ – y₃) + (y₃ – y₁)x₂ + x₃(y₁ – y₂)]

= 1/2 [6(5 + 2) + (-3)(-2 – 3) + 4(3 – 5)]

= 1/2 [6 * 7 + (-3)(-5) + 4(-2)]

= 1/2 [42 + 15 – 8] = 49/2 

and area of △DBC,

= 1/2[x(5 + 2) + (-3)(-2 – 3x) + 4(3x – 5)]

= 1/2[7x + 6 + 9x + 12x – 20]

= 1/2[28x – 14] = 14x – 7

Now,$\frac{∆DBC}{∆ABC}$[Tex]=\frac{14x-7}{\frac{49}{2}} [/Tex]

$=\frac{(14x-7)*2}{49}$

$|\frac{2(14x-7)}{49}|=\frac{1}{2}⇒4|14x-7|=±49$

If 56x – 28 = 49

⇒ 56x = 49 + 28 = 77

⇒ x = 77/56 = 11/8

If 4(14x – 7) = 49

⇒56x – 28 = -49

⇒56x = -49 + 28 = -21

⇒x = -21/56 = -3/8

x = 11/8 or -3/8  

问题 27. 如果三个点 (x ₁ , y ₁ ), (x ₂ , y ₂ ), (x ₃ , y ₃ ) 在同一条直线上，证明

$\frac{y_2-y_3}{x_2x_3}+\frac{y_3-y_1}{x_3x_1}+\frac{y_1-y_2}{x_1x_2}=0$

解决方案：

Let us assume that ABC is a triangle whose vertices are (x₁, y₁), (x₂, y₂), (x₃, y₃) 

Area of ∆ABC = 1/2[x₁(y₂ – y₃) + (y₃ – y₁)x₂ + x₃(y₁ – y₂)]

The points be on the same line, so

[x₁(y₂ – y₃) + (y₃ – y₁)x₂ + x₃(y₁ – y₂)] = 0

On dividing by x₁ x₂ x₃, we get

$\frac{x_1(y_2-y_1)}{x_1x_2x_3}+\frac{x_2(y_3-y_1)}{x_1x_2x_3}+\frac{x_3(y_1-y_2)}{x_1x_2x_3}=0\\ \frac{y_2-y_3}{x_2x_3}+\frac{y_3-y_1}{x_3x_1}+\frac{y_1-y_2}{x_1x_2}=0$

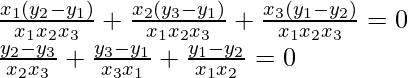
问题 28. 如果平行四边形 ABCD 的三个顶点是 A (2, 4)、B (2 + √3, 5) 和 C (2, 6)，则求其面积。

解决方案：

Given that ABCD is a ||gm whose vertices are A (2, 4), B (2 + √3, 5) and C (2, 6).

Now draw one diagonal AC of ||gm ABCD

Diagonal bisects the ||gm into two triangle  equal in area

Area of ∆ABC = 1/2[x₁(y₂ – y₃) + (y₃ – y₁)x₂ + x₃(y₁ – y₂)]

= 1/2 [2(5 – 6) + (2 + √3)(6 – 4) + 2(4 – 5)]

= 1/2 [2 * (-1) + (2 + √3) * 2 + 2 * (-1)]

= 1/2 [-2 + 4 + 2√3 – 2] = 1/2 (2√3) = √3sq.units

So, the area of ||gm ABCD = 2 * area(△ABC)

= 2 * (√3) = 2√3 sq.units

问题 29. 找出点 (3k – 1, k – 2)、(k, k – 7) 和 (k – 1, -k – 2) 共线的 k 值 (s)。

解决方案：

Let us assume that the ABC is a triangle whose vertices are A(3k – 1, k – 2), B(k, k – 7) and C(k – 1, -k – 2) 

and A, B, C are collinear

Area of ∆ABC = 1/2[x₁(y₂ – y₃) + (y₃ – y₁)x₂ + x₃(y₁ – y₂)]

= 1/2[(3k – 1)(k – 7 + k + 2) + k(-k – 2 – k + 2) + (k – 1)(k – 2 – k + 7)]

= 1/2 [(3k – 1)(2k – 5) + k(-2k + (k – 1) * 5)]

= 1/2 [6k² – 15k – 2k + 5 – 2k² + 5k – 5] 

= 1/2[4k² – 12k] = 2k² – 6k

= 2k(k – 3)

As we know that the points are collinear 

so, Area of ABC=0

2k(k – 3) = 0

Either 2k = 0 or k – 3 = 0, then k = 0 or k = 3

Therefore, k = 0, 3

问题 30. 如果点 A (-1, -4)、B (b, c) 和 C (5, -1) 共线且 2b + c = 4，求 b 和 c 的值。

解决方案：

Let us assume that the ABC is a triangle whose vertices are A (-1, -4), B (b, c) and C (5, -1)

and A, B, C are collinear

Area of ∆ABC = 1/2[x₁(y₂ – y₃) + (y₃ – y₁)x₂ + x₃(y₁ – y₂)]

= 1/2 [-1(c + 1) + b(-1 + 4) + 5(-4 – c)]

= 1/2 [-c – 1 – b + 4b – 20 – 5c]

= 1/2 [3b – 6c – 21]

As we know that the points are collinear 

so, Area of triangle ABC = 0

1/2(2b – 6c – 21) = 0

⇒3b – 6c = 21

3b – 6c = 21             ———–(i)

and 2b + c = 4        ———-(ii)

⇒c = 4 – 2b

On substituting the value of c in eq (i), we get

⇒3b – 6(4 – 2b) = 21

⇒3b – 24 + 12b = 21

15b = 21 + 24 = 45

⇒b = 45/15 = 3

c = 4 – 2b = 4 – 2 * 3 = 4 – 6 = -2

b = 3, c = -2

问题 31. 如果点 A (-2, 1)、B (a, b) 和 C (4, -1) 共线且 a – b = 1，求 a 和 6 的值。

解决方案：

Let us assume that the ABC is a triangle whose vertices are A (-2, 1), B (a, b), and C (4, -1) 

and A, B, C are collinear

Area of ∆ABC = 1/2[x₁(y₂ – y₃) + (y₃ – y₁)x₂ + x₃(y₁ – y₂)]

= 1/2 [-2(b + 1) + a(-1 – 1) + 4(1 – b)]

= 1/2[-2b – 2 – 2a + 4 – 4b] 

= 1/2 [-2b – 2 – 2a + 4 – 4b]

= 1/2 [-2a – 6b + 2] = -a – 3b + 1

As we know that the points are collinear 

so, Area of triangle ABC = 0

-a – 3b + 1 = 0 

a + 3b = 1                 ————(i)

a = 1 – 3b

and given that a – b = 1 ————(ii)

On solving eq(i) and (ii), we get

4b = 0 ⇒ b = 0

Therefore, a = 1 – 3b = 1 – 3 * 0 = 1 – 0 = 1

a = 1, b = 0

问题 32. 如果点 A (1, -2), B (2, 3), C (a, 2) 和 D (-4, -3) 形成一个平行四边形，求 a 的值和高度以AB为底的平行四边形。

解决方案：

Given that ABCD is a parallelogram whose vertices are A (1, -2), B (2, 3), C (a, 2), and D (-4, -3) 

As we know that, diagonals bisects each other

i.e., mid-point of AC = mid-point of BD

⇒$(\frac{1+a}{2},(\frac{-2+2}{2})=(\frac{2-4}{2},\frac{3-3}{2})\\ ⇒\frac{1+a}{2}=\frac{2-4}{2}=\frac{-2}{2}=-1$

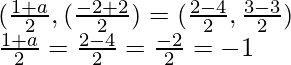
= 1/2[x₁(y₂ – y₃) + (y₃ – y₁)x₂ + x₃(y₁ – y₂)]

⇒1 + a = -2

⇒a = -3

So, the required value of a is -3

Given that, AB as base of a ||gm and drawn a perpendicular from A to AB  

which meet AB at P. So, DP is a height of a ||gm.

Now, equation of base AB, passing through the points (1, -2) and (2, 3) is 

⇒$(y-y_1)=\frac{y_2-y_1}{x_2-x_1}(x-x_1)$

⇒(y + 2)=$\frac{3+2}{2-1}(x-1)$

⇒(y + 2) = 5(x – 1)    

⇒5x – y = 7             ————(i)

So, the slope of AB, say $m_1=\frac{y_2-y_1}{x_2-x_1}=\frac{3+2}{2-1}=5$

Let the slope of DP be m₂.

Since, DP is perpendicular to AB.

By condition of perpendicularity

m₁ * m₂ = -1 ⇒ 5 * m₂ = -1

⇒m₂ = -1/5

Now, eq. of DP, having slope (-1/5)

and passing the point (-4,-3) is (y – y₁) = m₂(x – x₁)

⇒$(y+3)=-\frac{1}{5}(x+4)$

⇒5y + 15 = -x – 4

⇒x + 5y = -19                  ————-(ii)

On adding eq (i) and (ii), then we get the intersection point P.

Now put the value of y from eq. (i) in eq. (ii), we get

x + 5(5x – 7) = -19                  [using Eq. (i)]

⇒x + 25x – 35 = -19

⇒26x = 16

x = 8/13

Now put the value of x in Eq.(i), we get

$y=5(\frac{8}{13})-7=\frac{40}{13}-7\\ ⇒y=\frac{40-91}{13}⇒y=\frac{-51}{13}$

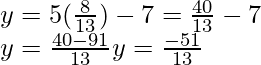
So, the coordinates of point P$=(\frac{8}{13},\frac{-51}{13})$

And, the length of the height of a parallelogram,

$DP=\sqrt{(\frac{8}{13}+4)^2+(\frac{-51}{13}+3)^2}$ 

Now, by using distance formula, we get

$d=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\\ ⇒DP=\sqrt{(\frac{60}{13})^2+(\frac{-12}{13})^2}\\ =\frac{1}{13}\sqrt{3600+144}\\ =\frac{1}{13}.\sqrt{3744}=\frac{12\sqrt{26}}{13}$

Hence, the required length of height of a parallelogram is 12√26/13   

问题 33. A (6, 1), B (8, 2) 和 C (9, 4) 是平行四边形 ABCD 的三个顶点。如果 E 是 DC 的中点，求 ΔADE 的面积。

解决方案：

Given that, ABCD is a parallelogram, whose vertices are A (6,1), B (8,2) and C (9,4)

Let us assume that the fourth vertex of parallelogram be D(x, y).

Now as we know that, the diagonal of a parallelogram bisect each other.

so, Mid point of BD = Mid point of AC

⇒$(\frac{8+x}{2},\frac{2+y}{2})=(\frac{6+9}{2},\frac{1+4}{2})$

⇒$(\frac{8+x}{2},\frac{2+y}{2})=(\frac{15}{2},\frac{5}{2})\\ \frac{8+x}{2}=\frac{15}{2}$

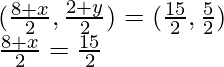
⇒8+x=15⇒x=7

and $\frac{2+y}{2}=\frac{5}{2}$

⇒2 + y = 5 ⇒ y = 3

So, fourth vertex of ||gm is D(7, 3)

Now, mid-point of side DC$=(\frac{7+9}{2},\frac{3+4}{2})$

E = (8, 7/2)

Now we find the area of ∆ADE

= 1/2[6(3 – 7/2) + 7(7/2 – 1) + 8(1 – 3)]

= 1/2 [6 * (-1/2 + 7(5/2 + 8(-2)]

= 1/2 (-3 + 35/2 – 16)

= 1/2 ( 35/2 – 19) 

= 1/2 (-3/2)

= -3/4                          [As we know that area cannot be negative]

Hence, the required area ∆ADE is 3/4sq. units

问题 34. 如果 D 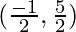$(\frac{-1}{2},\frac{5}{2})$ E (7, 3) 和 F 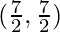$(\frac{7}{2},\frac{7}{2})$是 ΔABC 各边的中点，求 ΔABC 的面积。

解决方案：

Given that ABC is a triangle, so let us assume that the vertices of triangle ABC are A(x₁, y₁), B(x₂, y₂) and C(x₃, y₃) 

And the mid points of side BC, CA, and AB are D(-1/2, 5/2), E(7, 3), and F(7/2, 7/2)

Since, D(-1/2, 5/2) is the mid-point of BC.

So, $\frac{x_2+x_3}{2}=-\frac{1}{2}$

and $\frac{y_2+y_3}{2}=\frac{5}{2}$

⇒x₂ + x₃ = -1          ————(i)

and y₂ + y₃ = 5

As we know that E(7, 3) is the mid-point of CA.

So, $\frac{x_3+x_1}{2}=7$ and $\frac{y_3+y_1}{2}=3$

⇒x₃ + x₁ = 14                      ———–(iii)

and y₃ + y₁ = 6                   ————(iv)

Also, F(7/2, 7/2) is the mid-point of AB $\frac{x_1+x_2}{2}=\frac{7}{2}$

 and $\frac{y_1+y_2}{2}=\frac{7}{2}$

⇒x₁ + x₂ = 7             ————–(v)

and y₁ + y₂ = 7        —————-(vi) 

Now on adding Eq.(i), (ii) and (v), we get

2(x₁ + x₂ + x₃) = 20

x₁ + x₂ + x₃ = 10                    —————(vii)

On subtracting Eq. (i) (iii) and (v) from Eq. (vii), we get

x₁ = 11, x₂ = -4, x₃ = 3

On adding Eq.(ii), (iv) and (vi), we get

2(y₁ + y₂ + y₃) = 18

⇒y₁ + y₂ + y₃ = 9    ————-(viii)

On subtracting Eq. (ii), (iv) and (vi) from Eq. (viii), we get

y₁ = 4, y₂ = 3, y₃ = 9

Hence, the vertices of ∆ABC are A(11, 4), B(-4, 3) and C(3, 2)

Area of ∆ABC = 1/2[x₁(y₂ – y₃) + (y₃ – y₁)x₂ + x₃(y₁ – y₂)]

= 1/2[11(3 – 2) + (-4)(2 – 4) + 3(4 – 3)]

= 1/2[11 * 1 + (-4)(-2) + 3(1)]

= 1/2(11 + 8 + 3)

= 22/2 = 11

Hence, the required area of ∆ABC is 11 sq.unit