问题1.在x = 10处找到x 2 – 2的导数。
解决方案:
f(x) = x2 – 2
f(x+h) = (x+h)2 – 2
From the first principle,
![]()
When, x = 10
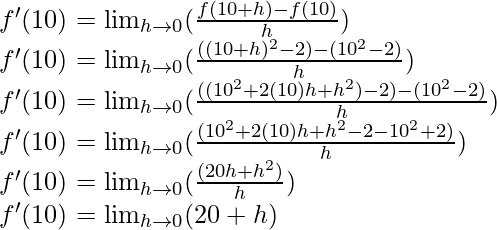
f'(10) = 20 + 0
f'(10) = 20
问题2.在x = 1处找到x的导数。
解决方案:
f(x) = x
f(x+h) = x+h
From the first principle,
![]()
When, x = 1
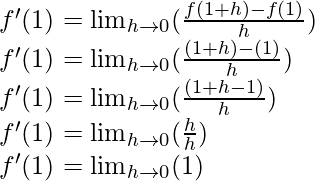
f'(1) = 1
问题3.在x = 100处找到99x的导数。
解决方案:
f(x) = 99x
f(x+h) = 99(x+h)
From the first principle,
![]()
When, x = 10
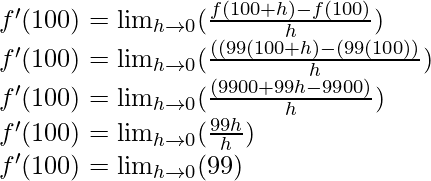
f'(100) = 99
问题4.从第一原理中找到以下函数的派生词。
(i)x 3 − 27
解决方案:
f(x) = x3 – 27
f(x+h) = (x+h)3 – 27
From the first principle,
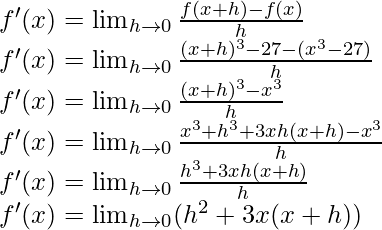
f'(x) = 02+3x(x+0)
f'(x) = 3x2
(ii)(x-1)(x-2)
解决方案:
f(x) = (x-1) (x-2) = x2 – 3x + 2
f(x) = (x+h)2 – 3(x+h) + 2
From the first principle,
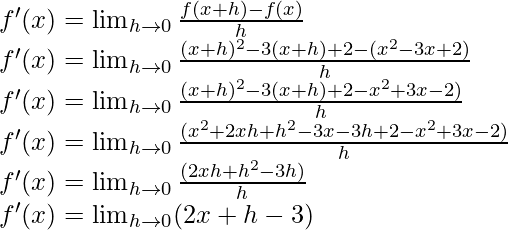
f'(x) = 2x+0 – 3
f'(x) = 2x – 3
(iii) 
解决方案:
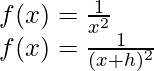
From the first principle,

(iv) 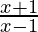
解决方案:
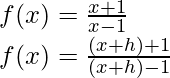
From the first principle,
![Rendered by QuickLaTeX.com f'(x) = \lim_{h \to 0} \frac{f(x+h)-f(x)}{h}\\ f'(x) = \lim_{h \to 0} \frac{\frac{(x+h)+1}{(x+h)-1}-(\frac{x+1}{x-1})}{h}\\ f'(x) = \lim_{h \to 0} \frac{\frac{(x+h+1)(x-1)-(x+h-1)(x+1)}{(x+h-1)(x-1)}}{h}\\ f'(x) = \lim_{h \to 0} \frac{\frac{(x^2+hx+x)-(x+h+1)-[(x^2+hx-x)+(x+h-1)]}{(x+h-1)(x-1)}}{h}\\ f'(x) = \lim_{h \to 0} \frac{\frac{(x^2+hx+x-x-h-1)-(x^2+hx-x+x+h-1)}{(x+h-1)(x-1)}}{h}\\ f'(x) = \lim_{h \to 0} \frac{\frac{(x^2+hx+x-x-h-1-x^2-hx+x-x-h+1)}{(x+h-1)(x-1)}}{h}\\ f'(x) = \lim_{h \to 0} \frac{\frac{(-h-h)}{(x+h-1)(x-1)}}{h}\\ f'(x) = \lim_{h \to 0} \frac{\frac{-2h}{(x+h-1)(x-1)}}{h}\\ f'(x) = \lim_{h \to 0} \frac{-2h}{h(x+h-1)(x-1)}\\ f'(x) = \lim_{h \to 0} \frac{-2}{(x+h-1)(x-1)}\\ f'(x) = \frac{-2}{(x-1)(x-1)}\\ f'(x) = \frac{-2}{(x-1)^2}\\](https://mangdo-1254073825.cos.ap-chengdu.myqcloud.com//front_eng_imgs/geeksforgeeks2021/Class%2011%20NCERT%20Solutions%20%E2%80%93%20Chapter%2013%20Limits%20And%20Derivatives%20%E2%80%93%20Exercise%2013.2_13.jpg)
问题5.对于函数
f(x)= 
证明f’(1)= 100 f’(0)
解决方案:
Given,
![]()
By using this, taking derivative both sides
![]()
As, the derivative of xn is nxn-1 and derivative of constant is 0.
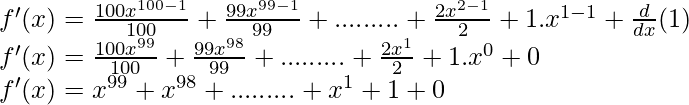
Now, then
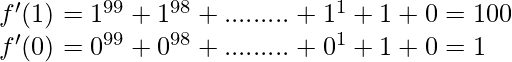
Hence, we conclude that
f'(1) = 100 f'(0)
问题6.求出x n + ax n-1 + a 2 x n-2 +………….. + a n-1 x + a n对于某个固定实数a的导数。
解决方案:
Given,
f(x) = xn + axn-1 + a2xn-2 + ……………….+ an-1x + an
As, the derivative of xn is nxn-1 and derivative of constant is 0.
By using this, taking derivative both sides

问题7.对于某些常数a和b,找到
(i)(xa)(xb)
解决方案:
f(x) = (x-a) (x-b)
f(x) = x2 – (a+b)x + ab
Taking derivative both sides,
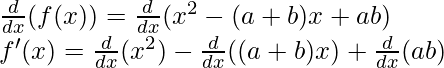
As, the derivative of xn is nxn-1 and derivative of constant is 0.
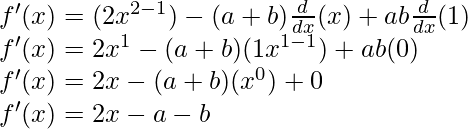
(ii)(ax 2 + b) 2
解决方案:
f(x) = (ax2 + b)2
f(x) = (ax2)2 + 2(ax2)(b) + b2
Taking derivative both sides,
![]()
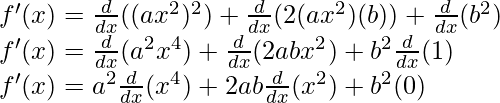
As, the derivative of xn is nxn-1 and derivative of constant is 0.
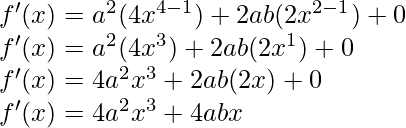
(iii) 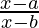
解决方案:
![]()
Taking derivative both sides,
![]()
Using quotient rule, we have
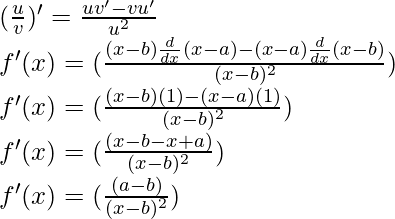
问题8.求出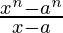 对于一些常数
对于一些常数
解决方案:
![]()
Taking derivative both sides,
![]()
Using quotient rule, we have
![Rendered by QuickLaTeX.com (\frac{u}{v})' = \frac{uv'-vu'}{u^2}\\ f'(x) = (\frac{(x-a)\frac{d}{dx}(x^n-a^n)-(x^n-a^n)\frac{d}{dx}(x-a)}{(x-a)^2})\\ f'(x) = (\frac{(x-a)[\frac{d}{dx}(x^n)-\frac{d}{dx}(a^n)]-(x^n-a^n)(1)}{(x-a)^2})](https://mangdo-1254073825.cos.ap-chengdu.myqcloud.com//front_eng_imgs/geeksforgeeks2021/Class%2011%20NCERT%20Solutions%20%E2%80%93%20Chapter%2013%20Limits%20And%20Derivatives%20%E2%80%93%20Exercise%2013.2_32.jpg)
As, the derivative of xn is nxn-1 and derivative of constant is 0.
![Rendered by QuickLaTeX.com f'(x) = (\frac{(x-a)[(nx^{n-1})-0)]-(x^n-a^n)}{(x-a)^2})\\ f'(x) = (\frac{(x-a)(nx^{n-1})-x^n+a^n}{(x-a)^2})\\ f'(x) = (\frac{(nx^{n-1+1}-anx^{n-1})-x^n+a^n}{(x-a)^2})\\ f'(x) = (\frac{(nx^n-anx^{n-1})-x^n+a^n}{(x-a)^2})](https://mangdo-1254073825.cos.ap-chengdu.myqcloud.com//front_eng_imgs/geeksforgeeks2021/Class%2011%20NCERT%20Solutions%20%E2%80%93%20Chapter%2013%20Limits%20And%20Derivatives%20%E2%80%93%20Exercise%2013.2_33.jpg)
问题9.求出
(一世) 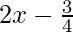
解决方案:
![]()
Taking derivative both sides,
![]()
As, the derivative of xn is nxn-1 and derivative of constant is 0.
f'(x) = (2x0)-0
f'(x) = 2
(ii)(5x 3 + 3x – 1)(x-1)
解决方案:
f(x) = (5x3 + 3x – 1)(x-1)
Taking derivative both sides,
![]()
Using product rule, we have
(uv)’ = uv’ + u’v
![]()
As, the derivative of xn is nxn-1 and derivative of constant is 0.
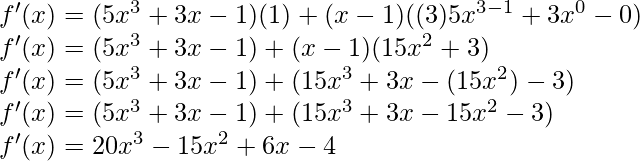
(iii)x -3 (5 + 3x)
解决方案:
f(x) = x-3 (5+3x)
Taking derivative both sides,
![]()
Using product rule, we have
(uv)’ = uv’ + u’v
![]()
As, the derivative of xn is nxn-1 and derivative of constant is 0.

(iv)x 5 (3-6x -9 )
解决方案:
f(x) = x5 (3-6x-9)
Taking derivative both sides,
![]()
Using product rule, we have
(uv)’ = uv’ + u’v
![]()
As, the derivative of xn is nxn-1 and derivative of constant is 0.
![Rendered by QuickLaTeX.com f'(x) = (x^5)[\frac{d}{dx}(3)-\frac{d}{dx}(6x^{-9})] + (3-6x^{-9})(5x^{5-1})\\ f'(x) = (x^5)[0-((-9)6x^{-9-1})] + (3-6x^{-9})(5x^{4})\\ f'(x) = (x^5)(54x^{-10}) + (3(5x^{4})-6x^{-9}(5x^{4}))\\ f'(x) = 54x^{-10+5} + (15x^{4} -30x^{-9+4})\\ f'(x) = 54x^{-5} + 15x^{4} -30x^{-5}\\ f'(x) = 24x^{-5} + 15x^{4}](https://mangdo-1254073825.cos.ap-chengdu.myqcloud.com//front_eng_imgs/geeksforgeeks2021/Class%2011%20NCERT%20Solutions%20%E2%80%93%20Chapter%2013%20Limits%20And%20Derivatives%20%E2%80%93%20Exercise%2013.2_45.jpg)
(v)x -4 (3-4x -5 )
解决方案:
f(x) = x-4 (3-4x-5)
Taking derivative both sides,
![]()
Using product rule, we have
(uv)’ = uv’ + u’v
![]()
As, the derivative of xn is nxn-1 and derivative of constant is 0.
![Rendered by QuickLaTeX.com f'(x) = (x^{-4})[\frac{d}{dx}(3)-\frac{d}{dx}(4x^{-5})] + (3-4x^{-5})(-4x^{-4-1})\\ f'(x) = (x^{-4})[0-(4(-5)x^{-5-1})] + (3-4x^{-5})(-4x^{-5})\\ f'(x) = (x^{-4})[20x^{-6})] + (3(-4x^{-5})-4x^{-5}(-4x^{-5}))\\ f'(x) = (20x^{-6-4}) + (-12x^{-5}-16x^{-5-5})\\ f'(x) = (20x^{-10}) - 12x^{-3} - 16x^{-12})\\ f'(x) = 36x^{-10} - 12x^{-3}](https://mangdo-1254073825.cos.ap-chengdu.myqcloud.com//front_eng_imgs/geeksforgeeks2021/Class%2011%20NCERT%20Solutions%20%E2%80%93%20Chapter%2013%20Limits%20And%20Derivatives%20%E2%80%93%20Exercise%2013.2_48.jpg)
(六) 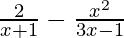
解决方案:
![]()
Taking derivative both sides,
![]()
Using quotient rule, we have
![Rendered by QuickLaTeX.com (\frac{u}{v})' = \frac{uv'-vu'}{u^2}\\ f'(x) = [\frac{(x+1)\frac{d}{dx}(2)-(2)\frac{d}{dx}(x+1)}{(x+1)^2})] - [\frac{(3x-1)\frac{d}{dx}(x^2)-(x^2)\frac{d}{dx}(3x-1)}{(3x-1)^2})]](https://mangdo-1254073825.cos.ap-chengdu.myqcloud.com//front_eng_imgs/geeksforgeeks2021/Class%2011%20NCERT%20Solutions%20%E2%80%93%20Chapter%2013%20Limits%20And%20Derivatives%20%E2%80%93%20Exercise%2013.2_52.jpg)
As, the derivative of xn is nxn-1 and derivative of constant is 0.
![Rendered by QuickLaTeX.com f'(x) = [\frac{(x+1)(0)-(2)(1)}{(x+1)^2})] - [\frac{(3x-1)(2x^{2-1})-(x^2)(3)}{(3x-1)^2})]\\ f'(x) = [\frac{-2}{(x+1)^2})] - [\frac{(3x-1)(2x)-(x^2)(3)}{(3x-1)^2})]\\ f'(x) = [\frac{-2}{(x+1)^2})] - [\frac{(6x^2-2x)-3x^2)}{(3x-1)^2})]\\ f'(x) = \frac{-2}{(x+1)^2}) - \frac{(3x^2-2x)}{(3x-1)^2})](https://mangdo-1254073825.cos.ap-chengdu.myqcloud.com//front_eng_imgs/geeksforgeeks2021/Class%2011%20NCERT%20Solutions%20%E2%80%93%20Chapter%2013%20Limits%20And%20Derivatives%20%E2%80%93%20Exercise%2013.2_53.jpg)
问题10:从第一原理求出cos x的导数。
解决方案:
Here, f(x) = cos x
f(x+h) = cos (x+h)
From the first principle,
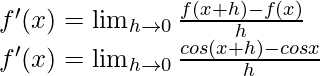
Using the trigonometric identity,
cos a – cos b = -2 sin ![]() sin
sin ![]()
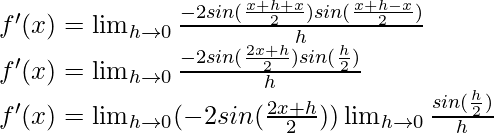
Multiplying and diving by 2,
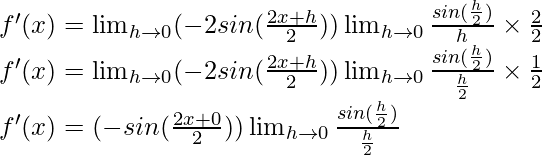
f'(x) = -sin (x) (1)
f'(x) = -sin x
问题11.查找以下函数的派生:
(i)罪恶x cos x
解决方案:
f(x) = sin x cos x
f(x+h) = sin (x+h) cos (x+h)
From the first principle,

Using the trigonometric identity,
sin A cos B = ![]() (sin (A+B) + sin(A-B))
(sin (A+B) + sin(A-B))
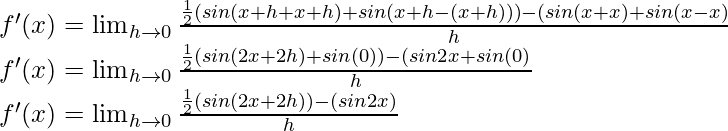
Using the trigonometric identity,
sin A – sin B = 2 cos ![]() sin
sin ![]()
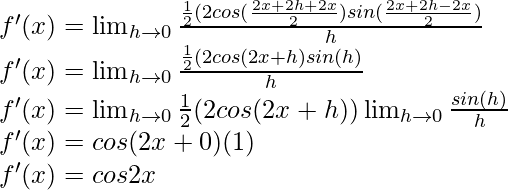
(ii)秒x
解决方案:
f(x) = sec x = ![]()
![]()
From the first principle,
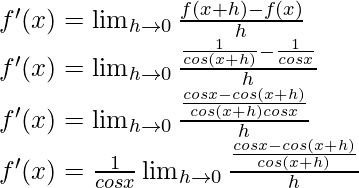
Using the trigonometric identity,
cos a – cos b = -2 sin ![]() sin
sin ![]()

Multiply and divide by 2, we have
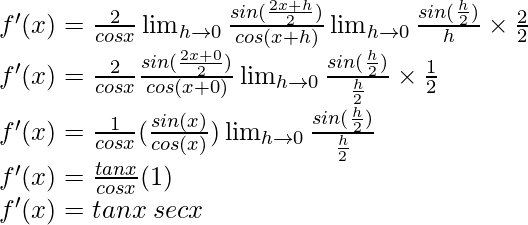
(iii)5秒x + 4 cos x
解决方案:
f(x) = 5 sec x + 4 cos x
Taking derivative both sides,
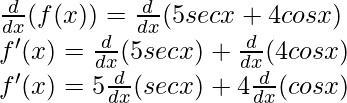
f'(x) = 5 (tan x sec x) + 4 (-sin x)
f'(x) = 5 tan x sec x – 4 sin x
(iv)cosec x
解决方案:
f(x) = cosec x = ![]()
![]()
From the first principle,
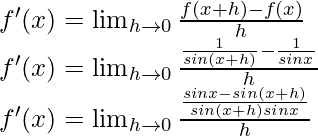
Using the trigonometric identity,
sin a – sin b = 2 cos ![]() sin
sin ![]()
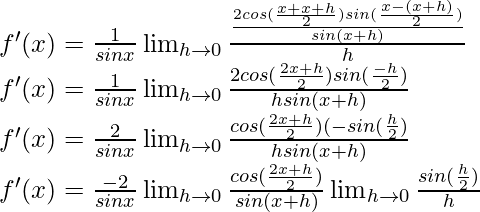
Multiply and divide by 2, we have
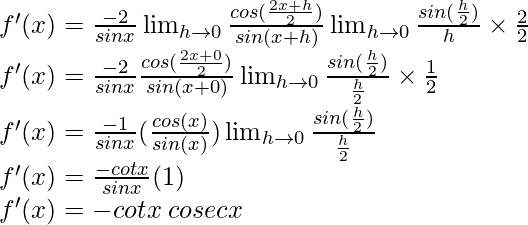
(v)3张婴儿床x + 5 sec x
解决方案:
f(x) = 3 cot x + 5 cosec x
Taking derivative both sides,
![]()
![]()
f'(x) = 3 g'(x) + 5 ![]()
Here,
g(x) = cot x = ![]()
![]()
From the first principle,
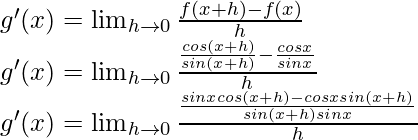
Using the trigonometric identity,
sin a cos b – cos a sin b = sin (a-b)
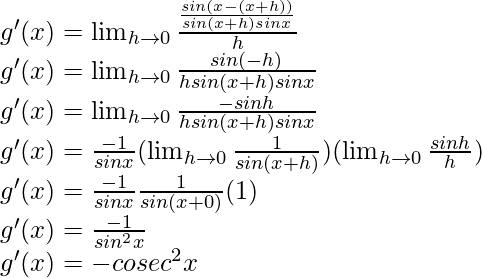
So, now
f'(x) = 3 g'(x) + 5 ![]()
f'(x) = 3 (- cosec2 x) + 5 (-cot x cosec x)
f'(x) = – 3cosec2 x – 5 cot x cosec x
(vi)5罪x – 6 cos x + 7
解决方案:
f(x) = 5 sin x – 6 cos x + 7
f(x+h) = 5 sin (x+h) – 6 cos (x+h) + 7
From the first principle,

Using the trigonometric identity,
sin a – sin b = 2 cos ![]() sin
sin ![]()
cos a – cos b = -2 sin ![]() sin
sin ![]()

Multiply and divide by 2, we get
![Rendered by QuickLaTeX.com f'(x) = \frac{2}{2}[10 \lim_{h \to 0} \frac{cos (\frac{2x+h}{2}) sin (\frac{h}{2})}{h} + 12 \lim_{h \to 0} (\frac{sin (\frac{2x+h}{2}) sin (\frac{h}{2}))}{h})]\\ f'(x) = \frac{1}{2}[10 \lim_{h \to 0} \frac{cos (\frac{2x+h}{2}) sin (\frac{h}{2})}{\frac{h}{2}} + 12 \lim_{h \to 0} (\frac{sin (\frac{2x+h}{2}) sin (\frac{h}{2}))}{\frac{h}{2}})]\\ f'(x) = 5 cos (\frac{2x+0}{2}) \lim_{h \to 0}\frac{ sin (\frac{h}{2})}{\frac{h}{2}} + 6 (sin (\frac{2x+0}{2}) \lim_{h \to 0} \frac{sin (\frac{h}{2}))}{\frac{h}{2}})](https://mangdo-1254073825.cos.ap-chengdu.myqcloud.com//front_eng_imgs/geeksforgeeks2021/Class%2011%20NCERT%20Solutions%20%E2%80%93%20Chapter%2013%20Limits%20And%20Derivatives%20%E2%80%93%20Exercise%2013.2_94.jpg)
f'(x) = 5 cos x (1) + 6 sin x (1)
f'(x) = 5 cos x + 6 sin x
(vii)2棕褐色x – 7秒x
解决方案:
f(x) = 2 tan x – 7 sec x
Taking derivative both sides,
![]()
f'(x) = ![]()
f'(x) = 2 g'(x) – 7 ![]()
Here,
g(x) = tan x = ![]()
![]()
From the first principle,
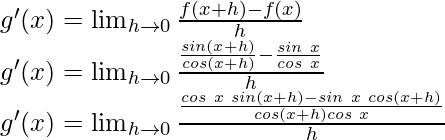
Using the trigonometric identity,
sin a cos b – cos a sin b = sin (a-b)
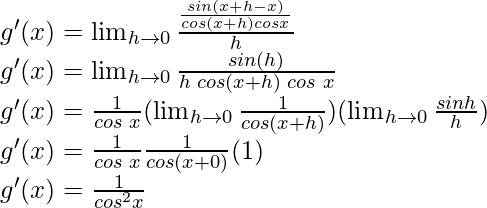
g'(x) = sec2x
So, now
f'(x) = 2 g'(x) – 7 ![]()
f'(x) = 2 (sec2x) – 7 (sec x tan x)
f'(x) = 2sec2x – 7 sec x tan x