9类NCERT解决方案-第13章表面积和体积–练习13.6

问题1.圆柱形容器底部的周长为132 cm，高度为25 cm。它可以容纳多少升水? (1000厘米³ = 1公升)

解决方案：

Given values,

Circumference of the base of a cylindrical = 132 cm

Height of cylinder (h)= 25 cm

Base of cylinder is of circle shape, having circumference = 2πr (r is radius)

Hence, 2πr = 132 cm

r = $\frac{132}{2 \times \frac{22}{7}}$               (taking π=$\frac{22}{7}$)

r =$\frac{132 \times 7}{2 \times 22}$

r = 21 cm

So, volume of cylinder = πr²h

= 22/7 × 21 × 21 × 25                                 (taking π=$\frac{22}{7}$)

= 34650 cm³

As, 1000 cm³ = 1 litre

34650 cm³ = $\frac{1}{1000}$× 34650

=$\frac{34650}{1000}$

= 34.650 litres

问题2.圆柱形木管的内径为24厘米，外径为28厘米。管道的长度为35厘米。^{如果1 cm 3}的木材的质量为0.6 g，请找到管道的质量。

解决方案：

Given values,

Inner radius of cylinder (r₁)= $\frac{24}{2}$= 12 cm

Outer radius of cylinder (r₂)= $\frac{28}{2}$= 14 cm

Height of cylinder (h)= 35 cm

So, volume used to make wood = volume of outer cylinder – volume of outer cylinder

= π(r₂²)h – π(r₁²)h

= π(r₂² – r₁²)h

= $\frac{22}{7}$× (14² – 12²) × 35                     (taking π=$\frac{22}{7}$)

= $\frac{22}{7}$× (52) × 35

= 5720 cm³

As, 1 cm³ = 0.6 g

5720 cm³ = 0.6 × 5720 g

= 3432 grams

= 3.432 kg

问题3：有两包软饮料–

(i)一个锡罐，其长为5厘米，宽为4厘米，高度为15厘米的矩形底，以及

(ii)直径为7厘米，高度为10厘米的圆形底座的塑料圆筒。

哪个容器容量更大，容量多少?

解决方案：

Let’s see each case,

(i) The shape of can is cuboid here, as having rectangular base 

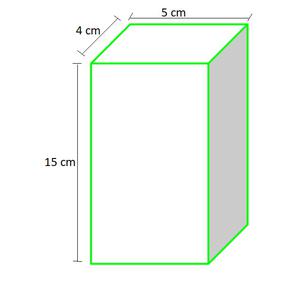
Given values,

Length of can (l) = 5 cm

Width of can (b) = 4 cm

Height of can (h) = 15 cm

So, The amount of soft drink it can hold = volume of cuboid

= (l × b × h)

= 5 × 4 × 15 cm³

= 300 cm³

(ii)The shape of can is cylinder here, as having circular base

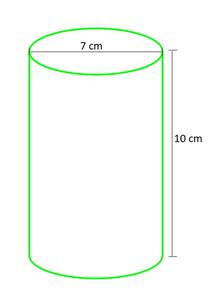
Given values,

Radius of can (r) = $\frac{7}{2}$cm

Height of can (h) = 10 cm

So, The amount of soft drink it can hold = volume of Cylinder

= (πr²h)

= $\frac{22}{7} \times \frac{7}{2} \times \frac{7}{2}$× 10 cm³                                                  (taking π=$\frac{22}{7}$)

= 385 cm³

Hence, we can see the can having circular base can contain (385 – 300 = 85 cm³) more amount of soft drinks than first can.

问题4.如果圆柱的侧面为94.2 cm ²且其高度为5 cm，则找到

(i)它的底面半径

(ii)其数量。 (使用π= 3.14)

解决方案：

Given values,

Lateral surface of cylinder = 94.2 cm²

Height of cylinder (h) = 5 cm

Let’s see each case,

(i) So, the lateral surface is of rectangle shape whose

length = (circumference of base circle of cylinder) and width = height of cylinder 

Let the base radius = r

Lateral surface = length × width

94.2 cm² = (2πr) × h                         (circumference of circle = 2πr)

94.2 cm² = (2 × 3.14 × r) × 5               (taking π = 3.14)

r = $\frac{94.2}{2 \times 3.14 \times 5}$

r = 3 cm

(ii) Given values,

Radius of cylinder (r)= 3 cm

So, the volume of cylinder = (πr²h)

= π × 3 × 3 × 5 cm³

= 3.14 × 3 × 3 × 5 cm³                    (taking π = 3.14)

= 141.3 cm³

问题5.油漆深10 m的圆柱形容器的内部曲面需要花费₹2200。如果绘画的费用为₹20每m ² ，请查找

(i)船只的内曲面面积，

(ii)基地的半径，

(iii)船只的容量

解决方案：

Given values,

Height of cylinder (h) = 10 m

Cost of painting rate = ₹20 per m²

Let’s see each case,

(i) For 1 m² = ₹20

For lateral surface = ₹2200

So the lateral surface = $\frac{2200}{20}$

= 110 m²

(ii) Let the base radius = r

So as, Lateral surface = (circumference of base circle of cylinder) × height

110 m²= (2πr) × h                      

110 = (2 × $\frac{22}{7}$× r) × 10               (taking π=$\frac{22}{7}$)

r = $\frac{110 \times 7}{2 \times 22 \times 10}$cm

r = $\frac{7}{4}$cm

r = 1.75 cm

(iii) Volume of cylinder = (πr²h)

= $\frac{22}{7} \times \frac{7}{4} \times \frac{7}{4}$× 10 cm³                  (taking π=$\frac{22}{7}$)

= 96.25 cm³

问题6.高度为1 m的封闭圆柱形容器的容量为15.4升。制作需要几平方米的金属板?

解决方案：

Given values,

Height of cylinder (h) = 1 m = 100 cm

Volume of cylinder (V) = 15.4 liters

As 1 liter = 1000 cm³

15.4 liters = 15.4 × 1000 cm³

V = 15,400 cm³

Volume of cylinder = (πr²h)

15,400 = $\frac{22}{7}$× r² × 100                                       (taking π=$\frac{22}{7}$)

r² = $\frac{15400 \times 7}{22 \times 100}$

r² = 49

r = √49

r = 7 cm

Surface area of a closed cylinder = (curve surface area + top and bottom circle) = 2πrh + (2 × πr²)

= 2πr (r+h)

= 2 × $\frac{22}{7}$× 7 × (7 + 100) cm²                                                   (taking π=$\frac{22}{7}$)

= 2 × 22 × 107

= 4708 cm²

= 0.4708 m²

Hence, 0.4708 m² of metal sheet would be needed to make it.

问题7.一支铅笔由一个圆柱体组成，内部填充有一个实心的石墨圆柱体。铅笔的直径为7毫米，石墨的直径为1毫米。如果铅笔的长度为14厘米，请找到木头和石墨的体积。

解决方案：

So here pencil = (cylinder of wood + cylinder of graphite)

Given values,

Height of wood (and graphite) cylinder (h) = 14 cm = 140 mm

Radius of pencil (R)= $\frac{7}{2}$mm

Radius of graphite (r)= $\frac{1}{2}$mm

Volume of Graphite = (πr²h)

= $\frac{22}{7} \times \frac{1}{2} \times \frac{1}{2}$× 140 mm³                                              (taking π=$\frac{22}{7}$)

= 110 mm³

= 0.11 cm³

Volume of wood = Volume of pencil – Volume of graphite

= (πR²h) – (πr²h) = π(R² – r²)h

= $\frac{22}{7}$× (($\frac{7}{2}$)² – ($\frac{1}{2}$)²) × 140 mm³                                      (taking π=$\frac{22}{7}$)

= 22 × 20 × ($\frac{49}{4}$– $\frac{1}{4}$) mm³

= 22 × 20 × 12 mm³

= 5280 mm³

= 52.80cm³

问题8.医院的病人每天在直径7厘米的圆柱形碗中喝汤。如果碗中盛有高至4厘米的汤，医院每天必须准备多少汤才能为250名患者提供服务?

解决方案：

So here Volume of soup for each patient = Volume of cylinder.

Given values,

Height of cylinder (h) = 4 cm

Radius of cylinder (r)= $\frac{7}{2}$cm

Volume of Cylinder = (πr²h)

= $\frac{22}{7}$× $\frac{7}{2} \times \frac{7}{2}$× 4 cm³                                             (taking π=$\frac{22}{7}$)

= 154 cm³ 

Volume of soup for 250 patient = 250 × Volume of cylinder.

= 250 × 154

= 38,500 cm³

Hence, 38,500cm³ soup is needed daily to serve 250 patients.