第12类NCERT解决方案-数学第I部分–第5章连续性和可微性–练习5.2

关于问题1至8中的x的微分函数

问题1.罪(x ² + 5)

解决方案：

y = sin(x² + 5)

$\frac{dy}{dx}$ = $\frac{d(sin(x^2+5)}{dx}$

= cos(x² + 5) × $\frac{d(x^2+5)}{dx}$

= cos(x² + 5) × (2x)

dy/dx = 2xcos(x² + 5)

问题2. cos(sin x)

解决方案：

y = cos(sin x)

$\frac{dy}{dx}$ = $\frac{d \ cos(sin x)}{dx}$

 = -sin(sin x) × $\frac{d \ sinx}{dx}$

= -sin(sin x)cos x  

问题3. sin(ax + b)

解决方案：

y = sin(ax + b)

$\frac{dy}{dx}=\frac{d}{dx}sin(ax+b)$

$=cos(ax+b)\frac{d}{dy}(ax+b)$

= a cos(ax + b)  

 问题4.秒(tan(√x)

解决方案：

y = sec(tan√x)

$\frac{dy}{dx}$ = $\frac{d \ (sec(tan√x)) }{dx}$ 

= sec(tan √x) × tan(√x) × $\frac{d \ (tan√x) }{dx}$

= sec (tan √x) × tan (tan √x) × sec2√x × $\frac{d \ (√x)}{dx}$ 

= sec(tan√x)tan(tan√x)(sec2√x)1/(2√x)

= 1/(2√x) × sec(tan√x)tan(tan√x)(sec2√x)

问题5 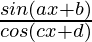$\frac{sin(ax+b)}{cos(cx+d)}$

解决方案：

y = $\frac{sin(ax+b)}{cos(cx+d)}$

$\frac{dy}{dx} = \frac{d (\frac{sin(ax+b)}{cos(cx+d)})}{dx}$

$=\frac{cos(cx+d)\frac{d}{dx}sin(ax+b)-sin(ax+b)\frac{d}{dx}cos(cx+d)}{(cos(cx+d))}$

=$\frac{cos(cx+d)cos(ax+b)(a)+sin(ax+b)sin(cx+d)(c)}{cos^2(cx+b)}$

问题6. cos x ³ .sin ² (x ⁵ )

解决方案：

y = cos x³.sin²(x⁵)

$\frac{dy}{dx} = cosx^3\frac{d(sin^2(x^5)}{dx}+sin^2(x^5)\frac{d(cosx^3)}{dx}$

= $cosx^3.2sin(x^5)\frac{d(sin(x^5)}{dx}+sin^2(x^5)(-sinx^3)\frac{d(x^3)}{dx}$

= cos x³.2sin(x⁵) .cos(x⁵(5x⁴)(5x⁴) – sin²(x⁵).sin x³.3x²

= 10x⁴ cos x³sin(x⁵)cos(x⁵) – 3x² sin²(x⁵)sin x³

问题7.2√(cos(x ² ))

解决方案：

y = 2√(cos(x²))

$\frac{dy}{dx}$ = $\frac{\ d(2\sqrt{cos(x2})}{dx}$

= 2$\frac{\ d(\sqrt{cos(x^2})}{dx}$

= $2\frac{1}{2\sqrt {cotx^2}}.\frac{d(cotx^2)}{dx}$

= $\frac{1}{\sqrt {cot(x^2)}}.(-cosec^2(x^2)).\frac{d(x^2)}{dx}$

= $\frac{1}{\sqrt {cot(x^2)}}.(-cosec^2(x^2)).2x$

=$\frac{-2xcosec^2x^2}{\sqrt{cot(x^2)}}$

= $\frac{-2x}{sin^2(x^2).\sqrt{\frac{cos(x^2)}{sin(x^2)}}}$

= $\frac{-2x}{sin(x^2) \times sin(x^2)\sqrt{\frac{cos(x^2)}{sin(x^2)}}}$

= $\frac{-2x}{sin(x^2) \times \sqrt{sin(x^2) \times \frac{cos(x^2)}{sin(x^2)}}}$

= $\frac{-2x}{sin(x^2) \times \sqrt{cos(x^2) \times {sin(x^2)}}}$

= $\frac{-2x}{sin(x^2) \times \sqrt{\frac{2}{2} \times cos(x^2) \times {sin(x^2)}}}$

= $\frac{-2\sqrt{2}x}{sin(x^2) \times \sqrt{2 \times cos(x^2) \times {sin(x^2)}}}$

= $\frac{-2\sqrt{2}x}{sin(x^2) \times \sqrt{sin(2x^2)}}$

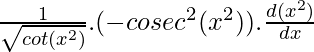
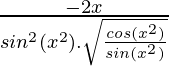
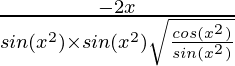
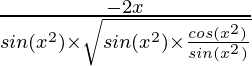
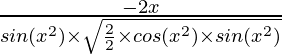
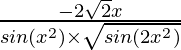
问题8. cos(√x)

解决方案：

y = cos (√x)

dy/dx = -sin√x$\frac{d\sqrt{x}}{dx}$

=$-sin\sqrt{x}\frac{1}{2}(x)^\frac{-1}{2}$

=$\frac{-sin\sqrt{x}}{2\sqrt{x}}$

问题9.证明由f(x)= | x – 1 |，x∈R给出的函数f在x = 1时不可微。

解决方案：

$RHD=\lim_{h\to 0}\frac{f(x+h)-f(x)}{h}$                                                            

= $\lim_{h\to 0}\frac{f(1+h)-f(1)}{h}$                                                      

$= \lim_{h\to 0}\frac{((1+h)-1)-(1-1)}{h}$ 

= $\lim_{h\to 0}\frac{h-0}{h}$ 

=$\lim_{h\to 0}(1)$                                                      

= +1                                                                                                            

 $LHD=\lim_{h\to 0}\frac{f(x)-f(x-h)}{h}$ 

= $\lim_{h\to 0}\frac{(1)-f(1-h)}{h}$

$\lim_{h\to 0}\frac{(1-1)-(-(1-h)-1)}{h}$

= $\lim_{h\to 0}\frac{0-h}{h}$

= $\lim_{h\to 0}(-1)$

= -1   

LHD ≠ RHD  

Hence, f(x) is not differentiable at x = 1  

问题10.证明由f(x)= [x]，0

解决方案：

Given: f(x) = [x], 0 < x < 3

LHS:

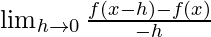
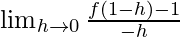
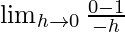
f'(1) = $\lim_{h\to0} \frac{f(x - h)-f(x)}{-h}$

= $\lim_{h\to0} \frac{f(1 - h)-1}{-h}$

=$\lim_{h\to0} \frac{0-1}{-h}$

= ∞

RHS:

f'(1) = $\lim_{h\to0} \frac{f(x + h) - f(x)}{h}$

= $\lim_{h\to0} \frac{f(1 + h) - f(1)}{h}$

= $\lim_{h\to0} \frac{1-1}{h}$

= $\lim_{h\to0} \frac{0}{h}$

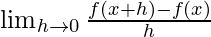
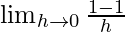
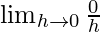
= 0

LHS ≠ RHS

So, the given f(x) = [x] is not differentiable at x = 1. 

Similarly, the given f(x) = [x] is not differentiable at x = 2.