找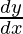 以下的:
以下的:
问题1. 2x + 3y = sin x
解决方案:
On differentiating both sides w.r.t. x, we get
2 + 3 ![]() = cos x
= cos x
3 ![]() = cos x – 2
= cos x – 2
![]() = (cosx – 2)/3
= (cosx – 2)/3
问题2。2x + 3y = sin y
解决方案:
On differentiating both sides w.r.t. x, we get
2 + 3 ![]() = cos y
= cos y![]()
(cosy – 3) ![]() = 2
= 2
![]()
问题3. ax + 2 = cos y
解决方案:
On differentiating both sides w.r.t. x, we get
a + b * 2y(![]() ) = -sin y *
) = -sin y * ![]()
(2by + siny) ![]() = -a
= -a
![]()
问题4. xy + y 2 =棕褐色x + y
解决方案:
On differentiating both sides w.r.t. x, we get
(x *![]() + y) + 2y
+ y) + 2y![]() = sec2x +
= sec2x + ![]()
(x + 2y – 1)![]() = sec2x – y
= sec2x – y
![]()
问题5. x 2 + xy + y 2 = 100
解决方案:
On differentiating both sides w.r.t. x, we get
2x + (x![]() + y) + 2y
+ y) + 2y![]() = 0
= 0
(x + 2y) * ![]() = -(2x + y)
= -(2x + y)
![]()
问题6. x 3 + x 2 y + xy 2 + y 3 = 81
解决方案:
Differentiate both sides w.r.t. x
3x2+(x2![]() + y * 2x) + (x * 2y *
+ y * 2x) + (x * 2y * ![]() + y2) + 3y2 *
+ y2) + 3y2 * ![]() = 0
= 0
(x2 + 2xy + 3y2)![]() = -(3x2 + 2xy + y2)
= -(3x2 + 2xy + y2)
![]()
问题7:Sin 2 y + cos xy =π
解决方案:
Differentiate both sides w.r.t. x
2 sin y * ![]() (siny) – sin(xy) *
(siny) – sin(xy) * ![]() xy = 0
xy = 0
2sin y * cosy ![]() – sin(xy)(x *
– sin(xy)(x * ![]() + y) = 0
+ y) = 0
(2sin cos y – sin (xy) – x)) ![]() = y(xy)
= y(xy)
![]()
![]()
问题8:sin 2 x + cos 2 y = 1
解决方案:
2 sin x * ![]() (sin x) + 2 cos y *
(sin x) + 2 cos y * ![]() (cos y) = 0
(cos y) = 0
2 sin x * cos x + 2 cos y*(-sin y) * ![]() = 0
= 0
2 sin x * cos x – 2 cos x – 2 cos y sin y * ![]() = 0
= 0
Sin(2x) – sin(2y) – ![]() = 0
= 0
![]()
问题9:y = sin -1 (\ frac {2x} {(1 + x 2 )}
解决方案:
Put x = tanθ
θ = tan-1x
![]()
y = sin-1(sin 2θ)
y = 2θ
y = 2tan-1x -(1)
On differentiating eq(1), we get
![]()
问题10。  ,-1 /√3
,-1 /√3
解决方案:
Put x = tanθ
θ = tan-1x
y = 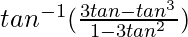
y = tan-1(tan 3θ)
y = 3θ
y = 3tan-1x -(1)
On differentiating eq(1), we get
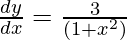
问题11  ,0
,0
解决方案:
Put x = tanθ
θ = tan-1 x
y = 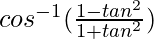
y = cos-1(cos 2θ)
y = 2θ
y = 2tan-1x -(1)
On differentiating eq(1), we get

问题12。 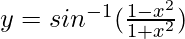 ,0
,0
解决方案:
Put x = tanθ
θ = tan-1x
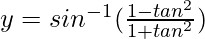
y = sin-1(cos 2θ)
y = sin-1(sin (π/2 – 2θ))
y = π/2 – 2θ
y = π/2 – 2 tan-1x

问题13。  ,-1
,-1
解决方案:
Put x = tanθ
θ = tan-1x
y = cos^{-1}(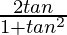 )
)
y = cos-1(sin 2θ)
y = cos-1(cos (π/2 – 2θ))
y = π/2 – 2θ
y = π/2 – 2tan-1x

问题14。  ,-1 /√2
,-1 /√2
解决方案:
Put x = sinθ
θ = sin-1 x
y = sin-1(2sinθ√(1 – sin2θ))
y = sin-1(sin 2θ) = 2θ
y = 2sin-1x

问题15。  ,0
,0
解决方案:
Put x = tanθ

y = sec-1(1/cos2θ))
y = sec-1(sec2θ) = 2θ
y = 2cos-1x
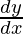 =
= 
解决方案:
Put x = tanθ
θ = tan-1x
y = ![]()
y = tan-1(tan 3θ)
y = 3θ
y = 3tan-1x -(1)
On differentiating eq(1), we get
![]()
问题11  ,0
,0
解决方案:
Put x = tanθ
θ = tan-1 x
y = 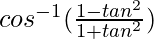
y = cos-1(cos 2θ)
y = 2θ
y = 2tan-1x -(1)
On differentiating eq(1), we get

问题12。 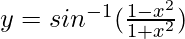 ,0
,0
解决方案:
Put x = tanθ
θ = tan-1x
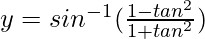
y = sin-1(cos 2θ)
y = sin-1(sin (π/2 – 2θ))
y = π/2 – 2θ
y = π/2 – 2 tan-1x

问题13。  ,-1
,-1
解决方案:
Put x = tanθ
θ = tan-1x
y = cos^{-1}(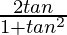 )
)
y = cos-1(sin 2θ)
y = cos-1(cos (π/2 – 2θ))
y = π/2 – 2θ
y = π/2 – 2tan-1x

问题14。  ,-1 /√2
,-1 /√2
解决方案:
Put x = sinθ
θ = sin-1 x
y = sin-1(2sinθ√(1 – sin2θ))
y = sin-1(sin 2θ) = 2θ
y = 2sin-1x

问题15。  ,0
,0
解决方案:
Put x = tanθ

y = sec-1(1/cos2θ))
y = sec-1(sec2θ) = 2θ
y = 2cos-1x
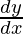 =
= 
解决方案:
Put x = tanθ
θ = tan-1 x
y = ![]()
y = cos-1(cos 2θ)
y = 2θ
y = 2tan-1x -(1)
On differentiating eq(1), we get
![]()
问题12。 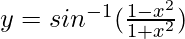 ,0
,0
解决方案:
Put x = tanθ
θ = tan-1x
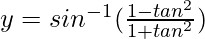
y = sin-1(cos 2θ)
y = sin-1(sin (π/2 – 2θ))
y = π/2 – 2θ
y = π/2 – 2 tan-1x

问题13。  ,-1
,-1
解决方案:
Put x = tanθ
θ = tan-1x
y = cos^{-1}(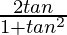 )
)
y = cos-1(sin 2θ)
y = cos-1(cos (π/2 – 2θ))
y = π/2 – 2θ
y = π/2 – 2tan-1x

问题14。  ,-1 /√2
,-1 /√2
解决方案:
Put x = sinθ
θ = sin-1 x
y = sin-1(2sinθ√(1 – sin2θ))
y = sin-1(sin 2θ) = 2θ
y = 2sin-1x

问题15。  ,0
,0
解决方案:
Put x = tanθ

y = sec-1(1/cos2θ))
y = sec-1(sec2θ) = 2θ
y = 2cos-1x
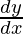 =
= 
解决方案:
Put x = tanθ
θ = tan-1x
![]()
y = sin-1(cos 2θ)
y = sin-1(sin (π/2 – 2θ))
y = π/2 – 2θ
y = π/2 – 2 tan-1x
![]()
问题13。  ,-1
,-1
解决方案:
Put x = tanθ
θ = tan-1x
y = cos^{-1}(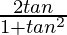 )
)
y = cos-1(sin 2θ)
y = cos-1(cos (π/2 – 2θ))
y = π/2 – 2θ
y = π/2 – 2tan-1x

问题14。  ,-1 /√2
,-1 /√2
解决方案:
Put x = sinθ
θ = sin-1 x
y = sin-1(2sinθ√(1 – sin2θ))
y = sin-1(sin 2θ) = 2θ
y = 2sin-1x

问题15。  ,0
,0
解决方案:
Put x = tanθ

y = sec-1(1/cos2θ))
y = sec-1(sec2θ) = 2θ
y = 2cos-1x
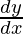 =
= 
解决方案:
Put x = tanθ
θ = tan-1x
y = cos^{-1}(![]() )
)
y = cos-1(sin 2θ)
y = cos-1(cos (π/2 – 2θ))
y = π/2 – 2θ
y = π/2 – 2tan-1x
![]()
问题14。  ,-1 /√2
,-1 /√2
解决方案:
Put x = sinθ
θ = sin-1 x
y = sin-1(2sinθ√(1 – sin2θ))
y = sin-1(sin 2θ) = 2θ
y = 2sin-1x

问题15。  ,0
,0
解决方案:
Put x = tanθ

y = sec-1(1/cos2θ))
y = sec-1(sec2θ) = 2θ
y = 2cos-1x
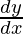 =
= 
解决方案:
Put x = sinθ
θ = sin-1 x
y = sin-1(2sinθ√(1 – sin2θ))
y = sin-1(sin 2θ) = 2θ
y = 2sin-1x
![]()
问题15。  ,0
,0
解决方案:
Put x = tanθ

y = sec-1(1/cos2θ))
y = sec-1(sec2θ) = 2θ
y = 2cos-1x
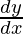 =
= 
解决方案:
Put x = tanθ
![]()
y = sec-1(1/cos2θ))
y = sec-1(sec2θ) = 2θ
y = 2cos-1x
![]() =
= ![]()