第12类RD Sharma解决方案–第29章飞机–练习29.1

问题1(i)。找到通过以下点(2，1，0)，(3，-2，-2)和(3，1，7)的平面方程。

解决方案：

Given points are (2, 1, 0), (3, -2, -2), and (3, 1, 7)

The equation of plane passing through three points is given by 

$\begin{vmatrix} x-x_1&y-y_1&z-z_1\\ x_2-x_1&y_2-y_1&z_2-z_1\\ x_3-x_1&y_3-y_1&z_3-z_1\\ \end{vmatrix} = 0$

$\begin{vmatrix} x-2&y-1&z-0\\ 3-2&-2-1&-2-0\\ 3-2&1-1&7-0\\ \end{vmatrix} = 0$

$\begin{vmatrix} x-2&y-1&z\\ 1&-3&-2\\ 1&0&7\\ \end{vmatrix} = 0$

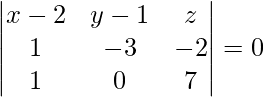
= (x – 2)(-21 – 0) – (y – 1)(7 + 2) + z(0 + 3) = 0

= -21x + 42 – 9y + 9 + 3z = 0

= -21x – 9y + 3z + 51 = 0

By taking -3 as common, we get resultant equation of plane

7x + 3y – z – 17 = 0

问题1(ii)。找到通过以下点(-5、0、6)，(-3、10，-9)和(-2、6，-6)的平面方程。

解决方案：

Given points are (-5, 0, 6), (-3, 10, -9) and (-2, 6, -6).

The equation of a plane passing through three points is given by 

$\begin{vmatrix} x-x_1&y-y_1&z-z_1\\ x_2-x_1&y_2-y_1&z_2-z_1\\ x_3-x_1&y_3-y_1&z_3-z_1\\ \end{vmatrix} = 0$

$\begin{vmatrix} x+5&y-0&z+6\\ -3+5&10-0&-9+6\\ -2+5&6-0&-6+6\\ \end{vmatrix} = 0$

$\begin{vmatrix} x+5&y&z+6\\ 2&10&-3\\ 3&6&0\\ \end{vmatrix} = 0$

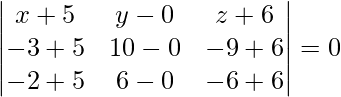
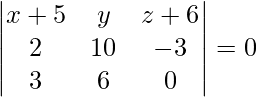
(x + 5)(0 + 18) – y(0 + 9) + (z + 6)(12 – 30) = 0

(x + 5)(18) – y(9) + (z + 6)(-18) = 0

18x + 90 – 9y – 18z -108 = 0

Taking 9 as common, we get equation

2x – y – 2z – 2 = 0

问题1(iii)。找到通过以下点(1、1、1)，(1 、、-1、2)和(-2，-2、2)的平面方程。

解决方案：

Given three points are (1, 1, 1), (1, -1, 2), and (-2, -2, 2)

The equation of plane passing through three points is given by 

$\begin{vmatrix} x-x_1&y-y_1&z-z_1\\ x_2-x_1&y_2-y_1&z_2-z_1\\ x_3-x_1&y_3-y_1&z_3-z_1\\ \end{vmatrix} = 0$

$\begin{vmatrix} x-1&y-1&z-1\\ 1-1&-1-1&2-1\\ -2-1&-2-1&2-1\\ \end{vmatrix} = 0$

$\begin{vmatrix} x-1&y-1&z-1\\ 0&-2&1\\ -3&-3&1\\ \end{vmatrix} = 0$

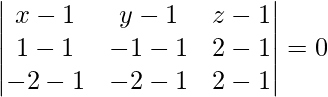
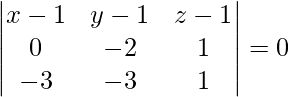
(x – 1)(-2 + 3) – (y – 1)(0 + 3) + (z – 1)(0 – 6) = 0

(x – 1)(1) – (y – 1)(3) + (z – 1)(-6) = 0 

x – 1 – 3y + 3 – 6z + 6 = 0

x -3y – 6z + 8 = 0

问题1(iv)。找到通过以下点(2、3、4)，(-3、5、1)和(4，-1、2)的平面方程。

解决方案：

Given points are (2, 3, 4), (-3, 5, 1), and (4, -1, 2).

The equation of plane passing through three points is given by 

$\begin{vmatrix} x-x_1&y-y_1&z-z_1\\ x_2-x_1&y_2-y_1&z_2-z_1\\ x_3-x_1&y_3-y_1&z_3-z_1\\ \end{vmatrix} = 0$

$\begin{vmatrix} x-2&y-3&z-4\\ -3-2&5-3&1-4\\ 4-2&-1-3&2-4\\ \end{vmatrix} = 0$

$\begin{vmatrix} x-2&y-3&z-4\\ -5&2&3\\ 2&-4&-2\\ \end{vmatrix} = 0$

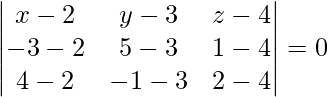
(x – 2)(-4 – 12) – (y – 3)(10 + 6) + (z – 4)(20 – 4) = 0

(x – 2)(-16) – (y – 3)(16) + (z – 4)(16) = 0

-16x – 32 – 16y + 48 + 16z – 64 = 0

-16x – 16y + 16z + 16 = 0

Taking -16 common we get equation of plane as,

x + y – z – 1 = 0

问题1(v)。找到通过以下点(0，-1、0)，(3、3、0)和(1、1、1)的平面方程。

解决方案：

Given points are (0, -1, 0), (3, 3, 0), and (1, 1, 1)

The equation of plane passing through three points is given by,

$\begin{vmatrix} x-x_1&y-y_1&z-z_1\\ x_2-x_1&y_2-y_1&z_2-z_1\\ x_3-x_1&y_3-y_1&z_3-z_1\\ \end{vmatrix} = 0$

$\begin{vmatrix} x-0&y+1&z-0\\ 3-0&3+1&0-0\\ 1-0&1+1&1-0\\ \end{vmatrix} = 0$

$\begin{vmatrix} x&y+1&z\\ 3&4&0\\ 1&2&1\\ \end{vmatrix} = 0$

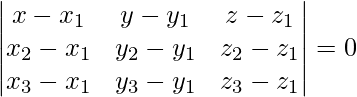
x(4 – 0) – (y + 1)(3 – 0) + z(6 – 4) = 0

4x – (y + 1)(3) + z(2) = 0

4x – 3y – 3 + 2z = 0

4x – 3y + 2z – 3 = 0

问题2。证明四个点(0，-1、1)，(4、5、1)，(3、9、4)和(-4、4、4)是共面的，并找到普通飞机。

解决方案：

To prove the given points (0, -1, 1), (4, 5, 1), (3, 9, 4), and (-4, 4, 4) are coplanar.

Efficient solution is to find the equation of plane passing through any three points. 

Then substitute the fourth point in the resultant equation. 

If it satisfies then the four points are coplanar.

Equation of plane passing through three points (0, -1, 1), (4, 5, 1), and (3, 9, 4) is given by

$\begin{vmatrix} x-x_1&y-y_1&z-z_1\\ x_2-x_1&y_2-y_1&z_2-z_1\\ x_3-x_1&y_3-y_1&z_3-z_1\\ \end{vmatrix} = 0$

$\begin{vmatrix} x-0&y+1&z+1\\ 4-0&5+1&1+1\\ 3-0&9+1&4+1\\ \end{vmatrix} = 0$

$\begin{vmatrix} x&y+1&z+1\\ 4&6&2\\ 3&10&5\\ \end{vmatrix} = 0$

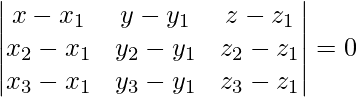
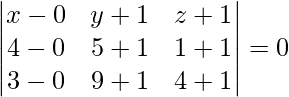
x(30 – 20) – (y + 1)(20 – 6) + (z + 1)(40 – 18) = 0

10x – (y + 1)(14) + (z + 1)(22) = 0

10x – 14y + 22z + 8 = 0

Taking 2 as common,

5x – 7y + 11z + 4 = 0         -Equation (1)

Substitute remaining point (-4, 4, 4) in above eq (1)

5(-4) – 7(4) + 11(4) + 4 = 0

-48 + 48 = 0

0 = 0

LHS = RHS

As the point satisfies the equation. So, the four points are coplanar.

Common plane equation is 5x – 7y + 11z + 4 = 0

问题3(i)。证明以下点是共面的(0，-1，0)，(2，1，-1)，(1，1，1)和(3，3，0)。

解决方案：

Given points are (0, -1, 0), (2, 1, -1), (1, 1, 1), and(3, 3, 0).

Equation of plane passing through three points (0, -1, 0), (2, 1, -1), (1, 1, 1) is given by 

$\begin{vmatrix} x-x_1&y-y_1&z-z_1\\ x_2-x_1&y_2-y_1&z_2-z_1\\ x_3-x_1&y_3-y_1&z_3-z_1\\ \end{vmatrix} = 0$

$\begin{vmatrix} x-0&y+1&z-0\\ 2-0&1+1&-1-0\\ 1-0&1+1&1-0\\ \end{vmatrix} = 0$

$\begin{vmatrix} x&y+1&z\\ 2&2&-1\\ 1&2&1\\ \end{vmatrix} = 0$

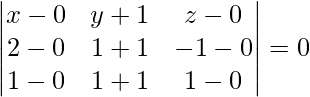
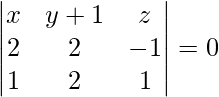
x(2 + 2) – (y + 1)(2 + 1) + z(4 – 2) = 0

x(4) – (y + 1)(3) + z(2) = 0

4x – 3y – 3 + 2z = 0

4x – 3y + 2z – 3 = 0          -Equation (1)

Substitute point four in equation (1)

4(3) – 3(3) + 2 (0) – 3 = 0

12 – 9 + 0 – 3 = 0

12 – 12 = 0

0 = 0

LHS = RHS

As fourth point satisfies the equation.

So, the four points are coplanar

问题3(ii)。证明以下点共面(0，4，3)，(-1，-5，-3)，(-2，-2，1)和(1，1，-1)。

解决方案：

Given four points are (0, 4, 3), (-1, -5, -3), (-2, -2, 1), and (1, 1, -1).

Equation of points passing through three points is given by 

$\begin{vmatrix} x-x_1&y-y_1&z-z_1\\ x_2-x_1&y_2-y_1&z_2-z_1\\ x_3-x_1&y_3-y_1&z_3-z_1\\ \end{vmatrix} = 0$

$\begin{vmatrix} x-0&y-4&z-3\\ -1-0&-5-4&-3-3\\ -2-0&-2-4&1-3\\ \end{vmatrix} = 0$

$\begin{vmatrix} x&y-4&z-3\\ -1&-9&-6\\ -2&-6&-2\\ \end{vmatrix} = 0$

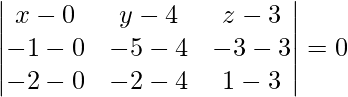
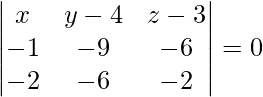
x(18 – 36) – (y – 4)(2 – 12) + (z – 3)(6 – 18) = 0

x(-18) – (y – 4)(-10) + (z – 3)(-12) = 0

-18x + 10y – 40 – 12z + 36 = 0

-18x + 10y – 12z – 4 = 0          -Equation (1)

Substitute fourth point in equation (1)

-18(1) + 10(1) – 12(-1) – 4 = 0

-18 +10 +12 -4 = 0

-22 + 22 = 0

0 = 0

LHS = RHS

As the fourth point satisfies the given equation. 

So, the four points are coplanar.