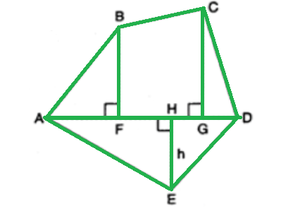
问题1.找到下图所示的五边形区域,如果AD = 10厘米,AG = 8厘米,AH = 6厘米,AF = 5厘米,BF = 5厘米,CG = 7厘米,EH = 3厘米。
解决方案:
Given:
AD = 10 cm, AG = 8 cm, AH = 6 cm, AF = 5 cm BF = 5 cm, CG = 7 cm, EH = 3 cm
From given data,
FG = AG – AF = 8 – 5 = 3 cm
And,
GD = AD – AG = 10 – 8 = 2 cm
From given figure:
Area of Pentagon = (Area of triangle AFB) + (Area of trapezium FBCG) +
(Area of triangle CGD) + (Area of triangle ADE)
= (0.5 x AF x BF) + [0.5 x (BF + CG) x (FG)] + (0.5 x GD x CG) + (1/2 x AD x EH).
= (0.5 x 5 x 5) + [0.5 x (5 + 7)x (3) + (0.5 x 2 x 7) + (0.5 x 10 x 3)
= 12.5 + 18 + 7 + 15 = 52.5 cm2
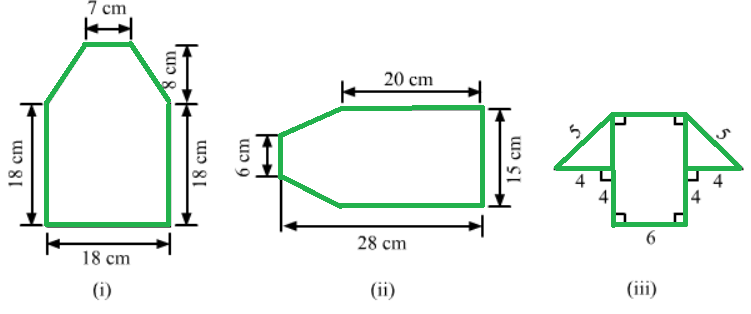
问题2.找到以下每个图(图(i)-(iii)J)所围成的面积,即矩形和梯形的面积之和。
解决方案:
(i) Figure can be divided into 2 parts a square and a trapezium as shown:
Area = (Area of square) + (Area of trapezium)
= (side)2 + (0.5 x (sum of parallel sides) x height
= (18 x 18) + 0.5 x (18 + 7) × (8)
= 324+100
= 424 cm2
(ii) Figure can be divided into 2 parts a rectangle and a trapezium as shown:
From the figure:
Height of trapezium = 28 – 20 = 8 cm
Area = (Area of rectangle) + (Area of trapezium)
= (length x breadth) + (0.5 x (sum of parallel sides) x height)
= (20 x 15) + [0.5 x (15 + 6) × (8)]
= 300 + 84
= 384 cm2
(iii) Figure can be divided into 2 parts one trapezium and one rectangle:
Pythagoras theorem in one of the right angle triangle:
52 = 42 + (base)2
base2 = 25 – 16
base = √9 = 3 cm
Therefore,
The height of trapezium = 3 cm
One side of trapezium = 6 + 4 + 6 = 14 cm
Area = (Area of rectangle) + (Area of trapezium)
= (length x breadth) + (0.5 x (sum of parallel sides) x height)
= (6 x 4) + (0.5 x (14 + 6) x (3))
= 24 + 30
= 54 cm2
问题3:有一个五边形的公园,如图。Jyoti和Kavita用两种不同的方式将其划分。

两种方法都可以找到这个公园的区域。您能建议其他找到其区域的方法吗?
解决方案:
Jyoti and Kavita divided the park in two different ways.
(i) Jyoti divided the park into two equal trapeziums:
From the figure the park divided into equal trapezium having height 7.5 m and sides 30 m and 15 m
Area of the park = 2 x (Area of a trapezium)
= 2 x (0.5 x (sum of parallel sides) x height)
= 2 x (0.5 x (30 + 15) x (7.5))
= 337.5 m2
(ii) Kavita divided the park into a rectangle and a triangle:
From diagram,
The height of the triangle = 30 – 15 = 15 m
Area of the park = (Area of square) + (Area of triangle)
= (15 x 15) + (0.5 x 15 x 15)
= 225 + 112.5
= 337.5 m2
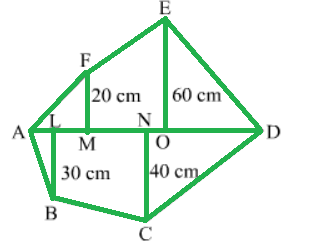
问题4.如果AL = 10 cm,AM = 20 cm,AN = 50 cm,AO = 60 cm和AD = 90 cm,则找到以下多边形的面积。
解决方案:
Given:
AL = 10 cm, AM = 20 cm, AN = 50 cm
AO = 60 cm, AD = 90 cm
From given data,
MO = AO – AM = 60 – 20 = 40 cm
OD = AD – A0 = 90 – 60 = 30 cm
ND = AD – AN = 90 – 50 = 40 cm
LN = AN – AL = 50 – 10 = 40 cm
Area of Polygon = (Area of triangle AMF) + (Area of trapezium MOEF) +
(Area of triangle DNC) + (Area of trapezium NLBC) +
(Area of triangle ALB)
= (0.5 x AM x MF) + [0.5 x (MF + OE) x OM] + (0.5 x OD x OE) +
(0.5 x DN x NC) + [0.5 x (LB + NC) x NL] + (0.5 x AL x LB)
= (0.5 x 20 x 20) + [0.5 x (20 + 60) x (40)] +(0.5 x 30 x 60) +
(0.5 x 40 x 40) +[0.5 x (30 + 40) x (40)] + (0.5 x 10 x 30)
= 200 + 1600 + 900 + 800 + 1400 +150 = 5050 cm2
问题5.找到以下正六边形的面积。

解决方案:
As it is a regular Hexagon so all sides are 13 cm and AN = BQ
From the figure,
As the diagonal QN is 23 cm,
QB + BA + AN = QN
AN + 13 + AN = 23
2AN = 23 – 13 = 10
AN = 5 cm
Hence, AN = BQ = 5 cm
Applying Pythagoras theorem in triangle MAN:
MN2 = AN2 + AM2
169 = 25 + AM2
AM² = 169 – 25
AM = √144
AM = 12cm
From figure,
OM = RP = 2 × AM = 2 x 12 = 24 cm
This Hexagon can be divided into 3 parts 2 triangles and one rectangle therefore,
Area of the regular hexagon = (area of triangle MON) + (area of rectangle MOPR) +
(area of triangle RPQ)
= (0.5 x OM x AN) + (RP X PO) + (0.5 x RP x BQ)
= (0.5 x 24 x 5) + (24 x 13) + (0.5 x 24 x 5)
= 60 + 312 + 60
= 432 cm2