通用和中间术语–二项式定理– 11类数学

二项式定理或展开描述了二项式的幂的代数展开。根据该定理，可以将多项式“ (a + b) ^{n ”}扩展为包含形式为“ ax ^z y ^{c ”的项的和}，指数z和c是非负整数，其中z + c = n，每个项的系数是一个正整数，取决于n和b的值。

Example: If n = 4

(a + b)⁴ = a⁴ + 4a³y + 6a²b² + 4ab³ + b

二项式展开式的总称

^{(x + y)n}的二项式展开式的一般项如下

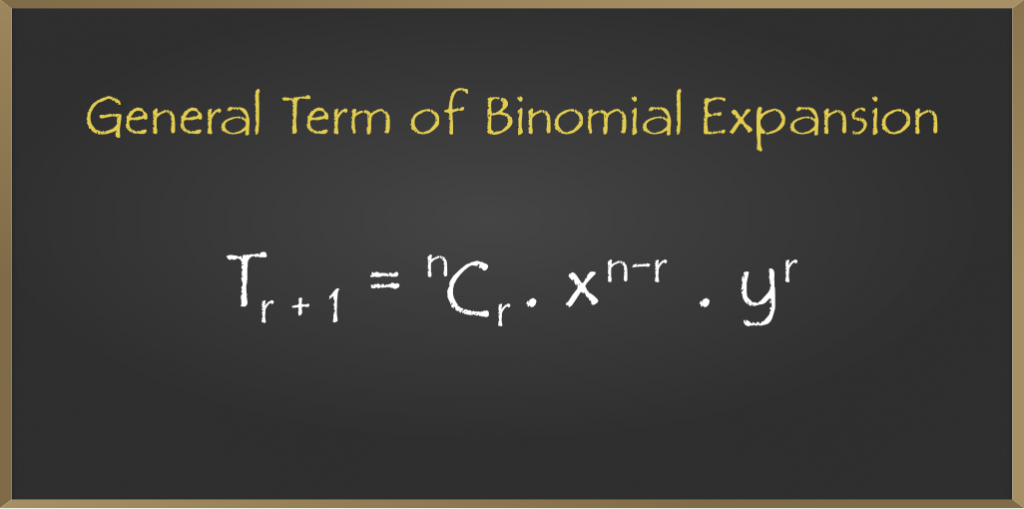
• T _{r + 1}是二项式展开式中的通用项
• 通用术语扩展用于查找上式中提到的术语。
• 为了找到二项式展开式中的术语，我们需要展开给定的展开式。
• 假设(a + b) ⁿ是等式，则其二项式展开式的级数如下：

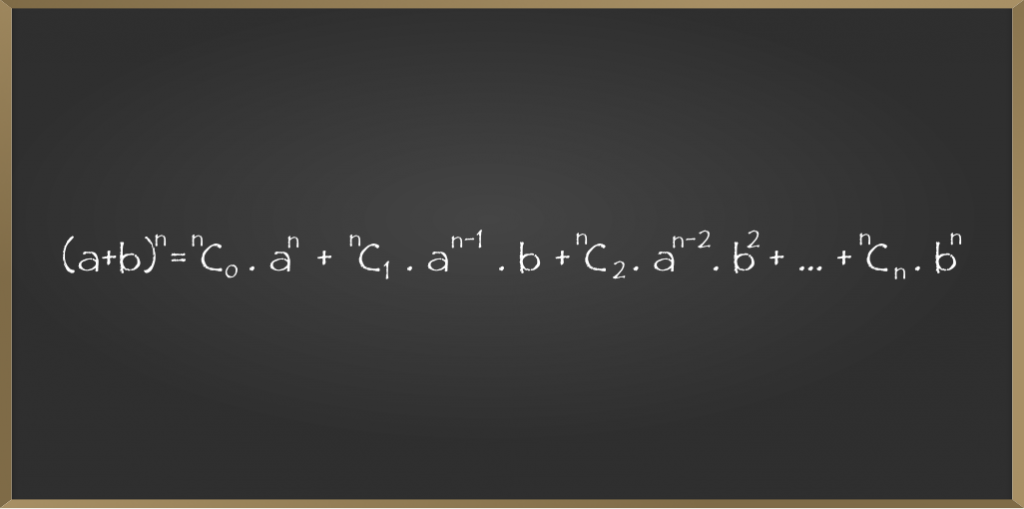
• 该系列的第一项是T ₁ = ⁿ C ₀ .a ⁿ
• 该系列的第二项是T ₂ = ⁿ C ₁ .a ^n-1 .b
• 该系列的第三项是T ₃ = ⁿ C ₂ .a ^n-2 .b ²
• 该级数的^第n个项是T _n = ⁿ C _n .b ⁿ

一般术语示例问题

示例1：查找给定二项式展开(x + 2y)的^第(r + 1)^个项5

解决方案：

Given expansion is (x + 2y)⁵

a = x, b= 2y, n = 5

The formula for (r+1)^th is  ⁿC_r .a^{n – r}.b^r

(r+1)^th term =⁵C_r.x^{5 -r}.2y^r

示例2：查找给定二项式展开式(a + 2b)的^第(r + 1)^个项7

解决方案：

Given expansion is (a + 2b)⁷

a = a, b = 2b, n = 7

The formula for (r+1)th is  ⁿC_r .a^{n – r}.b^r

(r+1)^th term = ⁷C_r.a^{7 -r}.b^r

示例3：查找给定二项式展开式(6p + 2q)的^第(r + 1)^个项12

解决方案：

Given expansion is  (6p + 2q)

a = 6p, b = 2q, n = 12

The formula for (r+1)^th is  ⁿC_r .a^{n – r}.b^r

(r + 1)^th term = ¹²C_r.6p^{12 -r}.2q^r

二项式展开式的中期

如果(x + y) ⁿ = ⁿ C _r .x ^{n – r。 r}   ，它具有(n +1)个项，中间项将取决于n的值。

对于二项式展开的中期，我们有两种情况：

如果n是偶数

如果n是偶数，则将其设为奇数，并考虑(n + 1)为奇数，而(n / 2 +1)为中间项。简单来说，如果n为偶数，则我们将其视为奇数。

假设n为偶数，则(n + 1)为奇数。找出中间项：

考虑二项式展开式的总称，即

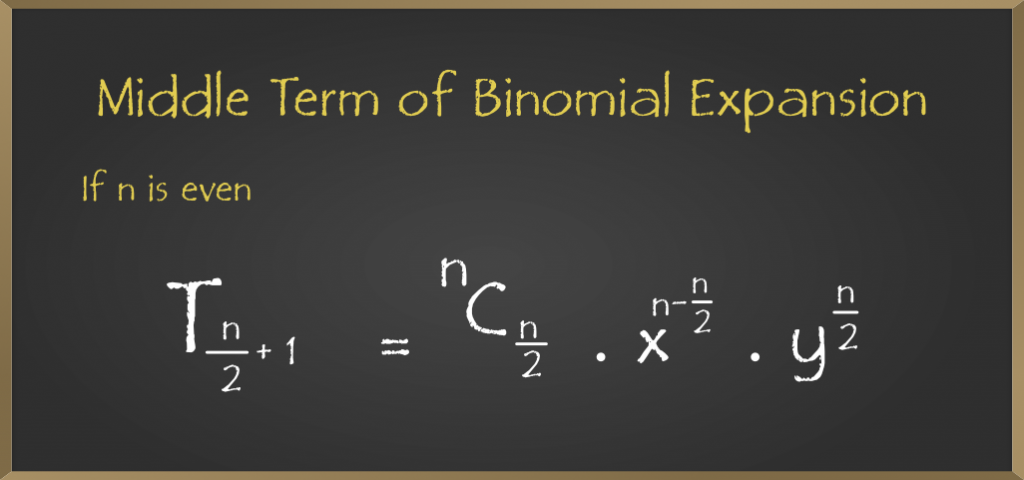
• 现在我们在上面的方程式中用“ n / 2”替换“ r”以找到中间项
• T _{r + 1} = T _{n / 2} + 1
• T _{n / 2 +1} = ⁿ C _{n / 2} .x ^{n – n / 2} .y ^{n / 2}

中间术语示例问题

示例1：找到以下二项式展开式的中间值(x + a) ⁸

解决方案：

Given expansion is (x + a)⁸

n = 8, we consider the expansion has (n + 1) terms so the above expansion has (8 + 1) i.e 9 terms 

we have T₁, T₂, T₃, T₄, T₅, T₆ , T₇, T₈, T₉.

T_r+1 = T_{n/2 + 1} = ⁿC_n/2.x^{n – n/2}.Y^n/2

T_{8/2 + 1} = ⁸C_8/2.x^8-8/2.a^8/2

T₅ = ⁸C₄.x⁴.a⁴ is the required middle term of the given binomial expansion.

示例2：找到以下二项式展开式的中间值(x + 3y) ⁶

解决方案：

Given expansion is (x + 3y)⁶

n = 6, we consider the expansion has (n + 1) terms so the above expansion has (6 + 1) i.e 7 terms

we have T₁, T₂, T₃, T₄, T₅, T₆ , T₇.

T_r+1 = T_{n/2 + 1} = ⁿC_n/2.x^{n – n/2}.Y^n/2

T_{6/2 + 1} = ⁶C_6/2.x^6-6/2.3y^6/2

T₄ = ⁶C₃.x³.3y³ is the required middle term of the given binomial expansion.

示例3：找到以下二项式展开式的中间值(2x + 5y) ⁴

解决方案：

Given expansion is  (2x – 5y)⁴

n = 4, we consider the expansion has (n + 1) terms so the above expansion has (4 + 1) i.e 5 terms

we have T₁, T₂, T₃, T₄, T₅.

T_r+1 = T_{n/2 + 1} = ⁿC_n/2.x^{n – n/2}.Y^n/2

T_{4/2 + 1} = ⁴C_4/2.2x^4-4/2.5y^4/2

T₃ = ⁴C₂.x².5y² is the required middle term of the given binomial expansion.

如果n为奇数

如果n是奇数，则将其设为偶数，并考虑将(n + 1)视为偶数，并将(n + 1/2)，(n + 3/2)是中间项。简单来说，如果n为奇数，则我们将其视为偶数。

如果n为奇数，我们有两个中间项。查找中间项：

考虑二项式展开式的总称，即

• 在这种情况下，我们将“ r”替换为两个不同的值
• 一个项是(n + 1/2)，而我们得到的(r + 1)个项

r + 1 = n + 1/2

r = n + 1/2 -1

r = n -1/2

• 第二个中期，将(r + 1)与(n + 3/2)进行比较

r +1 = n +3/2

r = n + 3/2 – 1

r = n + 1/2

当n为奇数时，两个中间项是(n – 1/2)和(n + 1/2) 。

中间术语示例问题

示例1：找到以下二项式展开式(x + a)的中间项⁹

解决方案：

Given expansion is (x + a)⁹

a = x, b = a, and n = 9

Middle terms will be (n – 1)/2 and (n + 1)/2

T_{r + 1} =T _{n – 1/2} and T_{n + 1/2}

First middle term:

T_{r + 1} = T_{n – 1/2} = ⁹C_{(n – 1)/2}.x^{9 – (n – 1)/2}.a^{(n – 1)/2}

T_{(9 – 1/2)} = ⁹C_{(9 – 1)/2}.x^{9 – (n – 1)/2}.a^{(n – 1)/2}

T₄ = ⁹C₄.x⁵.a⁴ 

Second middle term: 

T_{r + 1} = T_{n + 1/2} =  ⁹C_{(n + 1)/2}.x^{9 – (n + 1)/2}.a^{(n + 1)/2}

T_{(9 + 1)/2} = ⁹C_{(9 + 1)/2}.x^{9 – (9 + 1)/2}.a^{(9 + 1)/2}

T₅ = ⁹C₅.x⁴.a⁵

The middle terms of expansion are T₄, and T_5.

示例2：找到以下二项式展开式(4a + 9b)的中间项⁷

解决方案：

Given expansion is (4a + 9b)⁷

a = 4a,b = 9b, and n = 7

middle terms will be (n -1/2) and (n + 1/2) 

T_{r + 1} =T _{n – 1/2} and T_{n + 1/2}

First middle term:

T_{r + 1} = T_{n – 1/2} = ⁷C_{(n – 1)/2}.4a^{7 – (n – 1)/2}.9b^{(n – 1)/2}

T_{(7 – 1/2)} = ⁷C_{(7 – 1)/2}.4a^{7 – (7 – 1)/2}.9b^{(7 – 1)/2}

T₃ = ⁷C₃.4a⁴.9b³

Second middle term:

T_{r + 1} = T_{(n + 1)/2} =  ⁷C_{(n + 1)/2}.4a^{7 – (n + 1)/2}.9b^{(n + 1)/2}

T_{(7 + 1)/2} =  ⁷C_{(7 + 1)/2}.4a^{7 – (7 + 1)/2}.9b^{(7 + 1)/2}

T₄ = ⁷C₄.4a³.9b⁴

The middle terms of expansion are T₃, and T₄.

示例3：找到以下二项式展开式(2x + 8y)的中间项⁵

解决方案：

Given expansion is (2x + 8y)⁵

a = 2x,b = 8y, and n = 5

Middle terms will be (n – 1)/2 and (n + 1)/2 

T_{r + 1} = T _{(n – 1)/2} and T_{(n + 1)/2}

First middle term:

T_{r + 1} = T_{(n – 1)/2} = ⁵C_{(n – 1)/2}.2x^{5 – (n – 1)/2}.8y^{(n – 1)/2}

T_{(5 – 1)/2} = ⁵C_{(5 – 1)/2}.2x^{5 – (5 – 1)/2}.8y^{(5 – 1)/2}

T₂ = ⁵C₂.2x³.8y²

Second middle term:

T_{r + 1} = T_{(n + 1)/2} =  ⁵C_{(n + 1)/2}.2x^{5 – (n + 1)/2}.8y^{(n + 1)/2}

T_{(5 + 1)/2} =  ⁵C_{(5 + 1)/2}.2x^{5 – (5 + 1)/2}.8y^{(5 + 1)/2}

T₃ = ⁵C₃.2x².8y³

The middle terms of expansion are T₂, and T₃.