第 12 类 RD Sharma 解决方案 - 第 14 章微分、误差和近似值 - 练习 14.1 |设置 2
问题 9:使用微分,找到以下的近似值:
(十四) 
解决方案:
Considering the function as
y = f(x) = cos x
Taking x = π/3, and
x+△x = 11π/36
△x = 11π/36-π/3 = -π/36
y = cos x
![]() = cos (π/3) = 0.5
= cos (π/3) = 0.5
![]() = – sin x
= – sin x
![]() = – sin (π/3) = -0.86603
= – sin (π/3) = -0.86603
△y = dy = ![]() dx
dx
△y = (-0.86603) (-π/36)
△y = 0.0756
Hence, ![]() = 0.5+0.0756 = 0.5755
= 0.5+0.0756 = 0.5755
(十五) 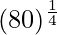
解决方案:
Considering the function as
y = f(x) = ![]()
Taking x = 81, and
x+△x = 80
△x = 80-81 = -1
![]()
![]()
![]()
![]()
△y = dy = ![]() dx
dx
△y = ![]() △x
△x
△y = ![]() (-1) =
(-1) = ![]() = -0.009259
= -0.009259
Hence,
![]() = y+△y = 3 + (-0.009259) = 2.99074
= y+△y = 3 + (-0.009259) = 2.99074
(十六) 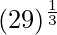
解决方案:
Considering the function as
y = f(x) = ![]()
Taking x = 27, and
x+△x = 29
△x = 29-27 = 2
![]()
![]()
![]()
![]()
△y = dy = ![]() dx
dx
△y = ![]() △x
△x
△y = ![]() (2) = 0.074
(2) = 0.074
Hence,
![]() = y+△y = 3+0.074 = 3.074
= y+△y = 3+0.074 = 3.074
(十七) 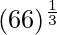
解决方案:
Considering the function as
y = f(x) = ![]()
Taking x = 64, and
x+△x = 66
△x = 66-64 = 2
![]()
![]()
![]()
![]()
△y = dy = ![]() dx
dx
△y = ![]() △x
△x
△y = ![]() (2) = 0.042
(2) = 0.042
Hence,
![]() = y+△y = 4+0.042 = 4.042
= y+△y = 4+0.042 = 4.042
(十八) 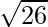
解决方案:
Considering the function as
y = f(x) = ![]()
Taking x = 25, and
x+△x = 26
△x = 26-25 = 1
![]()
![]()
![]()
![]()
△y = dy = ![]() dx
dx
△y = ![]() △x
△x
△y = ![]() (1) = 0.1
(1) = 0.1
Hence,
![]() = y+△y = 5 + 0.1 = 5.1
= y+△y = 5 + 0.1 = 5.1
(十九) 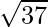
解决方案:
Considering the function as
y = f(x) = ![]()
Taking x = 36, and
x+△x = 37
△x = 37-36 = 1
![]()
![]()
![]()
![]()
△y = dy = ![]() dx
dx
△y = ![]() △x
△x
△y = ![]() (1) = 0.0833
(1) = 0.0833
Hence,
![]() = y+△y = 6 + 0.0833 = 6.0833
= y+△y = 6 + 0.0833 = 6.0833
(二十) 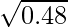
解决方案:
Considering the function as
y = f(x) = ![]()
Taking x = 0.49, and
x+△x = 0.48
△x = 0.48-0.49 = -0.01
![]()
![]()
![]()
![]()
△y = dy = ![]() dx
dx
△y = ![]() △x
△x
△y = ![]() (-0.01) = -0.007143
(-0.01) = -0.007143
Hence,
![]() = y+△y = 0.7 + (-0.007143) = 0.693
= y+△y = 0.7 + (-0.007143) = 0.693
(二十一) 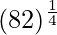
解决方案:
Considering the function as
y = f(x) = ![]()
Taking x = 81, and
x+△x = 82
△x = 82-81 = 1
![]()
![]()
![]()
![]()
△y = dy = ![]() dx
dx
△y =![]() △x
△x
△y = ![]() = 0.009259
= 0.009259
Hence,
![]() = y+△y = 3 + 0.009259 = 3.009259
= y+△y = 3 + 0.009259 = 3.009259
(二十二) 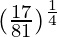
解决方案:
Considering the function as
y = f(x) = ![]()
Taking x = 16/81, and
x+△x = 17/81
△x = 17/81-16/81 = 1/81
![]()
![]()
![]()
![]()
△y = dy = ![]() dx
dx
△y = ![]() △x
△x
△y = ![]() = 0.01042
= 0.01042
Hence,
![]() = y+△y = 2/3 + 0.01042 = 0.6771
= y+△y = 2/3 + 0.01042 = 0.6771
(二十三) 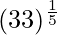
解决方案:
Considering the function as
y = f(x) = ![]()
Taking x = 32, and
x+△x = 33
△x = 33-32 = 1
![]()
![]()
![]()
![]()
△y = dy = ![]() dx
dx
△y = ![]() △x
△x
△y = ![]() = 0.0125
= 0.0125
Hence,
![]() = y+△y = 2 + 0.0125 = 2.0125
= y+△y = 2 + 0.0125 = 2.0125
(二十四) 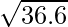
解决方案:
Considering the function as
y = f(x) = ![]()
Taking x = 36, and
x+△x = 36.6
△x = 36.6-36 = 0.6
![]()
![]()
![]()
![]()
△y = dy = ![]() dx
dx
△y = ![]() △x
△x
△y = ![]() (0.6) = 0.05
(0.6) = 0.05
Hence,
![]() = y+△y = 6 + 0.05 = 6.05
= y+△y = 6 + 0.05 = 6.05
(二十五) 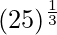
解决方案:
Considering the function as
y = f(x) = ![]()
Taking x = 27, and
x+△x = 25
△x = 25-27 = -2
![]()
![]()
![]()
![]()
△y = dy = ![]() dx
dx
△y = ![]() △x
△x
△y = ![]() (-2) = -0.07407
(-2) = -0.07407
Hence,
![]() = y+△y = 3+(-0.07407) = 2.9259
= y+△y = 3+(-0.07407) = 2.9259
(二十六) 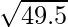
解决方案:
Considering the function as
y = f(x) = ![]()
Taking x = 49, and
x+△x = 49.5
△x = 49.5-49 = 0.5
![]()
![]()
![]()
![]()
△y = dy = ![]() dx
dx
△y = ![]() △x
△x
△y = ![]() (0.5) = 0.0357
(0.5) = 0.0357
Hence,
![]() = y+△y = 7 + 0.0357 = 7.0357
= y+△y = 7 + 0.0357 = 7.0357
问题 10:找到 f(2.01) 的适当值,其中 f(x) = 4x 2 +5x+2
解决方案:
Considering the function as
y = f(x) = 4x2+5x+2
Taking x = 2, and
x+△x = 2.01
△x = 2.01-2 = 0.01
y = 4x2+5x+2
![]() = 4(2)2+5(2)+2 = 28
= 4(2)2+5(2)+2 = 28
![]() = 8x+5
= 8x+5
![]() = 8(2)+5 = 21
= 8(2)+5 = 21
△y = dy = ![]() dx
dx
△y = (21) △x
△y = (21) (0.01) = 0.21
Hence,
f(2.01) = y+△y = 28 + 0.21 = 28.21
问题 11:找到 f(5.001) 的适当值,其中 f(x) = x 3 -7x 2 +15
解决方案:
Considering the function as
y = f(x) = x3-7x2+15
Taking x = 5, and
x+△x = 5.001
△x =5.001-5 = 0.001
y = x3-7x2+15
![]() = (5)3-7(5)2+15 = -35
= (5)3-7(5)2+15 = -35
![]() = 3x2-14x
= 3x2-14x
![]() = 3(5)2-14(5) = 5
= 3(5)2-14(5) = 5
△y = dy = ![]() dx
dx
△y = (5) △x
△y = (5) (0.001) = 0.005
Hence,
f(5.001) = y+△y = -35 + 0.005 = -34.995
问题 12:给定 log 10 e=0.4343,求 log 10 1005 的合适值
解决方案:
Considering the function as
y = f(x) = log10 x
Taking x = 1000, and
x+△x = 1005
△x =1005-1000 = 5
y = log10 x = ![]()
![]() = log10 1000 = 3
= log10 1000 = 3
![]()
![]() = 0.0004343
= 0.0004343
△y = dy = ![]() dx
dx
△y = (0.0004343) △x
△y = (0.0004343) (5) = 0.0021715
Hence, log10 1005 = y+△y = 3 + 0.0021715 = 3.0021715
问题13:如果一个球体的半径测量为9cm,误差为0.03m,求其表面积的近似误差。
解决方案:
According to the given condition,
As, Surface area = 4πx2
Let △x be the change in the radius and △y be the change in the surface area
x = 9
△x = 0.03m = 3cm
x+△x = 9+3 = 12cm
![]() = 4πx2 = 4π(9)2 = 324 π
= 4πx2 = 4π(9)2 = 324 π
![]() = 8πx
= 8πx
![]() = 8π(9) = 72π
= 8π(9) = 72π
△y = dy = ![]() dx
dx
△y = (72π) △x
△y = (72π) (3) = 216 π
Hence, approximate error in surface area of the sphere is 216 π cm2
问题 14:求边长减少 1% 时立方体表面积的近似变化(边 x 米)。
解决方案:
According to the given condition,
As, Surface area = 6x2
Let △x be the change in the length and △y be the change in the surface area
△x/x × 100 = 1
![]() = 6(2x) = 12x
= 6(2x) = 12x
△y = ![]() △x
△x
△y = (12x) (x/100)
△y = 0.12 x2
Hence, the approximate change in the surface area of a cubical box is 0.12 x2 m2
问题15:如果一个球体的半径测量为7m,误差为0.02m,求其体积的近似误差。
解决方案:
According to the given condition,
As, Volume of sphere = πx3
Let △x be the error in the radius and △y be the error in the volume
x = 7
△x = 0.02 cm
![]() π(3x2) = 4πx2
π(3x2) = 4πx2
![]() = 4π(7)2 = 196π
= 4π(7)2 = 196π
△y = dy = ![]() dx
dx
△y = (196π) △x
△y = (196π) (0.02) = 3.92 π
Hence, approximate error in volume of the sphere is 3.92 π cm2
问题 16:求边长增加 1% 时立方体体积的近似变化(边 x 米)。
解决方案:
According to the given condition,
As, Volume of cube = x3
Let △x be the change in the length and △y be the change in the volume
△x/x × 100 = 1
![]() = 3x2
= 3x2
△y = ![]() △x
△x
△y = (3x2) (x/100)
△y = 0.03 x3
Hence, the approximate change in the volume of a cubical box is 0.03 x3 m3