第 12 类 RD Sharma 解决方案 - 第 14 章微分、误差和近似值 - 练习 14.1 |设置 1

问题 1：如果 y=sin x 并且 x 从 π/2 变为 22/14，那么 y 的近似变化是多少？

解决方案：

According to the given condition,

x = π/2, and 

x+△x = 22/14

△x = 22/14-x = 22/14 – π/2

As, y = sin x

$\frac{dy}{dx}$= cos x

$(\frac{dy}{dx})_{x=\frac{\pi}{2}}$ = cos (π/2) = 0

△y = $(\frac{dy}{dx})_{x=\frac{\pi}{2}}$ △x

△y = 0 △x

△y = 0 (22/14 – π/2)

△y = 0

Hence, there will be no change in y.

问题 2：球体的半径从 10 厘米缩小到 9.8 厘米。大约找到它的体积减少？

解决方案：

According to the given condition,

Let’s take radius as x

x = 10, and

Let △x be the error in the radius and △y be the error in the volume

x+△x = 9.8

△x = 9.8-x = 9.8-10 = -0.2

As, Volume of sphere = $\frac{4}{3}\pi x^3$

$\frac{dy}{dx} = \frac{4}{3}(3 \pi x^2)$ = 4πx²

$(\frac{dy}{dx})_{x=10}$= 4π(10)² = 400 π

△y = $(\frac{dy}{dx})_{x=10}$ △x

△y = (400 π) (-0.2)

△y = -80 π

Hence, approximate decrease in its volume will be -80 π cm³

问题3：圆形金属板受热膨胀，其半径增加k%。如果加热前板的半径为 10 厘米，则求板面积的近似增加。

解决方案：

According to the given condition,

Let’s take radius as x

x = 10, and

Let △x be the error in the radius and △y be the error in the surface area

△x/x × 100 = k

△x = (k × 10)/100 = k/10

As, Area of circular metal = πx²

$\frac{dy}{dx}$ = π(2x) = 2πx

$(\frac{dy}{dx})_{x=10}$ = 2π(10) = 20 π

△y = $(\frac{dy}{dx})_{x=10}$ △x

△y = (20 π) (k/10)

△y = 2kπ

Hence, approximate increase in the area of the plate is 2kπ cm²

问题 4：如果在测量立方体的边长时出现 1% 的误差，求立方体表面积的百分比误差。

解决方案：

According to the given condition,

Let △x be the error in the length and △y be the error in the surface area

Let’s take length as x

△x/x × 100 = 1

△x = x/100

x+△x = x+(x/100)

As, surface area of the cube = 6x²

$\frac{dy}{dx}$ = 6(2x) = 12x

△y = $(\frac{dy}{dx})$ △x

△y = (12x) (x/100)

△y = 0.12 x²

So, △y/y = 0.12 x²/6 x² = 0.02

Percentage change in y = △y/y × 100 = 0.02 × 100 = 2

Hence, the percentage error in calculating the surface area of a cubical box is 2%

问题5：如果球体半径的测量有0.1%的误差，求球体体积计算的近似百分比误差。

解决方案：

According to the given condition,

As, Volume of sphere = $\frac{4}{3}\pi x^3$

Let △x be the error in the radius and △y be the error in the volume

△x/x × 100 = 0.1

△x/x = 1/1000

As, y = $\frac{4}{3}\pi x^3$

$\frac{dy}{dx} = \frac{4}{3}(3 \pi x^2)$ = 4πx²

dy = 4πx² dx

△y = (4πx²) △x

Change in volume,

△y/y = 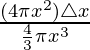$\frac{(4\pi x^2) \triangle x}{\frac{4}{3}\pi x^3}$

△y/y = $\frac{3}{x} \triangle x$

△y/y = $3(\frac{\triangle x}{x} )$ = 3(0.001) = 0.003

Percentage change in y = △y/y × 100 = 0.003 × 100 = 0.3

Hence, approximately the percentage error in the calculation of the volume of the sphere is 0.3%

问题 6：气体的压力 p 和体积 v 通过 pv ^1.4 = 常数的关系联系起来。求 p 中对应于 v 减少 1/2% 的百分比误差。

解决方案：

According to the given condition,

$\frac{\triangle v}{v} \times 100$ = – 1/2%

pv^1.4 = constant = k(say)

Taking log on both sides, we get

log(pv^1.4) = log (k)

log(p)+log(v^1.4) = log k

log(p) + 1.4 log(v) = log k

Differentiating wrt v, we get

$\frac{1}{p} \frac{dp}{dv} + 1.4 (\frac{1}{p}) = 0$

$\frac{dp}{p} = \frac{-1.4 dv}{v}$

Percentage change in p = △p/p × 100 = $\frac{-1.4 \triangle v}{v}$ × 100 = -1.4 $(\frac{\triangle v}{v} \times 100)$

= -1.4 $(\frac{-1}{2})$

= 0.7 %

Hence, percentage error in p is 0.7%.

问题7：圆锥的高度增加了k%，它的半垂直角保持不变。大约增加的百分比是多少？

解决方案：

According to the given condition,

Let h be the height, y be the surface area. V be the volume, l be the slant height and r be the radius of the cone.

Let △h be the change in the height. △r be the change in the radius of base and △l be the change in slant height.

Semi-vertical angle remaining the same.

△h/h = △r/r = △l/l

and,

△h/h × 100 = k

△h/h × 100 = △r/r × 100 = △l/l × 100 = k

(i) 总表面积，和

解决方案：

Total surface area of the cone

y = πrl + πr²

Differentiating both the sides wrt r, we get

$\frac{dy}{dr}$ = πl + πr $\frac{dl}{dr}$ + 2πr

$\frac{dy}{dr}$ = πl + πr $\frac{l}{r}$ + 2πr

$\frac{dy}{dr}$ = πl + πl + 2πr

$\frac{dy}{dr}$ = 2πl + 2πr = 2π(r+l)

△y = $(\frac{dy}{dr})$ △r

△y = (2π(r+l)) $(\frac{kr}{100}) = \frac{2\pi kr(r+l)}{100}$

Percentage change in y = △y/y × 100 = 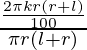$\frac{\frac{2\pi kr(r+l)}{100}}{\pi r(l+r)}$ × 100 

= 2k %

Hence, percentage increase in total surface area of cone 2k%.

(ii) 在体积中假设 k 很小？

解决方案：

Volume of cone (y) = $\frac{1}{3}\pi r^2h$

Differentiating both the sides wrt h, we get

$\frac{dy}{dh} = \frac{1}{3}\pi$ (r² + h(2r $\frac{dr}{dh}$)

$\frac{dy}{dh} = \frac{1}{3}\pi$(r² + h(2r $\frac{r}{h}$)

$\frac{dy}{dh} = \frac{1}{3}\pi$ (r² + 2r²)

$\frac{dy}{dh}$ = πr²

△y = $(\frac{dy}{dh})$ △h

△y = (πr²) $(\frac{kh}{100}) = \frac{kh \pi r^2}{100}$

Percentage change in y = △y/y × 100 = 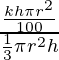$\frac{\frac{kh \pi r^2}{100}}{\frac{1}{3}\pi r^2h}$ × 100

= 3k %

Hence, percentage increase in the volume of cone 3k%.

问题8：证明计算球体体积的相对误差，由于测量半径的误差，大约等于半径相对误差的三倍。

解决方案：

According to the given condition,

Let △x be the error in the radius and △y be the error in volume.

Volume of cone (y) = $\frac{4}{3}\pi x^3$

Differentiating both the sides wrt x, we get

$\frac{dy}{dx} = \frac{4}{3}\pi$ (3x²)

$\frac{dy}{dx}$ = 4πx²

△y = $(\frac{dy}{dx})$ △x

△y = (4πx²) (△x)

△y/y = $\frac{(4 \pi x^2) (\triangle x)}{\frac{4}{3}\pi x^3}$

△y/y = $3 \frac{\triangle x}{x}$

Hence proved!!

问题 9：使用微分，找到以下的近似值：

（一世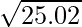） $\sqrt{25.02}$

解决方案：

Considering the function as

y = f(x) = $\sqrt{x}$

Taking x = 25, and

x+△x = 25.02

△x = 25.02-25 = 0.2

$y = \sqrt{x}$

$y_{(x=25)} = \sqrt{25} = 5$

$\frac{dy}{dx} = \frac{1}{2\sqrt{x}}$

$(\frac{dy}{dx})_{x=25} = \frac{1}{2\sqrt{25}} = \frac{1}{10}$

△y = dy = $(\frac{dy}{dx})_{x=25}$ dx

△y = $(\frac{1}{10})$ △x

△y = $(\frac{1}{10})$ (0.02) = 0.002

Hence, $\sqrt{25.02}$ = y+△y = 5 + 0.002 = 5.002

(二) $(0.009)^{\frac{1}{3}}$

解决方案：

Considering the function as

y = f(x) = $(x)^{\frac{1}{3}}$

Taking x = 0.008, and

x+△x = 0.009

△x = 0.009-0.008 = 0.001

$y = (x)^{\frac{1}{3}}$

$y_{(x=0.008)} = (0.008)^{\frac{1}{3}} = 0.2$

$\frac{dy}{dx} = \frac{1}{3(x)^{\frac{2}{3}}}$

$(\frac{dy}{dx})_{x=0.008} = \frac{1}{3(0.008)^{\frac{2}{3}}} = \frac{1}{0.12}$

△y = dy = $(\frac{dy}{dx})_{x=0.008}$ dx

△y = $(\frac{1}{0.12})$ △x

△y = $(\frac{1}{0.12})$ (0.001) = $\frac{1}{120}$ = 0.008333

Hence, $(0.009)^{\frac{1}{3}}$ = y+△y = 0.2 + 0.008333 = 0.208333

㈢ 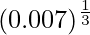$(0.007)^{\frac{1}{3}}$

解决方案：

Considering the function as

y = f(x) = $(x)^{\frac{1}{3}}$

Taking x = 0.008, and

x+△x = 0.007

△x = 0.007-0.008 = -0.001

$y = (x)^{\frac{1}{3}}$

$y_{(x=0.008)} = (0.008)^{\frac{1}{3}}$ = 0.2

$\frac{dy}{dx} = \frac{1}{3(x)^{\frac{2}{3}}}$

$(\frac{dy}{dx})_{x=0.008} = \frac{1}{3(0.008)^{\frac{2}{3}}} = \frac{1}{0.12}$

△y = dy = $(\frac{dy}{dx})_{x=0.008}$ dx

△y = $(\frac{1}{0.12})$ △x

△y = $(\frac{1}{0.12})$ (-0.001) = $\frac{-1}{120}$ = -0.008333

Hence, $(0.007)^{\frac{1}{3}}$ = y+△y = 0.2 + (-0.008333) = 0.191667

(四) 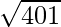$\sqrt{401}$

解决方案：

Considering the function as

y = f(x) = $\sqrt{x}$

Taking x = 400, and

x+△x = 401

△x = 401-400 = 1

$y = \sqrt{x}$

$y_{(x=400)} = \sqrt{400} = 20$

$\frac{dy}{dx} = \frac{1}{2\sqrt{x}}$

$(\frac{dy}{dx})_{x=400} = \frac{1}{2\sqrt{400}} = \frac{1}{40}$

△y = dy = $(\frac{dy}{dx})_{x=400}$ dx

△y = $(\frac{1}{40})$ △x

△y = $(\frac{1}{40})$ (1) = 0.025

Hence, $\sqrt{401}$ = y+△y = 20 + 0.025 = 20.025

(五) $(15)^{\frac{1}{4}}$

解决方案：

Considering the function as

y = f(x) = $(x)^{\frac{1}{4}}$

Taking x = 16, and

x+△x = 15

△x = 15-16 = -1

$y = (x)^{\frac{1}{4}}$

$y_{(x=16)} = (16)^{\frac{1}{4}} = 2$

$\frac{dy}{dx} = \frac{1}{4(x)^{\frac{3}{4}}}$

$(\frac{dy}{dx})_{x=16} = \frac{1}{4(16)^{\frac{3}{4}}} = \frac{1}{32}$

△y = dy = $(\frac{dy}{dx})_{x=16}$ dx

△y = $(\frac{1}{32})$ △x

△y = $(\frac{1}{32})$ (-1) = $\frac{-1}{32}$ = -0.03125

Hence, $(15)^{\frac{1}{4}}$ = y+△y = 0.2 + (-0.03125) = 1.96875

(六) 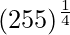$(255)^{\frac{1}{4}}$

解决方案：

Considering the function as

y = f(x) = $(x)^{\frac{1}{4}}$

Taking x = 256, and

x+△x = 255

△x = 255-256 = -1

$y = (x)^{\frac{1}{4}}$

$y_{(x=256)} = (256)^{\frac{1}{4}}$ = 4

$\frac{dy}{dx} = \frac{1}{4(x)^{\frac{3}{4}}}$

$(\frac{dy}{dx})_{x=256} = \frac{1}{4(256)^{\frac{3}{4}}} = \frac{1}{256}$

△y = dy = $(\frac{dy}{dx})_{x=256}$ dx

△y = $(\frac{1}{256})$ △x

△y = $(\frac{1}{256})$ (-1) = $\frac{-1}{256}$ = -0.003906

Hence, $(255)^{\frac{1}{4}}$ = y+△y = 0.2 + (-0.003906) = 3.9961

(七) 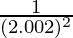$\frac{1}{(2.002)^2}$

解决方案：

Considering the function as

y = f(x) = $\frac{1}{x^2}$

Taking x = 2, and

x+△x = 2.002

△x = 2.002-2 = 0.002

$y = \frac{1}{x^2}$

$y_{(x=2)} = \frac{1}{2^2} = \frac{1}{4}$

$\frac{dy}{dx} = \frac{-2}{x^3}$

$(\frac{dy}{dx})_{x=2} = \frac{-2}{2^3} = \frac{-1}{4}$

△y = dy = $(\frac{dy}{dx})_{x=2}$ dx

△y = $(\frac{-1}{4})$ △x

△y = $(\frac{-1}{4})$ (0.002) = -0.0005

Hence, $\frac{1}{(2.002)^2}$ = y+△y = $\frac{1}{4}$ + (-0.005) = 0.2495

(viii) log _e 4.04，假设 log ₁₀ 4=0.6021 和 log ₁₀ e=0.4343

解决方案：

Considering the function as

y = f(x) = log_e x

Taking x = 4, and

x+△x = 4.04

△x = 4-4.04 = 0.04

y = log_e x 

$y_{(x=4)}$ = log_e 4 = $\frac{log_{10}4}{log_{10}e} = \frac{0.6021}{0.4343}$ = 1.386368

$\frac{dy}{dx} = \frac{1}{x}$

$(\frac{dy}{dx})_{x=4} = \frac{1}{4}$

△y = dy = $(\frac{dy}{dx})_{x=4}$ dx

△y = $(\frac{1}{4})$ △x

△y = $(\frac{1}{4})$ (0.04) = 0.01

Hence, log_e 4.04 = y+△y = 1.386368 + 0.01 = 1.396368

(ix) log _e 10.02，假设 log _e 10=2.3026

解决方案：

Considering the function as

y = f(x) = log_e x

Taking x = 10, and

x+△x = 10.02

△x = 10.02-10 = 0.02

y = log_e x

$y_{(x=10)}$ = log_e 10 = 2.3026

$\frac{dy}{dx} = \frac{1}{x}$

$(\frac{dy}{dx})_{x=10} = \frac{1}{10}$

△y = dy = $(\frac{dy}{dx})_{x=10}$ dx

△y = $(\frac{1}{10})$ △x

△y = $(\frac{1}{10})$ (0.02) = 0.002

Hence, log_e 10.02 = y+△y = 2.3026 + 0.002 = 2.3046

(x) log ₁₀ 10.1，假设 log ₁₀ e=0.4343

解决方案：

Considering the function as

y = f(x) = log₁₀ x

Taking x = 10, and

x+△x = 10.1

△x = 10.1-10 = 0.1

y = log₁₀ x = $\frac{log_ex}{log_e10}$

$y_{(x=10)}$ = log₁₀ 10 = 1

$\frac{dy}{dx} = \frac{1}{2.3025x}$

$(\frac{dy}{dx})_{x=10} = \frac{1}{23.025}$

△y = dy = $(\frac{dy}{dx})_{x=10}$ dx

△y = $(\frac{1}{23.025})$ △x

△y = $(\frac{1}{23.025})$ (0.1) = 0.004343

Hence, log_e 10.1 = y+△y = 1 + 0.004343 = 1.004343

(xi) cos 61°，假设 sin 60°=0.86603 和 1°=0.01745 弧度。

解决方案：

Considering the function as

y = f(x) = cos x

Taking x = 60°, and

x+△x = 61°

△x = 61°-60° = 1° = 0.01745 radian

y = cos x

$y_{(x=60 \degree)}$ = cos 60° = 0.5

$\frac{dy}{dx}$ = – sin x

$(\frac{dy}{dx})_{x=60\degree}$ = – sin 60° = -0.86603

△y = dy = $(\frac{dy}{dx})_{x=60\degree}$ dx

△y = (-0.86603) △x

△y = (-0.86603) (0.01745) = -0.01511

Hence, cos 61° = y+△y = 0.5 + (-0.01511) = 0.48489

(十二) $\frac{1}{\sqrt{25.1}}$

解决方案：

Considering the function as

y = f(x) = $\frac{1}{\sqrt{x}}$

Taking x = 25, and

x+△x = 25.1

△x = 25.1-25 = 0.1

$y = \frac{1}{\sqrt{x}}$

$y_{(x=25)} = \frac{1}{\sqrt{25}} = \frac{1}{5}$

$\frac{dy}{dx} = \frac{-1}{2(x)^{\frac{3}{2}}}$

$(\frac{dy}{dx})_{x=25} = \frac{-1}{2(25)^{\frac{3}{2}}} = \frac{-1}{250}$

△y = dy = $(\frac{dy}{dx})_{x=25}$ dx

△y = $(\frac{-1}{250})$ △x

△y = $(\frac{-1}{250})$ (0.1) = $\frac{-1}{2500}$ = -0.0004

Hence, $\frac{1}{\sqrt{25.1}}$ = y+△y = $\frac{1}{5}$ + (-0.0004) = 0.1996

(十三) 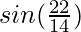$sin (\frac{22}{14})$

解决方案：

Considering the function as

y = f(x) = sin x

Taking x = 22/7, and

x+△x = 22/14

△x = 22/14-22/7 = -22/14

sin (-22/14) = -1

y = sin x

$y_{(x=22/7)}$ = sin (22/7) = 0

$\frac{dy}{dx}$ = cos x

$(\frac{dy}{dx})_{x=22/7}$ = cos (22/7)= -1

△y = dy = $(\frac{dy}{dx})_{x=22/7}$ dx

△y = (-1) △x

△y = (-1) (-1) = 1

Hence, sin(22/14) = 0+1 = 1