第 12 类 RD Sharma 解决方案 – 第 23 章向量代数 – 练习 23.4

问题1.若O为空间中的一点，ABC为三角形，D、E、F分别为三角形的边BC、CA、AB的中点，证明：

$\overrightarrow{OA}+\overrightarrow{OB}+\overrightarrow{OC}=\overrightarrow{OD}+\overrightarrow{OE}+\overrightarrow{OF}$

解决方案：

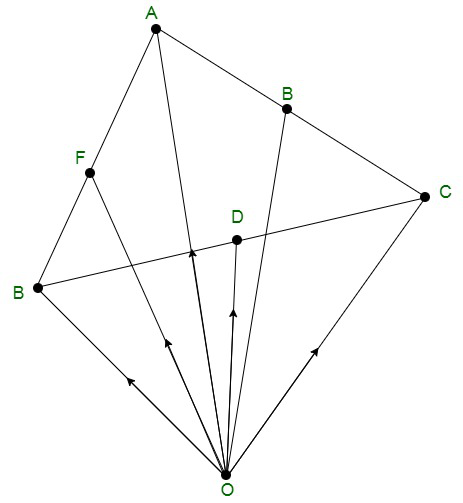
In the △ABC, D, E, F are the mid points of the sides of BC, CA and AB and O is any point in space.

Let us considered $\vec{a},\ \ \vec{b},\ \ \vec{c},\ \ \vec{d},\ \ \vec{e},\ \ \vec{f}$be the position vector of point A, B, C, D, E, F with respect to O.

Therefore, 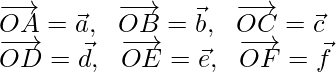$\overrightarrow{OA}=\vec{a},\ \ \overrightarrow{OB}=\vec{b},\ \ \overrightarrow{OC}=\vec{c}\\ \overrightarrow{OD}=\vec{d},\ \ \overrightarrow{OE}=\vec{e},\ \ \overrightarrow{OF}=\vec{f}$

So, according to the mid-point formula

 $\vec{d}=\frac{\vec{b}+\vec{c}}{2}\\ \vec{e}=\frac{\vec{a}+\vec{c}}{2}\\ \vec{f}=\frac{\vec{a}+\vec{b}}{2}$

$\overrightarrow{OD}+\overrightarrow{OE}+\overrightarrow{OF}=\vec{d}+\vec{e}+\vec{f}\\ =\frac{\vec{b}+\vec{c}}{2}+\frac{\vec{a}+\vec{c}}{2}+\frac{\vec{a}+\vec{b}}{2}\\ =\frac{\vec{b}+\vec{c}+\vec{a}+\vec{c}+\vec{a}+\vec{b}}{2}\\ =\frac{2(\vec{a}+\vec{b}+\vec{c})}{2}\\ =\vec{a}+\vec{b}+\vec{c}\\ =\overrightarrow{OA}+\overrightarrow{OB}+\overrightarrow{OC}$

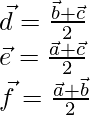
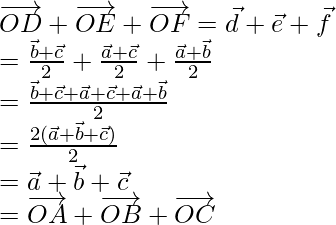
Hence, proved

$\overrightarrow{OD}+\overrightarrow{OE}+\overrightarrow{OF}=\overrightarrow{OA}+\overrightarrow{OB}+\overrightarrow{OC}$

问题 2. 证明由顶点指向的三角形的中线确定的三个向量之和为零。

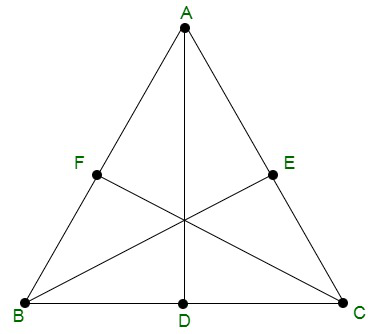
解决方案：

Let us considered ABC is triangle, so the position vector of A, B and C are $\vec{a},\ \ \vec{b}\ and\ \ \vec{c}$ 

Hence, AD, BE, CF are medium, so, D, E and F are mid points of line BC, AC, and AB.

Now, using mid point formula we get

Position vector of D = $\frac{\vec{b}+\vec{c}}{2}$                                          

Position vector of E = $\frac{\vec{c}+\vec{a}}{2}$                                             

Position vector of F = $\frac{\vec{a}+\vec{b}}{2}$   

Now, add all the three median                                          

$\overrightarrow{AD}+\overrightarrow{BE}+\overrightarrow{CF}$

$=\left(\frac{\vec{b}+\vec{c}}{2}-\vec{a}\right)+\left(\frac{\vec{c}+\vec{a}}{2}-\vec{b}\right)+\left(\frac{\vec{a}+\vec{b}}{2}-\vec{c}\right)\\ =\frac{\vec{b}+\vec{c}-2\vec{a}}{2}+=\frac{\vec{c}+\vec{a}-2\vec{b}}{2}+=\frac{\vec{a}+\vec{b}-2\vec{c}}{2}\\ =\frac{\vec{b}+\vec{c}-2\vec{a}+\vec{c}+\vec{a}-2\vec{b}+\vec{a}+\vec{b}-2\vec{c}}{2}\\ =\frac{2\vec{b}+2\vec{c}+2\vec{a}-2\vec{b}-2\vec{a}-2\vec{c}}{2}\\ =\frac{\vec{0}}{2}\\ =\vec{0}\\ \therefore\overrightarrow{AD}+\overrightarrow{BE}+\overrightarrow{CF}=\vec{0}$

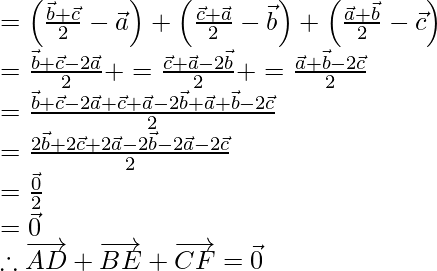
Hence, proved that the sum of the three vectors determined by the medians 

of a triangle directed from the vertices is zero.

问题 3. ABCD 是一个平行四边形，P 是它的对角线的交点。如果 O 是参考原点，证明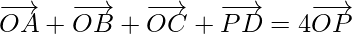$\overrightarrow{OA}+\overrightarrow{OB}+\overrightarrow{OC}+\overrightarrow{PD}=4\overrightarrow{OP}$

解决方案：

Given that ABCD is a parallelogram, P is the point of intersection of diagonals and O be the point of reference.

So, by using triangle law in △AOP, we get

$\overrightarrow{OP}+\overrightarrow{PA}=\overrightarrow{OA}$        -(1)

By using triangle law in △OBP, we get

$\overrightarrow{OP}+\overrightarrow{PB}=\overrightarrow{OB}$       -(2)

By using triangle law in △OPC, we get

$\overrightarrow{OP}+\overrightarrow{PC}=\overrightarrow{OC}$       -(3)

By using triangle law in △OPD, we get

$\overrightarrow{OP}+\overrightarrow{PD}=\overrightarrow{OD}$       -(4)

Now, on adding equation (1), (2), (3) and (4), we get

$\overrightarrow{OP}+\overrightarrow{PA}+\overrightarrow{OP}+\overrightarrow{PB}+\overrightarrow{OP}+\overrightarrow{PC}+\overrightarrow{OP}+\overrightarrow{PD}=\overrightarrow{OA}+\overrightarrow{OB}+\overrightarrow{OC}+\overrightarrow{OD}\\ 4\overrightarrow{OP}+\overrightarrow{PA}+\overrightarrow{PB}+\overrightarrow{PC}+\overrightarrow{PD}=\overrightarrow{OA}+\overrightarrow{OB}+\overrightarrow{OC}+\overrightarrow{OD}\\ 4\overrightarrow{OP}+\overrightarrow{PA}+\overrightarrow{PB}-\overrightarrow{PA}-\overrightarrow{PB}=\overrightarrow{OA}+\overrightarrow{OB}+\overrightarrow{OC}+\overrightarrow{OD}\\ Since, \overrightarrow{PC}=-\overrightarrow{PA}\ and\ \overrightarrow{PD}=-\overrightarrow{PB}\ as\ P\ is\ mid\ point\ of\ AC,\ BD\\ 4\overrightarrow{OP}=\overrightarrow{OA}+\overrightarrow{OB}+\overrightarrow{OC}+\overrightarrow{OD}$

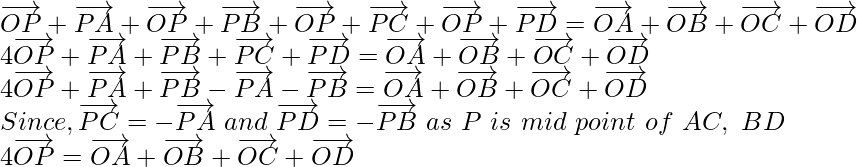
问题 4. 证明连接四边形对边中点的线段相互平分。

解决方案：

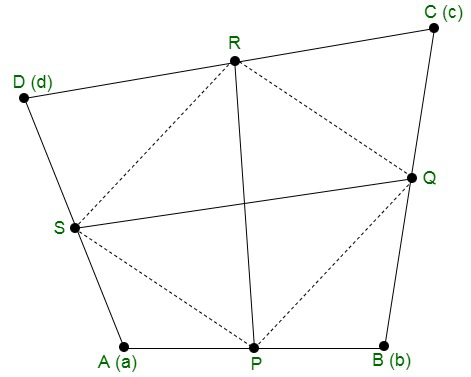
Let us considered ABCD be a quadrilateral and P, Q, R, S be the mid points of sides AB, BC, CD and DA.

So, the position vector of A, B, C and D be $\vec{a},\ \vec{b},\ \vec{c}\ and\ \vec{d}.$

Using the mid point formula 

Position vector of P = $\frac{\vec{a}+\vec{b}}{2}$

Position vector of Q = $\frac{\vec{b}+\vec{c}}{2}$

Position vector of R = $\frac{\vec{c}+\vec{d}}{2}$

Position vector of S = $\frac{\vec{d}+\vec{a}}{2}$

Position vector of $\overrightarrow{PQ}$ = Position vector of Q – Position vector of P

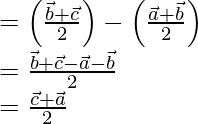$=\left(\frac{\vec{b}+\vec{c}}{2}\right)-\left(\frac{\vec{a}+\vec{b}}{2}\right)\\ =\frac{\vec{b}+\vec{c}-\vec{a}-\vec{b}}{2}\\ =\frac{\vec{c}+\vec{a}}{2}$        -(1)

Position vector of $\overrightarrow{SR}$ = Position vector of R – Position vector of S

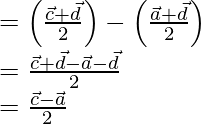$=\left(\frac{\vec{c}+\vec{d}}{2}\right)-\left(\frac{\vec{a}+\vec{d}}{2}\right)\\ =\frac{\vec{c}+\vec{d}-\vec{a}-\vec{d}}{2}\\ =\frac{\vec{c}-\vec{a}}{2}$      -(2)

From eq(1) and (2),

$\overrightarrow{PQ}=\overrightarrow{SR}$

So, PQRS is a parallelogram and PR bisects QS             -(as diagonals of parallelogram)

Hence, proved that the line segments joining the mid-points of 

opposite sides of a quadrilateral bisect each other. 

问题 5. ABCD 是平面上的四个点，Q 是 AB 和 CD 中点连线的交点；公元前和公元。显示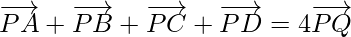$\overrightarrow{PA}+\overrightarrow{PB}+\overrightarrow{PC}+\overrightarrow{PD}=4\overrightarrow{PQ}$其中 P 是任意点。

解决方案：

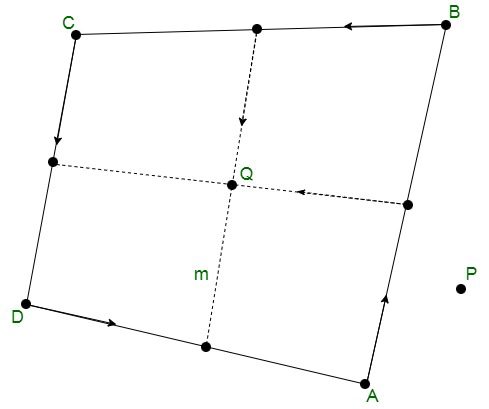
Let us considered the position vector of the points A, B, C and D are$\vec{a},\ \ \vec{b},\ \ \vec{c},\ \ \vec{d}$

Using the mid-point formula, we get

Position vector of AB = $\frac{\vec{a}+\vec{b}}{2}$

Position vector of BC = $\frac{\vec{b}+\vec{c}}{2}$

Position vector of CD = $\frac{\vec{c}+\vec{d}}{2}$

Position vector of DA = $\frac{\vec{a}+\vec{d}}{2}$

It is given that Q is the mid point of the line joining the mid points of AB and CD, so

$Q=\frac{\frac{\vec{a}+\vec{b}}{2}+\frac{\vec{c}+\vec{d}}{2}}{2}\\ =\frac{\vec{a}+\vec{b}+\vec{c}+\vec{d}}{4}$

Now, let us assume $\vec{p}$ be the position vector of P.

So,

$\overrightarrow{PA}+\overrightarrow{PB}+\overrightarrow{PC}+\overrightarrow{PD}\\ =\vec{a}-\vec{p}+\vec{b}-\vec{p}+\vec{c}-\vec{p}+\vec{d}-\vec{p}\\ =(\vec{a}+\vec{b}+\vec{c}+\vec{d})-4\vec{p}\\ =4\left(\frac{\vec{a}+\vec{b}+\vec{c}+\vec{d} }{4}-p\right)\\ =4\overrightarrow{PQ}$

Hence proved

问题 6. 用向量法证明三角形内角的内平分线是同时的。

解决方案：

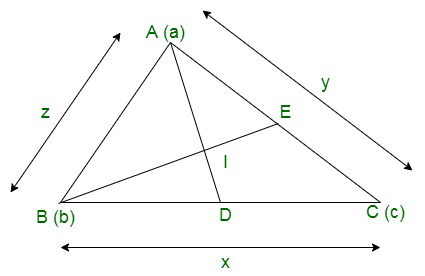
In triangle ABC, let us assume that the position vectors of the vertices of the triangle are $A(\vec{a}), B(\vec{b})\ and\ C(\vec{c}).$

Length of the sides:

BC = x

AC = y

AB = z

In triangle ABC, the internal bisector divides the opposite side in the ratio of the sides containing the angles.

Since AD is the internal bisector of the ∠ABC, so

BD/DC = AB/AC = z/y          -(1)

Therefore, position vector of D = $\frac{z\vec{c}+y\vec{b}}{y+z}$ 

Let the internal bisector intersect at point I.

ID/AI = BD/AB         -(2)

BD/DC = z/y

Therefore,

CD/BD = z/y

(CD + BD)/BD = (y + z)/z

BC/BD = (y + z)/z

BD = ln/y + z         -(3)

So, from eq(2) and (3), we get

ID/AI = ln/(y +z)

Therefore,

Position vector of I = $\frac{\left(\frac{zc+yb}{y+z}\right)(y+z)+la}{l+y+z}=\frac{la+yb+zc}{l+y+z}$

Similarly, we can also prove that I lie on the internal bisectors of ∠B and ∠C. 

Hence, the bisectors are concurrent.