第 10 类 NCERT 解决方案 - 第 13 章表面积和体积 - 练习 13.3
问题 1. 将一个半径为 4.2 厘米的金属球熔化并重铸成半径为 6 厘米的圆柱体。求圆柱体的高度。
解决方案:

Radius of sphere (R)= 4.2 cm
Radius of cylinder (r)= 6 cm
In recasting process the volume will be same, so
Volume of cylinder = Volume of sphere
πr2h = ![]() πR3
πR3
π(6)2h = ![]() π(4.2)3 (cancel π from both side)
π(4.2)3 (cancel π from both side)
36h = ![]() (4.2 × 4.2 × 4.2)
(4.2 × 4.2 × 4.2)
h = ![]() (cm)
(cm)
h = 1.4 × 1.4 × 1.4 (cm)
h = 2.74 cm
问题 2. 半径分别为 6 cm、8 cm 和 10 cm 的金属球被熔化形成一个单一的固体球。找到生成的球体的半径。
解决方案:

Radius of sphere 1 (r1)= 6 cm
Radius of sphere 2 (r2)= 8 cm
Radius of sphere 3 (r3)= 10 cm
Let Radius of resulting sphere = R
In recasting process the volume will be same, so
Volume of Resulting sphere = Volume of sphere 1 + Volume of sphere 2 + Volume of sphere 3
![]() π(R)3 =
π(R)3 = ![]() π(r1)3 +
π(r1)3 + ![]() π(r2)3 +
π(r2)3 +![]() π(r3)3 (cancel
π(r3)3 (cancel ![]() π from both side)
π from both side)
R3 = (r1)3 + (r2)3 + (r3)3
R3 = (6)3 + (8)3 + (10)3
R3 = 216 + 512 + 1000
R3 = 1728
R = (1728) 1/3
R = 12 cm
问题 3. 挖一口 20m 深、直径 7m 的井,将挖出的土均匀铺开,形成一个 22m×14m 的平台。求平台的高度。
解决方案:

So basically here, the digging of cylindrical shape is changed to cuboidal shape
Given values,
Diameter of cylinder = 7 m
Radius of cylinder (r)= ![]() m
m
Height of cylinder (H)= 20 m
Length of Cuboid (l) = 22 m
Breadth of Cuboid (b) =14 m
Let Height of Cuboid = h
In this process the volume will be same, so
Volume of Cuboid = Volume of Cylinder
l × b × h = πr2H
22 × 14 × h = π × ![]() × 20
× 20
h = ![]() m (taking π =
m (taking π =![]() )
)
h = ![]() m
m
h = 2.5 m
问题 4. 直径 3 m 的井被挖 14 m 深。从中取出的泥土均匀地散布在它周围,形成一个宽4m的圆环,形成一个堤坝。求路堤的高度。
解决方案:

So basically here, the digging of cylindrical shape is changed to another Hollow cylindrical shape
Given values,
Diameter of cylinder = 3 m
Radius of cylinder (r) = ![]() m
m
Height of cylinder (h) = 14 m
Width of embankment = 4 m
Outer Radius of embankment R1= radius of cylinder + width = 3/2 + 4 = m
Inner Radius of embankment R2= radius of cylinder = ![]() m
m
Height of embankment = H
In this process the volume will be same, so
Volume of Embankment = Volume of Cylinder
(πR12H) – (πR22H) = πr2h
(R12 – R22)H = (![]() )2× 14 (cancel π from both side)
)2× 14 (cancel π from both side)
H = 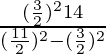
H =
H = ![]()
H =
H = ![]()
H = ![]() m
m
H = 1.125 m
问题 5. 一个直径 12 厘米,高 15 厘米的直圆柱容器装满冰淇淋。冰淇淋将被装入高 12 厘米、直径 6 厘米的圆锥体中,顶部呈半球形。找出可以装满冰淇淋的这种锥体的数量。
解决方案:

Given values,
Radius of cylinder (r) = 6 cm
Height of cylinder (h) = 15 cm
Radius of each cone (R) = 3 cm
Height of each cone (H) = 12 cm
Let n be the total number of ice creams
In this process the volume will be same, so
n × (Volume of each Cone + Volume of each hemisphere) = Volume of Cylinder
n × (![]() πR2H +
πR2H + ![]() πR3) = πr2h
πR3) = πr2h
n = (cancel π from both side)
n =
n =
n =
n = 10
Hence, 10 numbers of cones filled with ice-cream.
问题 6. 有多少银币,直径 1.75 厘米,厚 2 毫米,必须熔化成一个 5.5 厘米 × 10 厘米 × 3.5 厘米的长方体?
解决方案:

Given values,
Radius of cylindrical coin (r) = ![]()
Height of cylindrical coin (H) = 2 mm = ![]() cm
cm
Length of Cuboid (l) = 5.5 cm
Breadth of Cuboid (b) =10 cm
Height of Cuboid (h)= 3.5 cm
Let n be the total number of coins
In this process the volume will be same, so
n × (Volume of each Coin) = Volume of Cylinder
n × (πR2H) = l × b × h
n × π × (![]() )2×
)2× ![]() = 5.5 × 10 × 3.5
= 5.5 × 10 × 3.5
n = 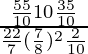 (taking π =
(taking π = ![]() )
)
n =
n = 400
Hence, 400 silver coins must be melted to form this cuboid.
问题 7. 一个圆柱形桶,高 32 厘米,底部半径 18 厘米,装满沙子。这个桶倒在地上,就形成了一个圆锥形的沙堆。如果圆锥堆的高度是 24 厘米,求堆的半径和斜高。
解决方案:
So basically here, the cylindrical shape is changed to conical shape

Given values,
Radius of cylinder (r) = 18 cm
Height of cylinder (h) = 32 cm
Height of cone (H) = 24 cm
Let Radius of cone = R
In this process the volume will be same, so
Volume of Cone = Volume of Cylinder
![]() πR2H = πr2h
πR2H = πr2h
![]() R2 × (24) = 182 × 32 (cancel π from both side)
R2 × (24) = 182 × 32 (cancel π from both side)
R2 =
R = √(18 × 18 × 4)
R = 36 cm
Slant height (l) = √H2 + R2
l = √(242 + 362)
l = √(12×2)2 + (12×3)2
l =12 √(4+9)
l = 12 √13 cm
问题 8. 一条宽 6 m、深 1.5 m 的运河中的水以 10 km/h 的速度流动。如果需要 8 厘米的积水,它会在 30 分钟内灌溉多少面积?
解决方案:
Given values,
Width of canal (w) = 6 m
Depth of canal (h) = 1.5 m
Speed of canal = 10 km/hr = (10,000 m/hr)
For 1hr (60 mins), we can take length (l) as = 10,000 m and
Volume in 1hr = (l × w × h)
= (10,000 × 6 × 1.5) m3
= 90,000 m3
So for 30 mins volume will be = ![]() m3
m3
= 45,000 m3
Area irrigated in 30 minutes will be as:
Area × length of standing = Volume of canal in 30mins
Area = ![]()
Area = 562500 m2
问题 9. 一位农民将一根内径为 20 cm 的管道从一条运河连接到她的田地中的一个圆柱形水箱中,该水箱直径为 10 m,深 2 m。如果水以 3 公里/小时的速度流过管道,水箱需要多长时间才能充满?
解决方案:
Given values,

Radius of Pipe (R) = 10 cm = m
Radius of Cylindrical tank (r) = 5 m
Depth of Cylindrical tank (h) = 2 m
Speed of water flows in pipe = 3 km/hr = (3,000 m/hr)
Let Time to fill cylindrical tank = t
For 1hr (60 mins), we can take height of Pipe (H) as = 3,000 m and
Volume in 1hr = (πR2H)
= (π × (![]() )2 × 3,000) m3
)2 × 3,000) m3
= 30π m3
In this process the volume will be same, so
t (in hr) × (Volume of Pipe in 1hr) = Volume of Cylindrical Tank
t × (30π) = πr2h
t × (30π) = π × 52 × 2
t = ![]()
t = ![]() hr
hr
t = ![]() × 60 mins
× 60 mins
t = 100 minutes
Hence, 100 minutes will be taken to fill the tank.