10类NCERT解决方案-第13章表面积和体积–练习13.2

问题1.固体呈半球状的圆锥体，其半径均等于1 cm，并且圆锥体的高度等于其半径。用π求出固体的体积。

解决方案：

Given:

Height of cone (h)= 1 cm

Radius of hemisphere (r) = 1 cm

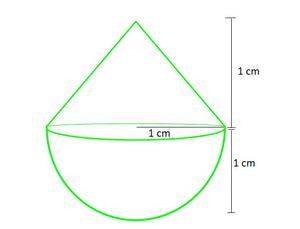
Total Volume = Volume of cone + Volume of Hemisphere

= $\frac{1}{3}$πr²h + $\frac{2}{3}$πr³

= $\frac{1}{3}$πr²(h+2r)

= $\frac{1}{3}$ × π × 1 × 1 × (1+2)

= π cm³

问题2.要求工程专业的学生瑞秋(Rachel)使用薄铝板制作一个形状像圆柱体的模型，在圆柱体的两端连接两个圆锥体。模型的直径为3厘米，长度为12厘米。如果每个锥体的高度为2厘米，请查找Rachel制作的模型中包含的空气量。 (假设模型的外部和内部尺寸几乎相同。)

解决方案：

Given:

Radius of cone and cylinder (r) = $\frac{3}{2}$ cm

Height of cone (h) = 2 cm

Height of cylinder (H) = 12- (2+2) = 8 cm

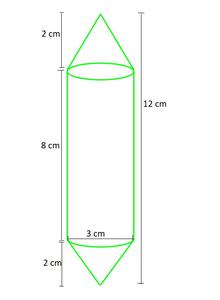
Total Volume = Volume of two cones + Volume of cylinder

 = $\frac{1}{3}$πr²h + $\frac{1}{3}$πr²h + πr²H

= $\frac{2}{3}$πr²h + πr²H

= πr²(($\frac{2}{3}$)h+H)

= $\frac{22}{7} × \frac{3}{2} × \frac{3}{2} (\frac{(2 × 2)}{3} + 8)$                      (taking π=$\frac{22}{7}$)

= $\frac{22}{7} × \frac{3}{2} × \frac{3}{2} × \frac{28}{3}$

= $\frac{21 × 22}{ 7}$

= 66 cm³

问题3. gulab jamun包含的糖浆最多占糖浆体积的30％。找出大约45个瓜拉果酱中的糖浆含量，每个瓜拉果酱的形状都像一个圆柱体，两个半球形末端的长度为5厘米，直径为2.8厘米(见图)。

解决方案：

Given, 

For 1 gulab jamun,

Height of cylindrical part (H)= 5-(2.8) = 2.2 cm

Radius of cylindrical and hemispherical part (r)= $\frac{2.8}{2}$ = 1.4 cm

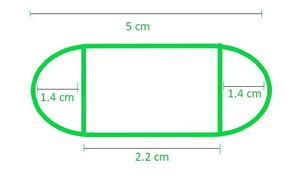
Total Volume of one gulab jamun = Volume of two Hemisphere + Volume of cylinder

= $\frac{2}{3}$πr³ + $\frac{2}{3}$πr³ + πr²H

= πr² ($\frac{4}{3}$r + H)

= $\frac{22}{7} × 1.4 × 1.4 × ((\frac{4}{3} × 1.4) + 2.2)$                       (taking π=$\frac{22}{7}$)

= 25.05 cm³

Hence, volume of 45 gulab jamun = 45 × Volume of 1 gulab jamun

= 45 × 25.05

= 1127.28 cm³

As, gulab jamun contains sugar syrup up to about 30% of its volume

Sugar syrup in 45 gulab jamun = 30% of its total volume

= $\frac{30}{100}$× 1127.28

= $\frac{33818.4}{100}$

= 338.184 cm³

问题4.木制笔架为长方体形状，带有四个圆锥形凹陷，可容纳笔。长方体的尺寸为15厘米x 10厘米x 3.5厘米。每个凹陷的半径为0.5厘米，深度为1.4厘米。找到整个支架中的木材量(参见图)。

解决方案：

Given:

Length of cuboid (l) = 15 cm

Breadth of cuboid (b) = 10 cm

Height of cuboid (h) = 3.5 cm

Radius of conical part (r) = 0.5 cm

Height of conical part (H) = 1.4 cm

Total Volume wood in the entire stand = Volume of Cuboid – Volume of four conical part

= (l × b × h) – 4 × ($\frac{1}{3}$πr²H)

= (15 × 10 × 3.5) – (4 × $\frac{1}{3} × \frac{22}{7}$ × 0.5 × 0.5 × 1.4)                           (taking π=$\frac{22}{7}$)

= 525 – (1.466)

= 523.5333 cm³

问题5.容器为倒圆锥形。它的高度是8厘米，顶部的半径是5厘米。它充满了水直到边缘。当每个直径为0.5厘米的球形铅球掉入容器中时，四分之一的水会流出。查找掉落到容器中的铅弹数量。

解决方案：

Given:

Height of cone (h) = 8 cm

Radius of cone (r) = 5 cm

Radius of sphere (R)= 0.5 cm

Let, No. of sphere in cone = n

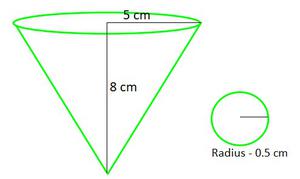
Volume of water = Volume of cone 

= $\frac{1}{3}$πr²h

= $\frac{1}{3}$ × π × 5 × 5 × 8

= $\frac{200 π}{ 3}$ cm³

Volume of water flows out = $\frac{1}{4}$ (total volume of water)

= $\frac{1}{4} × \frac{200 π}{ 3}$

= $\frac{50 π}{ 3}$ cm³

Hence, the volume of n spheres = $\frac{50 π}{ 3}$cm³

Volume of each sphere = $\frac{4}{3}$πr³

= $\frac{4}{3}$× π × 0.5 × 0.5 ×0.5

= $\frac{π}{6}$ cm³

Hence, n = $\frac{(Volume\hspace{0.1cm} of\hspace{0.1cm} n\hspace{0.1cm} sphere)}{ (volume \hspace{0.1cm}of \hspace{0.1cm}each \hspace{0.1cm}sphere)}$

n = $\frac{\frac{50 π}{3}} {\frac{π}{6}}$

n = 100 spheres

问题6.实心铁杆由一个高度为220厘米，底径为24厘米的圆柱体组成，并由另一个高度为60厘米，半径为8厘米的圆柱体覆盖。给定1 cm ³的铁大约有8g的质量，求出极的质量。 (使用π= 3.14)

解决方案：

Given:

Height of large cylinder (H)= 220 cm

Radius of large cylinder (R)= $\frac{24}{2}$ = 12 cm

Height of small cylinder (h)= 60 cm

Radius of small cylinder (r)= 8 cm

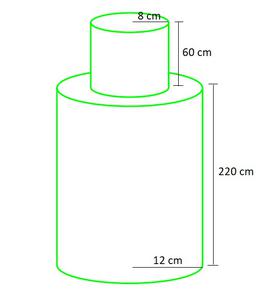
Total Volume = Volume of large cylinder + Volume of small cylinder 

= πR²H + πr²h

= π (R²H + r²h)

= 3.14 × ((12 × 12 × 220) + (8 × 8 × 60))                             (taking π=3.14)

= 3.14 (31680 + 3840)

= 111532.8 cm³

As given, Mass of 1cm³ = 8 g

Mass for 111532.8 cm³ = 8 × 111532.8 g

= 892,262.4 grams

= 892.2624 kg

问题7.将一个高度为120厘米，半径为60厘米的右圆锥体竖立在半径为60厘米的半球上的固体放置在充满水的右圆柱体中，使其与底部接触。如果圆柱体的半径为60厘米，高度为180厘米，则求出圆柱体内剩余的水量。

解决方案：

Given:

Height of cylinder (H)= 180 cm

Height of cone (h)= 180 – 60 = 120 cm

Radius of cone, cylinder and hemisphere (r) = 60 cm

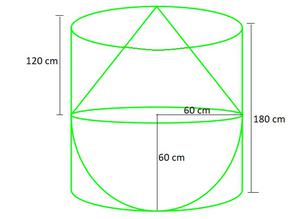
Volume of water left in the cylinder = Volume of cylinder – (Volume of cone + Volume of hemisphere)

= πr²H – ($\frac{1}{3}$πr²h + $\frac{2}{3}$πr³)

= πr² (H – $\frac{1}{3}$h + $\frac{2}{3}$r)

= $\frac{22}{7} × 60 × 60 × (180 - (\frac{1}{3} ×120 + \frac{2}{3}×60))$                         (taking π=$\frac{22}{7}$)

= $\frac{22}{7}$ × 60 × 60 × (100)

= 1131428.571 cm³

= 1.131 m³

Hence, the volume of water left in the cylinder = 1.131 m³

问题8.球形玻璃容器的圆柱颈长8厘米，直径2厘米；球形部分的直径为8.5厘米。通过测量水中的水量，儿童发现其水量为345 cm ³ 。将上面的内容作为内部测量结果，检查她是否正确，并且π= 3.14。

解决方案：

Given:

Height of cylinder (h)= 8 cm

Radius of cylinder (r) = 2/2 = 1 cm

Radius of sphere (R) = $\frac{8.5}{2}$cm

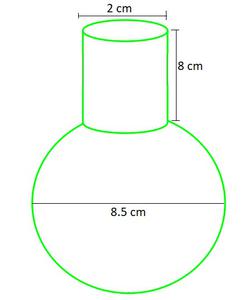
Amount of water it can hold = Total Volume of this vessel

= Volume of cylinder + Volume of sphere

= πr²h + $\frac{4}{3}$πR³

= $(π × 1 × 1 × 8) + (\frac{4}{3} × π × \frac{8.5}{2} × \frac{8.5}{2} × \frac{8.5}{2})$

= 8π + 102.35π

= 110.35π                       (taking π=3.14)

= 346.499 cm³

Volume measured by child = 345 cm³, which is INCORRECT

Correct volume = 346.5 cm³