第 12 类 RD Sharma 解决方案 - 第 31 章概率 - 练习 31.7 |设置 1

问题一、骨灰盒I、II、III的内容如下：

瓮 I：1 个白球、2 个黑球和 3 个红球

瓮 II：2 个白球、1 个黑球和 1 个红球

瓮 III：4 个白球、5 个黑球和 3 个红球

随机选择一个瓮，抽取两个球。它们恰好是白色和红色的。它们来自瓮 I、II、III 的概率是多少？

解决方案：

Let us assume that E₁, E₂ and E₃ be the events of selecting Urn I, Urn II and Urn III. And also A be the event that the two balls drawn are white and red.

So, P(E₁) = 1/3

P(E₂₎ = 1/3

P(E₃) = 1/3

Now,

P(A/E₁) = $\frac{{}^1 C_1 \times^3 C_1}{{}^6 C_2}$= 3/15 = 1/5

P(A/E₂) = $\frac{{}^2 C_1 \times^1 C_1}{{}^4 C_2}$= 2/6 = 1/3

P(A/E₃) = $\frac{{}^4 C_1 \times^3 C_1}{{}^{12} C_2}$= 12/66 = 2/11

By using Bayes’ theorem, the required probability is,

P(E₁/A) = $\frac{P\left( E_1 \right)P\left( A/ E_1 \right)}{P\left( E_1 \right)P\left( A/ E_1 \right) + P\left( E_2 \right)P\left( A/ E_2 \right) + P\left( E_3 \right)P\left( A/ E_3 \right)}$

= $\frac{\frac{1}{3} \times \frac{1}{5}}{\frac{1}{3} \times \frac{1}{5} + \frac{1}{3} \times \frac{1}{3} + \frac{1}{3} \times \frac{2}{11}}$

= $\frac{\frac{1}{5}}{\frac{1}{5} + \frac{1}{3} + \frac{2}{11}}$

= $\frac{\frac{1}{5}}{\frac{33 + 55 + 30}{165}}$

= 33/118

P(E₂/A) = $\frac{P\left( E_2 \right)P\left( A/ E_2 \right)}{P\left( E_1 \right)P\left( A/ E_1 \right) + P\left( E_2 \right)P\left( A/ E_2 \right) + P\left( E_3 \right)P\left( A/ E_3 \right)}$

= $\frac{\frac{1}{3} \times \frac{1}{3}}{\frac{1}{3} \times \frac{1}{5} + \frac{1}{3} \times \frac{1}{3} + \frac{1}{3} \times \frac{2}{11}}$

= $\frac{\frac{1}{3}}{\frac{1}{5} + \frac{1}{3} + \frac{2}{11}}$

= $\frac{\frac{1}{3}}{\frac{33 + 55 + 30}{165}}$

= 55/118

P(E₃/A) = $\frac{P\left( E_3 \right)P\left( A/ E_3 \right)}{P\left( E_1 \right)P\left( A/ E_1 \right) + P\left( E_2 \right)P\left( A/ E_2 \right) + P\left( E_3 \right)P\left( A/ E_3 \right)}$

= $\frac{\frac{1}{3} \times \frac{2}{11}}{\frac{1}{3} \times \frac{1}{5} + \frac{1}{3} \times \frac{1}{3} + \frac{1}{3} \times \frac{2}{11}}$

= $\frac{\frac{2}{11}}{\frac{1}{5} + \frac{1}{3} + \frac{2}{11}}$

= $\frac{\frac{2}{11}}{\frac{33 + 55 + 30}{165}}$

= 30/118

问题 2. 袋子 A 包含 2 个白色和 3 个红色球，袋子 B 包含 4 个白色和 5 个红色球。从其中一个袋子中随机抽出一个球，发现是红色的。求它是从袋子 B 中取出的概率。

解决方案：

Let us assume that the A, E₁ and E₂ be the events that the ball is red, bag A is chosen and bag B is chosen.

So, P(E₁) = 1/2

P(E₂) = 1/2

Now,

P(A/E₁) = 3/5

P(A/E₂) = 5/9

By using Bayes’ theorem, the required probability is,

P(E₂/A) = $\frac{P\left( E_2 \right)P\left( A/ E_2 \right)}{P\left( E_1 \right)P\left( A/ E_1 \right) + P\left( E_2 \right)P\left( A/ E_2 \right)}$

= $\frac{\frac{1}{2} \times \frac{5}{9}}{\frac{1}{2} \times \frac{3}{5} + \frac{1}{2} \times \frac{5}{9}}$

= 25/52

问题3.三个瓮里有2个白球和3个黑球；分别有 3 个白球和 2 个黑球和 4 个白球和 1 个黑球。一个球是从一个随机选择的瓮中抽出来的，结果发现它是白色的。找出它是从第一个瓮中取出的概率。

解决方案：

Let us consider E₁, E₂ and E₃ be the events of selecting Urn I, Urn II and Urn III.

Also, A be the event that the ball drawn is white.

So, P(E₁) = 1/3

P(E₂) = 1/3

P(E₃) = 1/3

Now,

P(A/E₁) = 2/5

P(A/E₂) = 3/5

P(A/E₃) = 4/5

By using Bayes’ theorem, the required probability is,

P(E₁/A) = $\frac{P\left( E_1 \right)P\left( A/ E_1 \right)}{P\left( E_1 \right)P\left( A/ E_1 \right) + P\left( E_2 \right)P\left( A/ E_2 \right) +P\left( E_3 \right)P\left( A/ E_3 \right)}$

= $\frac{\frac{1}{3} \times \frac{2}{5}}{\frac{1}{3} \times \frac{2}{5} + \frac{1}{3} \times \frac{3}{5} + \frac{1}{3} \times \frac{4}{5}}$

= $\frac{2}{2 + 3 + 4}$

= 2/9

问题四、三个骨灰盒的内容如下：

1号缸：7个白球，3个黑球，2号缸：4个白球，6个黑球，3号缸：2个白球，8个黑球。这些骨灰盒之一是随机选择的，概率分别为 0.20、0.60 和 0.20。从选定的瓮中随机抽取两个球，无需更换。如果这两个球都是白色的，它们来自 3 号罐的概率是多少？

解决方案：

Let us assume that E₁, E₂ and E₃ be the events of selecting Urn I, Urn II and Urn III. Also, A be the event that the two balls drawn are white.

So, P(E₁) = 20/100

P(E₂) = 60/100

P(E₃) = 20/100

Now,

P(A/E₁) = $\frac{{}^7 C_2}{{}^{10} C_2}$ = 21/45

P(A/E₂) = $\frac{{}^4 C_2}{{}^{10} C_2}$ = 6/45

P(A/E₃) = $\frac{{}^2 C_2}{{}^{10} C_2}$ = 1/45

By using Bayes’ theorem, the required probability is,

P(E₃/A) = $\frac{P\left( E_3 \right)P\left( A/ E_3 \right)}{P\left( E_1 \right)P\left( A/ E_1 \right) + P\left( E_2 \right)P\left( A/ E_2 \right) + P\left( E_3 \right)P\left( A/ E_3 \right)}$

= $\frac{\frac{20}{100} \times \frac{1}{45}}{\frac{20}{100} \times \frac{21}{45} + \frac{60}{100} \times \frac{6}{45} + \frac{20}{100} \times \frac{1}{45}}$

= $\frac{1}{21 + 18 + 1}$

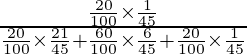
= 1/40

问题 5. 假设一个女孩掷骰子。如果她得到 1 或 2，她掷硬币 3 次并记下反面的数量。如果她得到 3、4、5 或 6，她会掷一次硬币，并记下得到的是“正面”还是“反面”。如果她正好得到一个“尾巴”，那么她用骰子掷出 3、4、5 或 6 的概率是多少？

解决方案：

Let us consider E₁ be the event that the outcome on the die is 1 or 2 and E₂ be the event that outcome on the die is 3, 4, 5 or 6. 

Now, P(E₁) = 2/6 = 1/3 and P(E₂) = 4/6 = 2/3

Also, let us assume A be the event of getting exactly one ‘tail’.

So, P(A/E₁) = 3/8

P(A/E₂) = 1/2

Now, P(E₂/A) is the probability that the girl threw 3, 4, 5 or 6 with the die, if she obtained exactly one tail

So, by using Bayes’ theorem, the required probability is,

P(E₂/A) = $\frac{P\left( E_2 \right) \times P\left( A/E_2 \right)}{P\left( E_1 \right) \times P\left( A/ E_1 \right) + P\left( E_2 \right) \times P\left( A/ E_2 \right)}$

= $\frac{\left( \frac{2}{3} \times \frac{1}{2} \right)}{\left( \frac{1}{3} \times \frac{3}{8} + \frac{2}{3} \times \frac{1}{2} \right)}$

= $\frac{ \frac{2}{6}}{ \frac{1}{8} + \frac{1}{3}}$

= $\frac{24 \times 2}{11 \times 6}$

= 8/11

问题 6. 两组竞争公司董事会的职位。第一组和第二组获胜的概率分别为 0.6 和 0.4。此外，如果第一组获胜，则推出新产品的概率为 0.7，如果第二组获胜，则相应的概率为 0.3。找出第二组推出的新产品的概率。

解决方案：

Let us consider E₁ and E₂ be the events that the first group and the second group win the competition. Also, A be the event of introducing a new product.

So, P(E₁) = 0.6

P(E₂) = 0.4

P(A/E₁) = 0.7

P(A/E₂) = 0.3

Now, P(E₂/A) is the probability that the new product is introduced by the second group

So, by using Bayes’ theorem, the required probability is,

P(E₂/A) = $\frac{P\left( E_2 \right)P\left( A/ E_2 \right)}{P\left( E_1 \right)P\left( A/ E_1 \right) + P\left( E_2 \right)P\left( A/ E_2 \right)}$

= $\frac{0 . 4 \times 0 . 3}{0 . 6 \times 0 . 7 + 0 . 4 \times 0 . 3}$

= 0.12/0.54

= 2/9

问题 7。假设 100 名男性中有 5 名男性和 1000 名女性中有 25 名是优秀的演说家。演讲者是随机选择的。求一个男性被选中的概率。假设男女人数相等。

解决方案：

Let us assume that A, E₁ and E₂ be the events that the person is a good orator, is a man and is a woman.

So, P(E₁) = 1/2

P(E₂) = 1/2

Now,

P(A/E₁) = 5/100

P(A/E₂) = 25/1000

So, using Bayes’ theorem, the required probability is,

P(E₁/A) = $\frac{P\left( E_1 \right)P\left( A/ E_1 \right)}{P\left( E_1 \right)P\left( A/ E_1 \right) + P\left( E_2 \right)P\left( A/ E_2 \right)}$

= $\frac{\frac{1}{2} \times \frac{5}{100}}{\frac{1}{2} \times \frac{5}{100} + \frac{1}{2} \times \frac{25}{1000}}$

= $\frac{1}{1 + \frac{1}{2}}$

= 2/3

问题 8。已知一封信来自伦敦或克利夫顿。在信封上只有两个连续的字母 ON 是可见的。这封信来自的概率是多少

(i) 伦敦 (ii) 克利夫顿？

解决方案：

Let us consider A, E₁ and E₂ be the events that the two consecutive letters are visible and the letter has come from LONDON and CLIFTON.

So, P(E₁) = 1/2

P(E₂) = 1/2

Now,

P(A/E₁) = 2/5

P(A/E₂) = 1/6

So, using Bayes’ theorem, the required probability is,

(i) P(E₁/A) = $\frac{P\left( E_1 \right)P\left( A/ E_1 \right)}{P\left( E_1 \right)P\left( A/ E_1 \right) + P\left( E_2 \right)P\left( A/ E_2 \right)}$

= $\frac{\frac{1}{2} \times \frac{2}{5}}{\frac{1}{2} \times \frac{2}{5} + \frac{1}{2} \times \frac{1}{6}}$

= $\frac{\frac{2}{5}}{\frac{2}{5} + \frac{1}{6}}$

= $\frac{\frac{2}{5}}{\frac{17}{30}}$

= 12/17

(ii) P(E₂/A) = $\frac{P\left( E_1 \right)P\left( A/ E_1 \right)}{P\left( E_1 \right)P\left( A/ E_1 \right) + P\left( E_2 \right)P\left( A/ E_2 \right)}$

= $\frac{\frac{1}{2} \times \frac{1}{6}}{\frac{1}{2} \times \frac{2}{5} + \frac{1}{2} \times \frac{1}{6}}$

= $\frac{\frac{1}{6}}{\frac{2}{5} + \frac{1}{6}}$

= $\frac{\frac{1}{6}}{\frac{17}{30}}$

= 5/17

问题 9. 在一个班级中，5% 的男生和 10% 的女生智商超过 150。在这个班级中，60% 的学生是男生。如果随机选择一个学生，发现其 IQ 超过 150，则求该学生是男孩的概率。

解决方案：

Let us consider A, E₁ and E₂ be the events that the IQ is more than 150, the selected student is a boy and the selected student is a girl.

So, P(E₁) = 60/100

P(E₂) = 40/100

Now,

P(A/E₁) = 5/100

P(A/E₂) = 10/100

So, using Bayes’ theorem, the required probability is,

P(E₁/A) = $\frac{P\left( E_1 \right)P\left( A/ E_1 \right)}{P\left( E_1 \right)P\left( A/ E_1 \right) + P\left( E_2 \right)P\left( A/ E_2 \right)}$

= $\frac{\frac{60}{100} \times \frac{5}{100}}{\frac{60}{100} \times \frac{5}{100} + \frac{40}{100} \times \frac{10}{100}}$

= $\frac{300}{300 + 400}$

= 300/700

= 3/7

问题 10. 一家工厂有三台机器 X、Y 和 Z，每天分别生产 1000、2000 和 3000 个螺栓。机器 X 生产 1% 的缺陷螺栓，Y 生产 1.5% 和 Z 生产 2% 的缺陷螺栓。一天结束时，随机抽出一个螺栓，发现有缺陷。这个有缺陷的螺栓由机器 X 生产的概率是多少？

解决方案：

Let us assume that E₁, E₂ and E₃ be the events that machine X produces bolts, machine Y produces bolts and machine Z produces bolts. Also, A be the event that the bolt is defective.

So, the total number of bolts = 1000 + 2000 + 3000 = 6000

P(E₁) = 1000/6000 = 1/6

P(E₂) = 2000/6000 = 1/3

P(E₃) = 3000/6000 = 1/2

Now, P(E₁/A) is the probability that the defective bolt is produced by machine X

So, 

P(A/E₁) = 1% = 1/100

P(A/E₂) = 1.5% = 15/1000

P(A/E₃) = 2% = 2/100

So, using Bayes’ theorem, the required probability is,

P(E₁/A) = $\frac{P\left( E_1 \right)P\left( A/ E_1 \right)}{P\left( E_1 \right)P\left( A/ E_1 \right) + P\left( E_2 \right)P\left( A/ E_2 \right) + P\left( E_2 \right)P\left( A/ E_2 \right)}$

= $\frac{\frac{1}{6} \times \frac{1}{100}}{\frac{1}{6} \times \frac{1}{100} + \frac{1}{3} \times \frac{15}{1000} + \frac{1}{2} \times \frac{2}{100}}$

= $\frac{\frac{1}{6}}{\frac{1}{6} + \frac{1}{2} + 1}$

= $\frac{\frac{1}{6}}{\frac{1 + 3 + 6}{6}}$

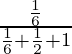
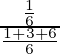
= 1/10

问题 11. 一家保险公司为 3000 辆踏板车、4000 辆汽车和 5000 辆卡车投保。涉及踏板车、汽车和卡车的事故概率分别为 0.02、0.03 和 0.04。其中一辆被保险车辆发生事故。找出它是 (i) 踏板车 (ii) 汽车 (iii) 卡车的概率。

解决方案：

Let us assume that E₁, E₂ and E₃ be the events that the vehicle is a scooter, a car and a truck. Also, A be the event that the vehicle meets with an accident.

From the question, insurance company insured 3000 scooters, 4000 cars and 5000 trucks.

So, the total number of vehicles = 3000 + 4000 + 5000 = 12000

P(E₁) = 3000/12000 = 1/4

P(E₂) = 4000/12000 = 1/3

P(E₃) = 5000/12000 = 5/12

Now, P(E₁/A) is the probability that the vehicle, which meets with an accident, is a scooter 

Now,

P(A/E₁) = 0.02 = 2/100

P(A/E₂) = 0.03 = 3/100

P(A/E₃) = 0.04 = 4/100

So, using Bayes’ theorem, the required probability is,

(i) P(E₁/A) = $\frac{P\left( E_1 \right)P\left( A/ E_1 \right)}{P\left( E_1 \right)P\left( A/ E_1 \right) + P\left( E_2 \right)P\left( A/ E_2 \right) + P\left( E_3 \right)P\left( A/ E_3 \right)}$

= $\frac{\frac{1}{4} \times \frac{2}{100}}{\frac{1}{4} \times \frac{2}{100} + \frac{1}{3} \times \frac{3}{100} + \frac{5}{12} \times \frac{4}{100}}$

= $\frac{\frac{1}{2}}{\frac{1}{2} + 1 + \frac{5}{3}}$

= $\frac{\frac{1}{2}}{\frac{3 + 6 + 10}{6}}$

= 3/19

(ii) P(E₂/A) = $\frac{P\left( E_2 \right)P\left( A/ E_2 \right)}{P\left( E_1 \right)P\left( A/ E_1 \right) + P\left( E_2 \right)P\left( A/ E_2 \right) + P\left( E_3 \right)P\left( A/ E_3 \right)}$

= $\frac{\frac{1}{3} \times \frac{3}{100}}{\frac{1}{4} \times \frac{2}{100} + \frac{1}{3} \times \frac{3}{100} + \frac{5}{12} \times \frac{4}{100}}$

= $\frac{1}{\frac{1}{2} + 1 + \frac{5}{3}}$

= $\frac{1}{\frac{3 + 6 + 10}{6}}$

= 6/19

(iii) P(E₃/A) = $\frac{P\left( E_3 \right)P\left( A/ E_3 \right)}{P\left( E_1 \right)P\left( A/ E_1 \right) + P\left( E_2 \right)P\left( A/ E_2 \right) + P\left( E_3 \right)P\left( A/ E_3 \right)}$

= $\frac{\frac{5}{12} \times \frac{4}{100}}{\frac{1}{4} \times \frac{2}{100} + \frac{1}{3} \times \frac{3}{100} + \frac{5}{12} \times \frac{4}{100}}$

= $\frac{\frac{5}{3}}{\frac{1}{2} + 1 + \frac{5}{3}}$

= $\frac{\frac{5}{3}}{\frac{3 + 6 + 10}{6}}$

= 10/19

问题 12。假设我们有四个盒子 A、B、C、D，其中包含如下所示的彩色弹珠：

Box	Colour
	Red	White	Black
A	1	6	3
B	6	2	2
C	8	1	1
D	0	6	4

其中一个盒子是随机选择的，并从中抽出一个弹珠。如果弹珠是红色的，它是从盒子 A、盒子 B 和盒子 C 中抽出来的概率是多少？

解决方案：

Let us assume that R be the event of drawing the red marble and E_A, E_B and E_C be the events of selecting box A, box B and box C.

According to the question, the total number of marbles are 40 and the number of red marbles are 15.

So, P(R) = 15/40 = 3/8

P(E_A/R) = The probability of drawing a red marble from box A.

P(E_A/R) = $\frac{P\left( E_A \cap R \right)}{P\left( R \right)}$

= $\frac{\frac{1}{40}}{\frac{3}{8}}$

= 1/15

P(E_B/R) = The probability of drawing a red marble from box B.

P(E_B/R \right) = $\frac{P\left( E_B \cap R \right)}{P\left( R \right)}$

= $\frac{\frac{6}{40}}{\frac{3}{8}}$

= 2/5

P(E_C/R) = The probability of drawing a red marble from box C.

P(E_C/R) = $\frac{P\left( E_C \cap R \right)}{P\left( R \right)}$

= $\frac{\frac{8}{40}}{\frac{3}{8}}$

= 8/15

问题 13. 一家制造商有三个机器运算符A、B 和 C。第一个运算符A 生产 1% 的缺陷品，而另外两个运算符B 和 C 分别生产 5% 和 7% 的缺陷品。 A 有 50% 的时间在工作，B 有 30% 的时间在工作，C 有 20% 的时间在工作。生产了有缺陷的项目。它由 A 产生的概率是多少？

解决方案：

Let us consider E₁, E₂ and E₃ be the time taken by machine operators A, B, and C. Also, X be the event of producing defective items.

P(E₁) = 50 % = 1/2

P(E₂) = 30 % = 3/10

P(E₃) = 20 % = 1/5

Now,

P(X/E₁) = 1 % = 1/100

P(X/E₂) = 5 % = 5/100

P(X/E₃) = 7 % = 7/100

So, using Bayes’ theorem, the required probability is,

P(E₁/X) = $\frac{P\left( E_1 \right)P\left( X/ E_1 \right)}{P\left( E_1 \right)P\left( X/ E_1 \right) + P\left( E_2 \right)P\left( X/ E_2 \right) + P\left( E_3 \right)P\left( X/ E_3 \right)}$

= $\frac{\frac{1}{2} \times \frac{1}{100}}{\frac{1}{2} \times \frac{1}{100} + \frac{3}{10} \times \frac{5}{100} + \frac{1}{5} \times \frac{7}{100}}$

= 5/34