第 12 类 RD Sharma 解决方案 – 第 20 章定积分 – 练习 20.5 |设置 3
将以下定积分计算为和的极限:
问题 23。 ![由 QuickLaTeX.com 渲染 \int_{0}^{4}(x+e^{2x})dx]()
解决方案:
We have,
I =
We know,
![]() , where h =
, where h =![]()
Here a = 0, b = 4 and f(x) = x + e2x.
=> h = 4/n
=> nh = 4
So, we get,
I =![]()
=![]()
=
=![]()
=![]()
=![Rendered by QuickLaTeX.com \lim_{h\to0}h^2\left[(\frac{n(n-1)}{2})+\left(\frac{e^{8}-1}{2(\frac{e^{2h}-1}{h})}\right)\right]](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_20_Definite_Integrals_%E2%80%93_Exercise_20.5_%7C_Set_3_9.jpg)
Now if h −> 0, then n −> ∞. So, we have,
=
=
=![]()
=
Therefore, the value ofas limit of sum is
.
问题 24。 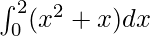
解决方案:
We have,
I =![]()
We know,
![]() , where h =
, where h =![]()
Here a = 0, b = 2 and f(x) = x2 + x.
=> h = 2/n
=> nh = 2
So, we get,
I =![]()
=
=
=![]()
Now if h −> 0, then n −> ∞. So, we have,
=
=
=![]()
=![]()
Therefore, the value of![]() as limit of sum is
as limit of sum is.
问题 25。 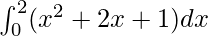
解决方案:
We have,
I =![]()
We know,
![]() , where h =
, where h =![]()
Here a = 0, b = 2 and f(x) = x2 + 2x + 1.
=> h = 2/n
=> nh = 2
So, we get,
I =![]()
=![]()
=![]()
=![]()
Now if h −> 0, then n −> ∞. So, we have,
=![]()
=![]()
=
=
Therefore, the value ofas limit of sum is
![]() .
.
问题 26。 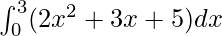
解决方案:
We have,
I =![]()
We know,
![]() , where h =
, where h =![]()
Here a = 0, b = 3 and f(x) = 2x2 + 3x + 5.
=> h = 3/n
=> nh = 3
So, we get,
I =![]()
=![Rendered by QuickLaTeX.com \lim_{h\to0}h[5+(2h^2+3h+5)+[2(2h)^2+3(2h)+5]+...+[2(n-1)^2h^2+3((n-1)h)+5]]](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_20_Definite_Integrals_%E2%80%93_Exercise_20.5_%7C_Set_3_49.jpg)
=![]()
=![]()
Now if h −> 0, then n −> ∞. So, we have,
=
=![]()
= 15 + 18 +![]()
=
Therefore, the value of![]() as limit of sum is
as limit of sum is![]() .
.
问题 27。 ![由 QuickLaTeX.com 渲染 \int_{a}^{b}xdx]()
解决方案:
We have,
I =
We know,
![]() , where h =
, where h =![]()
Here a = a, b = b and f(x) = x.
=> h =![]()
=> nh = b − a
So, we get,
I =
=
=
=
Now if h −> 0, then n −> ∞. So, we have,
=![]()
=
=![]()
=![]()
=![]()
=
Therefore, the value of![]() as limit of sum is
as limit of sum is.
问题 28。 ![由 QuickLaTeX.com 渲染 \int_{0}^{5}(x+1)dx]()
解决方案:
We have,
I =
We know,
![]() , where h =
, where h =![]()
Here a = 0, b = 5 and f(x) = x + 1.
=> h =5/n
=> nh = 5
So, we get,
I =![]()
=![]()
=
=![]()
Now if h −> 0, then n −> ∞. So, we have,
=![]()
=![]()
= 5 +![]()
=![]()
Therefore, the value of![]() as limit of sum is
as limit of sum is![]() .
.
问题 29。 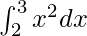
解决方案:
We have,
I =![]()
We know,
![]() , where h =
, where h =![]()
Here a = 2, b = 3 and f(x) = x2.
=> h = 1/n
=> nh = 1
So, we get,
I =![]()
=![]()
=![]()
=![]()
=![]()
Now if h −> 0, then n −> ∞. So, we have,
=![]()
=![]()
=![]()
=![]()
=![]()
Therefore, the value of![]() as limit of sum is
as limit of sum is![]() .
.
问题 30。 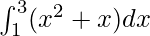
解决方案:
We have,
I =![]()
We know,
![]() , where h =
, where h =![]()
Here a = 1, b = 3 and f(x) = x2 + x.
=> h = 2/n
=> nh = 2
So, we get,
I =![]()
=![]()
=![]()
=![]()
Now if h −> 0, then n −> ∞. So, we have,
=![]()
=![]()
=![]()
=![]()
Therefore, the value of![]() as limit of sum is
as limit of sum is![]() .
.
问题 31。 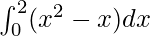
解决方案:
We have,
I =![]()
We know,
![]() , where h =
, where h =![]()
Here a = 0, b = 2 and f(x) = x2 − x.
=> h = 2/n
=> nh = 2
So, we get,
I =![]()
=![]()
=![]()
=![]()
Now if h −> 0, then n −> ∞. So, we have,
=![]()
=![]()
=![]()
=![]()
Therefore, the value of![]() as limit of sum is
as limit of sum is![]() .
.
问题 32。 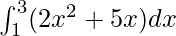
解决方案:
We have,
I =![]()
We know,
![]() , where h =
, where h =![]()
Here a = 1, b = 3 and f(x) = 2x2 + 5x.
=> h = 2/n
=> nh = 2
So, we get,
I =![]()
=![]()
=![]()
=![]()
Now if h −> 0, then n −> ∞. So, we have,
=![]()
=![]()
= 14 + 18 +![]()
=![]()
Therefore, the value of![]() as limit of sum is
as limit of sum is![]() .
.
问题 33。 
解决方案:
We have,
I =![]()
We know,
![]() , where h =
, where h =![]()
Here a = 1, b = 3 and f(x) = 3x2 + 1.
=> h = 2/n
=> nh = 2
So, we get,
I =![]()
=![]()
=![]()
=![]()
Now if h −> 0, then n −> ∞. So, we have,
=![]()
=![]()
= 8 + 12 + 8
= 28
Therefore, the value of![]() as limit of sum is 28.
as limit of sum is 28.