第 12 类 RD Sharma 解决方案 - 第 29 章飞机 - 练习 29.11 |设置 1

问题1.求直线之间的角度$\vec{r}=(2\hat{i}+3\hat{j}+9\hat{k})+λ(2\hat{i}+3\hat{j}+4\hat{k})$和飞机$\vec{r}.(\hat{i}+\hat{j}+\hat{k})=5$ .

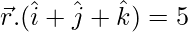解决方案：

Given that, the line is $\vec{r}=(2\hat{i}+3\hat{j}+9\hat{k})+λ(2\hat{i}+3\hat{j}+4\hat{k})$    and the plane is $\vec{r}.(\hat{i}+\hat{j}+\hat{k})=5$.

So, 

As we know that the angle between a line and a plane is 

$sin\theta=\frac{a_1a_2 + b_1b_2 + c_1c_2}{\sqrt{a_1^2 + b_1^2+c_1^2}{\sqrt{a_2^2 + b_2^2+c_2^2}}}$

So,

⇒ $sin\theta=\frac{2+3+4}{\sqrt{1+1+1}{\sqrt{4+9+16}}}$

⇒ 9/√87

问题 2. 求直线之间的夹角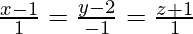$\frac{x-1}{1}=\frac{y-2}{-1}=\frac{z+1}{1}$平面 2x + y – z = 4。

解决方案：

The given line $\frac{x-1}{1}=\frac{y-2}{-1}=\frac{z+1}{1}$   is parallel to the vector $\vec{b}=\hat{i}-\hat{j}+\hat{k}$    and the plane 2x + y – z = 4 is normal to the vector $\vec{n}=2\hat{i}+\hat{j}-\hat{k}$.

So, the angle between line and plane is

$sin\theta=\frac{\vec{b}.\vec{n}}{|\vec{b}||\vec{n}|}$

= $\frac{(\hat{i}-\hat{j}+\hat{k}).(2\hat{i}+\hat{j}-\hat{k})}{|\hat{i}-\hat{j}+\hat{k}||2\hat{i}+\hat{j}-\hat{k}|}$

= 0

问题 3. 求连接点 (3, -4, -2) 和 (12, 2, 0) 的线与平面 3x – y + z = 1 之间的角度。

解决方案：

According to the question, the line passes through A(3,- 4,- 2) and B(12, 2, 0).

So, $\vec{b}=\overrightarrow{AB}=\overrightarrow{OB}-\overrightarrow{OA}$

= $12\hat{i}+2\hat{j}+0\hat{k}-(3\hat{i}-4\hat{j}-2\hat{k})$

= $9\hat{i}+6\hat{j}+2\hat{k}$

So, the line is parallel to the vector $\vec{b}=9\hat{i}+6\hat{j}+2\hat{k}$    and the plane is normal to the vector $\vec{n}=3\hat{i}-\hat{j}+\hat{k}$    

So, the angle between the line and the plane is,

$sin\theta=\frac{\vec{b}.\vec{n}}{|\vec{b}||\vec{n}|}$

= $\frac{(9\hat{i}+6\hat{j}+2\hat{k}).(3\hat{i}-\hat{j}+\hat{k})}{|9\hat{i}+6\hat{j}+2\hat{k}||3\hat{i}-\hat{j}+\hat{k}|}$

= $\frac{23}{11\sqrt11}$

Thus, $\theta=sin^{-1}(\frac{23}{11\sqrt{11}})$    .

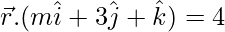问题 4. 线路$\vec{r}=\hat{i}+λ(2\hat{i}-m\hat{j}-3\hat{k})$平行于平面$\vec{r}.(m\hat{i}+3\hat{j}+\hat{k})=4$ .找米。

解决方案：

Give that the equation of line is $\vec{r}=\hat{i}+λ(2\hat{i}-m\hat{j}-3\hat{k})$    and the equation of plane is $\vec{r}.(m\hat{i}+3\hat{j}+\hat{k})=4$   

So, $\vec{b} = (2\hat{i} - m\hat{j} - 3\hat{k})$

$\vec{n}=(m\hat{i}+3\hat{j}+\hat{k})$

If a line is parallel to a plane, then the normal to the plane is perpendicular to the line.

⇒ $\vec{b}⊥\vec{n}$

⇒ $\vec{b}.\vec{n}=0$

⇒ $(2\hat{i}-m\hat{j}-3\hat{k}).(m\hat{i}+3\hat{j}+\hat{k})=0$

⇒ 2m – 3m – 3 = 0

⇒ – m – 3 = 0

⇒ m = –3

问题 5. 证明向量方程为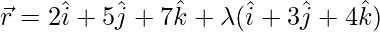$\vec{r}=2\hat{i}+5\hat{j}+7\hat{k}+\lambda(\hat{i}+3\hat{j}+4\hat{k})$平行于其矢量的平面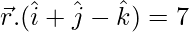$\vec{r}.( \hat{i}+\hat{j}-\hat{k})=7$ .另外，找出它们之间的距离。

解决方案：

Given that the plane passes through the point with the position vector $\vec{a}=2\hat{i}+5\hat{j}+7\hat{k}$    and is parallel to the vector $\vec{b}=\hat{i}+3\hat{j}+4\hat{k}$.

So, the normal vector $\vec{n}=\hat{i} +\hat{j}-\hat{k}$    and d = 7 .

$\vec{b}.\vec{n}=(\hat{i}+3\hat{j}+4\hat{k}).(\hat{i}+\hat{j}-\hat{k})$

= 1 + 3 – 4

= 4 – 4

= 0

As we know that $\vec{b}$    is perpendicular to $\vec{n}$   

So, the distance between the line and plane is 

$d =\frac{|\vec{a}.\vec{n}-d|}{|\vec{n}}$

= 7/√3units.

问题 6. 求通过原点垂直于平面的直线的矢量方程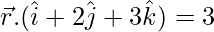$\vec{r}.(\hat{i}+2\hat{j}+3\hat{k})=3$ .

解决方案：

Given that the line is perpendicular to the plane $\vec{r}.(\hat{i}+2\hat{j}+3\hat{k})=3$

So,  the line is parallel to the normal $\hat{i}+2\hat{j}+3\hat{k}$    . 

As we know that the equation of a line is pass through $\vec{a}$  and parallel to $\vec{b}$  is $\vec{r}=\vec{a}+\lambda\hat{n}$

⇒ $\vec{r} = 0\hat{i}+0\hat{j}+0\hat{k}+\lambda(\hat{i}-2\hat{j}+3\hat{k})$

⇒ $\vec{r}=\lambda(\hat{i}-2\hat{j}+3\hat{k})$

问题 7. 求平面通过 (2, 3, -4) 和 (1, -1, 3) 并平行于 x 轴的方程。

解决方案：

Given that the equation of the plane through point (2, 3, -4) is 

a(x − 2) + b(y − 3)+c(z + 4) = 0           …(1)

Since this plane passes through point (1, -1, 3).

⇒ a(1 − 2) + b( −1 − 3) + c( 3 + 4) = 0

⇒ − a − 4b + 7c = 0                             …(2)

Equation(1) is parallel to x-axis and is perpendicular to the yz-plane whose equation is x = 0 or 1 . x + 0 . y + 0 . z = 0

⇒ a(1) + b(0) + c(0) = 0     …(3)

One solving eq(1), (2), and (3), we get

$\begin{vmatrix}x - 3 & y - 3 & z + 4 \\ - 1 & - 4 & 7 \\ 1 & 0 & 0\end{vmatrix} = 0$

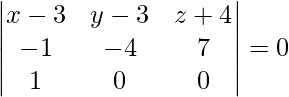
⇒ 7y + 4z – 5 = 0 is the required equation.

问题 8. 求通过点 (0, 0, 0) 和 (3, -1, 2) 并平行于直线的平面方程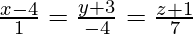$\frac{x-4}{1}=\frac{y+3}{-4}=\frac{z+1}{7}$

解决方案：

Given that the plane pass through point(0, 0, 0), so the equation of the plane is a(x − 0) + b(y − 0)+c(z + 0) = 0.

⇒ ax + by + cz = 0         …(1)

and the same plane passes through point (3, -1, 2). So, the equation of the plane is

3a – b + 2c = 0              …(2)

Equation(1) is parallel to the given line so, 

a(1) + b(-4) + c (7) = 0     …(3)

On solving eq(1), (2), and (3), we get

$\begin{vmatrix}x & y & z \\ 3 & - 1 & 2 \\ 1 & - 4 & 7\end{vmatrix} = 0$

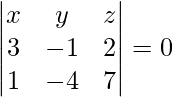
⇒ x – 19y – 11z = 0 

Hence, the required equation of the plane is x – 19y – 11z = 0 

问题 9. 求通过 (1, 2, 3) 并平行于平面的直线的向量和笛卡尔方程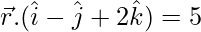$\vec{r}.(\hat{i}-\hat{j}+2\hat{k})=5$和$\vec{r}.(3\hat{i}+\hat{j}+2\hat{k})=6.$

解决方案：

Given that the equation of the line passing through (1, 2, 3) is 

$\frac{(x - 1)}{a}=\frac{(y - 2)}{b}=\frac{(z - 3)}{c}$    …(1)

Here, the given line is parallel to plane x – y + 2z = 5

So, a × 1 + b × – 1 + c × 2 = 0

⇒ a – b + 2c = 0     …(3)

Also, the given line is parallel to plane 3x + y + z = 6

So, a × 3 + b × 1 + c × 1 = 0

⇒ 3a + b + c = 0    …(4)

On solving the equation (3) and (4) we get,

⇒ $\frac{a}{(-1 - 2)} = \frac{b}{(6 - 1)} = \frac{c}{(1 + 3)}$

∴ a = – 3k, b = 5k and c = 4k

Now, put the value in the equation(1), we get

$\frac{(x - 1)}{(-3k)} = \frac{(y - 2)}{(5k)} = \frac{(z - 3)}{(4k)}$

Now, multiplying by k we get

The required equation is $\frac{(x - 1}{(-3)} = \frac{(y - 2)}{(5)} = \frac{(z - 3)}{(4)}$

⇒ $\vec{r}=(\hat{i}+2\hat{j}+3\hat{k})+k(-3\hat{i}+5\hat{j}+4\hat{k})$

Hence, the equation of the plane is $\vec{r}=(\hat{i}+2\hat{j}+3\hat{k})+k(-3\hat{i}+5\hat{j}+4\hat{k})$

问题 10. 证明平面 5x + 2y – 4z + 2 = 0 和 2x + 8y + 2z – 1 = 0 的截面线平行于平面 4x – 2y – 5z – 2 = 0。

解决方案：

Let us consider a, b and c be the direction ratios.

So, 

⇒ a + 4b + c = 0

and, 5a + 2b – 4c = 0

On solving the above two equations, we get

$\frac{a}{2+16}=\frac{b}{-4-5}=\frac{c}{20-2}$

⇒ $\frac{a}{2}=\frac{b}{-1}=\frac{c}{2}$

As we know that the line $\frac{x-x_1}{a_1}=\frac{y-y_1}{b_1}=\frac{z-z_1}{c_1}$ is parallel to plan a₂x + b₂y + c₂z + d₂ = 0, when a₁a₂ + b₁b₂ + c₁c₂ = 0

So, the line with direction ratio(a, b, c) is parallel to plane 4x – 2y – 5z – 2 = 0

aa₁ + bb₁ + cc₁ = 0

2(4) + (-1)(-2) + (2)(-5) = 0

Hence proved that the line of section of the given plane is parallel to the given plane.

问题 11. 求通过点 (1, -1, 2) 并垂直于平面 2x – y + 3z – 5 = 0 的直线的向量方程。

解决方案：

Let us consider a, b and c be the direction ratios.

Given that the equation of the line passing through the point (1, -1, 2)

So, 

$\frac{x-1}{a}=\frac{y+1}{b}=\frac{z-2}{c}$   …..(1)

Also, the line is parallel to the normal of the plane. 

⇒ $\frac{a}{2}=\frac{b}{-1}=\frac{c}{3}=\lambda$

⇒ a = 2λ, b = -λ , c = 3λ

Now put all these values in eq(1), we get

$\frac{x-1}{2}=\frac{y+1}{-1}=\frac{z-2}{3}$

So, the line passes through a point whose position vector is $\vec{a} = \hat{i} - \hat{j} + 2\hat{k}$and parallel to $\vec{b} = 2\hat{i} - \hat{j} + 3\hat{k}$

So, $\vec{r}=\vec{a} + \lambda\vec{b}$

⇒ $\vec{r}=(\hat{i}-\hat{j}+2\hat{k})+\lambda(2\hat{i}-\hat{j}+3\hat{k})$is the required equation.

问题 12. 求通过点 (2, 2, -1) 和 (3, 4, 2) 并平行于方向比为 7, 0, 6 的直线的平面方程。

解决方案：

Given that the equation of the plane through the point (2, 2, -1)

a(x – 2) + b(y – 2) + c(z + 1) = 0     ….(1)

Also, the plane passes through points (3, 4, 2)

a(3 – 2) + b(4 – 2)+ c(2 + 1) = 0

or, a + 2b + 3c = 0     …(2)

As we know that the line $\frac{x-x_1}{a_1}=\frac{y-y_1}{b_1}=\frac{z-z_1}{c_1}$ is parallel to plan a₂x + b₂y + c₂z + d₂ = 0, when a₁a₂ + b₁b₂ + c₁c₂ = 0

According to the question, the plane(1) is parallel to the line whose direction ratios are 7, 0, 6

So, 7a + 0b + 6c = 0   …(3)

Now, on solving the equation (1), (2), and (3), we have,

$\begin{vmatrix}x-2 & y-2 & z+1 \\ 1 & 2 & 3 \\ 7 & 0 & 6\end{vmatrix} = 0$

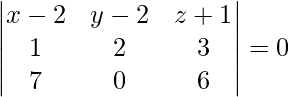
⇒ 12x + 15y – 14z – 68 = 0 is the required equation.

问题 13. 求直线之间的角度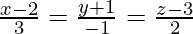$\frac{x-2}{3}=\frac{y+1}{-1}=\frac{z-3}{2}$平面 3x + 4y + z + 5 = 0。

解决方案：

Given that the equation of line is $\frac{x-2}{3}=\frac{y+1}{-1}=\frac{z-3}{2}$ and the equation of the plane is 3x + 4y + z + 5 = 0

So, $\vec{b} = 3\hat{i} - \hat{j} + 2\hat{k}$

$\vec{b} = 3\hat{i} - \hat{j} + 2\hat{k}$

Angle between a line and a plane is 

$sinθ=\frac{\vec{b}.\vec{n}}{|\vec{b}||\vec{n}|}$

⇒ $sinθ=\frac{(3\hat{i}-\hat{j}+2\hat{k}).(3\hat{i}+4\hat{j}+\hat{k})}{|3\hat{i}-\hat{j}+2\hat{k}||3\hat{i}+4\hat{j}+\hat{k}|}$

⇒ $sinθ=\frac{9-4+2}{\sqrt{9+1+4}\sqrt{9+16+1}}$

⇒ $sinθ=\sqrt{\frac{7}{52}}$

Thus, $θ=sin^{-1}(\sqrt{\frac{7}{52}})$