第 12 课 RD Sharma 解决方案 - 第 29 章飞机 - 练习 29.8

问题 1. 求平行于 2x – 3y + z = 0 并通过点 (1, –1, 2) 的平面方程。

解决方案：

We know that the equation of a plane parallel to 2x – 3y + z = 0 is given by:

2x – 3y + z + λ = 0 

Since the plane passes through the point (1, –1, 2), we have:

2(1) – 3(–1) + 2 + λ = 0 

⇒ λ = –7

On substituting the value of  λ in the equation, we have:

2x – 3y + z + (-7) = 0 

2x – 3y + z – 7= 0 is the required equation.

问题 2. 通过 (3, 4, –1) 求与平面平行的平面方程$\vec{r}.(2\hat{i}-3\hat{j}+5\hat{k})+2=0.$

解决方案：

The given plane passes through the vector $3\hat{i}+4\hat{j}-\hat{k}$. Thus,

$(3\hat{i}+4\hat{j}-\hat{k}).(2\hat{i}-3\hat{j}+5\hat{k})+ λ=0.$

(3)(2) + (4)(-3) + (-1)(5) + λ = 0

⇒  λ = 11

On substituting the value of  λ in the equation, we have:

$\vec{r}.(2\hat{i}-3\hat{j}+5\hat{k})+11=0$is the required equation.

问题 3. 求平面通过平面 2x – 7y + 4z – 3 = 0 和 3x – 5y + 4z + 11 = 0 的交线与点 (–2, 1, 3) 的方程。

解决方案：

The equation of the plane passing through the line of intersection of the given planes is:
(2x – 7y + 4z – 3) + λ(3x – 5y + 4z + 11) = 0

⇒ x(2 + 3λ) + y(–7 – 5λ) + z(4 + 4λ) – 3 + 11λ = 0

Also, since the plane passes through the point (–2, 1, 3), we have:

(–2)(2 + 3λ) + (1)(–7 – 5λ) + (3)(4 + 4λ) – 3 + 11λ = 0

⇒ λ = 1/6 

On substituting the value of  λ in the equation, we have:

x(2 + 3(1/6)) + y(–7 – 5(1/6)) + z(4 + 4(1/6)) – 3 + 11(1/6) = 0

15x – 47y + 28z = 7 is the required equation.

问题 4. 求平面通过该点的方程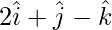$2\hat{i}+\hat{j}-\hat{k}$并通过平面的交线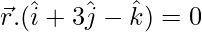$\vec{r}.(\hat{i}+3\hat{j}-\hat{k})=0$和$\vec{r}.(\hat{j}+\hat{k})=0.$

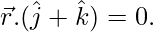
解决方案：

The equation of the plane passing through the line of intersection of the given planes is:

$\vec{r}.[(\hat{i}+3\hat{j}-\hat{k})+λ(\hat{j}+2\hat{k})]=0$

Also, since the plane passes through point $2\hat{i}+\hat{j}-\hat{k}$, we have:

$(2\hat{i}+\hat{j}-\hat{k})(\hat{i}+3\hat{j}-\hat{k})+λ(2\hat{i}+\hat{j}-\hat{k})(\hat{j}+2\hat{k})=0$

⇒  λ = 6

On substituting the value of  λ in the equation, we have:

$\vec{r}.(\hat{i}+9\hat{j}+11\hat{k})=0$is the required equation.

问题 5. 求通过 2x – y = 0 和 3z – y = 0 的交点并垂直于 4x + 5y – 3z = 8 的平面方程。

解决方案：

The equation of the plane passing through the line of intersection of the given planes is:

2x – y + λ(3z – y) = 0

⇒ 2x + y(–1 – λ) + z(3λ) = 0

Since the planes are perpendicular, we have:

2(4) + (–5)(–1 – λ) + (–3)(3λ) = 0

⇒ λ = 3/14 

On substituting the value of  λ in the equation, we have:

2x + y(–1 – 3/14) + z(3(3/14)) = 0

28x – 17y + 9z = 0 is the required equation.

问题 6. 求平面方程，其中包含平面 x + 2y + 3z – 4 = 0 和 2x + y – z + 5 = 0 的交线并垂直于平面 5x + 3y – 6z + 8 = 0。

解决方案：

The equation of the plane passing through the line of intersection of the given planes is:

x + 2y + 3z – 4 + λ(2x + y – z + 5) = 0

⇒ x(1 + 2λ) + y(2 + λ) + z(3 – λ) – 4 + 5λ = 0

Since the planes are perpendicular, we have:

5(1 + 2λ) + 3(2 + λ) + (–6)(3 – λ) = 0

⇒  λ = 7/19

On substituting the value of  λ in the equation, we have:

x(1 + 2(7/19)) + y(2 + 7/19) + z(3 – 7/19) – 4 + 5(7/19) = 0

33x + 45y + 50z – 41 = 0 is the required equation.

问题 7. 求平面通过平面 x + 2y + 3z + 4 = 0 和 x – y + z + 3 = 0 的交线并通过原点的方程。

解决方案：

The equation of the plane passing through the line of intersection of the given planes is:

x + 2y + 3z + 4 + λ(x – y + z + 3) = 0

⇒ x(1 + λ) + y(2 – λ) + z(3 + λ) + 4 + 3λ = 0

Also, since the plane passes through the origin, we have:

0(1 + λ) + 0(2 – λ) + 0(3 + λ) + 4 + 3λ = 0

⇒ λ = -4/3 

On substituting the value of  λ in the equation, we have:

 x(1 + (-4/3)) + y(2 – (-4/3)) + z(3 + (-4/3)) + 4 + 3(-4/3) = 0

x – 10y – 5z = 0 is the required equation.

问题 8. 找到平面的标量积形式的矢量方程，该平面包含平面 x – 3y + 2z – 5 = 0 和 2x – y + 3z – 1 = 0 的交线并通过 (1, –2, 3）。

解决方案：

The equation of the plane passing through the line of intersection of the given planes is:

x – 3y + 2z – 5 + λ(2x – y + 3z – 1) = 0

⇒ x(1 + 2λ) + y(–3 – λ) + z(2 + 3λ) – 5 – λ = 0

Also, since the plane passes through the origin, we have:

1(1 + 2λ) + (–2)(–3 – λ) + 3(2 + 3λ) – 5 – λ = 0

⇒  λ = -2/3

On substituting the value of  λ in the equation, we have:

x(1 + 2(-2/3)) + y(–3 – (-2/3)) + z(2 + 3(-2/3)) – 5 – (-2/3) = 0

$\vec{r}.(\hat{i}+7\hat{j})+13=0$is the required equation.

问题 9. 找到包含平面 x + 2y + 3z – 4 = 0 和 2x + y – z + 5 = 0 的交线并垂直于平面 5x + 3y + 6z + 8 = 的平面方程0。

解决方案：

The equation of the plane passing through the line of intersection of the given planes is:

x + 2y + 3z – 4 + λ(2x + y – z + 5) = 0

⇒ x(1 + 2λ) + y(2 + λ) + z(3 – λ) – 4 + 5λ = 0

We know that two planes are perpendicular when $a_1a_2+b_1b_2+c_1c_2=0.$

⇒ 5(1 + 2λ) + 3(2 + λ) + 6(3 – λ) = 0

⇒ λ = -29/7

On substituting the value of  λ in the equation, we have:

x(1 + 2(-29/7)) + y(2 + (-29/7)) + z(3 – (-29/7)) – 4 + 5(-29/7) = 0

51x + 15y – 50z + 173 = 0 is the required equation.

问题 10. 求平面通过平面交线的方程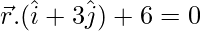$\vec{r}.(\hat{i}+3\hat{j})+6=0$和$\vec{r}.(3\hat{i}-\hat{j}-4\hat{k})=0$并且距离原点有一个单位距离。

解决方案：

The equation of the plane passing through the line of intersection of the given planes is:

x(1 + 3λ) + y(3 + λ) – 4zλ + 6 = 0

Distance from plane to the origin = 1

⇒ $|\frac{6}{\sqrt{(1+3λ )^2+(3-λ)^2+(4λ)^2}}|=1$

⇒ λ = ±1

Hence, 4x + 2y – 4z + 6 = 0 and –2x + 2y + 4z + 6 = 0 are the required equations.

问题 11. 求通过平面 2x + 3y – z + 1 = 0 和 x + y – 2z + 3 = 0 并垂直于平面 3x – 2y – z – 4 = 的交线的平面方程0。

解决方案：

The equation of the plane passing through the line of intersection of the given planes is:

2x + 3y – z + 1  + λ(x + y – 2z + 3) = 0

⇒ x(2 + λ) + y(3 + λ) + z(–1 – 2λ) + 1 + 3λ = 0

We know that two planes are perpendicular when $a_1a_2+b_1b_2+c_1c_2=0.$

⇒ 3(2 + λ) + (–1)(3 + λ) + (–2)(–1 – 2λ) = 0

⇒  λ = -5/6

On substituting the value of  λ in the equation, we have:

x(2 + (-5/6)) + y(3 + (-5/6)) + z(–1 – 2(-5/6)) + 1 + 3(-5/6) = 0

7x + 13y + 4z – 9 = 0 is the required equation.

问题 12. 找到包含平面相交线的平面方程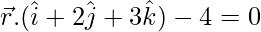$\vec{r}.(\hat{i}+2\hat{j}+3\hat{k})-4=0$和$\vec{r}.(2\hat{i}+\hat{j}-\hat{k})+5=0$并且垂直于平面$\vec{r}.(5\hat{i}+3\hat{j}-6\hat{k})+8=0.$

解决方案：

The equation of the plane passing through the line of intersection of the given planes is:

$\vec{r}.(\hat{i}+2\hat{j}+3\hat{k})-4+λ(\vec{r}.(2\hat{i}+\hat{j}-\hat{k})+5)=0$

⇒ $\vec{r}.[(\hat{i}+2\hat{j}+3\hat{k})+λ(2\hat{i}+\hat{j}-\hat{k})]-4+5λ=0$

We know that two planes are perpendicular if $\vec{n_1}.\vec{n_2}=0$

⇒ $[(\hat{i}+2\hat{j}+3\hat{k})+λ(2\hat{i}+\hat{j}-\hat{k})](5\hat{i}+3\hat{j}-6\hat{k})=0$

⇒ 5(1 + 2λ) + 3(2 + λ) + (–6)(3 – λ) = 0

⇒  λ = 7/19

On substituting the value of  λ in the equation, we have:

33x + 45y + 50z – 41 = 0 is the required equation.

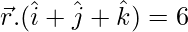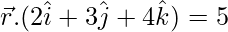问题 13. 求平面通过平面交点的向量方程$\vec{r}.(\hat{i}+\hat{j}+\hat{k})=6$和$\vec{r}.(2\hat{i}+3\hat{j}+4\hat{k})=5$和点 (1, 1, 1)。

解决方案：

The equation of the plane passing through the line of intersection of the given planes is:

$\vec{r}.(\hat{i}+\hat{j}+\hat{k})-6+λ(\vec{r}.(2\hat{i}+3\hat{j}+4\hat{k})-5)=0$

⇒ x(1 + 2λ) + y(1 + 3λ) +z(1 + 4λ) = 6  – 5λ

Also, since the plane passes through the point(1, 1, 1), we have:

1(1 + 2λ) + 1(1 + 3λ) +1(1 + 4λ) = 6  – 5λ

⇒ λ = 3/14

On substituting the value of  λ in the equation, we have:

x(1 + 2(3/14)) + y(1 + 3(3/14)) +z(1 + 4(3/14)) = 6  – 5(3/14)

$\vec{r}.(20\hat{i}+23\hat{j}+26\hat{k})=69$is the required equation.

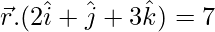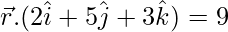问题 14. 求平面通过平面交点的方程$\vec{r}.(2\hat{i}+\hat{j}+3\hat{k})=7$和$\vec{r}.(2\hat{i}+5\hat{j}+3\hat{k})=9$和点 (2, 1, 3)。

解决方案：

The equation of the plane passing through the line of intersection of the given planes is:

$\vec{r}.(2\hat{i}+\hat{j}+3\hat{k})-7+λ(\vec{r}.(2\hat{i}+5\hat{j}+3\hat{k})-9)=0$

⇒ $\vec{r}.[(2+2λ)\hat{i}+(1+5λ)\hat{j}+(3+3λ)\hat{k}]-7-9λ=0$

Also, since the plane passes through the point (2, 1, 3) we have:

9λ = –7

⇒ λ = -7/9 

Substituting the value of  λ in the equation, we have:

$\vec{r}.(2\hat{i}-13\hat{j}+3\hat{k})=0$is the required equation.

问题 15. 求平面通过平面 3x – y + 2z = 4 和 x + y + z = 2 与点 (2, 2, 1) 的交点的方程。

解决方案：

The equation of the plane passing through the line of intersection of the given planes is:

3x – y + 2z – 4  + λ(x + y + z  – 2) = 0

Also, since the plane passes through the point (2, 2, 1), we have:

λ = -2/3

On substituting the value of  λ in the equation, we have:

3x – y + 2z – 4  + (-2/3)(x + y + z  – 2) = 0

7x – 5y + 4z = 0 is the required equation.

问题 16. 通过平面 x + 2y + z = 1 和 2x + 3y + 4z = 5 的交线求平面的矢量方程，该交线垂直于平面 x – y + z = 0。

解决方案：

The equation of the plane passing through the line of intersection of the given planes is:

x + 2y + z – 1  + λ(2x + 3y + 4z – 5) = 0

⇒ x(1 + 2λ) + y(1 + 3λ) +z(1 + 4λ) = 1 + 5λ

We know that two planes are perpendicular when $a_1a_2+b_1b_2+c_1c_2=0.$

⇒ 1(1 + 2λ) + (–1)(1 + 3λ) + 1(1 + 4λ) = 1 + 5λ 

⇒ λ = -1/3 

On substituting the value of  λ in the equation, we have:

x(1 + 2(-1/3)) + y(1 + 3(-1/3)) + z(1 + 4(-1/3)) = 1 + 5(-1/3)

x – z + 2 = 0 is the required equation.

问题 17. 求通过 (a, b, c) 并平行于平面的平面方程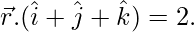$\vec{r}.(\hat{i}+\hat{j}+\hat{k})=2.$

解决方案：

Equation of the family of planes parallel to the given plane = $\vec{r}.(\hat{i}+\hat{j}+\hat{k})=d$

Since the plane passes through (a, b, c), we have:

a + b + c = d

Substituting the above equation in the equation of family of planes we have:

$\vec{r}(\hat{i}+\hat{j}+\hat{k})=a+b+c$

Hence, x + y + z = a + b + c is the required equation of the plane.