第 12 类 RD Sharma 解决方案 - 第 29 章飞机 - 练习 29.13
问题 1. 证明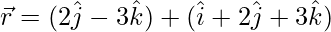 和
和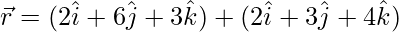 是共面的。此外,找到包含它们的平面方程。
是共面的。此外,找到包含它们的平面方程。
解决方案:
We know, the lines ![]() and
and ![]() are coplanar if:
are coplanar if:
![]()
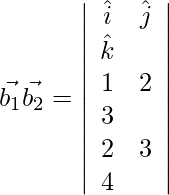
![]()
![]()
![]()
![]()
![]()
Since, ![]() , the lines are coplanar.
, the lines are coplanar.
Equation of the plane containing them: ![]()
![]()
![]()
![]()
问题 2. 显示线条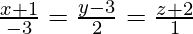 和
和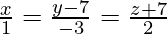 是共面的。此外,找到包含它们的平面方程。
是共面的。此外,找到包含它们的平面方程。
解决方案:
We know the lines ![]() and
and ![]() are coplanar if,
are coplanar if,
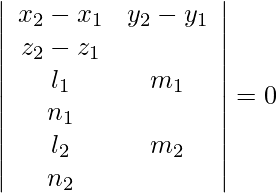
So, 
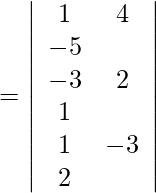
= 1(4 + 3) − 4(−6 − 1) − 5(9 − 2)
= 7 + 28 − 35
= 0.
So the lines are coplanar.
Equation of the plane: 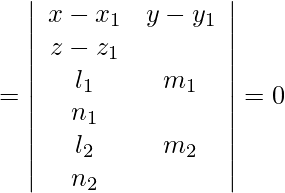
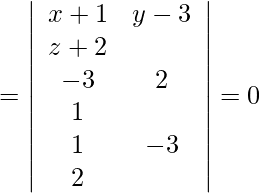
⇒ 7x + 7y + 7z = 0.
问题 3. 求包含直线的平面方程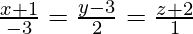 和点 (0,7,-7) 并显示该线
和点 (0,7,-7) 并显示该线 也位于同一平面。
也位于同一平面。
解决方案:
We know the equation of a plane passing through a point (x1,y1,z1) is given by
a(x−x1) + b(y−y1) + c(z−z1) = 0 ……..(1)
Since the required plane passes through (0,7,-7), the equation becomes
ax + b(y − 7) + c(z + 7) = 0 …….(2)
It also contains ![]() and point is (−1,3,−2).
and point is (−1,3,−2).
a(−1) + b(3 − 7) + c(−2 + 7) = 0
⇒ −a − 4b + 5c = 0
Also, −3a + 2b + c = 0
Solving the equations, we get x + y + z = 0
So, ![]() lies on the plane x + y + z = 0.
lies on the plane x + y + z = 0.
问题 4. 找出包含两条平行线的平面方程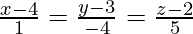 和
和
解决方案:
We know the equation of a plane passing through a point (x1,y1,z1) is given by
a(x−x1) + b(y−y1) + c(z−z1) = 0 ……..(1)
The required plane passes through (4,3,2). Hence,
a(x − 4) + b(y − 3) + c(z − 2) = 0
It also passes through (3,-2,0). Hence,
a(3 − 4) + b(−2 − 3) + c(0 − 2) = 0
⇒ a + 5b + 2c = 0 …….(2)
Also, a − 4b + 5c = 0 ……..(3)
Solving (2) and (3) by cross multiplication, we get the equation of the plane as:
⇒ 11x − y − 3z − 35 = 0.
问题 5. 显示线条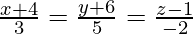 和 3x - 2y + 5 = 0 = 2x + 3y + 4z - 4 相交。求平面方程。
和 3x - 2y + 5 = 0 = 2x + 3y + 4z - 4 相交。求平面方程。
解决方案:
Using a1a2 + b1b2 + c1c2 = 0, we get
3a − 2b + c = 0 ….(1)
Also, 2a + 3b + 4c = 0. ….(2)
Solving (1) and (2) by cross multiplication, we have
![]()
![]()
Hence, the equation of the plane is 45x − 17y + 25z + 53 = 0
and the point of intersection is (2,4,−3).
问题 6. 证明向量方程为的平面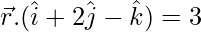 包含其矢量方程为的线
包含其矢量方程为的线
解决方案:
Here, ![]()
= 2(1) +1(2) + 4(−1)
![]()
Now, ![]()
= 1(1) + 1(2) + 0(−1)
= 3
![]()
Hence, the given line lies on the plane.
问题 7. 求直线的交点确定的平面方程 和
和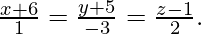
解决方案:
Let the plane be ax + by + cz + d = 0
Since the plane passes through the intersection of the given lines, normal of the plane is perpendicular to the two lines.
⇒ 3a − 2b + 6c = 0
and, a − 3b + 2c = 0
Using cross multiplication, we have
![]()
⇒ ![]()
问题 8. 求通过点 (3,4,2) 和 (7,0,6) 并垂直于平面 2x - 5y - 15 = 0 的平面的矢量方程。同时,证明由此得到的平面包含该行
解决方案:
Let the equation of the plane be ![]()
Since the plane passes through (3,4,2) and (7,0,6), we have
![]() and
and ![]()
Since the required plane is perpendicular to 2x − 5y − 15 = 0, we have,
![]()
⇒ b = 2.5a
Substituting the value of b in the above equations we have,
![]() and
and ![]()
Solving the above equations, we have
a = 17/5, b = 17/2 and c = −17/3.
Substituting the values in the equation of plane, we obtain
5x + 2y − 3z = 17.
Vector equation of the plane becomes: ![]() .
.
问题 9. 如果线条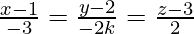 和
和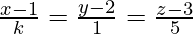 是垂直的,找到 k 的值以及包含这些线的平面的方程。
是垂直的,找到 k 的值以及包含这些线的平面的方程。
解决方案:
The direction ratios of the two lines are r1 = (−3,−2k,2) and r2 = (k,1,5).
Since the lines are perpendicular, we have
(−3,−2k,2).(k,1,5) = 0
⇒ 3k + 2k − 10 = 0
⇒ 5k = 10
⇒ k = 2
Now, equation of the plane containing the lines is: 
⇒ −22x + 19y + 5z + 31 = 0.
问题10.找到线所在点的坐标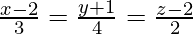 与平面 x - y + z - 5 = 0 相交。另外,求直线与平面之间的夹角。
与平面 x - y + z - 5 = 0 相交。另外,求直线与平面之间的夹角。
解决方案:
Any point on the given line is of the form (3k + 2, 4k − 1, 2k + 2).
We have, (3k + 2) − (4k − 1) + (2k + 2) − 5 = 0
⇒ k = 0.
Thus, the coordinates of the point become (2,−1,2).
Let v be the angle between the line and the plane. Then,
![]()
Here, l = 3, m = 4, n =2, a =1, b = −1, c = 1.
Hence, 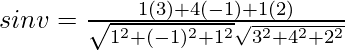
⇒ ![]()
⇒ ![]()
问题 11. 用位置向量求过三点平面的向量方程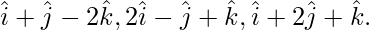
解决方案:
Let A, B and C be the three given vectors respectively.
![]()
![]()
and, ![]()
Now, 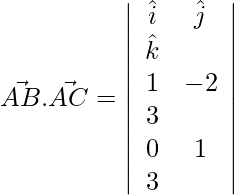
⇒ ![]()
Equation of the plane is: ![]()
Coordinates of the points are (1,1,−2).
问题 12. 显示线条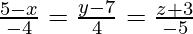 和
和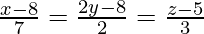 是共面的。
是共面的。
解决方案:
We know the lines ![]() and
and ![]() are coplanar if,
are coplanar if,
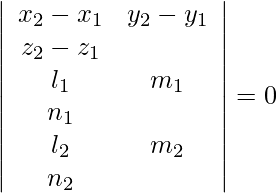
or, 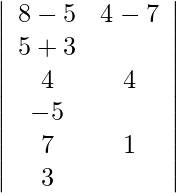
= 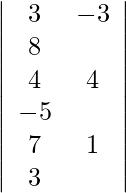
= 3(12 + 5) + 3(12 + 35) + 8(4 − 28)
= 0.
Hence the lines are coplanar.
问题 13. 求通过点 (3,2,0) 并包含直线的平面方程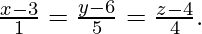
解决方案:
Required equation of the plane passing through (3,2,0) is:
a(x − 3) + b(y − 2) + cz = 0 ……(1)
Since the plane also passes through the given line, we have
4b + 4c = 0 ……(2)
Also, the plane will be parallel so,
a + 5b + 4c = 0 ……(3)
Solving (2) and (3), we have
![]()
⇒ ![]()
⇒ a = −z, b = z and c = −z
Putting the values in (1), we have
x − y + z − 1 = 0.